图像矫正----认识畸变(Distortion)
Distortion
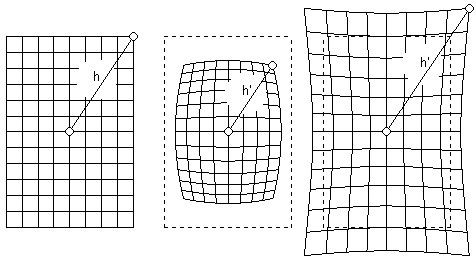
The last of the Seidel aberrations,distortion is the most easily recognized aberration as it deforms the image as whole. Since straight lines in the object space are rendered as curved lines on the film, the namecurvilinear distortion is frequently encountered. Fig. 1 shows the two fundamental manifestations of the aberration, barrel and pincushion distortion. Straight lines in the undistorted subject (left) bulge in the characteristic barrel fashion (middle) or bend inward in the pincushion representation (right). Straight lines running through the image center remain straight and a circle concentric with the image center remains a circle, although its radius isaffected.
Figure 1. Distortion of a rectangular grid. Left: undistorted grid. Middle grid: barrel distortion. Right grid: pincushion distortion.
Origin
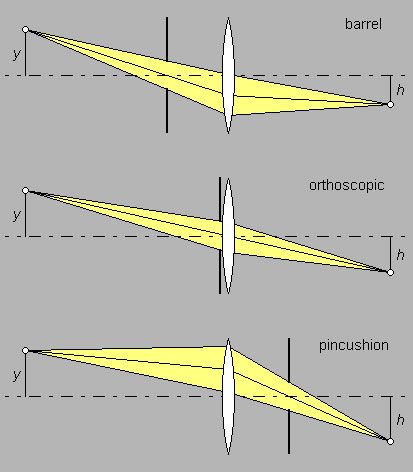
A common cause of distortion is the introduction of a stop in a system of (thin) lenses [1,2], e.g. to reduce spherical aberration or astigmatism. The position of such a stop determines the amount and the sign of the distortion, as illustrated in Fig. 2 for a simple positive lens.
Figure 2. The influence of stop position on distortion. Note that the image size h differs for a constant object size y.
The position of the image point is determined by the chief ray (solid line), which is the ray that passes through the center of the stop. The chief ray is characteristic for the cone of light marked by the margins of the stop. When the stop is located at the lens, the chief ray is the principal ray (i.e., it passes through the optical center) and leaves the lens at the same angle at which it entered. Such a system does not distort the image and is called orthoscopic. With the stop in front or in rear, the chief ray is refracted. The image and object distances, measured along the chief ray, differ from the orthoscopic case. The ratio of these distances determines the image magnification h/y, which is smaller with the stop in front and larger with a rear stop. Straight lines will be distorted when the magnification h/y is a function of the off-axis distancey. The top configuration leads to a situation where h/y decreases toward the image corners: barrel distortion. The bottom configuration leads to a situation where h/y increases toward the image corners: pincushion distortion.
The size of the stop has no effect on the distortion, as the chief ray does not alter its route when the aperture(光圈,孔) is made smaller or larger. To be sure, Fig. 2 cannot be understood in the context of a paraxial theory(近轴理论). In the absence of the stop, the lens in Fig. 2 suffers from spherical aberration(球面像差), coma(模糊), and astigmatism(散光), which result in a blurred image patch for each point in object space.
A complex lens such as a retrofocus wideangle(广角) design tends to exhibit barrel distortion as the front group of elements acts as an aperture stop for the positive rear group [3]. Telephoto lenses have a negative rear group and give rise to pincushion distortion. Distortion is difficult to correct for in zoom lenses(变焦镜头), which usually go from barrel at the wide end to pincushion at the tele end. The aberration is minimized by a symmetrical lens design, which is (near) orthoscopic. The aperture set by the variable leaf diaphragm has no effect on distortion, but is instrumental(helpful) in controlling other aberrations. When a lens is turned around with a reversal ring for the purpose of macro photography, distortion changes sign. Barrel distortion becomes pincushion and vice versa. When a distorted slide is projected with the original picture taking lens in reverse, curvilinear distortion is absent from the projected image. Distortion also depends on the focusing distance. Infinity focus and close focus may yield different amounts of distortion with the same lens.
How to read a distortion graph
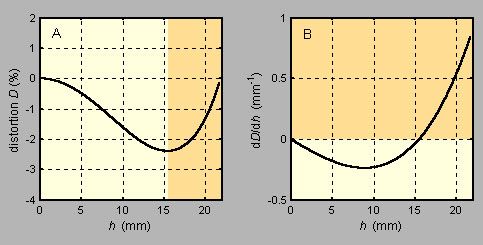
Distortion is measured as the relative change of h compared to its value predicted by the paraxial theory. In Fig. 1 the distance from the image center to the corner of the grid is indicated with h for the orthoscopic case, and with h' for the distorted grids. The relative distortion D is then given by (h'-h)/h. The corner points of the three grids in Fig. 1 are distorted by, from left to right, 0%, -30%, and +30% respectively. Carl Zeiss provide with their lenses a graph of the relative distortion versus the off-axis distance h. Fig. 3A shows such a graph for the Distagon 2.8/21.
Figure 3. Distortion curve for the Distagon 2.8/21 (A, Zeiss data)and its derivative (B).
It is frequently asserted that negative values of D correspond to barrel distortion, and positive values to pincushion distortion. For simple distortion curves this is true, but a few lenses with a more complex distortion curve do not follow this simple rule. As a case in point I consider the above Distagon 2.8/21. Fig. 4 shows a grid distorted according to the data in Fig. 3. Although curve A is negative everywhere, the grid reveals significant pincushion distortion toward the corners. Indeed, it is not the sign of D which determines the type of distortion, but the slope of the curve. A negative slope (yellow part) of D implies barrel distortion, a positive slope (orange part) pincushion distortion. The steeper the slope, the more pronounced the distortion.
Fig. 3A should be read as follows. Up to 15 mm the slope is negative, which corresponds to barrel distortion. From 15 mm to the far image corner the slope is positive: pincushion distortion. The latter slope is steeper than the former, so that the pincushion distortion in the image periphery is more pronounced than the barrel distortion in the more central regions. Thus, it is not the magnitude of D which is important for line straightness, but the slope of the curve. In this light Fig. 3B is a better guide toward line straightness than 3A. Graph B shows the derivative dD/dh, i.e. the slope of D. The sign of this curve is uniquely coupled to the distortion type and its amplitude to the degree of line deformation.
Figure 4. Distortion by the Distagon 2.8/21 according to the data in Fig. 3.
Distortion varies with the off-axis distance as h'- h = ah3 + bh5 + .... Relatively, D varies as (h'-h)/h = ah2 + bh4. The coefficient a is positive for pincushion and negative for barrel distortion. Usually the quadratic term outweighs the higher-order terms and D varies with hsquared, getting progressively worse toward the image corners. However, in certain wideangle designs the quartic term is large enough to overcome the quadratic term [4]. The Distagon 2.8/21 exemplifies such behavior, with the curve forced upward in the image corner.
Distortion in practice
Fig. 5 presents an image captured with the Distagon 2.8/21. The horizontal lines of the four concrete layers are very similar to the 'theoretical' lines of Fig. 4. The lower layer goes through the image center and is not distorted. The second and third layers show a modest barrel distortion, while the top layer reveals a more pronounced pincushion distortion(第2,3层是桶形畸变, 最顶层的是枕形畸变,还可以这样啊??).

Figure 5. Distortion by the Distagon 2.8/21 in practice.
Perspective
The curvilinear distortion treated above is also known as optical distortion(光学畸变,包括前面的桶形和枕形畸变). It should not be confused with other effects sometimes referred to as distortion.
The perspective of a photograph is determined by the viewpoint that the photographer takes in relation to the subject. Nearby subjects are rendered larger than faraway subjects of the same size, which can lead to a sense of depth or convergence(收敛,聚集,相交) of lines that are parallel in object space. A well-known example of a perspective effect are the converging verticals that occur when a building is photographed with a tilted camera (Fig. 6). Perspective is not affected by the lens focal length: it depends on the viewpoint only.(透视与焦距无关,只有视点决定?)
Figure 6. Converging verticals. (18-mm lens on a 24×36 mm slide)
The designation 'perspective distortion' is frequently encountered to describe converging verticals and other manifestations of the perspective, but this is questionable as it unjustly suggests a misrepresentation of the reality. There is actually nothing that is distorted in Fig. 6, the photograph reveals a natural perspective(作者认为透视想象被称为透视畸变是值得怀疑的). A perspective control lens(透视控制镜头,移轴镜头), which is a device that can can be used to 'correct' converging verticals, creates an illusion of a different perspective, but if anything it's the perspective control lens that presents an unnatural perspective, not the normal picture taking lens. (Strictly speaking a PC lens does not correct anything. Its large image circle and mechanical design merely allow a shift of the image to put the subject into the film frame. The prerequisite for nonconverging verticals is that the film plane is kept parallel to the building.)
Converging verticals often encounter psychological resistance, while photographs with converging horizontals such as a road that narrows toward the horizon are readily accepted or even considered as perspective art. Apart from the appreciation by the audience, however, there is no fundamental difference between the two cases.(垂直方向的透视,人们心里不易接受,然而水平方向,例如道路在远的地方变窄,这类透视则不然,会被在惩称之为艺术。这两个case的球面像差的原理是一样的。)
Another so-called form of distortion is 'geometric distortion'(几何畸变), which arises when a three-dimensional object is projected on a flat plane(当一个三维的物体投影在一个平面上会发生). For instance, a sphere in the image center is rendered as a round disk on the film, while a sphere in the image periphery(边缘) of a lens with a large angle of view is elliptically(椭圆) elongated. People in the corners of a wideangle image are deformed (Fig. 7) and may occasionally be saddled with the typical 'egg face'(蛋脸?). This geometric effect is a natural consequence of the projection obliquity(投影倾斜); the use of the word distortion wrongly suggests an imaging anomaly.
Figure 7. Geometric distortion: the photograph does not flatter the person at the left. (18-mm lens on a 24×36 mm slide)
In contrast to optical distortion, perspective and geometric distortions are no lens aberrations. The apparent anomaly is emphasized by a wrong viewpoint for the image. Ideally each photograph should be viewed from a viewpoint that corresponds to the viewpoint of the photographer in relation to the captured scene. A telephoto image should be viewed from far and a wideangle photograph from close. A photograph with converging verticals looks more natural from a low viewpoint, and, similarly, egg faces improve when the photograph is viewed from close.Unfortunately the human eye does not cover a sufficiently large angle to view the entire image of a wideangle lens from nearby, so in practice the viewer takes a distance, notices the deformation, and speaks of distortion.