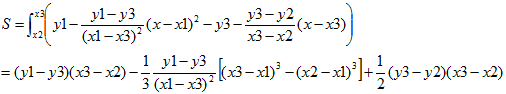- 推荐开源项目:Chat-Template - 快速构建聊天机器人原型的利器
侯深业Dorian
推荐开源项目:Chat-Template-快速构建聊天机器人原型的利器chat-templateChat-TemplateisaReactcomponentthatenablesquickprototypingofbotconversations.项目地址:https://gitcode.com/gh_mirrors/ch/chat-template1、项目介绍Chat-Template是一款基于
- 微信小程序【发送给朋友】和【复制链接】功能,灰色不可用
我有一棵树
微信小程序小程序
每日鸡汤:悲观者可能正确,但是乐观者往往成功假设你是一个用户,你随便找一个小程序可以看到这几个功能转发给朋友分享到朋友圈复制链接很常见的功能,但是如果你作为开发者,这几个功能就需要自己做喽,并不是你项目建起来了就有的。1.【转发给朋友】使用onShareAppMessage这个方法这个方法有一个坑,就是每个页面都得单独写一份,暂时没找到整个项目写一份,所有页面都能分享的方法【可能是我没找到?等我找
- element-tree组件连接线以及懒加载
{{data.fvAreaName}}default-expand-all为节点数默认全部展开,懒加载适用importbusfrom'@/utils/eventBus.js'import{TableAPI}from'@/api/valuation/my_valuation.js'exportdefault{props:{treelist:Array,ShowSave:Boolean,taskInf
- 将本地项目上传到 GitLab/GitHub
司南锤
GitHubgitlabgithubelasticsearch
以下是将本地项目上传到GitLab的完整步骤,从创建仓库到推送代码的详细流程:1.在GitLab上创建新项目登录GitLab,点击Newproject。选择Createblankproject。填写项目信息:Projectname:项目名称(如my-project)。VisibilityLevel:选择仓库可见性(公开/私有/内部)。InitializerepositorywithaREADME:
- UE5 特效
qq_42863961
虚幻基础ue5
能帮到你的话,就给个赞吧文章目录postprocessexposurebloomvignettesaturationunboundmaterial材质蓝图altzbasecolorconstant3Vectorroughnessconstantmetallicconstantpbrroughnessmetallicmakemorerealmakesomeareasrougherthanothers
- RTOS
weixin_34174422
操作系统嵌入式
这里参考wiki的英文,加上自己的理解翻译下RTOS的定义:Areal-timeoperatingsystem(RTOS)isanoperatingsystem(OS)intendedtoservereal-timeapplicationprocessdataasitcomesin,typicallywithoutbufferingdelays.Processingtimerequirements
- 构建局域网
sbiigu1
计算机相关网络应用网络协议数据结构应用服务器DOS
转自:[url]http://www.zhirui.com/it/2004/8-3/1185-2.html[/url]随着社会的不断进步,资源共享已经成为信息交流的一种重要方式,小到几个人的联网对战,大到覆盖全球的Internet,网络让许多人拉近了距离。而在学校、网吧和小型办公室首先接触的就是局域网。局域网基本知识1.基本概念局域网LAN(LocalAreaNetwork):通常指的都是规模不大
- No thread-bound request found: Are you referring to request attributes outside of an actual web requ
SUDO-1
javajava
Nothread-boundrequestfound:Areyoureferringtorequestattributesoutsideofanactualwebrequest,orprocessingarequestoutsideoftheoriginallyreceivingthread?Ifyouareactuallyoperatingwithinawebrequestandstillrec
- A novice’s attempt at translating
LuminaryDream
英语笔记笔记
声明:英文原文来自扇贝英语app,中文翻译仅供参考Businessesarealreadybeingradicallytransformedbyartificialintelligence(AI).企业已经被人工智能(AI)彻底改变。Toolsnowexistthatofferinstantaneous,high-qualityresultsinimprovingcertainoperations
- Hutools 构建树结构
理想二旬不止(上班了,好像没有理想了)
Hutools工具使用记录java
详细介绍Hutools官网我们平时在进行业务开发时,经常会碰到需要构建树形结构的业务,比如常见的区域树结构└──省└──市└──区这类数据的特点就是每条数据有明确的父节点和子节点,如果没有父节点或者子节点的话,没有父节点的数据为树的顶级节点,如果没有子节点的话为树的底级节点。示例数据表及数据CREATETABLEarea(area_idBIGINTNOTNULLAUTO_INCREMENT,par
- ARM下汇编语言编程
Kylin77626
arm开发
一、ARM汇编语言程序格式ARM汇编语言是以段(section)为单位来组织源文件的。段是相对独立的、具有特定名称的、不可分割的指令或者数据序列。段又可以分为代码段和数据段,代码段存放执行代码,数据段存放代码运行时需要用到的数据。一个ARM源程序至少需要一个代码段,大的程序可以包含多个代码段和数据段。二、ARM汇编语言中常用的伪操作例如:areareset,code,readonly;声明了一个名
- VLAN间路由配置实战
wespten
网络协议栈网络设备5G物联网网络工具开发网络
一、VLAN间路由1、VLAN隔离技术1.传统网络类型传统网络有三种类型:LAN–LocalAreaNetwork局域网;MAN–城域网;WAN–WideAreaNetwork广域网-internet;2.VLAN技术随着网络中计算机的数量越来越多,传统的以太网络开始面临广播泛滥以及安全性无法保障等各种问题。VLAN(VirtualLocalAreaNetwork)即虚拟局域网,是将一个物理的局域
- python 统计相同像素值个数
AI算法网奇
python基础opencv计算机视觉python
目录python统计相同像素值个数最大值附近的值python统计相同像素值个数importcv2importnumpyasnpimporttimefromcollectionsimportCounter#读取图像image=cv2.imread('mask16.jpg')#将图像转换为灰度图像gray_image=cv2.cvtColor(image,cv2.COLOR_BGR2GRAY)area
- html两级地区选择级联,index.html · RockYang/JAreaSelect-js省市区级联选择插件 - Gitee.com...
猫咪兔兔
html两级地区选择级联
地区选择插件.form-control{margin-right:5px;}传统表单selectUI样式获取地址仿京东UI样式选择区域vararea=$("#area-select-box").JAreaSelect({prov:1,city:72,dist:2839,level:3});$(".btn-success").on('click',function(){$(this).text(ar
- Element UI 中国省市区级联数据_provinceandcitydata 获取地区
2401_87288783
javascript前端开发语言
省市二级联动(不带“全部”选项): import { provinceAndCityData } from 'element-china-area-data' export default { data () { return { options: provinceAndCityData, selectedOptions: [
- 天梯赛 L3-009 长城 计算几何
样例过了就是过了
算法数据结构c++
一题目二思路这道题就是找凸点的个数,就是答案。可能有些人会说,那不就是大于左右两边就是凸点吗,只对了一半,如下图所示,B点也是算凸点,但是并没有大于左右两边。因此,我们判断凸点的依据,是看AB的斜率是否大于AC的斜率,如图所示,AB的斜率大于AC的斜率,所以是凸点。反之,不是。此外,我们用一个栈去维护凸点,就可以得到凸点的个数,也就是答案。三代码#include#include#defineint
- 写了一个关于root的介绍以及大致流程(全篇都是自己写的)本人是小菜,欢迎大家指点
王景程
算法scipy
IwanttogiveyouaintroductionofwhatROOTisandthemainstepsofhowtodothat.Iwilltrymybesttoexplain,andifthereareanywordsorsentencesthatarenotaccurate,feelfreetopiontout,asawaytopracticemywriting.Inlayman'ste
- WebRover :一个功能强大的 Python 库,用于从 Web 内容生成高质量的数据集。
数据集
2024-11-30,由Area-25团队开发的一个专门用于生成高质量网络内容数据集的Python库。该数据集旨在为大型语言模型(LLM)和人工智能应用的训练提供丰富的数据资源。数据集地址:WebRoverDataset|自然语言处理数据集|AI模型训练数据集一、让我们一起来看一下WebRoverWebRover通过智能网络爬虫技术,自动从网络中提取与特定主题相关的内容,并支持多种输入格式,如JS
- WebRover: 专为训练大型语言模型和 AI 应用程序而设计的 Python 库
数据集
2024-11-30,由Area-25团队开发的一个专门用于生成高质量网络内容数据集的Python库。该数据集旨在为大型语言模型(LLM)和人工智能应用的训练提供丰富的数据资源。数据集地址:WebRoverDataset|自然语言处理数据集|AI模型训练数据集一、让我们一起来看一下WebRoverWebRover通过智能网络爬虫技术,自动从网络中提取与特定主题相关的内容,并支持多种输入格式,如JS
- Delete the specified node in the linked list with dummy header
青眸ღ.
算法链表数据结构c语言
分数20作者伍建全单位重庆科技大学PleasecreateafunctionwiththeprototypevoiddeleteNode(ListL,intkey).ThisfunctionshoulddeletethefirstnodefromthelinkedlistLwithdummyheader,wherethedatafieldisequaltokey.Iftherearenonodes
- python snap7 plc_Python-Snap7获取西门子PLC 300数值
weixin_39541767
pythonsnap7plc
说明在一次数采项目中,对现场PLC300进行取数。本人小白,在网上搜罗了大量信息,终于找到解决方法。准备事项1.操作系统2.Python环境(本次使用Python3.6.6)3.Snap7connectconnect('192.168.0.1',rack=0,slot=1)三个参数分别为:IP地址,机架,插槽read_area及write_area函数read_area(self,area,dbn
- ios storekit_在iOS 14中的应用购买和storekit中
weixin_26745865
iospython
iosstorekitMuchofthebuzzatWWDC2020wasgivenovertoSwiftUI2.0andARKit,soyoushouldforgiveyourselfformissingaseismicchangetotheStoreKitframework—achangethatwillmakeyourlifeasanappdeveloperfareasier.WWDC202
- unity转微信小游戏:自定义分享标题和分享图片
会潜水的小火龙
unity转微信小游戏unity微信小程序
unity转微信小游戏:自定义分享标题和分享图片代码使用方法代码//获取微信小程序分享参数更改为自己的分享标题和分享图片publicvoidOnShareAppMessage(boolisListen=true,stringimageUrl="",stringtitle="",stringquery=""){if(isListen){WX.ShowShareMenu(newShowShareMen
- ILI9806G 4.3吋触摸屏 LVGL9 描点函数
UIUI
lvgl9stm32f407ucos3
staticvoiddisp_flush(lv_display_t*disp_drv,constlv_area_t*area,uint16_t*px_map){if(disp_flush_enabled){/*Themostsimplecase(butalsotheslowest)toputallpixelstothescreenone-by-one*/int32_tx;int32_ty;int3
- 用diff 生成patch文件的命令/方法
fcf1990501
LINUX系统
linuxdiff命令diff命令可以比较两个相同文件进而生成patch文件使用如下命令获得命令的帮助didff--help使用以下option-u,-UNUM,--unified[=NUM]outputNUM(default3)linesofunifiedcontext-r,--recursiverecursivelycompareanysubdirectoriesfound-N,--new-f
- 关于告警,要想做好,从这些方面着手
各类监控系统都会产生告警事件,于是,就产生了FlashDuty、PagerDuty、Opsgenie这类产品,做告警事件的收敛降噪、排班认领升级等。如果你想增强自己公司的告警事件处理能力,参考(chaoxi)这些产品的功能就可以了。告警集成:目标是在一个Oncall平台上处理所有告警,一般常见的监控工具,都有对接webhook的能力,因此Oncall平台可以对不同监控工具进行接口适配,提供一个相应
- Java应用的多租户架构设计
楠贝
java开发语言
Java应用的多租户架构设计大家好,我是微赚淘客返利系统3.0的小编,是个冬天不穿秋裤,天冷也要风度的程序猿!多租户架构(Multi-Tenancy)是SaaS(SoftwareasaService)应用中常见的设计模式,它允许多个租户(客户)共享同一个应用实例,同时保证数据隔离和独立性。在Java应用中实现多租户架构,涉及到数据模型设计、访问控制、资源隔离等多个方面。1.数据模型设计在多租户架构
- Autoware平台架构及设计
星火(star&fire)
autosar自动驾驶apolloApollo自动驾驶
官网:https://autoware.org/autoware-overview/gitlab仓库:https://github.com/autowarefoundation/autowaregithub仓库:https://github.com/autowarefoundation/autoware.universeAutowareAutoware是一个自动驾驶开发平台,由Apex.AI开发和
- 【Linux】【内存】Buddy 系统(伙伴系统)
钟离墨笺
Linuxlinux服务器运维
【Linux】【内存】Buddy系统(伙伴系统)根据上篇的NUMA架构可以知道它将物理内存分成多个节点(memorynode)每个memorynode分为不同的zone每个zone又有自己的free_areaBuddy伙伴系统就是对这些free_area的一种管理方式free_area里面的内存管理是根据页面大小管理的例如:下标页面大小free_area[0]2^0*4kfree_area[1]2
- git 常用命令
2301_78094384
gitgit
1.常用命令gitaddREADME跟踪README文件gitrm--cachedfilename从暂存区、本地库删除filename文件gitcommit-m“”fn//把文件提交到仓库(commit命令后添加-m选项,将提交信息与命令放在同一行例:gitcommit-m“wroteareadme”filen)gitcommit--amend-m‘xxx’合并上一次提交(用于反复修改)gitst
- HttpClient 4.3与4.3版本以下版本比较
spjich
javahttpclient
网上利用java发送http请求的代码很多,一搜一大把,有的利用的是java.net.*下的HttpURLConnection,有的用httpclient,而且发送的代码也分门别类。今天我们主要来说的是利用httpclient发送请求。
httpclient又可分为
httpclient3.x
httpclient4.x到httpclient4.3以下
httpclient4.3
- Essential Studio Enterprise Edition 2015 v1新功能体验
Axiba
.net
概述:Essential Studio已全线升级至2015 v1版本了!新版本为JavaScript和ASP.NET MVC添加了新的文件资源管理器控件,还有其他一些控件功能升级,精彩不容错过,让我们一起来看看吧!
syncfusion公司是世界领先的Windows开发组件提供商,该公司正式对外发布Essential Studio Enterprise Edition 2015 v1版本。新版本
- [宇宙与天文]微波背景辐射值与地球温度
comsci
背景
宇宙这个庞大,无边无际的空间是否存在某种确定的,变化的温度呢?
如果宇宙微波背景辐射值是表示宇宙空间温度的参数之一,那么测量这些数值,并观测周围的恒星能量输出值,我们是否获得地球的长期气候变化的情况呢?
&nbs
- lvs-server
男人50
server
#!/bin/bash
#
# LVS script for VS/DR
#
#./etc/rc.d/init.d/functions
#
VIP=10.10.6.252
RIP1=10.10.6.101
RIP2=10.10.6.13
PORT=80
case $1 in
start)
/sbin/ifconfig eth2:0 $VIP broadca
- java的WebCollector爬虫框架
oloz
爬虫
WebCollector主页:
https://github.com/CrawlScript/WebCollector
下载:webcollector-版本号-bin.zip将解压后文件夹中的所有jar包添加到工程既可。
接下来看demo
package org.spider.myspider;
import cn.edu.hfut.dmic.webcollector.cra
- jQuery append 与 after 的区别
小猪猪08
1、after函数
定义和用法:
after() 方法在被选元素后插入指定的内容。
语法:
$(selector).after(content)
实例:
<html>
<head>
<script type="text/javascript" src="/jquery/jquery.js"></scr
- mysql知识充电
香水浓
mysql
索引
索引是在存储引擎中实现的,因此每种存储引擎的索引都不一定完全相同,并且每种存储引擎也不一定支持所有索引类型。
根据存储引擎定义每个表的最大索引数和最大索引长度。所有存储引擎支持每个表至少16个索引,总索引长度至少为256字节。
大多数存储引擎有更高的限制。MYSQL中索引的存储类型有两种:BTREE和HASH,具体和表的存储引擎相关;
MYISAM和InnoDB存储引擎
- 我的架构经验系列文章索引
agevs
架构
下面是一些个人架构上的总结,本来想只在公司内部进行共享的,因此内容写的口语化一点,也没什么图示,所有内容没有查任何资料是脑子里面的东西吐出来的因此可能会不准确不全,希望抛砖引玉,大家互相讨论。
要注意,我这些文章是一个总体的架构经验不针对具体的语言和平台,因此也不一定是适用所有的语言和平台的。
(内容是前几天写的,现附上索引)
前端架构 http://www.
- Android so lib库远程http下载和动态注册
aijuans
andorid
一、背景
在开发Android应用程序的实现,有时候需要引入第三方so lib库,但第三方so库比较大,例如开源第三方播放组件ffmpeg库, 如果直接打包的apk包里面, 整个应用程序会大很多.经过查阅资料和实验,发现通过远程下载so文件,然后再动态注册so文件时可行的。主要需要解决下载so文件存放位置以及文件读写权限问题。
二、主要
- linux中svn配置出错 conf/svnserve.conf:12: Option expected 解决方法
baalwolf
option
在客户端访问subversion版本库时出现这个错误:
svnserve.conf:12: Option expected
为什么会出现这个错误呢,就是因为subversion读取配置文件svnserve.conf时,无法识别有前置空格的配置文件,如### This file controls the configuration of the svnserve daemon, if you##
- MongoDB的连接池和连接管理
BigCat2013
mongodb
在关系型数据库中,我们总是需要关闭使用的数据库连接,不然大量的创建连接会导致资源的浪费甚至于数据库宕机。这篇文章主要想解释一下mongoDB的连接池以及连接管理机制,如果正对此有疑惑的朋友可以看一下。
通常我们习惯于new 一个connection并且通常在finally语句中调用connection的close()方法将其关闭。正巧,mongoDB中当我们new一个Mongo的时候,会发现它也
- AngularJS使用Socket.IO
bijian1013
JavaScriptAngularJSSocket.IO
目前,web应用普遍被要求是实时web应用,即服务端的数据更新之后,应用能立即更新。以前使用的技术(例如polling)存在一些局限性,而且有时我们需要在客户端打开一个socket,然后进行通信。
Socket.IO(http://socket.io/)是一个非常优秀的库,它可以帮你实
- [Maven学习笔记四]Maven依赖特性
bit1129
maven
三个模块
为了说明问题,以用户登陆小web应用为例。通常一个web应用分为三个模块,模型和数据持久化层user-core, 业务逻辑层user-service以及web展现层user-web,
user-service依赖于user-core
user-web依赖于user-core和user-service
依赖作用范围
Maven的dependency定义
- 【Akka一】Akka入门
bit1129
akka
什么是Akka
Message-Driven Runtime is the Foundation to Reactive Applications
In Akka, your business logic is driven through message-based communication patterns that are independent of physical locatio
- zabbix_api之perl语言写法
ronin47
zabbix_api之perl
zabbix_api网上比较多的写法是python或curl。上次我用java--http://bossr.iteye.com/blog/2195679,这次用perl。for example: #!/usr/bin/perl
use 5.010 ;
use strict ;
use warnings ;
use JSON :: RPC :: Client ;
use
- 比优衣库跟牛掰的视频流出了,兄弟连Linux运维工程师课堂实录,更加刺激,更加实在!
brotherlamp
linux运维工程师linux运维工程师教程linux运维工程师视频linux运维工程师资料linux运维工程师自学
比优衣库跟牛掰的视频流出了,兄弟连Linux运维工程师课堂实录,更加刺激,更加实在!
-----------------------------------------------------
兄弟连Linux运维工程师课堂实录-计算机基础-1-课程体系介绍1
链接:http://pan.baidu.com/s/1i3GQtGL 密码:bl65
兄弟连Lin
- bitmap求哈密顿距离-给定N(1<=N<=100000)个五维的点A(x1,x2,x3,x4,x5),求两个点X(x1,x2,x3,x4,x5)和Y(
bylijinnan
java
import java.util.Random;
/**
* 题目:
* 给定N(1<=N<=100000)个五维的点A(x1,x2,x3,x4,x5),求两个点X(x1,x2,x3,x4,x5)和Y(y1,y2,y3,y4,y5),
* 使得他们的哈密顿距离(d=|x1-y1| + |x2-y2| + |x3-y3| + |x4-y4| + |x5-y5|)最大
- map的三种遍历方法
chicony
map
package com.test;
import java.util.Collection;
import java.util.HashMap;
import java.util.Iterator;
import java.util.Map;
import java.util.Set;
public class TestMap {
public static v
- Linux安装mysql的一些坑
chenchao051
linux
1、mysql不建议在root用户下运行
2、出现服务启动不了,111错误,注意要用chown来赋予权限, 我在root用户下装的mysql,我就把usr/share/mysql/mysql.server复制到/etc/init.d/mysqld, (同时把my-huge.cnf复制/etc/my.cnf)
chown -R cc /etc/init.d/mysql
- Sublime Text 3 配置
daizj
配置Sublime Text
Sublime Text 3 配置解释(默认){// 设置主题文件“color_scheme”: “Packages/Color Scheme – Default/Monokai.tmTheme”,// 设置字体和大小“font_face”: “Consolas”,“font_size”: 12,// 字体选项:no_bold不显示粗体字,no_italic不显示斜体字,no_antialias和
- MySQL server has gone away 问题的解决方法
dcj3sjt126com
SQL Server
MySQL server has gone away 问题解决方法,需要的朋友可以参考下。
应用程序(比如PHP)长时间的执行批量的MYSQL语句。执行一个SQL,但SQL语句过大或者语句中含有BLOB或者longblob字段。比如,图片数据的处理。都容易引起MySQL server has gone away。 今天遇到类似的情景,MySQL只是冷冷的说:MySQL server h
- javascript/dom:固定居中效果
dcj3sjt126com
JavaScript
<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://www.w3.org/TR/xhtml1/DTD/xhtml1-transitional.dtd">
<html xmlns="http://www.w3.org/1999/xhtml&
- 使用 Spring 2.5 注释驱动的 IoC 功能
e200702084
springbean配置管理IOCOffice
使用 Spring 2.5 注释驱动的 IoC 功能
developerWorks
文档选项
将打印机的版面设置成横向打印模式
打印本页
将此页作为电子邮件发送
将此页作为电子邮件发送
级别: 初级
陈 雄华 (
[email protected]), 技术总监, 宝宝淘网络科技有限公司
2008 年 2 月 28 日
&nb
- MongoDB常用操作命令
geeksun
mongodb
1. 基本操作
db.AddUser(username,password) 添加用户
db.auth(usrename,password) 设置数据库连接验证
db.cloneDataBase(fromhost)
- php写守护进程(Daemon)
hongtoushizi
PHP
转载自: http://blog.csdn.net/tengzhaorong/article/details/9764655
守护进程(Daemon)是运行在后台的一种特殊进程。它独立于控制终端并且周期性地执行某种任务或等待处理某些发生的事件。守护进程是一种很有用的进程。php也可以实现守护进程的功能。
1、基本概念
&nbs
- spring整合mybatis,关于注入Dao对象出错问题
jonsvien
DAOspringbeanmybatisprototype
今天在公司测试功能时发现一问题:
先进行代码说明:
1,controller配置了Scope="prototype"(表明每一次请求都是原子型)
@resource/@autowired service对象都可以(两种注解都可以)。
2,service 配置了Scope="prototype"(表明每一次请求都是原子型)
- 对象关系行为模式之标识映射
home198979
PHP架构企业应用对象关系标识映射
HELLO!架构
一、概念
identity Map:通过在映射中保存每个已经加载的对象,确保每个对象只加载一次,当要访问对象的时候,通过映射来查找它们。其实在数据源架构模式之数据映射器代码中有提及到标识映射,Mapper类的getFromMap方法就是实现标识映射的实现。
二、为什么要使用标识映射?
在数据源架构模式之数据映射器中
//c
- Linux下hosts文件详解
pda158
linux
1、主机名: 无论在局域网还是INTERNET上,每台主机都有一个IP地址,是为了区分此台主机和彼台主机,也就是说IP地址就是主机的门牌号。 公网:IP地址不方便记忆,所以又有了域名。域名只是在公网(INtERNET)中存在,每个域名都对应一个IP地址,但一个IP地址可有对应多个域名。 局域网:每台机器都有一个主机名,用于主机与主机之间的便于区分,就可以为每台机器设置主机
- nginx配置文件粗解
spjich
javanginx
#运行用户#user nobody;#启动进程,通常设置成和cpu的数量相等worker_processes 2;#全局错误日志及PID文件#error_log logs/error.log;#error_log logs/error.log notice;#error_log logs/error.log inf
- 数学函数
w54653520
java
public
class
S {
// 传入两个整数,进行比较,返回两个数中的最大值的方法。
public
int
get(
int
num1,
int
nu
![]() ,带入点(x3,y3)得
,带入点(x3,y3)得![]() ,由两交点得到直线
,由两交点得到直线![]() ,所以直线与抛物线围成的面积
,所以直线与抛物线围成的面积