POJ 1222 EXTENDED LIGHTS OUT(高斯消元)

传送门

EXTENDED LIGHTS OUT

Time Limit: 1000MS		Memory Limit: 10000K
Total Submissions: 8857		Accepted: 5740

Description

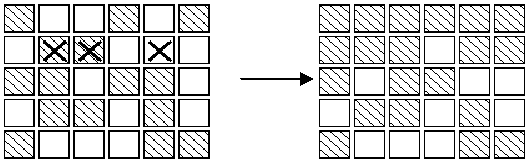
In an extended version of the game Lights Out, is a puzzle with 5 rows of 6 buttons each (the actual puzzle has 5 rows of 5 buttons each). Each button has a light. When a button is pressed, that button and each of its (up to four) neighbors above, below, right and left, has the state of its light reversed. (If on, the light is turned off; if off, the light is turned on.) Buttons in the corners change the state of 3 buttons; buttons on an edge change the state of 4 buttons and other buttons change the state of 5. For example, if the buttons marked X on the left below were to be pressed,the display would change to the image on the right. 

The aim of the game is, starting from any initial set of lights on in the display, to press buttons to get the display to a state where all lights are off. When adjacent buttons are pressed, the action of one button can undo the effect of another. For instance, in the display below, pressing buttons marked X in the left display results in the right display.Note that the buttons in row 2 column 3 and row 2 column 5 both change the state of the button in row 2 column 4,so that, in the end, its state is unchanged. 

Note: 
1. It does not matter what order the buttons are pressed. 
2. If a button is pressed a second time, it exactly cancels the effect of the first press, so no button ever need be pressed more than once. 
3. As illustrated in the second diagram, all the lights in the first row may be turned off, by pressing the corresponding buttons in the second row. By repeating this process in each row, all the lights in the first 
four rows may be turned out. Similarly, by pressing buttons in columns 2, 3 ?, all lights in the first 5 columns may be turned off. 
Write a program to solve the puzzle.

Input

The first line of the input is a positive integer n which is the number of puzzles that follow. Each puzzle will be five lines, each of which has six 0 or 1 separated by one or more spaces. A 0 indicates that the light is off, while a 1 indicates that the light is on initially.

Output

For each puzzle, the output consists of a line with the string: "PUZZLE #m", where m is the index of the puzzle in the input file. Following that line, is a puzzle-like display (in the same format as the input) . In this case, 1's indicate buttons that must be pressed to solve the puzzle, while 0 indicate buttons, which are not pressed. There should be exactly one space between each 0 or 1 in the output puzzle-like display.

Sample Input

2
0 1 1 0 1 0
1 0 0 1 1 1
0 0 1 0 0 1
1 0 0 1 0 1
0 1 1 1 0 0
0 0 1 0 1 0
1 0 1 0 1 1
0 0 1 0 1 1
1 0 1 1 0 0
0 1 0 1 0 0

Sample Output

PUZZLE #1
1 0 1 0 0 1
1 1 0 1 0 1
0 0 1 0 1 1
1 0 0 1 0 0
0 1 0 0 0 0
PUZZLE #2
1 0 0 1 1 1
1 1 0 0 0 0
0 0 0 1 0 0
1 1 0 1 0 1
1 0 1 1 0 1

Source

Greater New York 2002

题目大意：

有一个5*6的矩阵，每个方格代表一个灯，每操作一个灯，周围的上下左右四个灯会发生相应变化 即由灭变亮，由亮变灭，如何操作使灯全灭，并输出操作矩阵（1表示按，0表示不按）

解题思路：

其实这个题目跟上一个题目很类似（这个题目比上一个更简单），因为最后是让我们输出矩阵，而且根据题目意思来说，根本没有自由变元，也就是这个矩阵直接就是确定的，唯一解，然后我们构造一个A矩阵，跟上一个一样构造，然后直接套模板就行了。

My Code：

#include <iostream>
#include <cstdio>
#include <cstring>
#include <cstdlib>
#include <cmath>
using namespace std;
const int MAXN = 1e2+5;
int equ, var;///equ个方程 var个变量
int a[MAXN][MAXN];///增广矩阵
int x[MAXN];///解的数目
bool free_x[MAXN];///判断是不是自由变元
int free_num;///自由变元的个数
inline int GCD(int m, int n)
{
    if(n == 0)
        return m;
    return GCD(n, m%n);
}
inline int LCM(int a, int b)
{
    return a/GCD(a,b)*b;
}

int Gauss()
{
    int Max_r;///当前列绝对值最大的存在的行
    ///col：处理当前的列
    int row = 0;
    int free_x_num;
    int free_index;
    for(int col=0; row<equ&&col<var; row++,col++)
    {
        Max_r = row;
        for(int i=row+1; i<equ; i++)
            if(abs(a[i][col]) > abs(a[Max_r][col]))
                Max_r = i;

        if(Max_r != row)
            for(int i=0; i<var+1; i++)
                swap(a[row][i], a[Max_r][i]);

        if(a[row][col] == 0)
        {
            row--;
            continue;
        }
        for(int i=row+1; i<equ; i++)
        {
            if(a[i][col])
            {
                for(int j=col; j<var+1; j++)
                    a[i][j] ^= a[row][j];
            }
        }
    }
    for(int i=row; i<equ; i++)
        if(a[i][var])
            return -1;///无解

    if(row < var)
    {
        for(int i=row-1; i>=0; i--)
        {
            free_x_num = 0;
            for(int j=0; j<var; j++)
                if(a[i][j] && free_x[j])
                {
                    free_x_num++;
                    free_index = j;
                }

            if(free_x_num > 1)
                continue;
            int tmp = a[i][var];
            for(int j=0; j<var; j++)
                if(a[i][j] && j!=free_index)
                    tmp -= a[i][j]*x[j];
            x[free_index] = tmp/a[i][free_index];/// 求出该变元.
            free_x[free_index] = 0; /// 该变元是确定的.
        }
        return var - row;///自由变元的个数
    }
    for(int i=var-1; i>=0; i--)
    {
        int tmp = a[i][var];
        for(int j=i+1; j<var; j++)
            if (a[i][j])
                tmp ^= a[i][j]*x[j];
        if (tmp%a[i][i])
            return -2; /// 说明有浮点数解，但无整数解.
        x[i] = tmp/a[i][i];
    }
    return 0;///唯一解
}
int main()
{
    int T;
    cin>>T;
    for(int cas=1; cas<=T; cas++)
    {
        memset(a, 0, sizeof(a));
        memset(x, 0, sizeof(x));
        equ =  var = 30;
        for(int i=0; i<var; i++)
        {
            int ta = i%6, tb = i/6;
            a[i][i] = 1;
            if(ta > 0)
                a[i][i-1] = 1;
            if(ta < 5)
                a[i][i+1] = 1;
            if(tb > 0)
                a[i][i-6] = 1;
            if(tb < 5)
                a[i][i+6] = 1;
        }
        for(int i=0; i<equ; i++)
            cin>>a[i][var];

        int k = Gauss();
        printf("PUZZLE #%d\n",cas);
        for(int i=0; i<var; i++)
        {
            if(i%6 == 5)
                cout<<x[i]<<endl;
            else
                cout<<x[i]<<" ";
        }
    }
    return 0;
}