- 活给自己看,笑容才灿烂
听着了么
白岩松说“有时候,我们活得很累,并非生活过于刻薄,而是我们太容易被外界的氛围所感染,被他人的情绪所左右。”心情是自己的。若只是活在别人的眼里、嘴里,便掌握不了让自己开心的主动权。人活着,不是为了活给别人看的,唯有做最真实的自己,活给自己看,笑容才灿烂。诚然,世事纷繁复杂,人人都有一张嘴,管也管不了。永远有人欣赏你,也永远有人批评你,不可能做到让所有人都满意,开心做自己才是最重要的。人生苦短,有太多
- python是什么意思中文-在python中%是什么意思
编程大乐趣
Python中%有两种:1、数值运算:%代表取模,返回除法的余数。如:>>>7%212、%操作符(字符串格式化,stringformatting),说明如下:%[(name)][flags][width].[precision]typecode(name)为命名flags可以有+,-,''或0。+表示右对齐。-表示左对齐。''为一个空格,表示在正数的左侧填充一个空格,从而与负数对齐。0表示使用0填
- 东南林氏之九牧林候选父系
祖缘树TheYtree
渊源介绍东晋初年晋安林始祖林禄公入闽,传十世隋右丞林茂,由晋安迁居莆田北螺村。又五世而至林万宠,唐开元间任高平太守,生三子:韬、披、昌。韬公之孙攒,唐德宗立双阙以旌表其孝,时号"阙下林家"。昌公字茂吉,乃万宠公第三子,官兵部司马,配宋氏,生一子名萍。萍于唐贞元间明经及第,官沣洲司马(后追赠中宪大夫)。唐太和年间归隐后,迁居仙游游洋,世称“游洋林”;其后裔居游洋后迁移漳州漳浦路下,由路下林第四房平和
- 怎么做淘客赚钱(2022最新免费淘客盈利的方法)
高省_飞智666600
很多人都不知道什么是淘宝客,今天小编为大家解答一下吧。淘宝客,现在简称淘客,是时下比较流行的一个词语,特质为淘宝店推广商品获取提成的人,这些人没有自己的产品,只是在淘宝里面选择适合自己的产品,在自己比较熟悉的领域推广,把产品卖出去之后,会从淘宝店家那里获得百分之五到百分之五十左右的佣金。淘宝客付出的是什么呢?时间。你需要花时间去选适合自己推广的产品,需要花时间去选自己的推广方法,如果你打算自己做个
- 第六集如何安装CentOS7.0,3分钟学会centos7安装教程
date分享
从光盘引导系统按回车键继续进入引导程序安装界面,选择语言这里选择简体中文版点击继续选择桌面安装下面给系统分区选择磁盘,点击完成选择基本分区,点击加号swap分区,大小填内存的两倍在选择根分区,使用所有可用的磁盘空间选择文件系统ext4点击完成,点击开始安装设置root密码,点击完成设置普通用户和密码,点击完成整个过程持续八分钟左右根据个人配置不同,时间长短不同好,现在点击重启系统进入重启状态点击本
- Redis系列:Geo 类型赋能亿级地图位置计算
Ly768768
redisbootstrap数据库
1前言我们在篇深刻理解高性能Redis的本质的时候就介绍过Redis的几种基本数据结构,它是基于不同业务场景而设计的:动态字符串(REDIS_STRING):整数(REDIS_ENCODING_INT)、字符串(REDIS_ENCODING_RAW)双端列表(REDIS_ENCODING_LINKEDLIST)压缩列表(REDIS_ENCODING_ZIPLIST)跳跃表(REDIS_ENCODI
- 第四天旅游线路预览——从贾登峪到喀纳斯景区入口(贾登峪游客服务中心)
陟彼高冈yu
基于Googleearthstudio的旅游规划和预览旅游
第四天:从贾登峪到喀纳斯风景区入口,晚上住宿贾登峪;从贾登峪到喀纳斯景区入口(贾登峪游客服务中心):搭乘贾登峪①路车,路过三湾到达景区换乘中心,路程时长约40分钟;1)早上8:00起床,吃完早饭,8:30出发;2)从贾登峪到喀纳斯风景区,需要搭乘一站公交车,为免费公交车,路程4.3公里,车程约9分钟8:40左右到达喀纳斯景区入口(贾登峪游客服务中心);3)乘坐贾登峪①路车,路过三湾到达景区换乘中心
- 摩托车加装车载手机充电usb方案/雅马哈USB充电方案开发
诚芯微科技
社交电子
长途骑行需要给手机与行车记录仪等设备供电,那么,加装USB充电器就相继在两轮电动车上应用起来了。摩托车加装usb充电方案主要应用于汽车、电动自行车、摩托车、房车、渡轮、游艇等交通工具。提供电动车USB充电器方案/摩托车加装usb充电方案/渡轮加装usb充电方案/游艇加装usb充电方案开发。摩托车加装车载手机充电usb方案、汽车游艇改装四孔面板装双USB车充点烟器5V/4A电动车USB充电器输入4.
- 第一四三章:天降奇兵
逸川
“是她!”为了护住公孙枝,季姜(姜姓吕氏女,名子芸)舍身朝着刺来的长戟迎了过去。待公孙枝反应过来,长戟的尖刃已经抵到了季姜的胸前,让他只感手足无措。然就在这千钧一发之际,有一支羽箭突然从山巅飞来直插入狄兵脖颈,将其连人带戟射倒在地。顺着羽箭飞来的方向望去,却见到一名头戴白色纱笠的女子,正站在山脊上左右开弓。每有羽箭射出,便立时有狄兵应声而倒,端是飒爽无比:“竟不知她技艺如此娴熟!”“她是谁?”听到
- 安徽省这个湖,比西湖大8倍,称是安徽的北戴河, 合肥的后花园
旅游小号角
旅游爱好者都知道,安徽省是一个旅游资源十分丰富的省份,且不说黄山、九华山、天柱山这三大名山,单说湖泊就不比其它省份少,今天我们一起走遍世界将为大家说说一个号称安徽北戴河,合肥后花园的湖泊,看看到底是哪个湖泊?话说,这个湖泊位于安徽省六安市舒城县境内,东距合肥50千米,大约一个小时左右的车程,它号称是合肥的后花园,安徽的北戴河。相传,湖畔石壁之上有一奇石神似观音临湖,湖中漂动众多小岛栩栩如佛子,宛若
- #开始记日记#1235 胃口不好吃饺子
董克平日记
2020/06/24星期三北京吃个醋拌茄子消暑珠珠送了一个父亲节礼物,要我陪她一起去体检。这些年身上多了许多毛病,血压、血脂、血糖都需要吃药维持了,上一次体检知道血糖已经到了临界点,可是也没有予以重视,继续大吃大喝少锻炼,结果可想而知,现在是每一餐都离不开二甲双胍了。不过我还是不愿意去体检,总觉得体检没什么用,身体有毛病就去看医生,体检又不治病。我对体检的看法是“小病不用治,大病治不了”,通过体检
- 祭坛随笔
阿门不热
街角右拐,便是北宋的祠堂。平日里冉冉的佛香被雨水打湿了,一地枯黄的银杏显得平静哀伤,如同一地被踩碎的阳光。我喜欢在这样的阴暗里吞噬古代的讯息,那遥远的来自过去的历史风潮。谢却茶扉,轻轻地抚上墙壁,寒风不御,无数深浅的纹路交织在心底,如同一把古琴不堪重负的尾音。寂寞锁朱门,香客们已是三三两两,巨大的雨帘让天空失掉了颜色,灰蒙蒙掉在阁楼一角,沉稳不惊地暗下去,再暗下去......古树上红色的挂牌像一块
- 2024.8.22 Python,链表两数之和,链表快速反转,二叉树的深度,二叉树前中后序遍历,N叉树递归遍历,翻转二叉树
RaidenQ
python链表开发语言
1.链表两数之和输入:l1=[2,4,3],l2=[5,6,4]输出:[7,0,8]解释:342+465=807.示例2:输入:l1=[0],l2=[0]输出:[0]示例3:输入:l1=[9,9,9,9,9,9,9],l2=[9,9,9,9]输出:[8,9,9,9,0,0,0,1]昨天的这个题,用自己的办法写的麻烦的要死,然后刚才一看chat归类的办法,感觉自己像个智障。classListNode
- 手机上有什么兼职可以做?网上兼职 一单一结 手机就可以做?
优惠券高省
建议上班族和全职宝妈把空闲时间拿出来一点做做副业,什么也不耽搁还能多一笔收入!推荐大家一定要试一试!!!只要有手机就可以做,下面小编就为大家推荐用手机就可以做的三类网上兼职工作。一,高省APP高省APP佣金更高,模式更好,终端用户不流失。【高省】是一个自用省钱佣金高,分享推广赚钱多的平台,百度有几百万篇报道,也期待你的加入。万方导师高省邀请码005500,注册送双皇冠会员,送万元推广大礼包,教你如
- 3286、穿越网格图的安全路径
Lenyiin
题解c++算法leetcode
3286、[中等]穿越网格图的安全路径1、题目描述给你一个mxn的二进制矩形grid和一个整数health表示你的健康值。你开始于矩形的左上角(0,0),你的目标是矩形的右下角(m-1,n-1)。你可以在矩形中往上下左右相邻格子移动,但前提是你的健康值始终是正数。对于格子(i,j),如果grid[i][j]=1,那么这个格子视为不安全的,会使你的健康值减少1。如果你可以到达最终的格子,请你返回tr
- 高考后该不该给孩子买电脑,什么情况能买?什么情况不能买?
寻求改变
我知道家长们很担心,怕买了电脑小孩沉迷游戏,耽误了学业,也不利于身体健康。对于准大学生来说,基本上在18岁左右,也不算小了,但在很多父母眼里,依旧是个小孩子。数据显示,这种情况是有发生的,大学生约70%的电脑主要被用于玩网络游戏,如果没有养成一个用良好的习惯,对孩子影响是非常大的。我总结为三买,三不买。最近有看到群里很多家长再问,小孩上大学该不该给他买电脑,要买和不买两种观点的家长都有,那么哪种情
- 莆田鞋在哪买?推荐二个靠谱渠道
美鞋之家
莆田鞋在哪里买,莆田鞋一般在实体店或莆田鞋店购买,我觉得很多莆田鞋都是在莆田、广州、上海的鞋类批发市场购买的,价格非常方便。如果你想做莆田鞋生意,你可以去这些地方,如果你只想买一双莆田鞋穿,你可以在天猫和淘宝上买,因为淘宝的费用一般比较便宜。详细咨询VX→a40273莆田鞋在哪里买1、微商上购买,做莆田鞋微商代理的人群非常多,价格也比较实惠,但是也难免遇见高价卖的微商人群。其实莆田鞋的水很深,买之
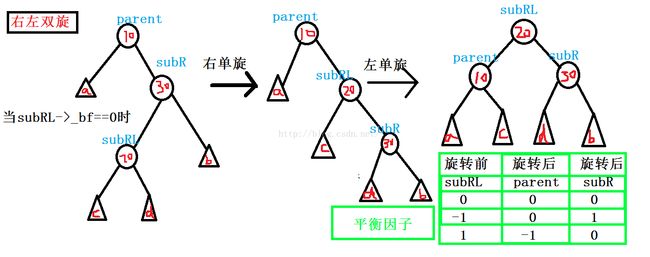
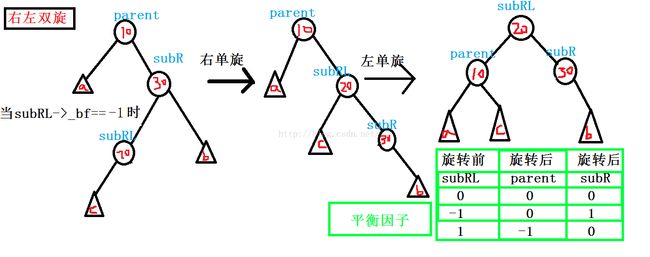
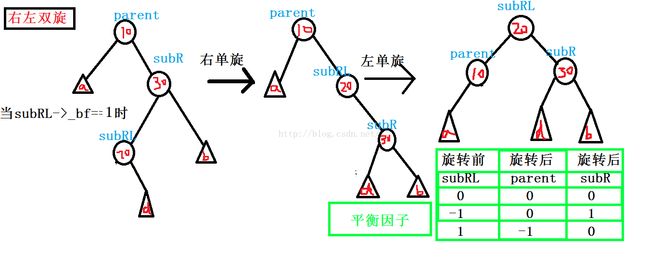
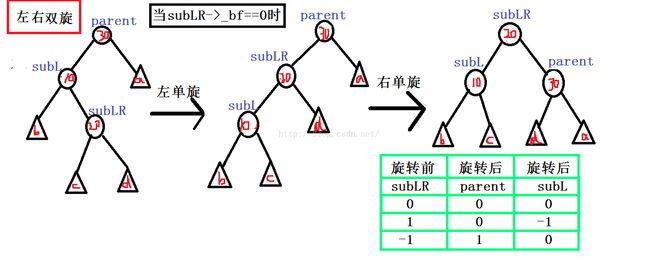
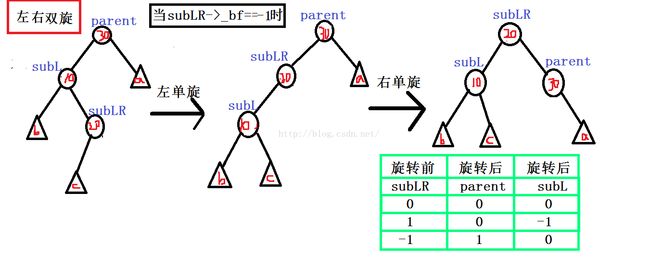
- 《 C++ 修炼全景指南:十 》自平衡的艺术:深入了解 AVL 树的核心原理与实现
Lenyiin
C++修炼全景指南技术指南c++数据结构stl
摘要本文深入探讨了AVL树(自平衡二叉搜索树)的概念、特点以及实现细节。我们首先介绍了AVL树的基本原理,并详细分析了其四种旋转操作,包括左旋、右旋、左右双旋和右左双旋,阐述了它们在保持树平衡中的重要作用。接着,本文从头到尾详细描述了AVL树的插入、删除和查找操作,配合完整的代码实现和详尽的注释,使读者能够全面理解这些操作的执行过程。此外,我们还提供了AVL树的遍历方法,包括中序、前序和后序遍历,
- 【树一线性代数】005入门
Owlet_woodBird
算法
Index本文稍后补全,推荐阅读:https://blog.csdn.net/weixin_60702024/article/details/141874376分析实现总结本文稍后补全,推荐阅读:https://blog.csdn.net/weixin_60702024/article/details/141874376已知非空二叉树T的结点值均为正整数,采用顺序存储方式保存,数据结构定义如下:t
- 每天赚50零花钱的方法,日赚50元左右的5个正规渠道
一起高省
每个人都希望拥有一笔自己的零花钱,但是很多人可能没有太多的时间去赚钱,或者没有太多的机会去赚取收入。但是,你可以通过一些简单的方法来赚取每天50元的零花钱。下面分享一些能每天赚50元的方法门路,总有一个适合你!①电商——高省高省APP佣金更高,模式更好,终端用户不流失。【高省】是一个自用省钱佣金高,分享推广赚钱多的平台,百度有几百万篇报道,也期待你的加入。拂晓导师高省邀请码989898,注册送双皇
- 周末充实的一天
敬亭山123
今天从上午10:45到下午5:30左右,一共学习了六个多小时的德语,另外还处理了30分钟左右的工作!值得一提的是,学习效率很高效!给自己鼓掌!PS:今天是在附近的如家蹭的暖气,蹭到下午三点多的时候实在不好意思了,就到隔壁水果店买了40块钱左右的水果送酒店前台。下午6点多,酒店前台忍着寒风,把门开了半个小时,委婉的催我离开了酒店了。如家酒店高效学习一天的心情就如下图——岁月流转中上海的冬天一样美丽多
- 辰辰日记四十四
谷子
昨天是重阳节,当然我们都没有刻意提起这个节日,似乎并不重要,生长在城市,连爬山都不是寻常事。人人都是忙碌之人,虽然人人也都难以说清自己都在忙些什么。其实这一天以又是你外婆的生日。原来妈妈想好给外婆买一双鞋子,不过,这段时间外婆生病了。病得挺严重,无法睡眠,不能行走。辰辰,你一定不能想象这是一种什么情况,人怎么会无法睡觉,躺不下床。当人颈椎有伤,且血管不通畅就会这样。你一躺下就整个人头晕目眩。那就是
- 27岁儿子不结婚,父亲决定先给自己相亲找个伴:我要给儿子打个样
清白路人
你妈逼你结婚了吗?现在的年轻人普遍结婚都比较晚,有的三十好几了八字还没一撇,这个时候最操心的就是父母了,他们话里话外都是催促着子女找对象结婚,甚至还会用出让人意想不到的方法,其实说到底,这也是可怜天下父母心呐。亲自给儿子打样的大爷李荣国,54岁,离异,打工收入2000多左右,家住农村平房120平。李大爷年轻时一直在工厂和工地打工,4年前才回到村里在镇政府当起了保安。李大爷的收入来自于三个部分,第一
- leetcode-617. 合并二叉树
manba_
leetcodehot100leetcode算法
题目描述给你两棵二叉树:root1和root2。想象一下,当你将其中一棵覆盖到另一棵之上时,两棵树上的一些节点将会重叠(而另一些不会)。你需要将这两棵树合并成一棵新二叉树。合并的规则是:如果两个节点重叠,那么将这两个节点的值相加作为合并后节点的新值;否则,不为null的节点将直接作为新二叉树的节点。返回合并后的二叉树。注意:合并过程必须从两个树的根节点开始。示例1:输入:root1=[1,3,2,
- 连环画中的冷门绝技:汉代宝藏“画像石”的神奇搬运(高清)
江户川小歪
虽然连环画只有巴掌大小,但是打开它,你会发现一座中国美术技法的宝库。单线白描是大家最熟悉不过了的,还有工笔重彩、黑白色块、素描、版画、剪纸等种种技术百花齐放。从80年代开始,画家们各显神通,做了诸多尝试,以至于达到了“万法皆为我用”的境界。今天我来说说一种冷门的美术——汉画像石。汉画像石是个什么东西?古时候,王族、有钱人会在墓穴的石头、砖头上绘制一些图案,这些东西有雕刻和绘画的双属性,在美术形式上
- 使用datepicker和uploadify的冲突解决(IE双击才能打开附件上传对话框)
zhanglb12
在开发的过程当中,IE的兼容无疑是我们的一块绊脚石,在我们使用的如期的datepicker插件和使用上传附件的uploadify插件的时候,两者就产生冲突,只要点击过时间的插件,uploadify上传框要双才能打开ie浏览器提示错误Missinginstancedataforthisdatepicker解决方案//if(.browser.msie&&'9.0'===.browser.version
- leetcode刷题day19|二叉树Part07(235. 二叉搜索树的最近公共祖先、701.二叉搜索树中的插入操作、450.删除二叉搜索树中的节点)
小冉在学习
leetcode算法数据结构
235.二叉搜索树的最近公共祖先思路:二叉搜索树首先考虑中序遍历。根据二叉搜索树的特性,如果p,q分别在中间节点的左右两边,该中间节点一定是最近公共祖先,如果在同一侧,则递归这一侧即可。递归三部曲:1、传入参数:根节点,p,q,返回节点。2、终止条件:因为p,q一定存在,所以不会遍历到树的最底层,因此可以不写终止条件3、递归逻辑:如果p,q均小于root的值,递归调用左子树;如果p,q均大于roo
- 是“王者荣耀”还是“王者农药”?
颓废思物者
近些日子,王者又双叒叕火了。而腾讯公司的老总马化腾也跟着他的游戏在人声鼎沸的汪洋中飘荡——我最先是在公众号文章《腾讯推出游戏未保“双减双打”新措施》中看见了未成年人将减少在线时长限制,非节假日从1.5小时降低至1小时,节假日从3小时减到2小时。心中不由掀起一丝波澜:又有家长对游戏出手了。不过大家心中你知我知,在这场纷争中,必定也带着市场的挤压和变化。除去这些市场变化,我们来探讨几个问题:1.没有游
- 2019年6月24日(写给我的宝贝苏诗雨6)坚持才能胜利
e5bd79d99424
晚上8点左右跟宝宝商量先弹30分钟钢琴,时间到了吃点水果再弹30分钟。平时告诉她以后会主动的座在钢琴前弹琴,今天反常,不高兴的说不想弹琴,太累了,一遍一遍的!我对宝宝说:学习就是要这样,反反复复,为的是让你更熟练的掌握。还是撅着嘴找各种理由……我对宝宝说,妈妈跟你探讨一个问题,你告诉妈妈你的答案。当时钢琴是你自己选择的对吗?宝宝点点头。那你当时是喜欢它的对吗?答是的。你现在喜欢它吗?答喜欢。喜欢怎
- 2021-10-19
清风似你
感恩日记姓名:黄振红日期:10月19日✨感恩时刻内容:1.感恩爸爸妈妈给予我生命,并养育我长大2.感恩自己今天能够健康的睁开眼睛活在这个世界上,因此可以做更多自己想做的事3.感恩自己健康我以后会好好的爱你们。5.感恩我有健康可爱帅气,漂亮,善良,懂事聪明的一双儿女,给我做午饭(西辣蛋),给我说“早点吃完饭,午休”6.感恩我有个健康有责任感疼我爱我的老公,打电话关心我,晚上接女儿,并且为我们做好丰盛
- 面向对象面向过程
3213213333332132
java
面向对象:把要完成的一件事,通过对象间的协作实现。
面向过程:把要完成的一件事,通过循序依次调用各个模块实现。
我把大象装进冰箱这件事为例,用面向对象和面向过程实现,都是用java代码完成。
1、面向对象
package bigDemo.ObjectOriented;
/**
* 大象类
*
* @Description
* @author FuJian
- Java Hotspot: Remove the Permanent Generation
bookjovi
HotSpot
openjdk上关于hotspot将移除永久带的描述非常详细,http://openjdk.java.net/jeps/122
JEP 122: Remove the Permanent Generation
Author Jon Masamitsu
Organization Oracle
Created 2010/8/15
Updated 2011/
- 正则表达式向前查找向后查找,环绕或零宽断言
dcj3sjt126com
正则表达式
向前查找和向后查找
1. 向前查找:根据要匹配的字符序列后面存在一个特定的字符序列(肯定式向前查找)或不存在一个特定的序列(否定式向前查找)来决定是否匹配。.NET将向前查找称之为零宽度向前查找断言。
对于向前查找,出现在指定项之后的字符序列不会被正则表达式引擎返回。
2. 向后查找:一个要匹配的字符序列前面有或者没有指定的
- BaseDao
171815164
seda
import java.sql.Connection;
import java.sql.DriverManager;
import java.sql.SQLException;
import java.sql.PreparedStatement;
import java.sql.ResultSet;
public class BaseDao {
public Conn
- Ant标签详解--Java命令
g21121
Java命令
这一篇主要介绍与java相关标签的使用 终于开始重头戏了,Java部分是我们关注的重点也是项目中用处最多的部分。
1
- [简单]代码片段_电梯数字排列
53873039oycg
代码
今天看电梯数字排列是9 18 26这样呈倒N排列的,写了个类似的打印例子,如下:
import java.util.Arrays;
public class 电梯数字排列_S3_Test {
public static void main(S
- Hessian原理
云端月影
hessian原理
Hessian 原理分析
一. 远程通讯协议的基本原理
网络通信需要做的就是将流从一台计算机传输到另外一台计算机,基于传输协议和网络 IO 来实现,其中传输协议比较出名的有 http 、 tcp 、 udp 等等, http 、 tcp 、 udp 都是在基于 Socket 概念上为某类应用场景而扩展出的传输协
- 区分Activity的四种加载模式----以及Intent的setFlags
aijuans
android
在多Activity开发中,有可能是自己应用之间的Activity跳转,或者夹带其他应用的可复用Activity。可能会希望跳转到原来某个Activity实例,而不是产生大量重复的Activity。
这需要为Activity配置特定的加载模式,而不是使用默认的加载模式。 加载模式分类及在哪里配置
Activity有四种加载模式:
standard
singleTop
- hibernate几个核心API及其查询分析
antonyup_2006
html.netHibernatexml配置管理
(一) org.hibernate.cfg.Configuration类
读取配置文件并创建唯一的SessionFactory对象.(一般,程序初始化hibernate时创建.)
Configuration co
- PL/SQL的流程控制
百合不是茶
oraclePL/SQL编程循环控制
PL/SQL也是一门高级语言,所以流程控制是必须要有的,oracle数据库的pl/sql比sqlserver数据库要难,很多pl/sql中有的sqlserver里面没有
流程控制;
分支语句 if 条件 then 结果 else 结果 end if ;
条件语句 case when 条件 then 结果;
循环语句 loop
- 强大的Mockito测试框架
bijian1013
mockito单元测试
一.自动生成Mock类 在需要Mock的属性上标记@Mock注解,然后@RunWith中配置Mockito的TestRunner或者在setUp()方法中显示调用MockitoAnnotations.initMocks(this);生成Mock类即可。二.自动注入Mock类到被测试类 &nbs
- 精通Oracle10编程SQL(11)开发子程序
bijian1013
oracle数据库plsql
/*
*开发子程序
*/
--子程序目是指被命名的PL/SQL块,这种块可以带有参数,可以在不同应用程序中多次调用
--PL/SQL有两种类型的子程序:过程和函数
--开发过程
--建立过程:不带任何参数
CREATE OR REPLACE PROCEDURE out_time
IS
BEGIN
DBMS_OUTPUT.put_line(systimestamp);
E
- 【EhCache一】EhCache版Hello World
bit1129
Hello world
本篇是EhCache系列的第一篇,总体介绍使用EhCache缓存进行CRUD的API的基本使用,更细节的内容包括EhCache源代码和设计、实现原理在接下来的文章中进行介绍
环境准备
1.新建Maven项目
2.添加EhCache的Maven依赖
<dependency>
<groupId>ne
- 学习EJB3基础知识笔记
白糖_
beanHibernatejbosswebserviceejb
最近项目进入系统测试阶段,全赖袁大虾领导有力,保持一周零bug记录,这也让自己腾出不少时间补充知识。花了两天时间把“传智播客EJB3.0”看完了,EJB基本的知识也有些了解,在这记录下EJB的部分知识,以供自己以后复习使用。
EJB是sun的服务器端组件模型,最大的用处是部署分布式应用程序。EJB (Enterprise JavaBean)是J2EE的一部分,定义了一个用于开发基
- angular.bootstrap
boyitech
AngularJSAngularJS APIangular中文api
angular.bootstrap
描述:
手动初始化angular。
这个函数会自动检测创建的module有没有被加载多次,如果有则会在浏览器的控制台打出警告日志,并且不会再次加载。这样可以避免在程序运行过程中许多奇怪的问题发生。
使用方法: angular .
- java-谷歌面试题-给定一个固定长度的数组,将递增整数序列写入这个数组。当写到数组尾部时,返回数组开始重新写,并覆盖先前写过的数
bylijinnan
java
public class SearchInShiftedArray {
/**
* 题目:给定一个固定长度的数组,将递增整数序列写入这个数组。当写到数组尾部时,返回数组开始重新写,并覆盖先前写过的数。
* 请在这个特殊数组中找出给定的整数。
* 解答:
* 其实就是“旋转数组”。旋转数组的最小元素见http://bylijinnan.iteye.com/bl
- 天使还是魔鬼?都是我们制造
ducklsl
生活教育情感
----------------------------剧透请原谅,有兴趣的朋友可以自己看看电影,互相讨论哦!!!
从厦门回来的动车上,无意中瞟到了书中推荐的几部关于儿童的电影。当然,这几部电影可能会另大家失望,并不是类似小鬼当家的电影,而是关于“坏小孩”的电影!
自己挑了两部先看了看,但是发现看完之后,心里久久不能平
- [机器智能与生物]研究生物智能的问题
comsci
生物
我想,人的神经网络和苍蝇的神经网络,并没有本质的区别...就是大规模拓扑系统和中小规模拓扑分析的区别....
但是,如果去研究活体人类的神经网络和脑系统,可能会受到一些法律和道德方面的限制,而且研究结果也不一定可靠,那么希望从事生物神经网络研究的朋友,不如把
- 获取Android Device的信息
dai_lm
android
String phoneInfo = "PRODUCT: " + android.os.Build.PRODUCT;
phoneInfo += ", CPU_ABI: " + android.os.Build.CPU_ABI;
phoneInfo += ", TAGS: " + android.os.Build.TAGS;
ph
- 最佳字符串匹配算法(Damerau-Levenshtein距离算法)的Java实现
datamachine
java算法字符串匹配
原文:http://www.javacodegeeks.com/2013/11/java-implementation-of-optimal-string-alignment.html------------------------------------------------------------------------------------------------------------
- 小学5年级英语单词背诵第一课
dcj3sjt126com
englishword
long 长的
show 给...看,出示
mouth 口,嘴
write 写
use 用,使用
take 拿,带来
hand 手
clever 聪明的
often 经常
wash 洗
slow 慢的
house 房子
water 水
clean 清洁的
supper 晚餐
out 在外
face 脸,
- macvim的使用实战
dcj3sjt126com
macvim
macvim用的是mac里面的vim, 只不过是一个GUI的APP, 相当于一个壳
1. 下载macvim
https://code.google.com/p/macvim/
2. 了解macvim
:h vim的使用帮助信息
:h macvim
- java二分法查找
蕃薯耀
java二分法查找二分法java二分法
java二分法查找
>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>
蕃薯耀 2015年6月23日 11:40:03 星期二
http:/
- Spring Cache注解+Memcached
hanqunfeng
springmemcached
Spring3.1 Cache注解
依赖jar包:
<!-- simple-spring-memcached -->
<dependency>
<groupId>com.google.code.simple-spring-memcached</groupId>
<artifactId>simple-s
- apache commons io包快速入门
jackyrong
apache commons
原文参考
http://www.javacodegeeks.com/2014/10/apache-commons-io-tutorial.html
Apache Commons IO 包绝对是好东西,地址在http://commons.apache.org/proper/commons-io/,下面用例子分别介绍:
1) 工具类
2
- 如何学习编程
lampcy
java编程C++c
首先,我想说一下学习思想.学编程其实跟网络游戏有着类似的效果.开始的时候,你会对那些代码,函数等产生很大的兴趣,尤其是刚接触编程的人,刚学习第一种语言的人.可是,当你一步步深入的时候,你会发现你没有了以前那种斗志.就好象你在玩韩国泡菜网游似的,玩到一定程度,每天就是练级练级,完全是一个想冲到高级别的意志力在支持着你.而学编程就更难了,学了两个月后,总是觉得你好象全都学会了,却又什么都做不了,又没有
- 架构师之spring-----spring3.0新特性的bean加载控制@DependsOn和@Lazy
nannan408
Spring3
1.前言。
如题。
2.描述。
@DependsOn用于强制初始化其他Bean。可以修饰Bean类或方法,使用该Annotation时可以指定一个字符串数组作为参数,每个数组元素对应于一个强制初始化的Bean。
@DependsOn({"steelAxe","abc"})
@Comp
- Spring4+quartz2的配置和代码方式调度
Everyday都不同
代码配置spring4quartz2.x定时任务
前言:这些天简直被quartz虐哭。。因为quartz 2.x版本相比quartz1.x版本的API改动太多,所以,只好自己去查阅底层API……
quartz定时任务必须搞清楚几个概念:
JobDetail——处理类
Trigger——触发器,指定触发时间,必须要有JobDetail属性,即触发对象
Scheduler——调度器,组织处理类和触发器,配置方式一般只需指定触发
- Hibernate入门
tntxia
Hibernate
前言
使用面向对象的语言和关系型的数据库,开发起来很繁琐,费时。由于现在流行的数据库都不面向对象。Hibernate 是一个Java的ORM(Object/Relational Mapping)解决方案。
Hibernte不仅关心把Java对象对应到数据库的表中,而且提供了请求和检索的方法。简化了手工进行JDBC操作的流程。
如
- Math类
xiaoxing598
Math
一、Java中的数字(Math)类是final类,不可继承。
1、常数 PI:double圆周率 E:double自然对数
2、截取(注意方法的返回类型) double ceil(double d) 返回不小于d的最小整数 double floor(double d) 返回不大于d的整最大数 int round(float f) 返回四舍五入后的整数 long round