Neo__Z
Neo__Z

bzoj4823 cqoi2017 老C的方块【最大流】

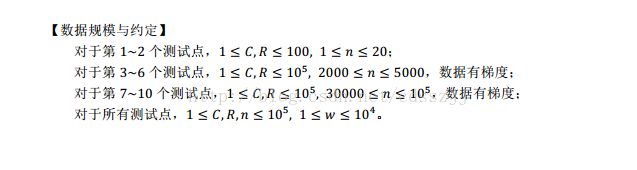
bzoj4823 cqoi2017 老C的方块【最大流】_第1张图片bzoj4823 cqoi2017 老C的方块【最大流】_第2张图片bzoj4823 cqoi2017 老C的方块【最大流】_第3张图片bzoj4823 cqoi2017 老C的方块【最大流】_第4张图片bzoj4823 cqoi2017 老C的方块【最大流】_第5张图片

看到题目其实感觉很麻烦，不知道从哪里入手，那么仔细观察所给出的有用信息...


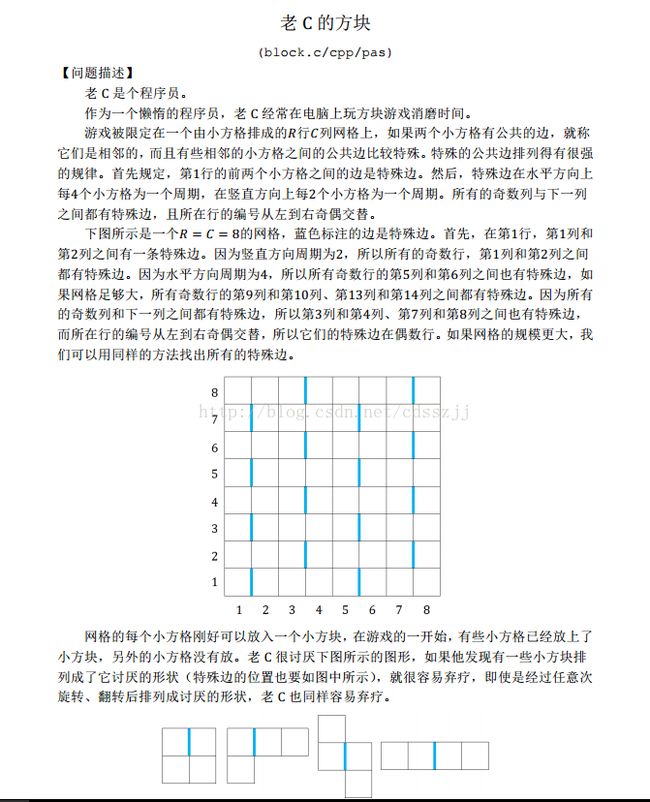
我们考虑网格图是一个含有挡板的图，这个挡板的分布很有规律，大概是每一行的相邻两个挡板都隔了四个格子，并且奇数行的排列相同，偶数行的排列相同...


然后考虑不合法的方块形状有什么共同点：仔细观察就会发现，所有的不合法图形中，挡板的左边至少有一个格子，右边至少有一个格子，并且左边的格子连着一个格子，右边的的格子连着一个格子...也就是说，其实我们如果要使得整张图的所有方块构成的图形全部合法就要满足下图中如果挡板两边的紫色格子都有方块存放的话，那么，和这两个紫色格子相邻的色格子和黑色格子不能同时存在...

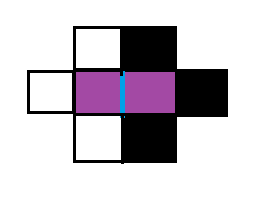
bzoj4823 cqoi2017 老C的方块【最大流】_第6张图片

我们发现刚好相邻隔板之间的四个格子就是为不合法图案而设计的...

于是就变成了经典的限制问题...经典的最小割...

如果不考虑紫色的格子，那么这整张网格图就是一个二分图...我们给这张图染色...

bzoj4823 cqoi2017 老C的方块【最大流】_第7张图片

那么对于所有的白点，我们连的边，对于所有的黑点我们连的边，然后因为要保证紫色格子周围黑白点不能同时存在，所以，对于所有的黑点，我们从紫色格子像黑点连$inf$的边，从白点像紫色格子连$inf$的边，然后因为我们两个紫色格子不同时存在的时候黑白点是可以同时存在的，所以两个紫色格子之间连min(w[x],w[y])的边...然后求最小割就好了...

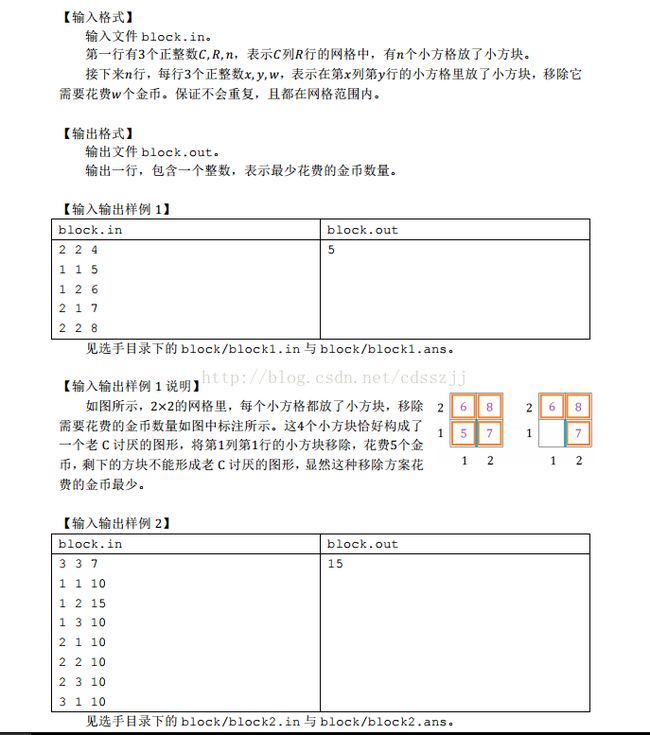
注意题目输入是先输列，后输行；

注意两个紫色格子也要分开讨论，所以共有四层，可以发现每个2*4的区域都是一样的，所以可以预处理出颜色；

讨论时要注意不能把边建重了，特别是连源点，会点的边。

#include
#include
#include
#include
#include
#include
#include
using namespace std; 

int getint()
{
	int i=0,f=1;char c;
	for(c=getchar();(c<'0'||c>'9')&&c!='-';c=getchar());
	if(c=='-')f=-1,c=getchar();
	for(;c>='0'&&c<='9';c=getchar())i=(i<<3)+(i<<1)+c-'0';
	return i*f;
}

const int N=1e5+5,M=2e6+5,INF=0x3f3f3f3f;
const int color[2][4]={2,1,4,3,1,2,3,4};
const int fx[4]={0,1,0,-1};
const int fy[4]={-1,0,1,0};
int r,c,n,cnt,src,des;
int val[N],type[N];
int tot=1,first[N],cur[N],step[N],next[M],to[M],cap[M],from[M];
bool visit[N];
map,int>p;

inline void add(const int &x,const int &y,const int &w)
{
	next[++tot]=first[x],first[x]=tot,to[tot]=y,cap[tot]=w,from[tot]=x;
	next[++tot]=first[y],first[y]=tot,to[tot]=x,cap[tot]=0;
}

inline bool bfs()
{
	static int que[N];
	int tail=1;
	for(int i=src;i<=des;i++)step[i]=-1,cur[i]=first[i];
	que[tail]=src,step[src]=0;
	for(int head=1;head<=tail;head++)
	{
		int u=que[head];
		for(int e=first[u];e;e=next[e])
		{
			int v=to[e];
			if(cap[e]>0&&step[v]==-1)
			{
				step[v]=step[u]+1;
				que[++tail]=v;
				if(v==des)return true;
			}
		}
	}
	return false;
}

inline int dinic(const int &u,const int &flow)
{
	if(u==des)return flow;
	int res=0;
	for(int &e=cur[u];e;e=next[e])
	{
		int v=to[e];
		if(cap[e]>0&&step[v]>step[u])
		{
			int delta=dinic(v,min(flow-res,cap[e]));
			if(delta)
			{
				cap[e]-=delta,cap[e^1]+=delta;
				res+=delta;if(res==flow)break;
			}
		}
	}
	if(res!=flow)step[u]=-1;
	return res;
}

inline int maxflow()
{
	int ans=0;
	while(bfs())ans+=dinic(src,INF); 
	return ans;
}

int main()
{
	//freopen("lx.in","r",stdin);
	r=getint(),c=getint(),n=getint();
	src=0,des=n+1;
	int x,y,u,v;
	for(int i=1;i<=n;i++)
	{
		y=getint(),x=getint(),val[i]=getint();
		p[make_pair(x,y)]=i;
		type[i]=color[x%2][y%4];
	}
	map,int>::iterator it;
	for(it=p.begin();it!=p.end();it++)
	{
		x=it->first.first;
		y=it->first.second;
		u=it->second;
		if(type[u]==3)
			for(int k=0;k<4;k++)
			{
				int nowx=x+fx[k],nowy=y+fy[k];
				if(p.count(make_pair(nowx,nowy)))
				{
					v=p[make_pair(nowx,nowy)];
					if(type[v]==4)
					{
						if(!visit[v])add(src,v,val[v]),visit[v]=true;
						add(v,u,INF);
					}
					if(type[v]==2)
						add(u,v,min(val[u],val[v]));
				}
			}
		if(type[u]==2)
			for(int k=0;k<4;k++)
			{
				int nowx=x+fx[k],nowy=y+fy[k];
				if(p.count(make_pair(nowx,nowy)))
				{
					v=p[make_pair(nowx,nowy)];
					if(type[v]==1)
					{
						if(!visit[v])add(v,des,val[v]),visit[v]=true;
						add(u,v,INF);
					}
				}
			}
	}
	cout<


你可能感兴趣的:(bzoj4823 cqoi2017 老C的方块【最大流】)

  • 斤斤计较的婚姻到底有多难？ 白心之岂必有为
    很多人私聊我会问到在哪个人群当中斤斤计较的人最多？我都会回答他，一般婚姻出现问题的斤斤计较的人士会非常多，以我多年经验，在婚姻落的一塌糊涂的人当中，斤斤计较的人数占比在20～30%以上，也就是说10个婚姻出现问题的斤斤计较的人有2-3个有多不减。在婚姻出问题当中，有大量的心理不平衡的、尖酸刻薄的怨妇。在婚姻中仅斤斤计较有两种类型：第一种是物质上的，另一种是精神上的。在物质与精神上抠门已经严重的影响
  • 情绪觉察日记第37天 露露_e800
    今天是家庭关系规划师的第二阶最后一天，慧萍老师帮我做了个案，帮我处理了埋在心底好多年的一份恐惧，并给了我深深的力量！这几天出来学习，爸妈过来婆家帮我带小孩，妈妈出于爱帮我收拾东西，并跟我先生和婆婆产生矛盾，妈妈觉得他们没有照顾好我…。今晚回家见到妈妈，我很欣赏她并赞扬她，妈妈说今晚要跟我睡我说好，当我们俩躺在床上准备睡觉的时候，我握着妈妈的手对她说:妈妈这几天辛苦你了，你看你多利害把我们的家收拾得
  • 芦花鞋一四 许叶晗
    又是在一个寒冷的夏日里，青铜和葵花决定今天一起去卖芦花鞋，奶奶亲手给他们做了一碗热乎乎的粥对他们说:“就靠你们两挣生活费了这碗粥赶紧趁热喝了吧！”于是青铜和葵花喝完了奶奶给她们做的粥，就准备去镇上卖卢花鞋，这回青铜和葵花穿着新的芦花鞋来到了镇上。青铜这回看到了很多人都在卖，用手势表达对葵花说:“这回有好多人在抢我们生意呢！我们必须得吆喝起来。”葵花点了点头。可是谁知他们也大声的叫，卖芦花喽！卖芦花
  • QQ群采集助手，精准引流必备神器 2401_87347160 其他经验分享
    功能概述微信群查找与筛选工具是一款专为微信用户设计的辅助工具，它通过关键词搜索功能，帮助用户快速找到相关的微信群，并提供筛选是否需要验证的群组的功能。主要功能关键词搜索：用户可以输入关键词，工具将自动查找包含该关键词的微信群。筛选功能：工具提供筛选机制，用户可以选择是否只显示需要验证或不需要验证的群组。精准引流：通过上述功能，用户可以更精准地找到目标群组，进行有效的引流操作。3.设备需求该工具可以
  • 关于沟通这件事，项目经理不需要每次都面对面进行 流程大师兄
    很多项目经理都会遇到这样的问题，项目中由于事情太多，根本没有足够的时间去召开会议，那在这种情况下如何去有效地管理项目中的利益相关者？当然，不建议电子邮件也不需要开会的话，建议可以采取下面几种方式来形成有效的沟通，这几种方式可以帮助你努力的通过各种办法来保持和各方面的联系。项目经理首先要问自己几个问题，项目中哪些利益相关者是必须要进行沟通的？可以列出项目中所有的利益相关者清单，同时也整理出项目中哪些
  • 机器学习与深度学习间关系与区别 ℒℴѵℯ心·动ꦿ໊ོ꫞ 人工智能学习深度学习python
    一、机器学习概述定义机器学习（MachineLearning,ML）是一种通过数据驱动的方法，利用统计学和计算算法来训练模型，使计算机能够从数据中学习并自动进行预测或决策。机器学习通过分析大量数据样本，识别其中的模式和规律，从而对新的数据进行判断。其核心在于通过训练过程，让模型不断优化和提升其预测准确性。主要类型1.监督学习（SupervisedLearning）监督学习是指在训练数据集中包含输入
  • android系统selinux中添加新属性property 辉色投像
    1.定位/android/system/sepolicy/private/property_contexts声明属性开头：persist.charge声明属性类型：u:object_r:system_prop:s0图12.定位到android/system/sepolicy/public/domain.te删除neverallow{domain-init}default_prop:property
  • 铭刻于星（四十二） 随风至
    69夜晚，绍敏同学做完功课后，看了眼房外，没听到动静才敢从书包的夹层里拿出那个心形纸团。折痕压得很深，都有些旧了，想来是已经写好很久了。绍敏同学慢慢地、轻轻地捏开折叠处，待到全部拆开后，又反复抚平纸张，然后仔细地一字字默看。只是开头的三个字是第一次看到，让她心漏跳了几拍。“亲爱的绍敏：从四年级的时候，我就喜欢你了，但是我一直不敢说，怕影响你学习。六年级的时候听说有人跟你表白，你接受了，我很难过，但
  • 底层逆袭到底有多难，不甘平凡的你准备好了吗？让吴起给你说说 造命者说
    底层逆袭到底有多难，不甘平凡的你准备好了吗？让吴起给你说说我叫吴起，生于公元前440年的战国初期，正是群雄并起、天下纷争不断的时候。后人说我是军事家、政治家、改革家，是兵家代表人物。评价我一生历仕鲁、魏、楚三国，通晓兵家、法家、儒家三家思想，在内政军事上都有极高的成就。周安王二十一年（公元前381年），因变法得罪守旧贵族，被人乱箭射死。我出生在卫国一个“家累万金”的富有家庭，从年轻时候起就不甘平凡
  • 2020-01-25 晴岚85
    郑海燕坚持分享590天2020.1.24在生活中只存在两个问题。一个问题是：你知道想要达成的目标是什么，但却不知道如何才能达成；另一个问题是：你不知道你的目标是什么。前一个是行动的问题，后一个是结果的问题。通过制定具体的下一步行动，可以解决不知道如何开始行动的问题。而通过去想象结果，对结果做预估，可以解决找不着目标的问题。对于所有吸引我们注意力，想要完成的任务，你可以先想象一下，预期的结果究竟是什
  • 随笔 | 仙一般的灵气 海思沧海
    仙岛今天，我看了你全部，似乎已经进入你的世界我不知道，这是否是梦幻，还是你仙一般的灵气吸引了我也许每一个人都要有一份属于自己的追求，这样才能够符合人生的梦想，生活才能够充满着阳光与快乐我不知道，我为什么会这样的感叹，是在感叹自己的人生，还是感叹自己一直没有孜孜不倦的追求只感觉虚度了光阴，每天活在自己的梦中，活在一个不真实的世界是在逃避自己，还是在逃避周围的一切有时候我嘲笑自己，嘲笑自己如此的虚无，
  • 想家 爆米花机
    也许不同于大家对家乡的思念，我对家乡甚至是疯狂的不舍。还未踏出车站就感觉到幸福，我享受这里的夕阳、这里的浓烈柴火味、这里每一口家常菜。我是宅女，我贪恋家的安逸。刚刚踏出大学校门，初出茅庐，无法适应每年只能国庆和春节回家。我焦虑、失眠、无端发脾气，是无法适应工作的节奏，是无法接受我将一步步离开家乡的事实。我不想承认自己胸无大志，选择再次踏上征程。图片发自App
  • 【iOS】MVC设计模式 Magnetic_h iosmvc设计模式objective-c学习ui
    MVC前言如何设计一个程序的结构，这是一门专门的学问，叫做"架构模式"（architecturalpattern），属于编程的方法论。MVC模式就是架构模式的一种。它是Apple官方推荐的App开发架构，也是一般开发者最先遇到、最经典的架构。MVC各层controller层Controller/ViewController/VC（控制器）负责协调Model和View，处理大部分逻辑它将数据从Mod
  • OC语言多界面传值五大方式 Magnetic_h iosui学习objective-c开发语言
    前言在完成暑假仿写项目时，遇到了许多需要用到多界面传值的地方，这篇博客来总结一下比较常用的五种多界面传值的方式。属性传值属性传值一般用前一个界面向后一个界面传值，简单地说就是通过访问后一个视图控制器的属性来为它赋值，通过这个属性来做到从前一个界面向后一个界面传值。首先在后一个界面中定义属性@interfaceBViewController:UIViewController@propertyNSSt
  • 一百九十四章. 自相矛盾 巨木擎天
    唉！就这么一夜，林子感觉就像过了很多天似的，先是回了阳间家里，遇到了那么多不可思议的事情儿。特别是小伙伴们，第二次与自己见面时，僵硬的表情和恐怖的气氛，让自己如坐针毡，打从心眼里难受！还有东子，他现在还好吗？有没有被人欺负？护城河里的小鱼小虾们，还都在吗？水不会真的干枯了吧？那对相亲相爱漂亮的太平鸟儿，还好吧！春天了，到了做窝、下蛋、喂养小鸟宝宝的时候了，希望它们都能够平安啊！虽然没有看见家人，也
  • UI学习——cell的复用和自定义cell Magnetic_h ui学习
    目录cell的复用手动（非注册）自动（注册）自定义cellcell的复用在iOS开发中，单元格复用是一种提高表格（UITableView）和集合视图（UICollectionView）滚动性能的技术。当一个UITableViewCell或UICollectionViewCell首次需要显示时，如果没有可复用的单元格，则视图会创建一个新的单元格。一旦这个单元格滚动出屏幕，它就不会被销毁。相反，它被添
  • element实现动态路由+面包屑 软件技术NINI vue案例vue.js前端
    el-breadcrumb是ElementUI组件库中的一个面包屑导航组件，它用于显示当前页面的路径，帮助用户快速理解和导航到应用的各个部分。在Vue.js项目中，如果你已经安装了ElementUI，就可以很方便地使用el-breadcrumb组件。以下是一个基本的使用示例：安装ElementUI（如果你还没有安装的话）:你可以通过npm或yarn来安装ElementUI。bash复制代码npmi
  • 10月|愿你的青春不负梦想-读书笔记-01 Tracy的小书斋
    本书的作者是俞敏洪，大家都很熟悉他了吧。俞敏洪老师是我行业的领头羊吧，也是我事业上的偶像。本日摘录他书中第一章中的金句：『一个人如果什么目标都没有，就会浑浑噩噩，感觉生命中缺少能量。能给我们能量的，是对未来的期待。第一件事，我始终为了进步而努力。与其追寻全世界的骏马，不如种植丰美的草原，到时骏马自然会来。第二件事，我始终有阶段性的目标。什么东西能给我能量？答案是对未来的期待。』读到这里的时候，我便
  • C语言宏函数 南林yan C语言c语言
    一、什么是宏函数？通过宏定义的函数是宏函数。如下，编译器在预处理阶段会将Add(x,y)替换为((x)*(y))#defineAdd(x,y)((x)*(y))#defineAdd(x,y)((x)*(y))intmain(){inta=10;intb=20;intd=10;intc=Add(a+d,b)*2;cout<
  • 地推话术，如何应对地推过程中家长的拒绝 校师学
    相信校长们在做地推的时候经常遇到这种情况：市场专员反馈家长不接单，咨询师反馈难以邀约这些家长上门，校区地推疲软，招生难。为什么？仅从地推层面分析，一方面因为家长受到的信息轰炸越来越多，对信息越来越“免疫”；而另一方面地推人员的专业能力和营销话术没有提高，无法应对家长的拒绝，对有意向的家长也不知如何跟进，眼睁睁看着家长走远；对于家长的疑问，更不知道如何有技巧地回答，机会白白流失。由于回答没技巧和专业
  • 谢谢你们，爱你们！ 鹿游儿
    昨天家人去泡温泉，二个孩子也带着去，出发前一晚，匆匆下班，赶回家和孩子一起收拾。饭后，我拿出笔和本子（上次去澳门时做手帐的本子）写下了1\2\3\4\5\6\7\8\9,让后让小壹去思考，带什么出发去旅游呢？她在对应的数字旁边画上了，泳衣、泳圈、肖恩、内衣内裤、tapuy、拖鞋……画完后，就让她自己对着这个本子，将要带的，一一带上，没想到这次带的书还是这本《便便工厂》(晚上姑婆发照片过来，妹妹累得
  • C语言如何定义宏函数？ 小九格物 c语言
    在C语言中，宏函数是通过预处理器定义的，它在编译之前替换代码中的宏调用。宏函数可以模拟函数的行为，但它们不是真正的函数，因为它们在编译时不会进行类型检查，也不会分配存储空间。宏函数的定义通常使用#define指令，后面跟着宏的名称和参数列表，以及宏展开后的代码。宏函数的定义方式：1.基本宏函数：这是最简单的宏函数形式，它直接定义一个表达式。#defineSQUARE(x)((x)*(x))2.带参
  • 微服务下功能权限与数据权限的设计与实现 nbsaas-boot 微服务java架构
    在微服务架构下，系统的功能权限和数据权限控制显得尤为重要。随着系统规模的扩大和微服务数量的增加，如何保证不同用户和服务之间的访问权限准确、细粒度地控制，成为设计安全策略的关键。本文将讨论如何在微服务体系中设计和实现功能权限与数据权限控制。1.功能权限与数据权限的定义功能权限：指用户或系统角色对特定功能的访问权限。通常是某个用户角色能否执行某个操作，比如查看订单、创建订单、修改用户资料等。数据权限：
  • 理解Gunicorn：Python WSGI服务器的基石 范范0825 ipythonlinux运维
    理解Gunicorn：PythonWSGI服务器的基石介绍Gunicorn，全称GreenUnicorn，是一个为PythonWSGI（WebServerGatewayInterface）应用设计的高效、轻量级HTTP服务器。作为PythonWeb应用部署的常用工具，Gunicorn以其高性能和易用性著称。本文将介绍Gunicorn的基本概念、安装和配置，帮助初学者快速上手。1.什么是Gunico
  • 小丽成长记（四十三） 玲玲54321
    小丽发现，即使她好不容易调整好自己的心态下一秒总会有不确定的伤脑筋的事出现，一个接一个的问题，人生就没有停下的时候，小问题不断出现。不过她今天看的书，她接受了人生就是不确定的，厉害的人就是不断创造确定性，在Ta的领域比别人多的确定性就能让自己脱颖而出，显示价值从而获得的比别人多的利益。正是这样的原因，因为从前修炼自己太少，使得她现在在人生道路上打怪起来困难重重，她似乎永远摆脱不了那种无力感，有种习
  • 学点心理知识，呵护孩子健康 静候花开_7090
    昨天听了华中师范大学教育管理学系副教授张玲老师的《哪里才是学生心理健康的最后庇护所，超越教育与技术的思考》的讲座。今天又重新学习了一遍，收获匪浅。张玲博士也注意到了当今社会上的孩子由于心理问题导致的自残、自杀及伤害他人等恶性事件。她向我们普及了一个重要的命题，她说心理健康的一些基本命题，我们与我们通常的一些教育命题是不同的，她还举了几个例子，让我们明白我们原来以为的健康并非心理学上的健康。比如如果
  • 2021年12月19日，春蕾教育集团团建活动感受——黄晓丹 黄错错加油
    感受:1.从陌生到熟悉的过程。游戏环节让我们在轻松的氛围中得到了锻炼，也增长了不少知识。2.游戏过程中，我们贡献的是个人力量，展现的是团队的力量。它磨合的往往不止是工作的熟悉，更是观念上契合度的贴近。3.这和工作是一样的道理。在各自的岗位上，每个人摆正自己的位置、各司其职充分发挥才能，并团结一致劲往一处使，才能实现最大的成功。新知:1.团队精神需要不断地创新。过去，人们把创新看作是冒风险，现在人们
  • Cell Insight | 单细胞测序技术又一新发现，可用于HIV-1和Mtb共感染个体诊断 尐尐呅
    结核病是艾滋病合并其他疾病中导致患者死亡的主要原因。其中结核病由结核分枝杆菌（Mycobacteriumtuberculosis,Mtb）感染引起，获得性免疫缺陷综合症（艾滋病）由人免疫缺陷病毒（Humanimmunodeficiencyvirustype1,HIV-1）感染引起。国家感染性疾病临床医学研究中心/深圳市第三人民医院张国良团队携手深圳华大生命科学研究院吴靓团队，共同研究得出单细胞测序
  • c++ 的iostream 和 c++的stdio的区别和联系 黄卷青灯77 c++算法开发语言iostreamstdio
    在C++中，iostream和C语言的stdio.h都是用于处理输入输出的库，但它们在设计、用法和功能上有许多不同。以下是两者的区别和联系：区别1.编程风格iostream（C++风格）：C++标准库中的输入输出流类库，支持面向对象的输入输出操作。典型用法是cin（输入）和cout（输出），使用>操作符来处理数据。更加类型安全，支持用户自定义类型的输入输出。#includeintmain(){in
  • 瑶池防线 谜影梦蝶
    冥华虽然逃过了影梦的军队，但他是一个忠臣，他选择上报战况。败给影梦后成逃兵，高层亡尔还活着，七重天失守......随便一条，即可处死冥华。冥华自然是知道以仙界高层的习性此信一发自己必死无疑，但他还选择上报实情，因为责任。同样此信送到仙宫后，知道此事的人，大多数人都认定冥华要完了，所以上到仙界高层，下到扫大街的，包括冥华自己，全都准备好迎接冥华之死。如果仙界现在还属于两方之争的话，冥华必死无疑。然而
  • java杨辉三角 3213213333332132 java基础
    package com.algorithm; /** * @Description 杨辉三角 * @author FuJianyong * 2015-1-22上午10:10:59 */ public class YangHui { public static void main(String[] args) { //初始化二维数组长度 int[][] y
  • 《大话重构》之大布局的辛酸历史 白糖_ 重构
    《大话重构》中提到“大布局你伤不起”，如果企图重构一个陈旧的大型系统是有非常大的风险，重构不是想象中那么简单。我目前所在公司正好对产品做了一次“大布局重构”，下面我就分享这个“大布局”项目经验给大家。   背景         公司专注于企业级管理产品软件，企业有大中小之分，在2000年初公司用JSP/Servlet开发了一套针对中
  • 电驴链接在线视频播放源码 dubinwei 源码电驴播放器视频ed2k
    本项目是个搜索电驴（ed2k）链接的应用,借助于磁力视频播放器（官网： http://loveandroid.duapp.com/ 开放平台），可以实现在线播放视频，也可以用迅雷或者其他下载工具下载。 项目源码： http://git.oschina.net/svo/Emule,动态更新。也可从附件中下载。 项目源码依赖于两个库项目，库项目一链接： http://git.oschina.
  • Javascript中函数的toString()方法 周凡杨 JavaScriptjstoStringfunctionobject
    简述     The toString() method returns a string representing the source code of the function.     简译之，Javascript的toString()方法返回一个代表函数源代码的字符串。 句法     function.
  • struts处理自定义异常 g21121 struts
    很多时候我们会用到自定义异常来表示特定的错误情况，自定义异常比较简单，只要分清是运行时异常还是非运行时异常即可，运行时异常不需要捕获，继承自RuntimeException，是由容器自己抛出，例如空指针异常。 非运行时异常继承自Exception，在抛出后需要捕获，例如文件未找到异常。 此处我们用的是非运行时异常，首先定义一个异常LoginException: /** * 类描述：登录相
  • Linux中find常见用法示例 510888780 linux
    Linux中find常见用法示例 ·find   path   -option   [   -print ]   [ -exec   -ok   command ]   {} \; find命令的参数；
  • SpringMVC的各种参数绑定方式 Harry642 springMVC绑定表单
    1. 基本数据类型(以int为例，其他类似)： Controller代码： @RequestMapping("saysth.do") public void test(int count) { } 表单代码： <form action="saysth.do" method="post&q
  • Java 获取Oracle ROWID aijuans javaoracle
    A ROWID is an identification tag unique for each row of an Oracle Database table. The ROWID can be thought of as a virtual column, containing the ID for each row. The oracle.sql.ROWID class i
  • java获取方法的参数名 antlove javajdkparametermethodreflect
    reflect.ClassInformationUtil.java package reflect; import javassist.ClassPool; import javassist.CtClass; import javassist.CtMethod; import javassist.Modifier; import javassist.bytecode.CodeAtt
  • JAVA正则表达式匹配 查找 替换 提取操作 百合不是茶 java正则表达式替换提取查找
    正则表达式的查找;主要是用到String类中的split();       String str;      str.split();方法中传入按照什么规则截取,返回一个String数组   常见的截取规则: str.split("\\.")按照.来截取 str.
  • Java中equals()与hashCode()方法详解 bijian1013 javasetequals()hashCode()
    一.equals()方法详解     equals()方法在object类中定义如下：  public boolean equals(Object obj) { return (this == obj); }    很明显是对两个对象的地址值进行的比较（即比较引用是否相同）。但是我们知道，String 、Math、I
  • 精通Oracle10编程SQL(4)使用SQL语句 bijian1013 oracle数据库plsql
    --工资级别表 create table SALGRADE ( GRADE NUMBER(10), LOSAL NUMBER(10,2), HISAL NUMBER(10,2) ) insert into SALGRADE values(1,0,100); insert into SALGRADE values(2,100,200); inser
  • 【Nginx二】Nginx作为静态文件HTTP服务器 bit1129 HTTP服务器
     Nginx作为静态文件HTTP服务器 在本地系统中创建/data/www目录，存放html文件(包括index.html) 创建/data/images目录，存放imags图片 在主配置文件中添加http指令   http { server { listen 80; server_name
  • kafka获得最新partition offset blackproof kafkapartitionoffset最新
    kafka获得partition下标，需要用到kafka的simpleconsumer   import java.util.ArrayList; import java.util.Collections; import java.util.Date; import java.util.HashMap; import java.util.List; import java.
  • centos 7安装docker两种方式 ronin47
          第一种是采用yum 方式              yum install -y docker           
  • java-60-在O(1)时间删除链表结点 bylijinnan java
    public class DeleteNode_O1_Time { /** * Q 60 在O(1)时间删除链表结点 * 给定链表的头指针和一个结点指针(!!)，在O(1)时间删除该结点 * * Assume the list is: * head->...->nodeToDelete->mNode->nNode->..
  • nginx利用proxy_cache来缓存文件 cfyme cache
    user  zhangy users; worker_processes 10; error_log  /var/vlogs/nginx_error.log  crit; pid        /var/vlogs/nginx.pid; #Specifies the value for ma
  • [JWFD开源工作流]JWFD嵌入式语法分析器负号的使用问题 comsci 嵌入式
        假如我们需要用JWFD的语法分析模块定义一个带负号的方程式，直接在方程式之前添加负号是不正确的，而必须这样做：     string str01 = "a=3.14;b=2.71;c=0;c-((a*a)+(b*b))"     定义一个0整数c,然后用这个整数c去
  • 如何集成支付宝官方文档 dai_lm android
    官方文档下载地址 https://b.alipay.com/order/productDetail.htm?productId=2012120700377310&tabId=4#ps-tabinfo-hash 集成的必要条件 1. 需要有自己的Server接收支付宝的消息 2. 需要先制作app，然后提交支付宝审核，通过后才能集成 调试的时候估计会真的扣款，请注意
  • 应该在什么时候使用Hadoop datamachine hadoop
    原帖地址：http://blog.chinaunix.net/uid-301743-id-3925358.html 存档，某些观点与我不谋而合，过度技术化不可取，且hadoop并非万能。 --------------------------------------------万能的分割线-------------------------------- 有人问我，“你在大数据和Hado
  • 在GridView中对于有外键的字段使用关联模型进行搜索和排序 dcj3sjt126com yii
    在GridView中使用关联模型进行搜索和排序 首先我们有两个模型它们直接有关联: class Author extends CActiveRecord { ... } class Post extends CActiveRecord { ... function relations() { return array( '
  • 使用NSString 的格式化大全 dcj3sjt126com Objective-C
    格式定义The format specifiers supported by the NSString formatting methods and CFString formatting functions follow the IEEE printf specification; the specifiers are summarized in Table 1. Note that you c
  • 使用activeX插件对象object滚动有重影 蕃薯耀 activeX插件滚动有重影
      使用activeX插件对象object滚动有重影   <object style="width:0;" id="abc" classid="CLSID:D3E3970F-2927-9680-BBB4-5D0889909DF6" codebase="activex/OAX339.CAB#
  • SpringMVC4零配置 hanqunfeng springmvc4
    基于Servlet3.0规范和SpringMVC4注解式配置方式，实现零xml配置，弄了个小demo，供交流讨论。     项目说明如下： 1.db.sql是项目中用到的表，数据库使用的是oracle11g 2.该项目使用mvn进行管理，私服为自搭建nexus,项目只用到一个第三方 jar，就是oracle的驱动； 3.默认项目为零配置启动，如果需要更改启动方式，请
  • 《开源框架那点事儿16》：缓存相关代码的演变 j2eetop 开源框架
    问题引入 上次我参与某个大型项目的优化工作，由于系统要求有比较高的TPS，因此就免不了要使用缓冲。 该项目中用的缓冲比较多，有MemCache，有Redis，有的还需要提供二级缓冲，也就是说应用服务器这层也可以设置一些缓冲。 当然去看相关实现代代码的时候，大致是下面的样子。 [java] view plain copy print ? public vo
  • AngularJS浅析 kvhur JavaScript
    概念 AngularJS is a structural framework for dynamic web apps. 了解更多详情请见原文链接：http://www.gbtags.com/gb/share/5726.htm Directive 扩展html，给html添加声明语句，以便实现自己的需求。对于页面中html元素以ng为前缀的属性名称，ng是angular的命名空间
  • 架构师之jdk的bug排查(一)---------------split的点号陷阱 nannan408 split
    1.前言.    jdk1.6的lang包的split方法是有bug的,它不能有效识别A.b.c这种类型,导致截取长度始终是0.而对于其他字符,则无此问题.不知道官方有没有修复这个bug. 2.代码 String[] paths = "object.object2.prop11".split("'"); System.ou
  • 如何对10亿数据量级的mongoDB作高效的全表扫描 quentinXXZ mongodb
      本文链接:  http://quentinXXZ.iteye.com/blog/2149440 一、正常情况下，不应该有这种需求 首先，大家应该有个概念，标题中的这个问题，在大多情况下是一个伪命题，不应该被提出来。要知道，对于一般较大数据量的数据库，全表查询，这种操作一般情况下是不应该出现的，在做正常查询的时候，如果是范围查询，你至少应该要加上limit。 说一下，
  • C语言算法之水仙花数 qiufeihu c算法
    /** * 水仙花数 */ #include <stdio.h> #define N 10 int main() { int x,y,z; for(x=1;x<=N;x++) for(y=0;y<=N;y++) for(z=0;z<=N;z++) if(x*100+y*10+z == x*x*x
  • JSP指令 wyzuomumu jsp
    jsp指令的一般语法格式： <%@ 指令名 属性 =”值 ” %> 常用的三种指令： page,include,taglib page指令语法形式： <%@ page 属性 1=”值 1” 属性 2=”值 2”%> include指令语法形式： <%@include file=”relative url”%> (jsp可以通过 include
按字母分类： ABCDEFGHIJKLMNOPQRSTUVWXYZ其他