求逆序对

Powered by:AB_IN 局外人

P1908 逆序对

归并排序
对于给定的一段正整数序列，逆序对就是序列中 ai>aj且i的有序对。
其实就是冒泡排序时，将数组升序排列时交换的次数。但$n\le 5\times 10^5$ ，$O(n^2)$肯定不行 。看题解 可以用树状数组做，但菜鸡还没学。
so,归并排序。

#include 
using namespace std;
typedef long long ll;
#pragma GCC optimize(2)
#pragma GCC optimize(3)
const ll maxn=1e6+10;
ll n,cnt,a[maxn],b[maxn];
char buf[1 << 21], *p1=buf, *p2=buf;
inline ll getc(){
    return p1 == p2 && (p2 = (p1 = buf) + fread(buf, 1, 1 << 21, stdin), p1 == p2) ? EOF : *p1++;
}
inline ll read() {
    ll ret = 0,f = 0;char ch = getc();
    while (!isdigit (ch)) {
        if (ch == '-') f = 1;
        ch = getc();
    }
    while (isdigit (ch)) {
        ret = ret * 10 + ch - 48;
        ch = getc();
    }
    return f ? -ret : ret;
}
inline void write(ll x) { if (!x) { putchar('0'); return; } char F[200]; ll tmp = x > 0 ? x : -x; if (x < 0)putchar('-'); int cnt = 0;    while (tmp > 0) { F[cnt++] = tmp % 10 + '0';     tmp /= 10; }    while (cnt > 0)putchar(F[--cnt]); }
void _merge(ll l,ll mid,ll r)
{
    ll p1=l,p2=mid+1;
    for(ll i=l;i<=r;i++){
        if((p1<=mid) && ((p2>r) || a[p1] <= a[p2])){
            b[i]=a[p1];
            p1++;
        }
        else{
            b[i]=a[p2];
            p2++;
            cnt+=mid-p1+1;//最关键的一句
        }
    }
    for(ll i=l;i<=r;i++) a[i]=b[i];
}
void erfen(ll l,ll r)
{
    ll mid=(l+r)/2;
    if(l<r){
        erfen(l,mid);
        erfen(mid+1,r);
    }
    _merge(l,mid,r);
}
int main()
{
    n=read();
    for(ll i=1;i<=n;i++)
        a[i]=read();
    erfen(1,n);
    write(cnt);putchar(10);
    return 0;
}

归并排序的原理要是知道了，那是什么时候像冒泡排序一样消除的逆序对呢？
对，就是这个时候。

else{
            b[i]=a[p2];
            p2++;
            cnt+=mid-p1+1;//最关键的一句
        }

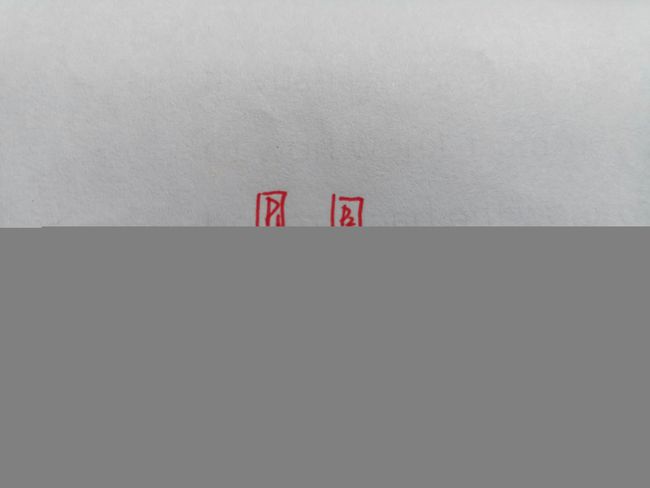
什么意思呢，看下面的图，就是让a数组右区间在p2的值提到了前面(左区间)，而红色涂满的区间(对应图2)都与p2指针指的数构成逆序对，故求区间长度就可以了。


树状数组
戳这！！（在 小鱼比可爱 那有说明）
跑的比归并还慢点，但是好写啊！！
就是：求在按顺序放的前提下，这个数此时在树状数组中右边有多少个数？（即比它大的数）
先离散化去重。

#include 
#define lowbit(x) ((x) & -(x))
#define rep(i,x,y) for (int i=(x);i<=(y);i++)
using namespace std;
const int maxn=5e5+10;
int n,a[maxn],t[maxn],tree[maxn];
void add(int x,int d)
{
    while(x<=n){
        tree[x]+=d;
        x+=lowbit(x);
    }
}
int sum(int x)
{
    int sum=0;
    while(x>0){
        sum+=tree[x];
        x-=lowbit(x);
    }
    return sum;
}

long long ans;
namespace IO{
    char ibuf[1<<21],*ip=ibuf,*ip_=ibuf;
    char obuf[1<<21],*op=obuf,*op_=obuf+(1<<21);
    inline char gc(){
        if(ip!=ip_)return *ip++;
        ip=ibuf;ip_=ip+fread(ibuf,1,1<<21,stdin);
        return ip==ip_?EOF:*ip++;
    }
    inline void pc(char c){
        if(op==op_)fwrite(obuf,1,1<<21,stdout),op=obuf;
        *op++=c;
    }
    inline int read(){
        register int x=0,ch=gc(),w=1;
        for(;ch<'0'||ch>'9';ch=gc())if(ch=='-')w=-1;
        for(;ch>='0'&&ch<='9';ch=gc())x=x*10+ch-48;
        return w*x;
    }
    template<class I>
    inline void write(I x){
        if(x<0)pc('-'),x=-x;
        if(x>9)write(x/10);pc(x%10+'0');
    }
    class flusher_{
    public:
        ~flusher_(){if(op!=obuf)fwrite(obuf,1,op-obuf,stdout);}
    }IO_flusher;
}
using namespace IO;

int main()
{
    n=read();
    rep(i,1,n){
        a[i]=read();
        t[i]=a[i];
    }
    sort(t+1,t+1+n);
    int m=unique(t+1,t+1+n)-t-1;
    rep(i,1,n){
        a[i]=lower_bound(t+1,t+1+m,a[i])-t;
    }
    rep(i,1,n){
        add(a[i],1);
        ans+=(i-sum(a[i]));
    }
    write(ans);
    pc('\n');
}

完结。