【动态规划-最大连续子序列和】HDU 1003 Max Sum 最大连续子序列和的左右区间

http://acm.hdu.edu.cn/showproblem.php?pid=1003

Max Sum

Time Limit: 2000/1000 MS (Java/Others)    Memory Limit: 65536/32768 K (Java/Others)
Total Submission(s): 294367    Accepted Submission(s): 69893

Problem Description

Given a sequence a[1],a[2],a[3]......a[n], your job is to calculate the max sum of a sub-sequence. For example, given (6,-1,5,4,-7), the max sum in this sequence is 6 + (-1) + 5 + 4 = 14.

Input

The first line of the input contains an integer T(1<=T<=20) which means the number of test cases. Then T lines follow, each line starts with a number N(1<=N<=100000), then N integers followed(all the integers are between -1000 and 1000).

Output

For each test case, you should output two lines. The first line is "Case #:", # means the number of the test case. The second line contains three integers, the Max Sum in the sequence, the start position of the sub-sequence, the end position of the sub-sequence. If there are more than one result, output the first one. Output a blank line between two cases.

Sample Input

2
5 6 -1 5 4 -7
7 0 6 -1 1 -6 7 -5

Sample Output

Case 1:
14 1 4

Case 2:
7 1 6

Author

Ignatius.L

Recommend

We have carefully selected several similar problems for you:  1176 1087 1069 1058 1159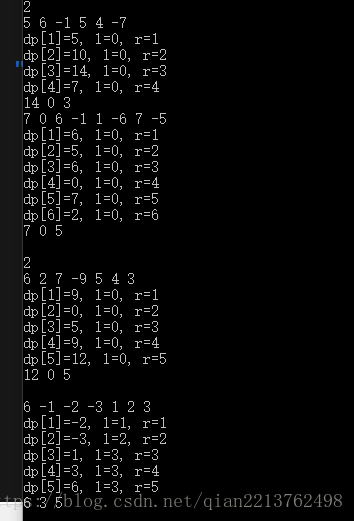 

AC Code

#include 
#include  
#include 
#include 
using namespace std; 
const int nmax=100000+10;
int a[nmax];
int dp[nmax];//必须以a[i]作为结尾的连续子序列的最大和 
int l[nmax];//必须以a[i]作为结尾的连续子序列的左端点 
int r[nmax];//必须以a[i]作为结尾的连续子序列的右端点 

int main(int argc, char** argv) {
    int t;
    while(cin>>t){
    	for(int k=1;k<=t;k++){//t组测试数据 
    		memset(a,0,sizeof(a));
    	    memset(dp,0,sizeof(dp));
    		memset(l,0,sizeof(l));
    		memset(r,0,sizeof(r));
    		int n;
    		cin>>n;
    		for(int i=1;i<=n;i++){
    			cin>>a[i];
    			dp[i]=a[i];
			}
			l[1]=1;r[1]=1; 
			for(int i=2;i<=n;i++){
				//dp[i]=max(dp[i],dp[i-1]+a[i]);
				if(dp[i-1]+a[i]>=dp[i]){
					dp[i]=dp[i-1]+a[i];
					l[i]=l[i-1];//左端点不变，右移右端点 
					r[i]=i;
				}
				else{
                    l[i]=i;//新起一个数 
					r[i]=i; 
				}
				//printf("dp[%d]=%d, l=%d, r=%d\n",i,dp[i],l[i],r[i]);
			}
			int ans=dp[1];
        	int pos=1;
        	for(int i=2;i<=n;i++){//<= 
            	if(dp[i]>ans){
                	ans=dp[i];
                	pos=i;
            	}
       		 }
			cout<<"Case "<