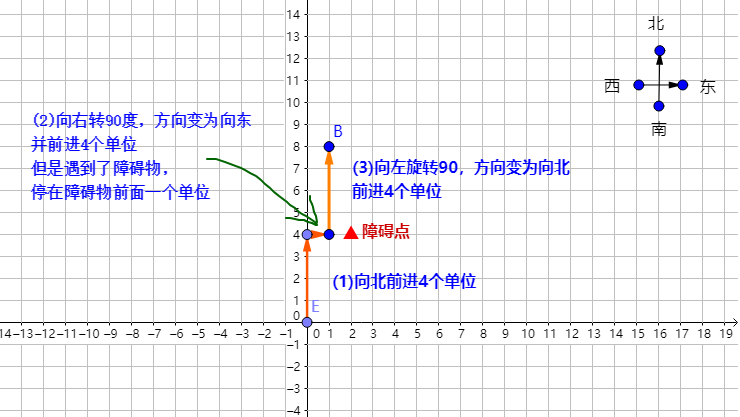
- LLM-Agent方法评估与效果分析
agent人工智能ai开发
1.引言近年来,随着大型语言模型(LLM)的快速发展,基于强化学习(RL)对LLM进行微调以使其具备代理(Agent)能力成为研究热点。从基础的单智能体强化学习算法(如PPO)到多智能体协作、语料重组以及在线自学习等新技术不断涌现,研究人员致力于探索如何提高LLM在实际应用中的决策能力、推理能力和任务执行效率。本文主要聚焦于当前LLM-Agent方法的检索与评估,旨在全面探讨各类方法的技术实现、实
- 初阶数据结构习题【16】(4栈和队列)——622. 设计循环队列
graceyun
##Leetcode数据结构算法
1.题目描述力扣在线OJ——622.设计循环队列设计你的循环队列实现。循环队列是一种线性数据结构,其操作表现基于FIFO(先进先出)原则并且队尾被连接在队首之后以形成一个循环。它也被称为“环形缓冲器”。循环队列的一个好处是我们可以利用这个队列之前用过的空间。在一个普通队列里,一旦一个队列满了,我们就不能插入下一个元素,即使在队列前面仍有空间。但是使用循环队列,我们能使用这些空间去存储新的值。你的实
- TreeNode底层实现原理
zhglhy
开发语言java
TreeNode是树结构的基本单元,通常用于表示树形数据结构中的节点。其底层实现原理涉及以下几个方面:1.TreeNode的基本结构在Java中,TreeNode通常是一个类,包含以下核心属性:数据域:存储节点的数据。子节点引用:指向子节点的引用(对于二叉树,通常是左子节点和右子节点)。父节点引用:指向父节点的引用(可选,取决于具体实现)。以下是一个典型的二叉树节点的实现:classTreeNod
- MongoDB
z小天才b
MongoDBmongodb数据库
一、MongoDB简介1.1什么是MongoDB?MongoDB是一个基于分布式文件存储的开源NoSQL数据库系统,由C++语言编写,旨在为Web应用提供可扩展的高性能数据存储解决方案。MongoDB将数据存储为一个文档,数据结构由键值对组成,类似于JSON对象,字段值可以包含其他文档、数组及文档数组。1.2MongoDB的核心特性文档型数据库:数据以BSON(BinaryJSON)格式存储灵活的
- 算法刷题记录——LeetCode篇(1) [第1~100题](持续更新)
Allen Wurlitzer
实战-算法解题算法leetcode职场和发展
更新时间:2025-03-21LeetCode刷题目录:算法刷题记录——专题目录汇总技术博客总目录:计算机技术系列博客——目录页优先整理热门100及面试150,不定期持续更新,欢迎关注!1.两数之和给定一个整数数组nums和一个整数目标值target,请你在该数组中找出和为目标值target的那两个整数,并返回它们的数组下标。你可以假设每种输入只会对应一个答案,并且你不能使用两次相同的元素。你可以
- LLM 大模型技术知识最佳学习路径图发布!
AGI-杠哥
学习人工智能语言模型agi自然语言处理
近日,经常有小伙伴私信我,大模型知识太多了,有点懵啊,我该如何学习LLM大模型?今天我们就来剖析下LLM大模型技术知识的学习路径。如果你是一个LLM大模型的“技术小白”,我们建议的学习路径如下:技术交流群前沿技术资讯、算法交流、求职内推、算法竞赛、面试交流(校招、社招、实习)等、与10000+来自港科大、北大、清华、中科院、CMU、腾讯、百度等名校名企开发者互动交流~我们建了大模型技术与面试交流群
- Stacking算法:集成学习的终极武器
civilpy
算法集成学习机器学习
Stacking算法:集成学习的终极武器在机器学习的竞技场中,集成学习方法以其卓越的性能而闻名。其中,Stacking(堆叠泛化)作为一种高级集成技术,更是被誉为“集成学习的终极武器”。本文将带你深入了解Stacking算法的原理和实现,并提供一些实战技巧和最佳实践。1.Stacking算法原理探秘Stacking算法的核心思想是训练多个不同的基模型,并将它们的预测结果作为新模型的输入特征,以此来
- 集成学习(上):Bagging集成方法
万事可爱^
机器学习修仙之旅#监督学习集成学习机器学习人工智能Bagging随机森林
一、什么是集成学习?在机器学习的世界里,没有哪个模型是完美无缺的。就像古希腊神话中的"盲人摸象",单个模型往往只能捕捉到数据特征的某个侧面。但当我们把多个模型的智慧集合起来,就能像拼图一样还原出完整的真相,接下来我们就来介绍一种“拼图”算法——集成学习。集成学习是一种机器学习技术,它通过组合多个模型(通常称为“弱学习器”或“基础模型”)的预测结果,构建出更强、更准确的学习算法。这种方法的主要思想是
- 直方图梯度提升:大数据时代的极速决策引擎
万事可爱^
大数据机器学习深度学习直方图梯度提升GBDT算法
一、为什么需要直方图梯度提升?在Kaggle竞赛的冠军解决方案中,超过70%的获奖方案都使用了梯度提升算法。但当数据量突破百万级时,传统梯度提升树(GBDT)面临三大致命瓶颈:训练耗时剧增:每个特征的分割点计算都需要全量数据排序内存消耗爆炸:存储排序后的特征值需要额外空间处理效率低下:无法有效利用现代CPU的多核特性而梯度提升决策树(GBDT)作为集成学习的代表算法,通过迭代构建决策树实现预测能力
- 【集成学习】:Stacking原理以及Python代码实现
Geeksongs
机器学习python机器学习深度学习人工智能算法
Stacking集成学习在各类机器学习竞赛当中得到了广泛的应用,尤其是在结构化的机器学习竞赛当中表现非常好。今天我们就来介绍下stacking这个在机器学习模型融合当中的大杀器的原理。并在博文的后面附有相关代码实现。总体来说,stacking集成算法主要是一种基于“标签”的学习,有以下的特点:用法:模型利用交叉验证,对训练集进行预测,从而实现二次学习优点:可以结合不同的模型缺点:增加了时间开销,容
- Redis 哨兵模式的选举算法是什么?
少林码僧
redissentinel
Redis哨兵模式中的选举算法主要用于在主节点出现故障时,从多个Sentinel节点中选出一个领导者(Leader)来执行故障转移操作。Redis哨兵的选举算法基于Raft算法的简化版本,但不完全等同于标准的Raft算法。以下是其主要过程:一、发现主节点故障当一个Sentinel节点主观地认为主节点不可达时(通常是在一定时间内没有收到主节点的PING回复),它会将主节点标记为主观下线(Subjec
- 初级:数组与字符串面试题深度剖析
佩奇的技术笔记
Java面试小册java
一、引言在Java开发中,数组和字符串是最常用的数据结构之一。面试官通过相关问题考察候选人对数组和字符串的理解和运用能力,以及在实际开发中解决相关问题的经验。本文将深入剖析常见的数组与字符串面试题,结合实际开发场景,帮助读者全面掌握这些知识点。二、数组面试题:如何对数组进行初始化和遍历?答案:数组的初始化可以使用直接初始化、动态初始化等方式。遍历数组可以使用传统的for循环、增强型for循环(fo
- Kafka 的消息压缩机制:优化存储与传输的利器
阿贾克斯的黎明
javalinqc#java
目录Kafka的消息压缩机制:优化存储与传输的利器一、消息压缩机制的重要意义1.减少存储成本2.提升网络传输效率二、Kafka常用的消息压缩算法1.GZIP压缩2.Snappy压缩3.前端展示压缩状态(Vue3+TS)在消息中间件的大家族中,Kafka以其卓越的性能而备受瞩目。其中,Kafka的消息压缩机制是一项非常重要的特性,它就像是一个高效的“压缩包”,在不损失数据内容的前提下,有效减少数据的
- 关于AI OS那点事
大囚长
科普天地大模型人工智能
AIOS(人工智能操作系统)作为面向智能时代的操作系统,其功能定位和架构设计与传统操作系统(如Linux、Windows、iOS等)存在显著差异。一、AIOS需具备的核心功能智能体全生命周期管理智能体调度与并发:需支持多智能体任务的优先级排序、资源分配及并发执行,例如通过轮询调度或动态优先级算法优化LLM资源利用率。上下文感知与切换:通过上下文管理器实现智能体交互状态的快照保存与恢复,解决LLM生
- 贪心算法之分发饼干
努 力 小 子
#刷题(简单难度)#贪心算法
假设你是一位很棒的家长,想要给你的孩子们一些小饼干。但是,每个孩子最多只能给一块饼干。对每个孩子i,都有一个胃口值gi,这是能让孩子们满足胃口的饼干的最小尺寸;并且每块饼干j,都有一个尺寸sj。如果sj>=gi,我们可以将这个饼干j分配给孩子i,这个孩子会得到满足。你的目标是尽可能满足越多数量的孩子,并输出这个最大数值。注意:你可以假设胃口值为正。一个小朋友最多只能拥有一块饼干。示例1:输入:[1
- JAVA刷Leecode-贪心算法-分配问题-分发饼干
搬砖的水鱼
leetcode算法javapythonleetcode贪心算法
JAVA刷Leecode-贪心算法算法思想分配问题-分发饼干(135,hard)算法思想采用贪心的策略,保证每次操作都是局部最优解,从而最终的结果是全局最优。贪心算法不是对所有问题都能得到整体最优解,选择的贪心策略必须具有无后效性,即某个状态以前的过程不会影响以后的状态,只和当前的状态相关。包括分配问题(455,135)和区间问题(435);练习:605,452,763,122,406。分配问题-
- 【贪心算法】1、分发饼干
念奕玥
【Java】数据结构与算法javaleetcode贪心算法
贪心算法或贪心思想采用贪心的策略,保证每次操作都是局部最优的,从而使最后得到的结果是全局最优的。可用于解决分配问题e.g.leetcode455分发饼干解题思路:目标:尽可能满足越多数量的孩子。根据目标,可以容易想到,先去满足胃口值小的孩子。为了尽量使饼干可以满足更多的孩子,所以要把饼干尺寸大于等于孩子胃口值的饼干中挑尺寸最小的饼干给孩子。满足了这个孩子之后,再采取同样的策略去考虑剩下的孩子,直到
- 流浪地球 - 华为OD机试真题(E卷、C++)
什码情况
华为odc++算法数据结构面试机试
针对刷题难,效率慢,我们提供一对一算法辅导,针对个人情况定制化的提高计划(全称1V1效率更高)。有兴趣的同学可以扫码添加我们的微信(code5bug)了解,免费试课一下。题目描述流浪地球计划在赤道上均匀部署了N个转向发动机,按位置顺序编号为0~N。1).初始状态下所有的发动机都是未启动状态;2).发动机启动的方式分为”手动启动”和”关联启动”两种方式;3).如果在时刻1一个发动机被启动,下一个时刻
- Yolo系列之Yolo的基本理解
是十一月末
YOLOpython开发语言yolo
YOLO的基本理解目录YOLO的基本理解1YOLO1.1概念1.2算法2单、多阶段对比2.1FLOPs和FPS2.2one-stage单阶段2.3two-stage两阶段1YOLO1.1概念YOLO(YouOnlyLookOnce)是一种基于深度学习的目标检测算法,由JosephRedmon等人于2016年提出。它的核心思想是将目标检测问题转化为一个回归问题,通过一个神经网络直接预测目标的类别和位
- 数据结构双向链表的创建与初始化
拉梅洛.
数据结构链表
#include#include#include//定义节点类型typedefintdata_t;typedefstructnode{data_tdata;//以整型数据为例structnode*prev;//指向structnode点的指针structnode*next;//指向structnode点的指针}node_t;intdlist_create(node_t**,data_t);//函数
- 贪心算法-455分发饼干
工大一只猿
贪心算法算法
classSolution{public:intfindContentChildren(vector&g,vector&s){sort(g.begin(),g.end());sort(s.begin(),s.end());intcount=0;inti=g.size()-1;intj=s.size()-1;for(i;i>=0;i--){if(j>=0&&s[j]>=g[i]){j--;count
- 455. 分发饼干(贪心算法)
穿过漫长林径
LeetCode
455.分发饼干题目描述:有一群孩子和一堆饼干,每个孩子有一个饥饿度,每个饼干都有一个大小。每个孩子只能吃一个饼干,且只有饼干的大小不小于孩子的饥饿度时,这个孩子才能吃饱。求解最多有多少孩子可以吃饱。示例1:输入:g=[1,2,3],s=[1,1]输出:1解释:你有三个孩子和两块小饼干,3个孩子的胃口值分别是:1,2,3。虽然你有两块小饼干,由于他们的尺寸都是1,你只能让胃口值是1的孩子满足。所以
- 贪心算法:分发饼干
AlphaFinance
求职面试
假设你是一位很棒的家长,想要给你的孩子们一些小饼干。但是,每个孩子最多只能给一块饼干。对每个孩子i,都有一个胃口值g[i],这是能让孩子们满足胃口的饼干的最小尺寸;并且每块饼干j,都有一个尺寸s[j]。如果s[j]>=g[i],我们可以将这个饼干j分配给孩子i,这个孩子会得到满足。你的目标是尽可能满足越多数量的孩子,并输出这个最大数值。示例1:输入:g=[1,2,3],s=[1,1]输出:1解释:
- 2021-11-12 455. 分发饼干(贪心算法)
TABE_
贪心算法leetcode算法
注:题目:假设你是一位很棒的家长,想要给你的孩子们一些小饼干。但是,每个孩子最多只能给一块饼干。对每个孩子i,都有一个胃口值g[i],这是能让孩子们满足胃口的饼干的最小尺寸;并且每块饼干j,都有一个尺寸s[j]。如果s[j]>=g[i],我们可以将这个饼干j分配给孩子i,这个孩子会得到满足。你的目标是尽可能满足越多数量的孩子,并输出这个最大数值。示例1:输入:g=[1,2,3],s=[1,1]输出
- 贪心算法(9)(java)最优除法
奋进的小暄
java贪心算法算法
题目:给定一正整数数组nums,nums中的相邻整数将进行浮点除法。例如,[2,3.4]->2/3/4.例如,nums=[2,3,4],我们将求表达式的值“2/3/4"。但是,你可以在任意位置添加任意数目的括号,来改变算数的优先级。你需要找出怎么添加括号,以便计算后的表达式的值为最大值。以字符串格式返回具有最大值的对应表达式。注意:你的表达式不应该包含多余的括号。输入:【1000,100,10,2
- 机器学习中的贝叶斯网络:如何构建高效的风险预测模型
AI天才研究院
DeepSeekR1&大数据AI人工智能大模型自然语言处理人工智能语言模型编程实践开发语言架构设计
作者:禅与计算机程序设计艺术文章目录机器学习中的贝叶斯网络:如何构建高效的风险预测模型1.背景介绍2.基本概念术语说明2.1马尔科夫随机场(MarkovRandomField)2.2条件随机场(ConditionalRandomField,CRF)2.3变量elimination算法2.4贝叶斯网络3.核心算法原理和具体操作步骤以及数学公式讲解3.1原理介绍1.贝叶斯网络基础2.贝叶斯网络构建风险
- 优化 Java 数据结构选择与使用,提升程序性能与可维护性
A-Kamen
java数据结构开发语言
引言在软件开发中,数据结构的选择是影响程序性能、内存使用以及代码可维护性的关键因素之一。Java作为一门广泛使用的编程语言,提供了丰富的内置数据结构,如数组、链表、栈、队列、树、图以及集合框架中的各种接口实现(如List,Set,Map等)。然而,面对不同的应用场景,如何合理地选择和优化数据结构,成为了一个值得深入探讨的话题。本文将介绍几种常见的Java数据结构,并探讨如何根据实际需求进行优化选择
- 机器臂运动控制算法工程师面试
道亦无名
面试算法人工智能机器学习
大厂的经验总结:一、基础概念理解请解释机器臂运动学正解和逆解的概念,并分别说明其用途。正解:已知机器臂各关节的角度(或位移),通过运动学模型计算出机器臂末端执行器在笛卡尔空间中的位置和姿态。用途在于可以根据给定的关节驱动值,预测末端的实际位置,用于运动仿真、路径验证等,比如在工业生产前模拟机器臂的动作是否能准确到达加工位置。逆解:已知机器臂末端执行器在笛卡尔空间中的期望位置和姿态,求解出各关节应处
- Java高并发容器的内核解析:从无锁算法到分段锁的架构演进
猿享天开
开发语言java
《Java高并发容器的内核解析:从无锁算法到分段锁的架构演进》本文将以JUC包核心容器为切入点,深入剖析ConcurrentHashMap在Java8中的64位Hash分段技术,解密LinkedBlockingQueue双锁队列设计的吞吐量秘密,并给出各容器在亿级流量场景下的性能压测对比与选型决策矩阵。一、BlockingQueue体系:生产者-消费者模式的工业级实现1.阻塞队列的四大行为矩阵行为
- 【北上广深杭大厂AI算法面试题】计算机视觉篇...如何解决多尺度问题?
努力毕业的小土博^_^
AI算法题库人工智能算法计算机视觉深度学习神经网络
【北上广深杭大厂AI算法面试题】计算机视觉篇…如何解决多尺度问题?【北上广深杭大厂AI算法面试题】计算机视觉篇…如何解决多尺度问题?文章目录【北上广深杭大厂AI算法面试题】计算机视觉篇...如何解决多尺度问题?前言数据级别的多尺度模型架构上的多尺度表示FPN代码示例(PyTorch)说明其他多尺度处理方法总结欢迎铁子们点赞、关注、收藏!祝大家逢考必过!逢投必中!上岸上岸上岸!upupup大多数高校
- 插入表主键冲突做更新
a-john
有以下场景:
用户下了一个订单,订单内的内容较多,且来自多表,首次下单的时候,内容可能会不全(部分内容不是必须,出现有些表根本就没有没有该订单的值)。在以后更改订单时,有些内容会更改,有些内容会新增。
问题:
如果在sql语句中执行update操作,在没有数据的表中会出错。如果在逻辑代码中先做查询,查询结果有做更新,没有做插入,这样会将代码复杂化。
解决:
mysql中提供了一个sql语
- Android xml资源文件中@、@android:type、@*、?、@+含义和区别
Cb123456
@+@?@*
一.@代表引用资源
1.引用自定义资源。格式:@[package:]type/name
android:text="@string/hello"
2.引用系统资源。格式:@android:type/name
android:textColor="@android:color/opaque_red"
- 数据结构的基本介绍
天子之骄
数据结构散列表树、图线性结构价格标签
数据结构的基本介绍
数据结构就是数据的组织形式,用一种提前设计好的框架去存取数据,以便更方便,高效的对数据进行增删查改。正确选择合适的数据结构,对软件程序的高效执行的影响作用不亚于算法的设计。此外,在计算机系统中数据结构的作用也是非同小可。例如常常在编程语言中听到的栈,堆等,就是经典的数据结构。
经典的数据结构大致如下:
一:线性数据结构
(1):列表
a
- 通过二维码开放平台的API快速生成二维码
一炮送你回车库
api
现在很多网站都有通过扫二维码用手机连接的功能,联图网(http://www.liantu.com/pingtai/)的二维码开放平台开放了一个生成二维码图片的Api,挺方便使用的。闲着无聊,写了个前台快速生成二维码的方法。
html代码如下:(二维码将生成在这div下)
? 1
&nbs
- ImageIO读取一张图片改变大小
3213213333332132
javaIOimageBufferedImage
package com.demo;
import java.awt.image.BufferedImage;
import java.io.File;
import java.io.IOException;
import javax.imageio.ImageIO;
/**
* @Description 读取一张图片改变大小
* @author FuJianyon
- myeclipse集成svn(一针见血)
7454103
eclipseSVNMyEclipse
&n
- 装箱与拆箱----autoboxing和unboxing
darkranger
J2SE
4.2 自动装箱和拆箱
基本数据(Primitive)类型的自动装箱(autoboxing)、拆箱(unboxing)是自J2SE 5.0开始提供的功能。虽然为您打包基本数据类型提供了方便,但提供方便的同时表示隐藏了细节,建议在能够区分基本数据类型与对象的差别时再使用。
4.2.1 autoboxing和unboxing
在Java中,所有要处理的东西几乎都是对象(Object)
- ajax传统的方式制作ajax
aijuans
Ajax
//这是前台的代码
<%@ page language="java" import="java.util.*" pageEncoding="UTF-8"%> <% String path = request.getContextPath(); String basePath = request.getScheme()+
- 只用jre的eclipse是怎么编译java源文件的?
avords
javaeclipsejdktomcat
eclipse只需要jre就可以运行开发java程序了,也能自动 编译java源代码,但是jre不是java的运行环境么,难道jre中也带有编译工具? 还是eclipse自己实现的?谁能给解释一下呢问题补充:假设系统中没有安装jdk or jre,只在eclipse的目录中有一个jre,那么eclipse会采用该jre,问题是eclipse照样可以编译java源文件,为什么呢?
&nb
- 前端模块化
bee1314
模块化
背景: 前端JavaScript模块化,其实已经不是什么新鲜事了。但是很多的项目还没有真正的使用起来,还处于刀耕火种的野蛮生长阶段。 JavaScript一直缺乏有效的包管理机制,造成了大量的全局变量,大量的方法冲突。我们多么渴望有天能像Java(import),Python (import),Ruby(require)那样写代码。在没有包管理机制的年代,我们是怎么避免所
- 处理百万级以上的数据处理
bijian1013
oraclesql数据库大数据查询
一.处理百万级以上的数据提高查询速度的方法: 1.应尽量避免在 where 子句中使用!=或<>操作符,否则将引擎放弃使用索引而进行全表扫描。
2.对查询进行优化,应尽量避免全表扫描,首先应考虑在 where 及 o
- mac 卸载 java 1.7 或更高版本
征客丶
javaOS
卸载 java 1.7 或更高
sudo rm -rf /Library/Internet\ Plug-Ins/JavaAppletPlugin.plugin
成功执行此命令后,还可以执行 java 与 javac 命令
sudo rm -rf /Library/PreferencePanes/JavaControlPanel.prefPane
成功执行此命令后,还可以执行 java
- 【Spark六十一】Spark Streaming结合Flume、Kafka进行日志分析
bit1129
Stream
第一步,Flume和Kakfa对接,Flume抓取日志,写到Kafka中
第二部,Spark Streaming读取Kafka中的数据,进行实时分析
本文首先使用Kakfa自带的消息处理(脚本)来获取消息,走通Flume和Kafka的对接 1. Flume配置
1. 下载Flume和Kafka集成的插件,下载地址:https://github.com/beyondj2ee/f
- Erlang vs TNSDL
bookjovi
erlang
TNSDL是Nokia内部用于开发电信交换软件的私有语言,是在SDL语言的基础上加以修改而成,TNSDL需翻译成C语言得以编译执行,TNSDL语言中实现了异步并行的特点,当然要完整实现异步并行还需要运行时动态库的支持,异步并行类似于Erlang的process(轻量级进程),TNSDL中则称之为hand,Erlang是基于vm(beam)开发,
- 非常希望有一个预防疲劳的java软件, 预防过劳死和眼睛疲劳,大家一起努力搞一个
ljy325
企业应用
非常希望有一个预防疲劳的java软件,我看新闻和网站,国防科技大学的科学家累死了,太疲劳,老是加班,不休息,经常吃药,吃药根本就没用,根本原因是疲劳过度。我以前做java,那会公司垃圾,老想赶快学习到东西跳槽离开,搞得超负荷,不明理。深圳做软件开发经常累死人,总有不明理的人,有个软件提醒限制很好,可以挽救很多人的生命。
相关新闻:
(1)IT行业成五大疾病重灾区:过劳死平均37.9岁
- 读《研磨设计模式》-代码笔记-原型模式
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
/**
* Effective Java 建议使用copy constructor or copy factory来代替clone()方法:
* 1.public Product copy(Product p){}
* 2.publi
- 配置管理---svn工具之权限配置
chenyu19891124
SVN
今天花了大半天的功夫,终于弄懂svn权限配置。下面是今天收获的战绩。
安装完svn后就是在svn中建立版本库,比如我本地的是版本库路径是C:\Repositories\pepos。pepos是我的版本库。在pepos的目录结构
pepos
component
webapps
在conf里面的auth里赋予的权限配置为
[groups]
- 浅谈程序员的数学修养
comsci
设计模式编程算法面试招聘
浅谈程序员的数学修养
- 批量执行 bulk collect与forall用法
daizj
oraclesqlbulk collectforall
BULK COLLECT 子句会批量检索结果,即一次性将结果集绑定到一个集合变量中,并从SQL引擎发送到PL/SQL引擎。通常可以在SELECT INTO、
FETCH INTO以及RETURNING INTO子句中使用BULK COLLECT。本文将逐一描述BULK COLLECT在这几种情形下的用法。
有关FORALL语句的用法请参考:批量SQL之 F
- Linux下使用rsync最快速删除海量文件的方法
dongwei_6688
OS
1、先安装rsync:yum install rsync
2、建立一个空的文件夹:mkdir /tmp/test
3、用rsync删除目标目录:rsync --delete-before -a -H -v --progress --stats /tmp/test/ log/这样我们要删除的log目录就会被清空了,删除的速度会非常快。rsync实际上用的是替换原理,处理数十万个文件也是秒删。
- Yii CModel中rules验证规格
dcj3sjt126com
rulesyiivalidate
Yii cValidator主要用法分析:
yii验证rulesit 分类: Yii yii的rules验证 cValidator主要属性 attributes ,builtInValidators,enableClientValidation,message,on,safe,skipOnError
- 基于vagrant的redis主从实验
dcj3sjt126com
vagrant
平台: Mac
工具: Vagrant
系统: Centos6.5
实验目的: Redis主从
实现思路
制作一个基于sentos6.5, 已经安装好reids的box, 添加一个脚本配置从机, 然后作为后面主机从机的基础box
制作sentos6.5+redis的box
mkdir vagrant_redis
cd vagrant_
- Memcached(二)、Centos安装Memcached服务器
frank1234
centosmemcached
一、安装gcc
rpm和yum安装memcached服务器连接没有找到,所以我使用的是make的方式安装,由于make依赖于gcc,所以要先安装gcc
开始安装,命令如下,[color=red][b]顺序一定不能出错[/b][/color]:
建议可以先切换到root用户,不然可能会遇到权限问题:su root 输入密码......
rpm -ivh kernel-head
- Remove Duplicates from Sorted List
hcx2013
remove
Given a sorted linked list, delete all duplicates such that each element appear only once.
For example,Given 1->1->2, return 1->2.Given 1->1->2->3->3, return&
- Spring4新特性——JSR310日期时间API的支持
jinnianshilongnian
spring4
Spring4新特性——泛型限定式依赖注入
Spring4新特性——核心容器的其他改进
Spring4新特性——Web开发的增强
Spring4新特性——集成Bean Validation 1.1(JSR-349)到SpringMVC
Spring4新特性——Groovy Bean定义DSL
Spring4新特性——更好的Java泛型操作API
Spring4新
- 浅谈enum与单例设计模式
247687009
java单例
在JDK1.5之前的单例实现方式有两种(懒汉式和饿汉式并无设计上的区别故看做一种),两者同是私有构
造器,导出静态成员变量,以便调用者访问。
第一种
package singleton;
public class Singleton {
//导出全局成员
public final static Singleton INSTANCE = new S
- 使用switch条件语句需要注意的几点
openwrt
cbreakswitch
1. 当满足条件的case中没有break,程序将依次执行其后的每种条件(包括default)直到遇到break跳出
int main()
{
int n = 1;
switch(n) {
case 1:
printf("--1--\n");
default:
printf("defa
- 配置Spring Mybatis JUnit测试环境的应用上下文
schnell18
springmybatisJUnit
Spring-test模块中的应用上下文和web及spring boot的有很大差异。主要试下来差异有:
单元测试的app context不支持从外部properties文件注入属性
@Value注解不能解析带通配符的路径字符串
解决第一个问题可以配置一个PropertyPlaceholderConfigurer的bean。
第二个问题的具体实例是:
- Java 定时任务总结一
tuoni
javaspringtimerquartztimertask
Java定时任务总结 一.从技术上分类大概分为以下三种方式: 1.Java自带的java.util.Timer类,这个类允许你调度一个java.util.TimerTask任务; 说明: java.util.Timer定时器,实际上是个线程,定时执行TimerTask类 &
- 一种防止用户生成内容站点出现商业广告以及非法有害等垃圾信息的方法
yangshangchuan
rank相似度计算文本相似度词袋模型余弦相似度
本文描述了一种在ITEYE博客频道上面出现的新型的商业广告形式及其应对方法,对于其他的用户生成内容站点类型也具有同样的适用性。
最近在ITEYE博客频道上面出现了一种新型的商业广告形式,方法如下:
1、注册多个账号(一般10个以上)。
2、从多个账号中选择一个账号,发表1-2篇博文