数学杂谈:圆上随机落点问题(一)
- 数学杂谈:圆上随机落点问题(一)
- 1. 问题描述
- 2. 问题解答
- 1. 解法一:递推
- 2. 解法二:受限制的均匀分布
- 3. 数值模拟验证
- 3. 讨论 & 扩展
1. 问题描述
这道题其实很早之前自己做过一遍,然后前阵子发现苏神也关于这道题进行了一些讨论,于是就把这道题重新拎出来做了一遍,这里也稍微整理一下这道题的解答。
问题:
- 在一个圆上随机抛 n n n个点,求问这 n n n个点均在同一个半圆上的概率是多少?
更进一步地,这道题还可以拓展为:
- 在一个圆上随机抛 n n n个点,求问这 n n n个点均可以被一个弧度为 θ \theta θ( θ ≤ π \theta \leq \pi θ≤π)的弧形完全覆盖的概率是多少?
2. 问题解答
我们首先直接给出答案如下:
- n n n个点恰好落在弧度为 θ \theta θ的弧形中的概率密度函数为:
p n ( θ ) = n ( n − 1 ) 2 π ( θ 2 π ) n − 2 p_n(\theta) = \frac{n(n-1)}{2\pi}(\frac{\theta}{2\pi})^{n-2} pn(θ)=2πn(n−1)(2πθ)n−2
- n n n个点能够被弧度为 θ \theta θ的弧形完全覆盖的概率为:
P n ( θ ) = ∫ 0 θ p n ( φ ) d φ = n ( θ 2 π ) n − 1 P_n(\theta) = \int_{0}^{\theta} p_n(\varphi)d\varphi = n(\frac{\theta}{2\pi})^{n-1} Pn(θ)=∫0θpn(φ)dφ=n(2πθ)n−1
1. 解法一:递推
这一题我的一个直接的思路就是递推,显然,我们有:
p 2 ( θ ) = 1 π p_2(\theta) = \frac{1}{\pi} p2(θ)=π1
要使得 n n n个点恰好构成一个弧度为 θ \theta θ的弧形,可以由以下两种情况构成:
- 前 n − 1 n-1 n−1个点构成一个弧度为 φ \varphi φ的弧形,且有 φ ≤ θ \varphi \leq \theta φ≤θ,此时第 n n n个点需要与前 n − 1 n-1 n−1个点所构成的弧形的左边界或者右边界恰好构成 θ \theta θ角,且这个 θ \theta θ的弧形包含之前全部的 n − 1 n-1 n−1个点;
- 前 n − 1 n-1 n−1个点已经构成一个弧度恰好为 θ \theta θ的弧形,此时第 n n n个点只要落在弧形当中就可以。
因此,我们可以给出递推公式如下:
p n ( θ ) = 1 2 π [ 2 ⋅ ∫ 0 θ p n − 1 ( φ ) d φ + ∫ 0 θ d φ ⋅ p n − 1 ( θ ) ] p_n(\theta) = \frac{1}{2\pi} [2 \cdot \int_{0}^{\theta} p_{n-1}(\varphi) d\varphi + \int_{0}^{\theta}d\varphi \cdot p_{n-1}(\theta)] pn(θ)=2π1[2⋅∫0θpn−1(φ)dφ+∫0θdφ⋅pn−1(θ)]
综上,我们即可由数学归纳法解出 f n ( θ ) f_n(\theta) fn(θ)的通解表达式如下:
p n ( θ ) = n ( n − 1 ) 2 π ( θ 2 π ) n − 2 p_n(\theta) = \frac{n(n-1)}{2\pi}(\frac{\theta}{2\pi})^{n-2} pn(θ)=2πn(n−1)(2πθ)n−2
积分即可得到:
P n ( θ ) = ∫ 0 θ p n ( φ ) d φ = n ( θ 2 π ) n − 1 P_n(\theta) = \int_{0}^{\theta} p_n(\varphi)d\varphi = n(\frac{\theta}{2\pi})^{n-1} Pn(θ)=∫0θpn(φ)dφ=n(2πθ)n−1
2. 解法二:受限制的均匀分布
除了递推解法之外,事实上我们也可以将其视为一个受限制的均匀分布的概率求解问题。
我们以任意一个点作为起始点,然后顺时针旋转,显然圆上的 n n n个点就会将整个圆切分为 n n n个圆弧,然后我们要令这 n n n个点可以恰好被一个弧度为 θ \theta θ的弧形覆盖,就是令这 n n n个圆弧中最大的一个值恰好等于 2 π − θ 2\pi-\theta 2π−θ。
因此,问题也就可以转换为:
p n ( θ ) = C n 1 ⋅ p ( θ i = 2 π − θ ∣ ∑ i = 1 n θ i = 2 π ) = C n 1 ⋅ p ( x i = 1 − θ 2 π ∣ ∑ i = 1 n x i = 1 ) \begin{aligned} p_n(\theta) &= C_{n}^{1} \cdot p(\theta_i = 2\pi - \theta | \sum\limits_{i=1}^{n} \theta_i = 2\pi) \\ &= C_{n}^{1} \cdot p(x_i = 1 - \frac{\theta}{2\pi} | \sum\limits_{i=1}^{n} x_i = 1) \end{aligned} pn(θ)=Cn1⋅p(θi=2π−θ∣i=1∑nθi=2π)=Cn1⋅p(xi=1−2πθ∣i=1∑nxi=1)
而关于这个受限制的概率问题,我们在之前的博客文章【数学杂谈:限制条件下的均匀分布考察】当中,事实上已经对这个问题有过了考察,我们直接给出答案如下:
p ( x i = 1 − θ 2 π ∣ ∑ i = 1 n x i = 1 ) = ( n − 1 ) ( θ 2 π ) n − 2 p(x_i = 1 - \frac{\theta}{2\pi} | \sum\limits_{i=1}^{n} x_i = 1) = (n-1) (\frac{\theta}{2\pi})^{n-2} p(xi=1−2πθ∣i=1∑nxi=1)=(n−1)(2πθ)n−2
又因为 x = θ 2 π x = \frac{\theta}{2\pi} x=2πθ,因此,我们将其作变量代换之后即有:
p n ( θ ) = n ( n − 1 ) 2 π ( θ 2 π ) n − 2 p_n(\theta) = \frac{n(n-1)}{2\pi}(\frac{\theta}{2\pi})^{n-2} pn(θ)=2πn(n−1)(2πθ)n−2
而积分即可计算得到:
P n ( θ ) = ∫ 0 θ p n ( φ ) d φ = n ( θ 2 π ) n − 1 P_n(\theta) = \int_{0}^{\theta} p_n(\varphi)d\varphi = n(\frac{\theta}{2\pi})^{n-1} Pn(θ)=∫0θpn(φ)dφ=n(2πθ)n−1
与上述递推解法得到的结果是完全一致的。
3. 数值模拟验证
最后,我们用monte-carlo模拟来验证一下我们上述结论的可靠性。
给出monte-carlo模拟的代码实现如下:
import math
from random import random
from matplotlib import pyplot as plt
def simulate(theta, n, N=100000):
def get_radian(plist):
plist = sorted(plist)
deltas = [plist[(i+1) % n] - plist[i] + 2 * math.pi for i in range(n)]
deltas = [x if x < 2 * math.pi else x - 2 * math.pi for x in deltas]
return math.pi * 2 - max(deltas)
cnt = 0
for _ in range(N):
plist = [random() * 2 * math.pi for _ in range(n)]
radian = get_radian(plist)
if radian <= theta:
cnt += 1
return cnt / N
def plot_simulate(n, delta=10, max_degree=180, N=10000):
degrees = list(range(0, max_degree, delta))
degrees.append(max_degree)
x = [deg / 180 * math.pi for deg in degrees]
y = [simulate(phi, n) for phi in x]
z = [n * (deg/360)**(n-1) for deg in degrees]
plt.figure(figsize=(15, 7))
plt.scatter(degrees, y, marker="x", label="monte-carlo")
plt.plot(degrees, z, color="orange", label=r"$P_n ( \theta )$")
plt.legend()
plt.grid()
plt.show()
return
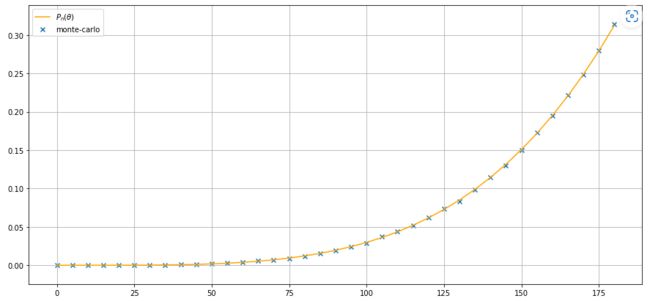
plot_simulate(5, delta=5, max_degree=180, N=10000)
可以看到:
- 当 θ ≤ 180 ° \theta \leq 180\degree θ≤180°时,曲线与拟合结果符合的非常完美。
3. 讨论 & 扩展
这里,其实大部分读者也都注意到了,这里我们反复地在强调, θ ≤ 180 ° \theta \leq 180\degree θ≤180°。
但是,对于圆上的随机 n n n个点,事实上要能够覆盖住这 n n n个点,所需的最小弧形的弧度是可以大于 180 ° 180\degree 180°的。更确切地,简单计算可以发现,这个最小弧度的最大值可以一直取到 θ = n − 1 n π \theta = \frac{n-1}{n}\pi θ=nn−1π。
那么,对于 θ ≥ 180 ° \theta \geq 180\degree θ≥180°的情况时,上述推论是否还成立呢?
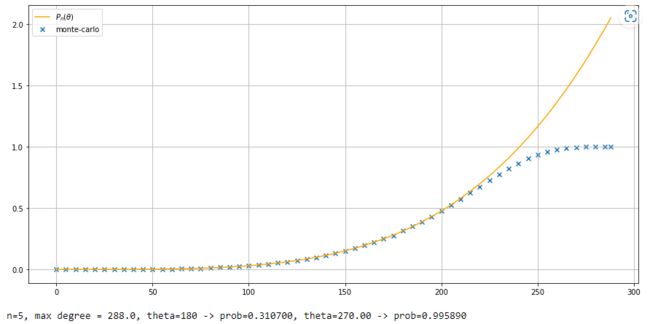
答案是不成立,我们直接给出上述曲线拟合结果即可:
可以看到:
- 显然,当弧度超过 180 ° 180\degree 180°时,上述拟合公式就不再成立,且两者差距会越来越大。
我们分别说明一下上述两种方法为何在 180 ° 180\degree 180°以上的情况下会失效:
- 递推方法
- 递推方法的第一个部分存在一个隐藏条件,就是当前 n − 1 n-1 n−1个点所构成的角度为 ϕ \phi ϕ时,新加入的第 n n n个点所构成的新的弧形所需要覆盖的最小角度为 θ \theta θ,但是这个假设在 θ ≥ 180 ° \theta \geq 180\degree θ≥180°时不一定成立的,因为有可能另一侧覆盖的角度反而小于 θ \theta θ。
- 受限均匀分布方法
- 同样的,首先的均匀分布其实要求的条件是,存在某一个角度不小于 2 π − θ 2\pi-\theta 2π−θ,这个条件在 θ ≤ 180 ° \theta \leq 180\degree θ≤180°的情况下是唯一的,因为至多只能有一个角度大于这个值,因此我们可以直接乘以系数 C n 1 C_{n}^{1} Cn1,但是当 θ ≥ 180 ° \theta \geq 180\degree θ≥180°时,这个条件就不一定满足了,因此推导的结果自然也就不再满足了。
综上,我们就说明白了为啥上述结论在 θ ≥ 180 ° \theta \geq 180\degree θ≥180°时不成立。
不过真要求当 θ ≥ 180 ° \theta \geq 180 \degree θ≥180°的情况下时的解的话,事实上也可以仿照上述受限制下的分布给出 P n ( θ ) P_n(\theta) Pn(θ)的概率如下:
P n ( θ ) = 1 − ∫ 0 2 π − θ d θ 1 ∫ 0 m i n ( 2 π − θ , 2 π − θ 1 ) d θ 2 . . . ∫ 0 m i n ( 2 π − θ , 2 π − ∑ i = 1 n − 2 θ i ) d θ n − 1 ∫ 0 m i n ( 2 π − θ , 2 π − ∑ i = 1 n − 1 θ i ) δ ( θ n = 2 π − ∑ i = 1 n − 1 θ i ) d θ n ∫ 0 2 π d θ 1 ∫ 0 2 π − θ 1 d θ 2 . . . ∫ 0 2 π − ∑ i = 1 n − 2 θ i d θ n − 1 ∫ 0 2 π − ∑ i = 1 n − 1 θ i δ ( θ n = 2 π − ∑ i = 1 n − 1 θ i ) d θ n P_n(\theta) = 1 - \frac{\int_{0}^{2\pi-\theta}d\theta_1 \int_{0}^{\mathop{min}(2\pi-\theta, 2\pi-\theta_1)}d\theta_2 ... \int_{0}^{\mathop{min}(2\pi-\theta, 2\pi-\sum\limits_{i=1}^{n-2}\theta_i)}d\theta_{n-1} \int_{0}^{\mathop{min}(2\pi-\theta, 2\pi-\sum\limits_{i=1}^{n-1}\theta_i)}\delta(\theta_n = 2\pi-\sum\limits_{i=1}^{n-1}\theta_i) d\theta_{n}}{\int_{0}^{2\pi}d\theta_1\int_{0}^{2\pi-\theta_1}d\theta_2 ... \int_{0}^{2\pi-\sum\limits_{i=1}^{n-2}\theta_i}d\theta_{n-1} \int_{0}^{2\pi-\sum\limits_{i=1}^{n-1}\theta_i} \delta(\theta_n = 2\pi-\sum\limits_{i=1}^{n-1}\theta_i) d\theta_{n}} Pn(θ)=1−∫02πdθ1∫02π−θ1dθ2...∫02π−i=1∑n−2θidθn−1∫02π−i=1∑n−1θiδ(θn=2π−i=1∑n−1θi)dθn∫02π−θdθ1∫0min(2π−θ,2π−θ1)dθ2...∫0min(2π−θ,2π−i=1∑n−2θi)dθn−1∫0min(2π−θ,2π−i=1∑n−1θi)δ(θn=2π−i=1∑n−1θi)dθn
即在 ∑ i = 1 n θ i = 2 π \sum\limits_{i=1}^{n}\theta_i=2\pi i=1∑nθi=2π的情况下,至少存在一个 θ i \theta_i θi使得 θ i ≥ 2 π − θ \theta_i \geq 2\pi-\theta θi≥2π−θ时的概率。
事实上,考虑到 δ \delta δ函数事实上已经内隐的限制条件内容,我们可以简化上式为:
P n ( θ ) = 1 − ∫ 0 2 π − θ d θ 1 ∫ 0 2 π − θ d θ 2 . . . ∫ 0 2 π − θ d θ n − 1 ∫ 0 2 π − θ δ ( θ n = 2 π − ∑ i = 1 n − 1 θ i ) d θ n ∫ 0 2 π d θ 1 ∫ 0 2 π − θ 1 d θ 2 . . . ∫ 0 2 π − ∑ i = 1 n − 2 θ i d θ n − 1 ∫ 0 2 π − ∑ i = 1 n − 1 θ i δ ( θ n = 2 π − ∑ i = 1 n − 1 θ i ) d θ n P_n(\theta) = 1 - \frac{\int_{0}^{2\pi-\theta}d\theta_1 \int_{0}^{2\pi-\theta}d\theta_2 ... \int_{0}^{2\pi-\theta}d\theta_{n-1} \int_{0}^{2\pi-\theta}\delta(\theta_n = 2\pi-\sum\limits_{i=1}^{n-1}\theta_i) d\theta_{n}}{\int_{0}^{2\pi}d\theta_1\int_{0}^{2\pi-\theta_1}d\theta_2 ... \int_{0}^{2\pi-\sum\limits_{i=1}^{n-2}\theta_i}d\theta_{n-1} \int_{0}^{2\pi-\sum\limits_{i=1}^{n-1}\theta_i} \delta(\theta_n = 2\pi-\sum\limits_{i=1}^{n-1}\theta_i) d\theta_{n}} Pn(θ)=1−∫02πdθ1∫02π−θ1dθ2...∫02π−i=1∑n−2θidθn−1∫02π−i=1∑n−1θiδ(θn=2π−i=1∑n−1θi)dθn∫02π−θdθ1∫02π−θdθ2...∫02π−θdθn−1∫02π−θδ(θn=2π−i=1∑n−1θi)dθn
但是,不幸的是,即便有了上述一般的表达式,这依然不是一个可以快速求解的表达式,甚至不确定这个表达式能否真的去解析求解。
不过问了我同学之后,他倒是修正了迭代的方法,给出了一个非常完美的回答,不过这里我就先不做展开了,后面我会花点时间把他的解答好好整理一下之后然后再发出来吧。