leetcode 236 二叉树最近公共祖先

Given a binary tree, find the lowest common ancestor (LCA) of two given nodes in the tree.

According to the definition of LCA on Wikipedia: “The lowest common ancestor is defined between two nodes p and q as the lowest node in T that has both p and q as descendants (where we allow a node to be a descendant of itself).”

Given the following binary tree: root = [3,5,1,6,2,0,8,null,null,7,4]

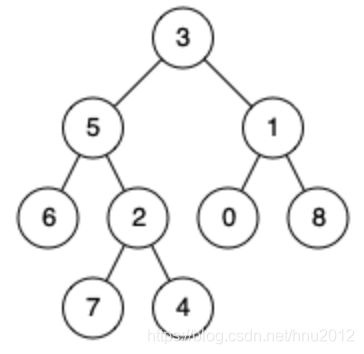
Example 1:

Input: root = [3,5,1,6,2,0,8,null,null,7,4], p = 5, q = 1
Output: 3
Explanation: The LCA of nodes 5 and 1 is 3.
Example 2:

Input: root = [3,5,1,6,2,0,8,null,null,7,4], p = 5, q = 4
Output: 5
Explanation: The LCA of nodes 5 and 4 is 5, since a node can be a descendant of itself according to the LCA definition.

思路：从根结点开始遍历，如果与p或q匹配，则返回，递归遍历root结点的左右子树，如果一个结点出现在左子树，一个出现在右子树，那root就是其公共结点；如果两个结点都出现在左子树，那其最近公共祖先肯定在左子树；反之，其最近公共祖先都出现在右子树。
代码如下：
另一种思路是遍历树，记录每个节点对应的父结点，可以用 dict 存下， 然后记录结点 p 到 root 结点路径的所有结点，存在 dict(a) 中，再遍历节点 q，遍历其父结点，一直到 root 结点，如果结点在 dict(a)中，该节点就是两者最近的公共祖先。

def lowestCommonAncestor(self, root, p, q):
        """
        :type root: TreeNode
        :type p: TreeNode
        :type q: TreeNode
        :rtype: TreeNode
        """
        if root==None or root==p or root==q:
            return root
        left=self.lowestCommonAncestor(root.left,p,q)
        right=self.lowestCommonAncestor(root.right,p,q)
        if left!=None:
            if right==None:
                return left
            else:
                return root
        else:  ### 注意这儿不用写如果 right==None, return root
            return right