web技术分享| LRU 缓存淘汰算法

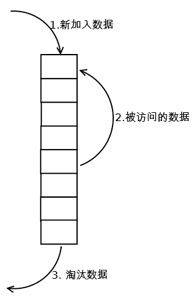
了解 LRU 之前，我们应该了解一下缓存，大家都知道计算机具有缓存内存，可以临时存储最常用的数据，当缓存数据超过一定大小时，系统会进行回收，以便释放出空间来缓存新的数据，但从系统中检索数据的成本比较高。

缓存要求：

• 固定大小：缓存需要有一些限制来限制内存使用。
• 快速访问：缓存插入和查找操作应该很快，最好是 O(1) 时间。
• 在达到内存限制的情况下替换条目：缓存应该具有有效的算法来在内存已满时驱逐条目

如果提供一个缓存替换算法来辅助管理，按照设定的内存大小，删除最少使用的数据，在系统回收之前主动释放出空间，会使得整个检索过程变得非常快，因此 LRU 缓存淘汰算法就出现了。

LRU 原理与实现

LRU (Least Recently Used) 缓存淘汰算法提出最近被频繁访问的数据应具备更高的留存，淘汰那些不常被访问的数据，即最近使用的数据很大概率将会再次被使用，抛弃最长时间未被访问的数据，目的是为了方便以后获取数据变得更快，例如 Vue 的 keep-live 组件就是 LRU 的一种实现。

实现的中心思想拆分为以下几步：

• 新的数据插入到链表头部。
• 每当缓存命中（即缓存数据被访问），则将数据移到链表头部。
• 当缓存内存已满时（链表数量已满时），将链表尾部的数据淘汰。

Example

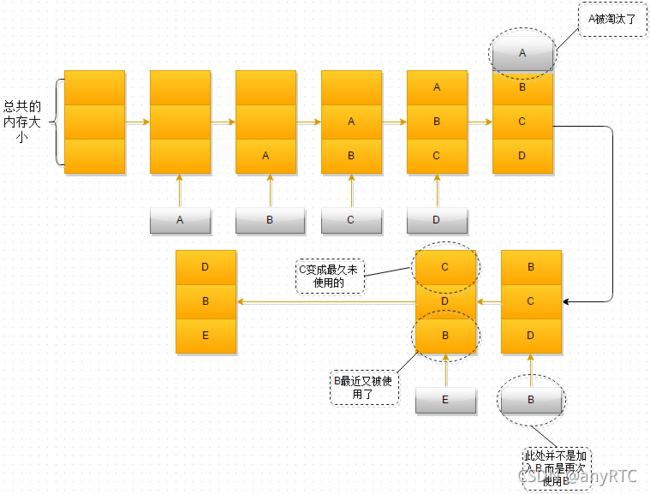
这里使用一个例子来说明 LRU 实现的流程，详细请参考这里。

1. 最开始时，内存空间是空的，因此依次进入A、B、C是没有问题的
2. 当加入D时，就出现了问题，内存空间不够了，因此根据LRU算法，内存空间中A待的时间最为久远，选择A,将其淘汰
3. 当再次引用B时，内存空间中的B又处于活跃状态，而C则变成了内存空间中，近段时间最久未使用的
4. 当再次向内存空间加入E时，这时内存空间又不足了，选择在内存空间中待的最久的C将其淘汰出内存，这时的内存空间存放的对象就是E->B->D

基于双向链表和 HashMap 实现 LRU

常见的 LRU 算法是基于双向链表和 HashMap 实现的。

双向链表：用于管理缓存数据结点的顺序，新增数据和缓存命中（最近被访问）的数据被放置在 Header 结点，尾部的结点根据内存大小进行淘汰。

HashMap：存储所有结点的数据，当 LRU 缓存命中（进行数据访问）时，进行拦截进行数据置换和删除操作。

双向链表

双向链表是众多链表中的一种，链表都是采用链式存储结构，链表中的每一个元素，我们称之为数据结点。

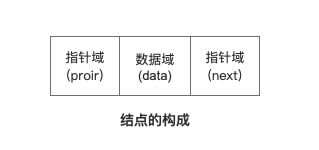
每个数据结点都包含一个数据域和指针域，指针域可以确定结点与结点之间的顺序，通过更新数据结点的指针域的指向可以更新链表的顺序。

双向链表的每个数据结点包含一个数据域和两个指针域：

• proir 指向上一个数据结点；
• data 当前数据结点的数据；
• next 指向下一个数据结点；

指针域确定链表的顺序，那么双向链表拥有双向指针域，数据结点的之间不在是单一指向，而是双向指向。即 proir 指针域指向上一个数据结点，next 指针域指向下一个数据结点。

同理：

• 单向链表只有一个指针域。
• 循环（环状）链表则是拥有双向指针域，且头部结点的指针域指向尾部结点，尾部结点的指针域指向头部结点。

特殊结点：Header 和 Tailer 结点

链表中还有两个特殊的结点，那就算 Header 结点和 Tailer 结点，分别表示头部结点和尾部结点，头部结点表示最新的数据或者缓存命中（最近访问过的数据），尾部结点表示长时间未被使用，即将被淘汰的数据节点。

作为算法大家都会关注其时间和空间复杂度 O(n)，基于双向链表双向指针域的优势，为了降级时间复杂度，因此
为了保证 LRU 新数据和缓存命中的数据都位于链表最前面（Header），缓存淘汰的时候删除最后的结点（Tailer），又要避免数据查找时从头到尾遍历，降低算法的时间复杂度，同时基于双向链表带来的优势，可以改变个别数据结点的指针域从而达到链表数据的更新,如果提供 Header 和 Tailer 结点作为标识的话，可以使用头插法快速增加结点，根据 Tailer 结点也可以在缓存淘汰时快速更新链表的顺序，避免遍历从头到尾遍历，降低算法的时间复杂度。

排序示例

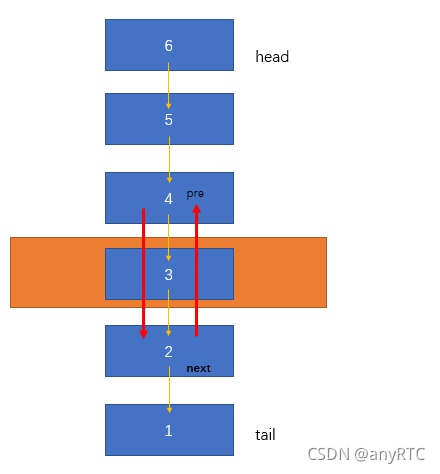
LRU 链表中有 [6，5，4，3，2，1] 6个数据结点，其中 6 所在的数据结点为 Header（头部）结点，1 所在的数据结点为 Tailer（尾部）结点。如果此时数据 3 被访问（缓存命中），3 应该被更新至链表头，用数组的思维应该是删除 3，但是如果我们利用双向链表双向指针的优势，可以快速的实现链表顺便的更新：

• 3 被删除时，4 和 2 中间没有其他结点，即 4 的 next 指针域指向 2 所在的数据结点；同理，2 的 proir 指针域指向 2 所在的数据结点。

HashMap

至于为什么使用 HashMap，用一句话来概括主要是因为 HashMap 通过 Key 获取速度会快的多，降低算法的时间复杂度。

例如：

• 我们在 get 缓存的时候从 HashMap 中获取的时候基本上时间复杂度控制在 O(1)，如果从链表中一次遍历的话时间复杂度是 O(n)。
• 我们访问一个已经存在的节点时候，需要将这个节点移动到 header 节点后，这个时候需要在链表中删除这个节点，并重新在 header 后面新增一个节点。这个时候先去 HashMap 中获取这个节点删除节点关系，避免了从链表中遍历，将时间复杂度从 O(N) 减少为 O(1)

由于前端没有 HashMap 的相关 API，我们可以使用 Object 或者 Map 来代替。

代码实现

现在让我们运用所掌握的数据结构，设计和实现一个，或者参考 LeeCode 146 题。

链表结点 Entry

export class Entry<T> {

	value: T

	key: string | number

	next: Entry<T>

	prev: Entry<T>

	constructor(val: T) {
		this.value = val;
	}
}

双向链表 Double Linked List

主要职责：

• 管理头部结点和尾部结点
• 插入新数据时，将新数据移到头部结点
• 删除数据时，更新删除结点前后两个结点的指向域

/**
* Simple double linked list. Compared with array, it has O(1) remove operation.
* @constructor
*/
export class LinkedList<T> {

	head: Entry<T>
	tail: Entry<T>

	private _len = 0

	/**
	* Insert a new value at the tail
	*/
	insert(val: T): Entry<T> {
		const entry = new Entry(val);
		this.insertEntry(entry);
		return entry;
	}

	/**
	* Insert an entry at the tail
	*/
	insertEntry(entry: Entry<T>) {
		if (!this.head) {
			this.head = this.tail = entry;
		}
		else {
			this.tail.next = entry;
			entry.prev = this.tail;
			entry.next = null;
			this.tail = entry;
		}
		this._len++;
	}

	/**
	* Remove entry.
	*/
	remove(entry: Entry<T>) {
		const prev = entry.prev;
		const next = entry.next;

		if (prev) {
			prev.next = next;
		}
		else {
			// Is head
			this.head = next;
		}
		if (next) {
			next.prev = prev;
		}
		else {
			// Is tail
			this.tail = prev;
		}
		entry.next = entry.prev = null;
		this._len--;
	}

	/**
	* Get length
	*/
	len(): number {
		return this._len;
	}

	/**
	* Clear list
	*/
	clear() {
		this.head = this.tail = null;
		this._len = 0;
	}
}

LRU 核心算法

主要职责：

• 将数据添加到链表并更新链表顺序
• 缓存命中时更新链表的顺序
• 内存溢出抛弃过时的链表数据

/**

* LRU Cache

*/

export default class LRU<T> {

	private _list = new LinkedList<T>()

	private _maxSize = 10

	private _lastRemovedEntry: Entry<T>

	private _map: Dictionary<Entry<T>> = {}

	constructor(maxSize: number) {

		this._maxSize = maxSize;

	}

	/**

	* @return Removed value

	*/

	put(key: string | number, value: T): T {

		const list = this._list;

		const map = this._map;

		let removed = null;

		if (map[key] == null) {

			const len = list.len();

			// Reuse last removed entry

			let entry = this._lastRemovedEntry;

			if (len >= this._maxSize && len > 0) {

				// Remove the least recently used

				const leastUsedEntry = list.head;

				list.remove(leastUsedEntry);

				delete map[leastUsedEntry.key];

				removed = leastUsedEntry.value;

				this._lastRemovedEntry = leastUsedEntry;
				}
			if (entry) {
				entry.value = value;
			}
			else {
				entry = new Entry(value);
			}

			entry.key = key;
			list.insertEntry(entry);
			map[key] = entry;
		}
		return removed;
	}

	get(key: string | number): T {

		const entry = this._map[key];
		const list = this._list;

		if (entry != null) {
			// Put the latest used entry in the tail
			if (entry !== list.tail) {
				list.remove(entry);
				list.insertEntry(entry);
			}
			return entry.value;
		}
	}

	/**
	* Clear the cache
	*/
	clear() {
		this._list.clear();
		this._map = {};
	}

	len() {
		return this._list.len();
	}
}

其他 LRU 算法

除了以上常见的 LRU 算法，随着需求的复杂多样，基于 LRU 的思想也衍生出了许多优化算法，例如：

• LRU-K 算法
• LRU-Two queues(2Q)算法
• LRU-Multi queues(MQ)算法
• LFU 算法
• LRU变种算法

参考链接

• Zrender - LRU
• 知乎 - 存淘汰算法–LRU算法
• LRU算法
• LRU 策略详解和实现