- 软件设计师之树与二叉树:非线性数据结构的深度探索
一杯年华@编程空间
软考中级数据结构
软件设计师之树与二叉树:非线性数据结构的深度探索在软件开发领域,数据结构是程序设计的核心基础,其中树和二叉树作为重要的非线性数据结构,在众多场景中都有着广泛应用。我写这篇博客,就是希望和大家一起学习进步,深入解析树和二叉树的相关知识,用通俗易懂的语言结合图表和Java代码示例进行讲解,帮助大家更好地掌握这些内容。一、树的定义与基本概念树的定义树是由n(n≥0)个结点组成的有限集合。当n=0时,为空
- c51中断优先级c语言,51单片机的中断优先级及中断嵌套
金融四十人论坛
c51中断优先级c语言
说最基本的,老的51单片机(80C51系列)有5个中断源,2个优先级,可以实现二级中断服务嵌套。现在很多扩展的51单片机已经有4个优先级(或更多)和更多的中断源了。在说到中断之前,我先来定义一下优先级,明白了什么是优先级,后面的阐述就容易明白了。实际上很多人都是混淆了优先级的含义,所以才觉得糊里糊涂。中断的优先级有两个:查询优先级和执行优先级。什么是查询优级呢?我们从datasheet或书上看到的
- http框架核心之ngx_http.c源码分析
qiuhui00
nginx源码分析nginx源码分析http框架
ngx_http.c内主要实现了一个模块:ngx_http_module。ngx_http_module是nginx的http框架的一部分,它是所有http模块能够被加载的唯一入口,承担了http块配置解析,合并,以及http框架及其相关数据结构的初始化。它本身是NGX_CORE_MODULE类型,只有一个指令,就是http,如下所示:staticngx_command_tngx_http_com
- Java数据类型 Arrays VS ArraysList VS LikedList 解析
fantasy_4
Javajava
在学习Java过程中,在刷题时总是搞不清楚这三种数据结构的区别,打算写篇文章记录一下ArraysVSArrayListArrayListVSLinkedList总结ArraysVSArrayListArraysArrayList类型Java的基本数据类型Java集合框架中的一个类,实现了List接口存储内容基本数据类型+对象引用对象引用可变性数组长度创建后不可变长度可变适用场景查询元素会比较快,直
- C语言中,#define和typedef 定义int* 一个容易混淆的点
阿龍1787
C++随记c语言
前言首先来看一个代码:#include#include#defineint_ptrint*intmain(){intc=100;int_ptra,b;//等效于int*a,b;那么b就是int类型,不是int*类型a=&c;b=&c;//报错return0;}原意,我本来想让a和b都是int*类型,但是发现并不是。这段代码的主要问题在于宏定义和指针声明的使用方式上:当使用#defineint_pt
- Pandas完全指南:数据处理与分析从入门到实战
xiaoyu❅
pythonpythonpandas开发语言
目录引言一、Pandas环境配置与核心概念1.1安装Pandas1.2导入惯例1.3核心数据结构二、数据结构详解2.1Series创建与操作2.2DataFrame创建三、数据查看与基本操作3.1数据预览3.2索引与选择3.3数据排序四、数据清洗实战4.1处理缺失值4.2处理重复值4.3数据类型转换4.4字符串处理五、数据处理进阶5.1数据筛选5.2列操作5.3应用函数六、数据分组与聚合6.1基础
- 嵌入式C语言学习笔记(2)
愿抬头有阳光
c语言学习笔记
1.数组指针数组指针本质上就是一个指针,它里面存放的是数组的首地址。#includevoidshow(int(*p)[4],intn){for(inti=0;i4*4=16;3.命令行传递参数,main函数的标准格式intmain(intargc,constchar*argv[]){return0;}//argc:参数的个数包括./a.out//argv:参数的值列表argv[0]="./a.ou
- 【C++】:位图(bitset)
-元清-
重制C++版c++开发语言c语言数据结构算法
目录位图的概念位图的应用场景位图的构造函数位图的使用位图的概念位图(Bitmap)是一种基于二进制位(bit)的高效数据结构,用于表示一组布尔值(存在或不存在、真或假)。它的核心思想是:用每一个二进制位(0或1)来标记某个状态或资源是否被占用。第i位为1→表示第i个元素存在/被占用。第i位为0→表示第i个元素不存在/未被占用。关键特性:内存高效:每个布尔值仅占用1个二进制位(bit),而非传统布尔
- C语言基础(函数)
指尖DE格桑花
c语言开发语言初学者嵌入式
函数的概述函数:实现一定功能的,独立的代码模块。对于函数的使用,一定是先定义,后使用。使用函数的优势:①我们可以通过函数提供功能给别人使用。当然我们也可以使用别人提供的函数,减少代码量。②借助函数可以减少重复性的代码。③实现结构化(模块化)程序设计思想。关于结构化设计思想:将大型的任务功能划分为相互独立的小型的任务模块来设计。函数是C语言程序的基本组成单元:C语言程序是由一个(必然是main函数)
- 【数据库】MySQL的索引详解
此木|西贝
数据库数据库mysql
简介索引是一种用于快速查询和检索数据的数据结构,类似于书的目录。在几百页的书通过几页目录就可以精确定位到我们想看的章节优点和缺点优点正确的使用索引可以大大提高检索速度可以使用唯一索引保证数据在库中的唯一性使用聚合索引减少回表,降低IO次数缺点索引不宜创建的太多,否则增删改时不仅修改数据,还要修改大量的索引数据索引也会占用磁盘空间索引结构B树:多路平衡查找树,B树的所有节点都会存储key(索引)和d
- 深度剖析C语言数组和指针的区别(非常详细)
xiecoding.cn
c语言算法开发语言c++青少年编程C语言入门数据结构
对于刚刚接触C语言指针的初学者,很容易认为数组和指针是等价的,数组名表示数组的首地址。不幸的是,这是一种非常危险的想法,并不完全正确。耐心看完本文,我保证会颠覆你的认知。数组和指针绝不等价数组和指针不等价的一个典型案例就是求数组的长度,这个时候只能使用数组名,不能使用数组指针,前面我们已经强调过了,这里不妨再来演示一下:#includeintmain(){inta[6]={0,1,2,3,4,5}
- 深入理解 TypeScript 中的迭代器(Iterators)与生成器(Generators)
念九_ysl
typescript前端typescript
一、为什么需要迭代协议?在现代JavaScript/TypeScript开发中,我们经常需要处理各种集合型数据:数组、Map、Set甚至是自定义数据结构。ES6引入的迭代协议(IterationProtocols)正是为了解决统一遍历机制的问题。通过迭代器模式,我们可以:为不同的数据结构提供统一的访问接口实现惰性计算(LazyEvaluation)支持现代语言特性(for...of,扩展运算符等)
- 成为编程大佬!!----->数据结构与算法(2)——顺序表!!
Elnaij
算法数据结构c语言
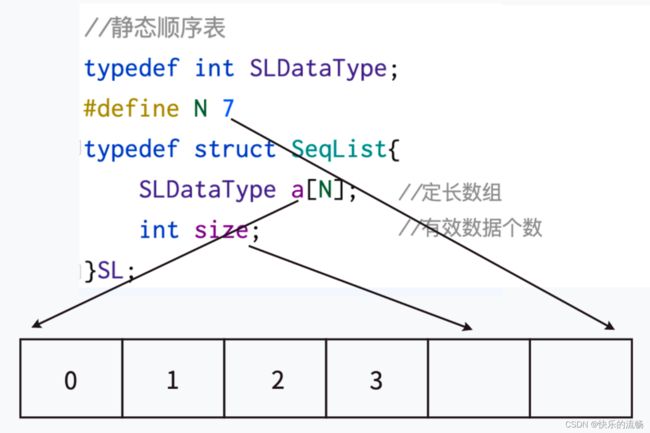
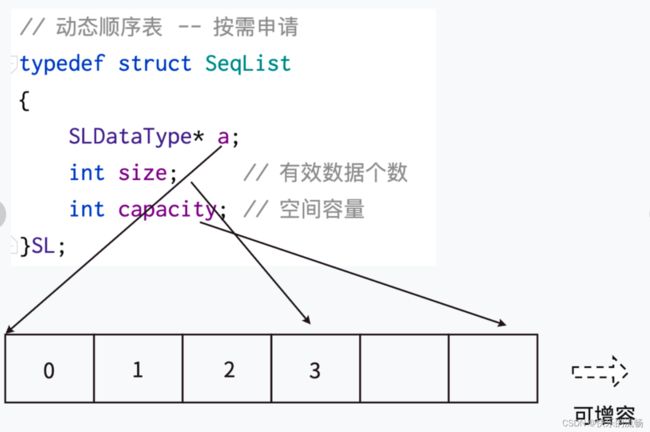
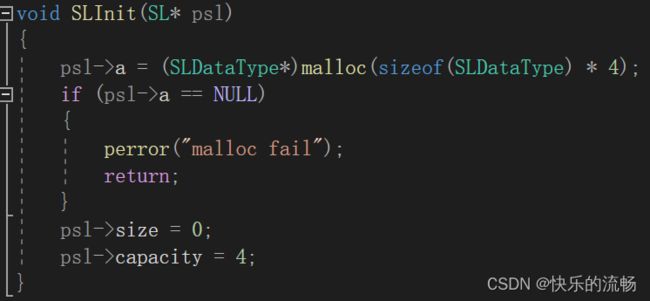
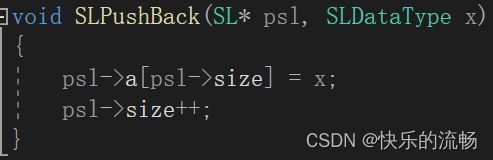
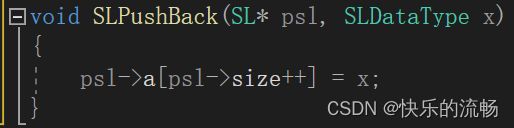
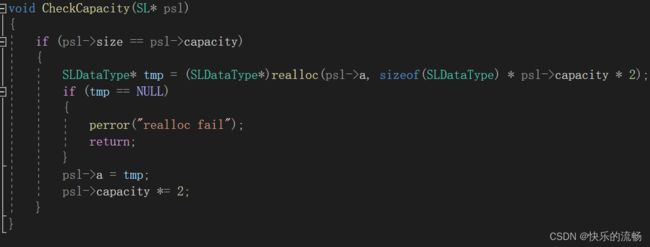
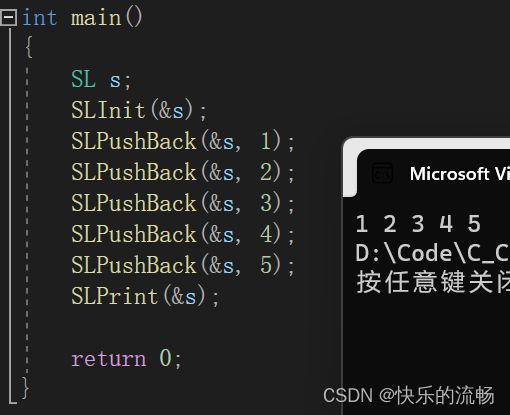
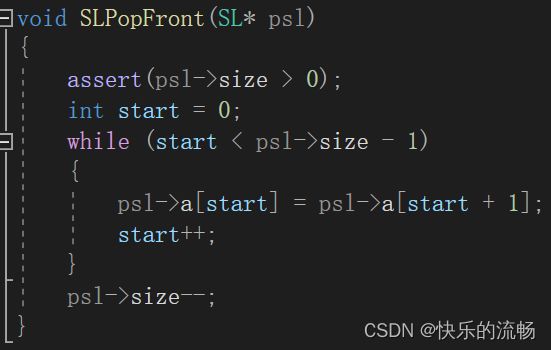
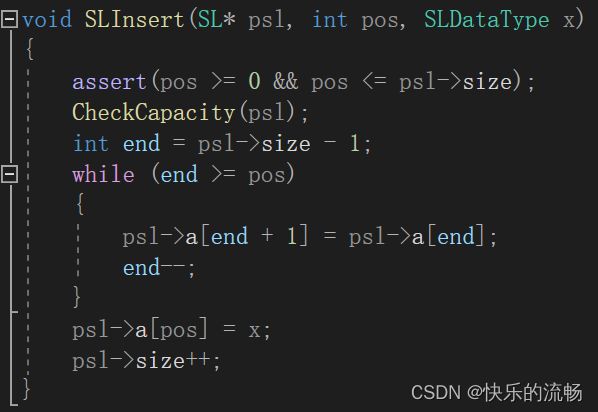
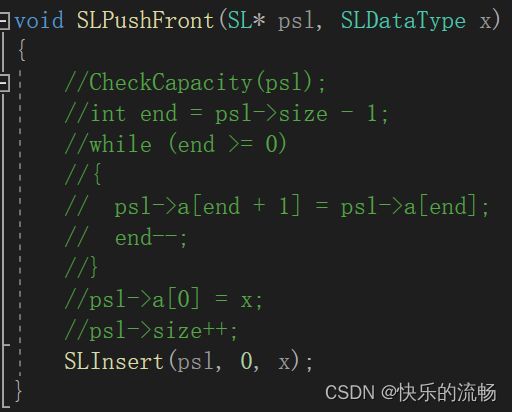
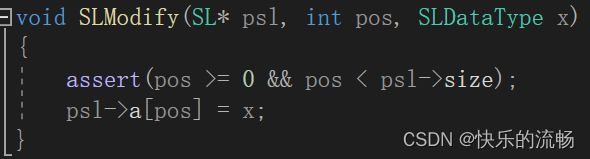
前言:线性表是数据结构与算法的重中之重,所有具有线性逻辑结构的数据结构,都能称为线性表。这篇文章我们先来讨论线性表中的顺序表,顺序表和线性表都是后续实现栈,树,串和图等等结构的重要基础。目录❀简单介绍线性表❀顺序表❀顺序表的存储❀动态存储❀静态存储❀静态存储与动态存储的优缺点❀顺序表操作❀1.初始化顺序表❀2.销毁顺序表❀3.插入数据❀插入数据之判断已满否❀插入操作之尾插❀插入操作之头插❀插入数据
- 基于C语言的数据结构之串——带你熟练掌握串的基本操作!!超级详细!!
Elnaij
数据结构c语言算法
目录前言1.数据结构——串1.1基本知识主串、子串、模式串1.2对几个字符串库函数的简单介绍1.2.1strcmp1.2.2strcpy1.2.3strlen1.2.4strcat1.3串的分类1.3.1静态分配内存的串1.3.2动态分配内存的串2.串的基本操作2.1初始化串2.2输出字符2.3插入子串2.4删除子串2.5取子串操作2.6撤销删除操作结束语前言掌握串之前最好先去学习好顺序表和单链表
- C++与C语言的区别
@haihi
c++c语言开发语言
前言本文主要用C语言和C++做对比来学习C++,便于个人理解。C++包含C语言,是对C语言的扩展,在C++中,支持C语言的语法使用,C++是C语言的超集一、C++与C语言的区别C语言简单高效,适合低级系统编程和硬件相关的开发。C++更加灵活、强大,适合大型项目开发,尤其是需要面向对象、代码复用和复杂数据结构的应用。1.编程范式C语言:C是一种过程式编程语言,主要关注函数和过程。程序是通过一系列函数
- 从C语言开始的C++编程生活(1)
Elnaij
C++基础知识c语言c++
前言本系列文章承接C语言的学习,需要有C语言的基础才能学会哦。第1篇主要讲的是有关于C++的命名空间、输入和输出。C++才起步,都很简单呢!目录前言命名空间namespace基本语法作用使用命名空间域作用限定符::基本语法usingnamespace命名空间域名基本语法作用using命名空间域名::变量名基本语法作用C++的输入和输出“>”流提取符基本语法代码解释命名空间namespace基本语法
- 什么是Apache Avro?
maozexijr
apache
什么是ApacheAvro?ApacheAvro是一个开源的数据序列化框架,主要用于高效的数据交换和存储。它由ApacheHadoop项目开发,广泛应用于大数据生态系统中(如Hadoop、Kafka等)。Avro提供了一种紧凑、快速的二进制数据格式,同时支持丰富的数据结构和模式演化。核心特性跨语言支持Avro支持多种编程语言(如Java、Python、C++、Go等),使得不同语言之间的数据交换变
- Python常用的库讲解(易懂版)
不辉放弃
python开发语言
NumPy:用于科学计算的基础库,提供多维数组对象、各种派生对象和对数组执行操作的工具。importnumpyasnp#创建一个numpy数组arr=np.array([1,2,3,4,5])print(arr)Pandas:数据处理库,提供数据结构和数据分析工具,特别适合处理结构化数据。importpandasaspd#创建一个Pandas数据帧df=pd.DataFrame({'A':[1,2
- HoRain云--Node.js文件下载服务实战:Express实现安全高效的文件传输
HoRain云小助手
node.jsexpress安全
HoRain云小助手:个人主页个人专栏:《Linux系列教程》《c语言教程》⛺️生活的理想,就是为了理想的生活!⛳️推荐前些天发现了一个超棒的服务器购买网站,性价比超高,大内存超划算!忍不住分享一下给大家。点击跳转到网站。专栏介绍专栏名称专栏介绍《C语言》本专栏主要撰写C干货内容和编程技巧,让大家从底层了解C,把更多的知识由抽象到简单通俗易懂。《网络协议》本专栏主要是注重从底层来给大家一步步剖析网
- 学习第十一天-树
大橙子房
ai学习
一、树的基础概念1.定义树是一种非线性数据结构,由n个有限节点组成层次关系集合。特点:有且仅有一个根节点其余节点分为若干互不相交的子树节点间通过父子关系连接2.关键术语术语定义节点包含数据和子节点引用的单元根节点树的起始节点,没有父节点子节点直接连接到父节点的节点叶子节点没有子节点的节点度节点拥有的子树数目树的高度从根节点到最远叶子节点的最长路径边数树的深度从根节点到当前节点的层数路径从根到某节点
- Windows 图形显示驱动开发-WDDM 3.0功能- IOMMU DMA 重新映射(二)
程序员王马
windows图形显示驱动开发驱动开发
地址描述符列表为了同时支持物理和逻辑访问模式,并在运行时无缝切换这两种模式,Dxgkrnl提供了一个描述地址描述符列表(ADL)的DXGK_ADL结构。此数据结构类似于MDL,但描述了一个可以是物理或逻辑的页面数组。由于这些页可以是逻辑页,因此不能将ADL描述的地址映射到虚拟地址以直接访问CPU。DxgkddiBuildpagingbuffer的DXGK_OPERATION_MAP_APERTUR
- 文件的输出与读写 2.0
大力水手偷吃菠菜变成米老鼠
c语言
一、文章内容概述(一)知识要点文件操作函数概述:介绍了C语言中用于文件操作的一系列函数,这些函数是实现文件读写功能的基础工具。文件流概念定义与分类:FILE*stream这种定义方式包含了各种各样的流。流是一种用于在程序和外部设备(如文件、控制台、网络等)之间进行数据传输的抽象概念。具体类型文件流:用于读取与写入在磁盘上的文件。例如,通过文件流可以从硬盘上的文本文件中读取数据,并将其显示在程序中,
- 【华为OD机试】日志采集系统--C语言
weixin_51635462
华为odc语言开发语言
#includeintinput[1000]={0};intfun(intx){intret=0;for(inti=0;i100){printf("100");}else{for(intj=1;j100){max_score=max_score>(100-fun(j))?max_score:(100-fun(j));break;}else{max_score=max_score>(cnt-fun(
- 学习单片机需要多长时间才能进行简单的项目开发?
无际单片机编程
单片机嵌入式硬件stm32嵌入式java
之前有老铁问我,学单片机到底要多久,才能进行简单的项目开发?是三个月速成,还是三年磨一剑?今天咱们就来聊聊这个话题,我不是什么高高在上的专家,就是个踩过无数坑、烧过几块板子的“技术老友”。本文将用最接地气的话给你讲清楚,答案可能比你想的简单,也可能比你想的残酷,但肯定会让你心里有谱。单片机这东西,入门的第一道坎其实没那么高。你得先搞清楚几件基本装备:C语言、硬件基础、开发工具。C语言是单片机的“母
- Objective-C语言的网络编程
俞嫦曦
包罗万象golang开发语言后端
Objective-C语言中的网络编程引言Objective-C是一种面向对象的编程语言,广泛应用于iOS和macOS应用程序的开发。随着移动互联网的快速发展,网络编程成为了现代应用程序开发中不可或缺的一部分。无论是从服务器获取数据、上传文件,还是实现实时通信,网络编程都扮演着至关重要的角色。本文将深入探讨Objective-C语言中的网络编程,涵盖从基础的网络请求到高级的异步处理、安全通信等内容
- 【C语言】:学生管理系统(多文件版)
彬彬1313
c语言开发语言经验分享学习方法笔记
一、文件框架二、Datadata.txt三、Inc1.list.h学生结构体#ifndef__LIST_H__#define__LIST_H__#include#include#include#include#include#defineMAX_LEN20//学生信息结构体typedefstruct{//登录用charuser_name[128];//账号charpassword[128];//密
- SparkSQL编程-RDD、DataFrame、DataSet
早拾碗吧
Sparksparkhadoop大数据sparksql
三者之间的关系在SparkSQL中Spark为我们提供了两个新的抽象,分别是DataFrame和DataSet。他们和RDD有什么区别呢?首先从版本的产生上来看:RDD(Spark1.0)—>Dataframe(Spark1.3)—>Dataset(Spark1.6)如果同样的数据都给到这三个数据结构,他们分别计算之后,都会给出相同的结果。不同是的他们的执行效率和执行方式。在后期的Spark版本中
- C语言的数据:变量、常量、数据类型及其使用
c++
目录引言变量与常量1.1变量1.2常量数据类型关键字C语言基本数据类型3.1整型(int)3.1.1定义与含义3.1.2内存占用和取值范围3.1.3声明和初始化3.1.4运算操作3.1.5实际应用场景3.2浮点型(float和double)3.2.1定义与含义3.2.2内存占用和取值范围3.2.3声明和初始化3.2.4运算操作3.2.5实际应用场景3.3字符型(char)3.3.1定义与含义3.3
- C++数据结构 数组加链表
哈儿1号
数据结构C++c++
#includeusingnamespacestd;//对于线性表有必要执行的操作://创建,撤销//确定线性表是否为空//确定线性表的长度//按索引查找一个元素//按元素查找索引。//按索引删除元素//按索引插入元素//从左到右的顺序输出线性表元素//这是个老祖宗templateclasslinearList{public:virtual~linearList(){};virtualboolem
- c语言编写字母解密,用c语言实现文本的加密及解密
傅南台
c语言编写字母解密
编写程序,实现对文本的加密及解密。加密程序代码:#include"stdio.h"main(){charc;FILE*fp1,*fp2;fp1=fopen("yuanwen.txt","r");fp2=fopen("miwen.txt","w");do{fscanf(fp1,"%c",&c);if(c>=32&&c=32&&c<=126){c=126-c;c=c+32;}fprintf(fp2,"
- 矩阵求逆(JAVA)初等行变换
qiuwanchi
矩阵求逆(JAVA)
package gaodai.matrix;
import gaodai.determinant.DeterminantCalculation;
import java.util.ArrayList;
import java.util.List;
import java.util.Scanner;
/**
* 矩阵求逆(初等行变换)
* @author 邱万迟
*
- JDK timer
antlove
javajdkschedulecodetimer
1.java.util.Timer.schedule(TimerTask task, long delay):多长时间(毫秒)后执行任务
2.java.util.Timer.schedule(TimerTask task, Date time):设定某个时间执行任务
3.java.util.Timer.schedule(TimerTask task, long delay,longperiod
- JVM调优总结 -Xms -Xmx -Xmn -Xss
coder_xpf
jvm应用服务器
堆大小设置JVM 中最大堆大小有三方面限制:相关操作系统的数据模型(32-bt还是64-bit)限制;系统的可用虚拟内存限制;系统的可用物理内存限制。32位系统下,一般限制在1.5G~2G;64为操作系统对内存无限制。我在Windows Server 2003 系统,3.5G物理内存,JDK5.0下测试,最大可设置为1478m。
典型设置:
java -Xmx
- JDBC连接数据库
Array_06
jdbc
package Util;
import java.sql.Connection;
import java.sql.DriverManager;
import java.sql.ResultSet;
import java.sql.SQLException;
import java.sql.Statement;
public class JDBCUtil {
//完
- Unsupported major.minor version 51.0(jdk版本错误)
oloz
java
java.lang.UnsupportedClassVersionError: cn/support/cache/CacheType : Unsupported major.minor version 51.0 (unable to load class cn.support.cache.CacheType)
at org.apache.catalina.loader.WebappClassL
- 用多个线程处理1个List集合
362217990
多线程threadlist集合
昨天发了一个提问,启动5个线程将一个List中的内容,然后将5个线程的内容拼接起来,由于时间比较急迫,自己就写了一个Demo,希望对菜鸟有参考意义。。
import java.util.ArrayList;
import java.util.List;
import java.util.concurrent.CountDownLatch;
public c
- JSP简单访问数据库
香水浓
sqlmysqljsp
学习使用javaBean,代码很烂,仅为留个脚印
public class DBHelper {
private String driverName;
private String url;
private String user;
private String password;
private Connection connection;
privat
- Flex4中使用组件添加柱状图、饼状图等图表
AdyZhang
Flex
1.添加一个最简单的柱状图
? 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28
<?xml version=
"1.0"&n
- Android 5.0 - ProgressBar 进度条无法展示到按钮的前面
aijuans
android
在低于SDK < 21 的版本中,ProgressBar 可以展示到按钮前面,并且为之在按钮的中间,但是切换到android 5.0后进度条ProgressBar 展示顺序变化了,按钮再前面,ProgressBar 在后面了我的xml配置文件如下:
[html]
view plain
copy
<RelativeLa
- 查询汇总的sql
baalwolf
sql
select list.listname, list.createtime,listcount from dream_list as list , (select listid,count(listid) as listcount from dream_list_user group by listid order by count(
- Linux du命令和df命令区别
BigBird2012
linux
1,两者区别
du,disk usage,是通过搜索文件来计算每个文件的大小然后累加,du能看到的文件只是一些当前存在的,没有被删除的。他计算的大小就是当前他认为存在的所有文件大小的累加和。
- AngularJS中的$apply,用还是不用?
bijian1013
JavaScriptAngularJS$apply
在AngularJS开发中,何时应该调用$scope.$apply(),何时不应该调用。下面我们透彻地解释这个问题。
但是首先,让我们把$apply转换成一种简化的形式。
scope.$apply就像一个懒惰的工人。它需要按照命
- [Zookeeper学习笔记十]Zookeeper源代码分析之ClientCnxn数据序列化和反序列化
bit1129
zookeeper
ClientCnxn是Zookeeper客户端和Zookeeper服务器端进行通信和事件通知处理的主要类,它内部包含两个类,1. SendThread 2. EventThread, SendThread负责客户端和服务器端的数据通信,也包括事件信息的传输,EventThread主要在客户端回调注册的Watchers进行通知处理
ClientCnxn构造方法
&
- 【Java命令一】jmap
bit1129
Java命令
jmap命令的用法:
[hadoop@hadoop sbin]$ jmap
Usage:
jmap [option] <pid>
(to connect to running process)
jmap [option] <executable <core>
(to connect to a
- Apache 服务器安全防护及实战
ronin47
此文转自IBM.
Apache 服务简介
Web 服务器也称为 WWW 服务器或 HTTP 服务器 (HTTP Server),它是 Internet 上最常见也是使用最频繁的服务器之一,Web 服务器能够为用户提供网页浏览、论坛访问等等服务。
由于用户在通过 Web 浏览器访问信息资源的过程中,无须再关心一些技术性的细节,而且界面非常友好,因而 Web 在 Internet 上一推出就得到
- unity 3d实例化位置出现布置?
brotherlamp
unity教程unityunity资料unity视频unity自学
问:unity 3d实例化位置出现布置?
答:实例化的同时就可以指定被实例化的物体的位置,即 position
Instantiate (original : Object, position : Vector3, rotation : Quaternion) : Object
这样你不需要再用Transform.Position了,
如果你省略了第二个参数(
- 《重构,改善现有代码的设计》第八章 Duplicate Observed Data
bylijinnan
java重构
import java.awt.Color;
import java.awt.Container;
import java.awt.FlowLayout;
import java.awt.Label;
import java.awt.TextField;
import java.awt.event.FocusAdapter;
import java.awt.event.FocusE
- struts2更改struts.xml配置目录
chiangfai
struts.xml
struts2默认是读取classes目录下的配置文件,要更改配置文件目录,比如放在WEB-INF下,路径应该写成../struts.xml(非/WEB-INF/struts.xml)
web.xml文件修改如下:
<filter>
<filter-name>struts2</filter-name>
<filter-class&g
- redis做缓存时的一点优化
chenchao051
redishadooppipeline
最近集群上有个job,其中需要短时间内频繁访问缓存,大概7亿多次。我这边的缓存是使用redis来做的,问题就来了。
首先,redis中存的是普通kv,没有考虑使用hash等解结构,那么以为着这个job需要访问7亿多次redis,导致效率低,且出现很多redi
- mysql导出数据不输出标题行
daizj
mysql数据导出去掉第一行去掉标题
当想使用数据库中的某些数据,想将其导入到文件中,而想去掉第一行的标题是可以加上-N参数
如通过下面命令导出数据:
mysql -uuserName -ppasswd -hhost -Pport -Ddatabase -e " select * from tableName" > exportResult.txt
结果为:
studentid
- phpexcel导出excel表简单入门示例
dcj3sjt126com
PHPExcelphpexcel
先下载PHPEXCEL类文件,放在class目录下面,然后新建一个index.php文件,内容如下
<?php
error_reporting(E_ALL);
ini_set('display_errors', TRUE);
ini_set('display_startup_errors', TRUE);
if (PHP_SAPI == 'cli')
die('
- 爱情格言
dcj3sjt126com
格言
1) I love you not because of who you are, but because of who I am when I am with you. 我爱你,不是因为你是一个怎样的人,而是因为我喜欢与你在一起时的感觉。 2) No man or woman is worth your tears, and the one who is, won‘t
- 转 Activity 详解——Activity文档翻译
e200702084
androidUIsqlite配置管理网络应用
activity 展现在用户面前的经常是全屏窗口,你也可以将 activity 作为浮动窗口来使用(使用设置了 windowIsFloating 的主题),或者嵌入到其他的 activity (使用 ActivityGroup )中。 当用户离开 activity 时你可以在 onPause() 进行相应的操作 。更重要的是,用户做的任何改变都应该在该点上提交 ( 经常提交到 ContentPro
- win7安装MongoDB服务
geeksun
mongodb
1. 下载MongoDB的windows版本:mongodb-win32-x86_64-2008plus-ssl-3.0.4.zip,Linux版本也在这里下载,下载地址: http://www.mongodb.org/downloads
2. 解压MongoDB在D:\server\mongodb, 在D:\server\mongodb下创建d
- Javascript魔法方法:__defineGetter__,__defineSetter__
hongtoushizi
js
转载自: http://www.blackglory.me/javascript-magic-method-definegetter-definesetter/
在javascript的类中,可以用defineGetter和defineSetter_控制成员变量的Get和Set行为
例如,在一个图书类中,我们自动为Book加上书名符号:
function Book(name){
- 错误的日期格式可能导致走nginx proxy cache时不能进行304响应
jinnianshilongnian
cache
昨天在整合某些系统的nginx配置时,出现了当使用nginx cache时无法返回304响应的情况,出问题的响应头: Content-Type:text/html; charset=gb2312 Date:Mon, 05 Jan 2015 01:58:05 GMT Expires:Mon , 05 Jan 15 02:03:00 GMT Last-Modified:Mon, 05
- 数据源架构模式之行数据入口
home198979
PHP架构行数据入口
注:看不懂的请勿踩,此文章非针对java,java爱好者可直接略过。
一、概念
行数据入口(Row Data Gateway):充当数据源中单条记录入口的对象,每行一个实例。
二、简单实现行数据入口
为了方便理解,还是先简单实现:
<?php
/**
* 行数据入口类
*/
class OrderGateway {
/*定义元数
- Linux各个目录的作用及内容
pda158
linux脚本
1)根目录“/” 根目录位于目录结构的最顶层,用斜线(/)表示,类似于
Windows
操作系统的“C:\“,包含Fedora操作系统中所有的目录和文件。 2)/bin /bin 目录又称为二进制目录,包含了那些供系统管理员和普通用户使用的重要
linux命令的二进制映像。该目录存放的内容包括各种可执行文件,还有某些可执行文件的符号连接。常用的命令有:cp、d
- ubuntu12.04上编译openjdk7
ol_beta
HotSpotjvmjdkOpenJDK
获取源码
从openjdk代码仓库获取(比较慢)
安装mercurial Mercurial是一个版本管理工具。 sudo apt-get install mercurial
将以下内容添加到$HOME/.hgrc文件中,如果没有则自己创建一个: [extensions] forest=/home/lichengwu/hgforest-crew/forest.py fe
- 将数据库字段转换成设计文档所需的字段
vipbooks
设计模式工作正则表达式
哈哈,出差这么久终于回来了,回家的感觉真好!
PowerDesigner的物理数据库一出来,设计文档中要改的字段就多得不计其数,如果要把PowerDesigner中的字段一个个Copy到设计文档中,那将会是一件非常痛苦的事情。