实验八 内部排序

实验八 内部排序
1.（必做题）在完善“12.11.4 参考源程序”的基础上，进行典型内部排序算法的比较。
(1) 随机产生整数样本，进行8种排序，并比较各种排序算法的执行时间，如执行时间均为0，可考虑增大样本，如加大至5000或10000。
(2) 设计方案，修改“12.11.4 参考源程序”，对8种排序算法的数据元素比较次数和移动次数进行比较。
(3) 修改“12.11.4 参考源程序”，输出8种排序算法每一趟排序的输出结果。

学习一下
第一篇1
第二篇2
抄来的参考代码如下：原链接

堆排序
堆的概念
堆：本质是一种数组对象。特别重要的一点性质：任意的叶子节点小于（或大于）它所有的父节点。对此，又分为大顶堆和小顶堆，大顶堆要求节点的元素都要大于其孩子，小顶堆要求节点元素都小于其左右孩子，两者对左右孩子的大小关系不做任何要求。
利用堆排序，就是基于大顶堆或者小顶堆的一种排序方法。下面，我们通过大顶堆来实现。

基本思想：
堆排序可以按照以下步骤来完成：

1.首先将序列构建称为大顶堆；
（这样满足了大顶堆那条性质：位于根节点的元素一定是当前序列的最大值）

2.构建大顶堆
3.取出当前大顶堆的根节点，将其与序列末尾元素进行交换；
（此时：序列末尾的元素为已排序的最大值；由于交换了元素，当前位于根节点的堆并不一定满足大顶堆的性质）
对交换后的n-1个序列元素进行调整，使其满足大顶堆的性质；

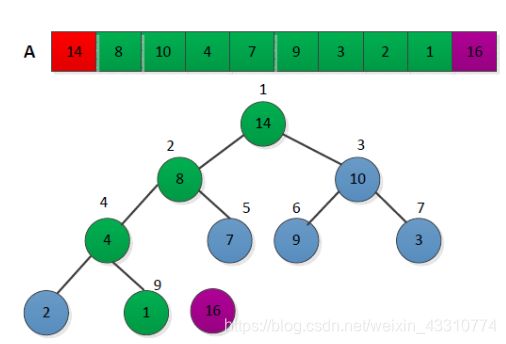
重复2.3步骤，直至堆中只有1个元素为止
代码实现：

#-------------------------堆排序--------------------------------
#**********获取左右叶子节点**********
def LEFT(i):
    return 2*i + 1
def RIGHT(i):
    return 2*i + 2
#********** 调整大顶堆 **********
#L:待调整序列 length: 序列长度 i:需要调整的结点
def adjust_max_heap(L,length,i):
#定义一个int值保存当前序列最大值的下标
    largest = i
#执行循环操作：两个任务：1 寻找最大值的下标；2.最大值与父节点交换
    while (1):
#获得序列左右叶子节点的下标
        left,right = LEFT(i),RIGHT(i)
#当左叶子节点的下标小于序列长度 并且 左叶子节点的值大于父节点时，将左叶子节点的下标赋值给largest
        if (left < length) and (L[left] > L[i]):
            largest = left
            print('左叶子节点')
        else:
            largest = i
#当右叶子节点的下标小于序列长度 并且 右叶子节点的值大于父节点时，将右叶子节点的下标值赋值给largest
        if (right < length) and (L[right] > L[largest]):
            largest = right
            print('右叶子节点')
#如果largest不等于i 说明当前的父节点不是最大值，需要交换值
        if (largest != i):
            temp = L[i]
            L[i] = L[largest]
            L[largest] = temp
            i = largest
            print(largest)
            continue
        else:
            break
#********** 建立大顶堆 **********
def build_max_heap(L):
    length = len(L)
    for x in range((int)((length-1)/2),-1,-1):
        adjust_max_heap(L,length,x)
#********** 堆排序 **********
def heap_sort(L):
#先建立大顶堆，保证最大值位于根节点；并且父节点的值大于叶子结点
    build_max_heap(L)
#i：当前堆中序列的长度.初始化为序列的长度
    i = len(L)
#执行循环：1. 每次取出堆顶元素置于序列的最后(len-1,len-2,len-3...)
 2. 调整堆，使其继续满足大顶堆的性质，注意实时修改堆中序列的长度
    while (i > 0):
        temp = L[i-1]
        L[i-1] = L[0]
        L[0] = temp
#堆中序列长度减1
        i = i-1
#调整大顶堆
        adjust_max_heap(L,i,0)

归并排序
算法思想：

归并排序是建立在归并操作上的一种有效的排序算法，该算法是采用分治法的一个典型的应用。它的基本操作是：将已有的子序列合并，达到完全有序的序列；即先使每个子序列有序，再使子序列段间有序。
归并排序其实要做两件事：
分解----将序列每次折半拆分
合并----将划分后的序列段两两排序合并
因此，归并排序实际上就是两个操作，拆分+合并

如何合并？

L[first…mid]为第一段，L[mid+1…last]为第二段，并且两端已经有序，现在我们要将两端合成达到L[first…last]并且也有序。
首先依次从第一段与第二段中取出元素比较，将较小的元素赋值给temp[]
重复执行上一步，当某一段赋值结束，则将另一段剩下的元素赋值给temp[]
此时将temp[]中的元素复制给L[]，则得到的L[first…last]有序
如何分解？
在这里，我们采用递归的方法，首先将待排序列分成A,B两组；然后重复对A、B序列
分组；直到分组后组内只有一个元素，此时我们认为组内所有元素有序，则分组结束。
代码实现

# 归并排序
#这是合并的函数
# 将序列L[first...mid]与序列L[mid+1...last]进行合并
def mergearray(L,first,mid,last,temp):
#对i,j,k分别进行赋值
    i,j,k = first,mid+1,0
#当左右两边都有数时进行比较，取较小的数
    while (i <= mid) and (j <= last):
        if L[i] <= L[j]:
            temp[k] = L[i]
            i = i+1
            k = k+1
        else:
            temp[k] = L[j]
            j = j+1
            k = k+1
#如果左边序列还有数
    while (i <= mid):
        temp[k] = L[i]
        i = i+1
        k = k+1
#如果右边序列还有数
    while (j <= last):
        temp[k] = L[j]
        j = j+1
        k = k+1
#将temp当中该段有序元素赋值给L待排序列使之部分有序
    for x in range(0,k):
        L[first+x] = temp[x]
# 这是分组的函数
def merge_sort(L,first,last,temp):
    if first < last:
        mid = (int)((first + last) / 2)
#使左边序列有序
        merge_sort(L,first,mid,temp)
#使右边序列有序
        merge_sort(L,mid+1,last,temp)
#将两个有序序列合并
        mergearray(L,first,mid,last,temp)
# 归并排序的函数
def merge_sort_array(L):
#声明一个长度为len(L)的空列表
    temp = len(L)*[None]
#调用归并排序
    merge_sort(L,0,len(L)-1,temp)

用了很多功夫抄了别人的代码过来，一定要好好学习，天天向上!!!

#include
#include
#include
#include
#include
#include
#include
#include
#include

#define OK 1
#define ERROR 0
#define TRUE 1
#define FALSE 0
#define MAXSIZE 10002
typedef int Status;
typedef int ElemType;
char s[12][60],f[12][30];
typedef struct {
	ElemType *r;
	int length;
	int cmpcount,excount;
} SqList;
Status printSqList(SqList *L);//遍历输出表
Status ListCreate(SqList *&L) {
	int i;
	int value;
	printf("---<表的创建>---\n");
	L->cmpcount=L->excount=0;
	do {
		printf("请输入表的元素数:");
		scanf("%d",&value);
		L->r = (ElemType *)malloc((value+1)*sizeof(ElemType));
		srand(time(NULL));
		if(value>0&&value<MAXSIZE) {
			printf("\n");
			for(i=1; i<=value; i++,L->length++) {
				L->r[i]=rand()%value;
			}
		} else {
			printf("\n");
			printf("输入的数量不合法或超出!\n");
			printf("请重新输入:");
		}
	} while(value<0&&value>MAXSIZE);
	return OK;
}
void swap(SqList *L,int i,int j) {
	int temp=L->r[i];
	L->r[i]=L->r[j];
	L->r[j]=temp;
}

//简单选择排序
void SelectSort(SqList *L) {
	L->cmpcount=L->excount=0;
	int i,j,min;
	printf("\n");
	printf("简单选择排序：\n");

	for(i=1; i<L->length; i++) {
		min=i;
		for(j=i+1; j<=L->length; j++) {
			L->cmpcount++;
			if(L->r[min]>L->r[j]) {
				min=j;
			}
		}
		if(i!=min) {
			swap(L,i,min);
			L->excount++;
			printSqList(L);
		}
	}
	printf("比较次数是：%d\t交换次数是：%d\n",L->cmpcount,L->excount);
	sprintf(s[6],"简单选择排序\t\t%-10d\t%d\t",L->cmpcount,L->excount);
}
//简单选择排序

//直接插入排序
void InsertSort(SqList *L) {
	L->cmpcount=L->excount=0;
	int i,j;
	printf("\n");
	printf("直接插入排序：\n");

	for(i=2; i<=L->length; i++) {
		L->cmpcount++;
		if(L->r[i]<L->r[i-1]) {
			L->r[0]=L->r[i];
			for(j=i-1; L->r[j]>L->r[0]; j--) {
				L->cmpcount++;
				L->r[j+1]=L->r[j];
			}
			L->r[j+1]=L->r[0];
			L->excount++;
			printSqList(L);
		}
	}
	printf("比较次数是：%d\t交换次数是：%d\n",L->cmpcount,L->excount);
	sprintf(s[1],"直接插入排序\t\t%d\t\t%d\t",L->cmpcount,L->excount);
}
//直接插入排序

//冒泡排序改进算法
void BubbleSort(SqList *L) {
	L->cmpcount=L->excount=0;
	int i,j;
	ElemType Flag=TRUE;
	printf("\n");
	printf("冒泡算法排序：\n");

	for(i=1; i<L->length&&Flag; i++) {
		Flag=FALSE;
		for(j=L->length-1; j>=i; j--) {
			L->cmpcount++;
			if(L->r[j]>L->r[j+1]) {
				swap(L,j,j+1);
				L->excount++;
				printSqList(L);
				Flag=TRUE;
			}
		}
	}
	printf("比较次数是：%d\t交换次数是：%d\n",L->cmpcount,L->excount);
	sprintf(s[4],"冒泡排序算法\t\t%-10d\t%d\t",L->cmpcount,L->excount);
}
//冒泡排序改进算法

//希尔排序
void ShellSort(SqList *L) {
	L->cmpcount=L->excount=0;
	int i,j;
	printf("\n");
	printf("希尔算法排序：\n");

	int increment=L->length;
	do {
		increment=(int)increment/2;
		for(i=increment+1; i<=L->length; i++) {
			L->cmpcount++;
			if(L->r[i]<L->r[i-increment]) {
				L->r[0]=L->r[i];
				for(j=i-increment; j>0&&L->r[0]<L->r[j]; j-=increment) {
					L->r[j+increment]=L->r[j];
				}
				L->r[j+increment]=L->r[0];
				L->excount++;
				printSqList(L);
			}
		}
	} while(increment>1);
	printf("比较次数是：%d\t交换次数是：%d\n",L->cmpcount,L->excount);
	sprintf(s[3],"希尔排序算法\t\t%d\t\t%d\t",L->cmpcount,L->excount);
}
//希尔排序

//折半插入排序
void BInsertSort(SqList *L) {
	L->cmpcount=L->excount=0;
	int low,high,m;
	printf("\n");
	printf("折半插入排序：\n");

	for(int i=2; i<=L->length; i++) {
		L->r[0]=L->r[i];
		low=1;
		high=i-1;
		while(low<=high) {
			m=(low+high)/2;
			L->cmpcount++;
			if(L->r[0]<L->r[m]) {
				high=m-1;
			} else {
				low=m+1;
			}
		}
		for(int j=i-1; j>=high+1; --j) {
			L->r[j+1]=L->r[j];
		}
		L->r[high+1]=L->r[0];
		L->excount++;
		printSqList(L);
	}
	printf("比较次数是：%d\t交换次数是：%d\n",L->cmpcount,L->excount);
	sprintf(s[2],"折半排序算法\t\t%d\t\t%d\t",L->cmpcount,L->excount);
}
//折半插入排序

//快速排序算法
int partition(SqList *L,  int low, int high) {
	int key = L->r[low];
	while( low < high ) {
		while(low < high && L->r[high] >= key) {
			L->cmpcount++;
			high--;
		}
		L->r[low] = L->r[high];
		while(low < high && L->r[low] <= key) {
			L->cmpcount++;
			low++;
		}
		L->r[high] = L->r[low];
	}
	L->r[low] = key;
	return low;
}
void quicksort(SqList *L, int low, int high) {
	if(low >= high) return;
	int keypos = partition(L, low, high);
	L->excount++;
	printSqList(L);
	quicksort(L, low, keypos-1);
	quicksort(L, keypos+1, high);
}
void QuickSort(SqList *L) {
	L->cmpcount=L->excount=0;
	printf("\n");
	printf("快速排序算法：\n");
	quicksort(L,1,L->length);
	printf("比较次数是：%d\t交换次数是：%d\n",L->cmpcount,L->excount);
	sprintf(s[5],"快速排序算法\t\t%d\t\t%d\t",L->cmpcount,L->excount);
}
//快速排序算法

//堆排序
void HeapAdjust(SqList *L,int s,int m) {
	int temp,j;
	temp=L->r[s];
	for(j=2*s; j<=m; j*=2) {
		L->cmpcount++;
		if(j<m&&L->r[j]<L->r[j+1]) {
			++j;
		}
		L->cmpcount++;
		if(temp>=L->r[j]) {
			break;
		}
		L->r[s]=L->r[j];
		s=j;
	}
	L->r[s]=temp;
}
void HeapSort(SqList *L) {
	L->cmpcount=L->excount=0;
	int i;
	printf("\n");
	printf("堆排序：\n");
	for(i=L->length/2; i>0; i--) {
		HeapAdjust(L,i,L->length);
	}
	for(i=L->length; i>1; i--) {
		swap(L,1,i);
		L->excount++;
		printSqList(L);
		HeapAdjust(L,1,i-1);
	}
	printf("比较次数是：%d\t交换次数是：%d\n",L->cmpcount,L->excount);
	sprintf(s[7],"堆排序算法\t\t%d\t\t%d\t",L->cmpcount,L->excount);
}
//堆排序

//归并排序算法
void Merge(int SR[],int TR[],int i,int m,int n,SqList *L) {
	int j,k,l;
	for(j=m+1,k=i; i<=m&&j<=n; k++) {
		L->cmpcount++;
		if(SR[i]<SR[j]) {
			TR[k]=SR[i++];
		} else {
			TR[k]=SR[j++];
		}
	}
	L->cmpcount++;
	if(i<=m) {
		for(l=0; l<=m-i; l++) {
			TR[k+l]=SR[i+l];
		}
	}
	L->cmpcount++;
	if(j<=n) {
		for(l=0; l<=n-j; l++) {
			TR[k+l]=SR[j+l];
		}
	}
	L->excount++;
	printSqList(L);
}
void MSort(int SR[],int TR1[],int first,int last,SqList *L) {
	int mid;
	int TR2[MAXSIZE+1];
	L->cmpcount++;
	if(first==last) {
		TR1[first]=SR[first];
	} else {
		mid=(last+first)/2;
		MSort(SR,TR2,first,mid,L);
		MSort(SR,TR2,mid+1,last,L);
		Merge(TR2,TR1,first,mid,last,L);
	}
}
void MergeSort(SqList *L) {
	L->cmpcount=L->excount=0;
	printf("\n");
	printf("归并算法排序(递归)：\n");
	MSort(L->r,L->r,1,L->length,L);
	printf("比较次数是：%d\t交换次数是：%d\n",L->cmpcount,L->excount);
	sprintf(s[1],"归并排序算法算法\t\t%d\t\t%d\t",L->cmpcount,L->excount);
}
//归并排序算法

//非递归归并算法
void MergePass(int SR[],int TR[],int s,int n,SqList *L) {
	int i=1;
	int j;
	while(i<=n-2*s+1) {
		L->cmpcount++;
		Merge(SR,TR,i,i+s-1,i+2*s-1,L);
		i=i+2*s;
	}
	L->cmpcount++;
	if(i<n-s+1) {
		Merge(SR,TR,i,i+s-1,n,L);
	} else {
		for(j=i; j<=n; j++) {
			TR[j]=SR[j];
		}
		L->excount++;
		printSqList(L);
	}
}
void MergeSort2(SqList *L) {
	L->cmpcount=L->excount=0;
	int *TEMP=(int *)malloc(L->length*sizeof(int));
	int k=1;
	printf("\n");
	printf("归并算法排序(非递归)：\n");

	while(k<L->length) {
		MergePass(L->r,TEMP,k,L->length,L);
		k=2*k;
		MergePass(TEMP,L->r,k,L->length,L);
		k=2*k;
	}
	printf("比较次数是：%d\t交换次数是：%d\n",L->cmpcount,L->excount);
	sprintf(s[8],"归并排序算法\t\t%d\t\t%d\t",L->cmpcount,L->excount);
}
//非递归归并算法

Status printSqList(SqList *L) {
	int i,flag;
	if(L->excount==0||flag==L->excount) {
		return ERROR;
	} else {
//		return 0;
		printf("第%d次交换后",L->excount);
	}
	printf("遍历表结果：\n\t");
	for(i=1; i<=L->length; i++) {
		printf("%d\t",L->r[i]);
	}
	printf("\n");
	return OK;
}
Status DataSave(SqList *L,int *a) {
	int i;
	for(i=1; i<=L->length; i++) {
		a[i]=L->r[i];
	}
	return OK;
}
Status SqListMenu() {
//	printf("\n");
//	printf(" _______>>>排序算法<<<______\n");
//	printf("|                           |\n");
//	printf("|    1.直接插入排序         |\n");
//	printf("|    2.折半插入排序         |\n");
//	printf("|    3.希尔算法排序         |\n");
//	printf("|    4.冒泡算法排序         |\n");
//	printf("|    5.快速算法排序         |\n");
//	printf("|    6.简单选择排序         |\n");
//	printf("|    7.堆排序               |\n");
//	printf("|    8.归并算法排序         |\n");
//	printf("|    0.退出                 |\n");
//	printf("|___________________________|\n");
	int value;
	printf("请选择你要执行的排序操作:");
	do {
		scanf("%d",&value);
		if(value>0&&value<12) {
			return value;
		} else {
			printf("你输入的操作有误，请重新输入:");
		}
	} while(value>0&&value<12);
}
int main() {
	SqList *L;
	int i;
	int value;
	int array[MAXSIZE];
	srand(GetTickCount());
	ListCreate(L);
	DataSave(L,array);
	do {
		printf("\n");
		for(i=1; i<=L->length; i++) {
			L->r[i]=array[i];
		}
		value=SqListMenu();

		if(value==1) {
			clock_t start = clock();
			printSqList(L);
			InsertSort(L);
			printSqList(L);
			double end = (double)(clock() - start);
			printf("|    1.直接插入排序         |\n");
			printf("用时%.0fms\n",(double)end);
			sprintf(f[value],"\t%.0fms\n",(double)end);
		} else if(value==2) {
			clock_t start = clock();
//		printSqList(L);
			BInsertSort(L);
			printSqList(L);
			double end = (double)(clock() - start);
			printf("|    2.折半插入排序         |\n");
			printf("用时%.0fms\n",(double)end);
			sprintf(f[value],"\t%.0fms\n",(double)end);
		} else if(value==3) {
			clock_t start = clock();
//		printSqList(L);
			ShellSort(L);
			printSqList(L);
			double end = (double)(clock() - start);
			printf("|    3.希尔算法排序         |\n");
			printf("用时%.0fms\n",(double)end);
			sprintf(f[value],"\t%.0fms\n",(double)end);
		} else if(value==4) {
			clock_t start = clock();
//		printSqList(L);
			BubbleSort(L);
			printSqList(L);
			double end = (double)(clock() - start);
			printf("|    4.冒泡算法排序         |\n");
			printf("用时%.0fms\n",(double)end);
			sprintf(f[value],"\t%.0fms\n",(double)end);
		} else if(value==5) {
			clock_t start = clock();
//		printSqList(L);
			QuickSort(L);
			printSqList(L);
			double end = (double)(clock() - start);
			printf("|    5.快速算法排序         |\n");
			printf("用时%.0fms\n",(double)end);
			sprintf(f[value],"\t%.0fms\n",(double)end);
		} else if(value==6) {
			clock_t start = clock();
//		printSqList(L);
			SelectSort(L);
			printSqList(L);
			double end = (double)(clock() - start);
			printf("|    6.简单选择排序         |\n");
			printf("用时%.0fms\n",(double)end);
			sprintf(f[value],"\t%.0fms\n",(double)end);
		} else if(value==7) {
			clock_t start = clock();
//		printSqList(L);
			HeapSort(L);
			printSqList(L);
			double end = (double)(clock() - start);
			printf("|    7.堆排序               |\n");
			printf("用时%.0fms\n",(double)end);
			sprintf(f[value],"\t%.0fms\n",(double)end);
		} else if(value==8) {
			clock_t start = clock();
//		printSqList(L);
			MergeSort2(L);
			printSqList(L);
			double end = (double)(clock() - start);
			printf("|    8.归并算法排序         |\n");
			printf("用时%.0fms\n",(double)end);
			sprintf(f[value],"\t%.0fms\n",(double)end);
		} else if(value==9) {
			printf("样本数为%d\n八大排序算法的结果：\t总的比较次数\t交换次数\t时间\n",L->length);
			for(i=1; i<=8; i++) {
				printf("%s%s\n",s[i],f[i]);
			}
//			printf("退出!\n");
		} else {
			printf("\n");
			printf("错误输入!重新输入！\n");
		}
	} while(value!=0);
	return 0;
}
/*测试样例1
50
1
2
3
4
5
6
7
8
9
*/