图论——AOV网络及拓扑排序

引入

有向无环图（DAG）

如果一个有向图不存在环，也就是任意结点都无法通过一些有向边回到自身，那么称这个有向图为有向无环图

AOV 网络

在有向图中，用顶点表示活动，用有向边$<V_i,V_j>$表示活动 $i$ 是活动 $j$ 的必须条件。这种有向图称为用顶点表示活动的网络(Active on vertices)，简称AOV网络。

在AOV网络中，如果活动$V_i$必须在$V_j$之前进行，则存在有向边$<V_i,V_j>$，并称$V_i$是$V_j$的直接前驱，$V_j$是$V_i$的直接后继。这种前驱与后继的关系具有传递性 （$V_i$ 对于 $V_j$ 的后继依然具有前导性）和反自反性（AOV网络中不能出现回路，即有向环）。因此，对于给定的AOV网络，必须先判断它是否存在有向环。

拓扑排序

检测有向环可以通过对AOV网络进行拓扑排序

用计算机专业的几门课程的学习次序来描述拓扑关系 ，显然对于一门课来说，必须先学习它的先导课程才能更好地学习这门课程，比如学数据结构必须先学习C语言和离散数学，而且先导课程中不能有环，否则没有尽头了

而且还可以发现，如果两门课程之间没有直接或间接的先导关系，那么这两门课的学习先后是任意的（比如“C语言”和“离散数学”的学习顺序就是任意的），于是上述课程就可以排成一个水平展开的先后顺序，如下图

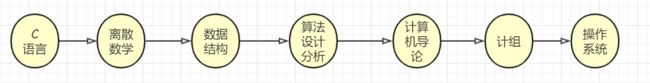
拓扑排序的结果不唯一，比如“C语言”和“离散数学”就可以换下顺序，又或者把“计算机导论”向前放在任何一个位置都可以。总结一下就是，如果某一门课没有先导课程或是所有的先导课程都已经学习完毕，那么这门课就可以学习了。如果同时有多门这样的课，它们的学习顺序任意。

如果拓扑排序能够将AOV网络的所有顶点都排入一个拓扑有序的序列中，则说明该AOV网络中没有有向环。

算法描述

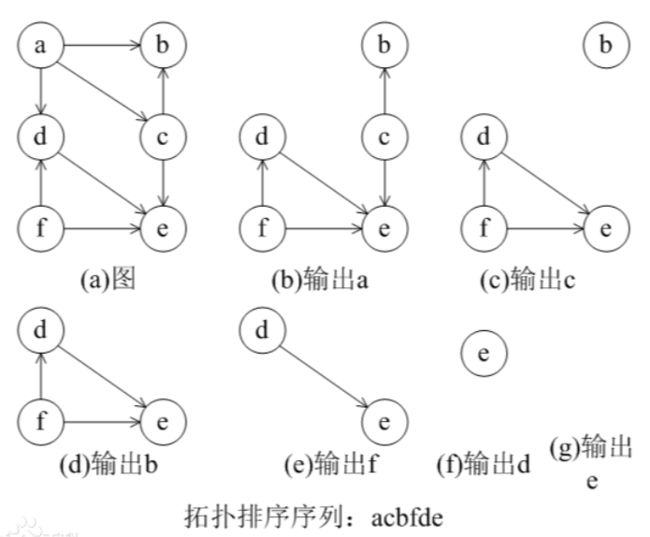
假设A是一个入度为0的结点，也就表示A结点没有前驱结点，可以“直接做”，把A完成后，对于A的所有后继结点来说，前驱结点就完成了一个，它们的入度数$-1$ ，再选择一个入度为0的点重复。

对于一个有向无环图

（1）统计所有节点的入度，对于入度为0的节点就可以分离出来，然后把这个节点指向所有的节点的入度$-1$。

（2）重复（1），直到所有的节点都被分离出来，拓扑排序结束。

（3）如果最后不存在入度为0的节点，那就说明有环，无解。

时间复杂度

如果AOV网络有n个顶点，e条边，在拓扑排序的过程中，搜索入度为零的顶点所需的时间是O(n)。在正常情况下，每个顶点进一次栈，出一次栈，所需时间O(n)。每个顶点入度减1的运算共执行了e次。所以总的时间复杂为O(n+e)。

因为拓扑排序的结果不唯一，所以题目一般会要求按某种顺序输出，就需要使用优先级队列，这里采取了最小字典序输出。

vector<int>head[505], ans;
int n, m, in[505];//入度序列

void topologicalSorting() {
        cin >> n >> m;
    for (int i = 0; i < m; i++) {
        int c1, c2;
        scanf("%d%d", &c1, &c2);
        head[c1].push_back(c2);
        in[c2]++;
    }
    priority_queue<int, vector<int>, greater<int>>q;
    for (int i = 1; i <= n; i++) {
        if (!in[i]) {
            q.push(i);
        }
    }
    while (!q.empty() && ans.size() < n) {
        int v = q.top(); q.pop();
        ans.push_back(v);
        for (int i = 0; i < head[v].size(); i++) {
            in[head[v][i]]--;
            if (!in[head[v][i]])
                q.push(head[v][i]);
        }
    }

    if (ans.size() == n) {
        //找到拓扑排序序列
    }
    else {
        //图中有环
    }
}    

练习

模板题

题目链接：http://acm.hdu.edu.cn/showproblem.php?pid=1285

#include 
#include 
#include 
#include 
#include 
using namespace std;

vector<int>head[505];
int in[505];

int main() {
    int n, m;
    while (cin >> n >> m) {
        priority_queue<int, vector<int>, greater<int>>q;
        vector<int>ans;
        for (int i = 0; i < m; i++) {
            int c1, c2;
            scanf("%d%d", &c1, &c2);
            head[c1].push_back(c2);
            in[c2]++;
        }
        for (int i = 1; i <= n; i++) {
            if (!in[i]) {
                q.push(i);
            }
        }

        while (!q.empty()) {
            int temp = q.top(); q.pop();
            ans.push_back(temp);
            for (int i = 0; i < head[temp].size(); i++) {
                in[head[temp][i]]--;
                if (!in[head[temp][i]])
                    q.push(head[temp][i]);
            }
        }
        if (ans.size() == n) {
            for (int i = 0; i < n; i++) {
                head[i + 1].clear();
                cout << ans[i];
                if (i != n - 1)cout << ' ';
            }
            cout << endl;
        }
        q.emplace();
        ans.clear();
        
    }
    
    return 0;
}

反向拓扑排序

题目链接：http://acm.hdu.edu.cn/showproblem.php?pid=4857

题意：$n$个结点，给定$m$个拓扑关系$(a,b)$表示$a$必须排在$b$前面，在满足$m$个拓扑关系关系的同时，使得小的结点尽可能的排在前面。
乍一看好像直接拓扑排序就行，但是看一个例子：
$$\begin{gathered} 6\to 3\to 1 \\ 5\to 4\to 2 \end{gathered}$$
直接拓扑排序的结果是：$542631$ ，是错误的，因为我们可以把1号安排到更前面的位置 即：$631542$（正确答案）。所以直接拓扑排序是不行的，为什么会出现这样的状况，对于多个拓扑关系，我们本来的策略是优先删除首结点较小的拓扑序列（比如5号结点比6号结点小，我们先删除了5号结点），但我们希望的是优先删除尾结点较小的拓扑序列（比如1号结点比2号结点小，应当先删除1号结点）。问题找到了，我们可以尝试一下逆向思维，即我们先考虑哪些点应该靠后释放，就是把原来的拓扑关系反过来

$$\begin{gathered} 1\to 3\to 6 \\ 2\to 4\to 5 \end{gathered}$$
这样我们按照优先删除首结点较大的拓扑序列得到的结果是$245136$，好像还是不太对，把它逆序输出就对啦！

#include 
#include 
#include 
#include 
#include 
using namespace std;

vector<int>head[30005];
int in[30005];

int main() {
    int T;
    cin >> T;
    while (T--) {
        int n, m;
        cin >> n >> m;
        priority_queue<int>q;
        vector<int>ans;
        for (int i = 0; i < m; i++) {
            int c1, c2;
            scanf("%d%d", &c1, &c2);
            /*head[c1].push_back(c2);
            in[c2]++;*/
            head[c2].push_back(c1);
            in[c1]++;
        }
        for (int i = 1; i <= n; i++) {
            if (!in[i]) {
                q.push(i);
            }
        }

        while (!q.empty()) {
            int temp = q.top(); q.pop();
            ans.push_back(temp);
            for (int i = 0; i < head[temp].size(); i++) {
                in[head[temp][i]]--;
                if (!in[head[temp][i]])
                    q.push(head[temp][i]);
            }
        }

        if (ans.size() == n) {
            for (int i = n - 1; i >= 0 ; i--) {
                head[i + 1].clear();
                cout << ans[i];
                if (i != 0)cout << ' ';
            }
            cout << endl;
        }
        q.emplace();
        ans.clear();
    }
    return 0;
}