元旦特辑：Note6---选择排序

---

目录

前言❌

1. 基本思想⚠️

2. 直接选择排序

2.1 思路分析✳️

2.2 代码实现❎

2.2.1 sort.h

2.2.2 sort.c

2.2.3 test.c

2.3 问题解决❇️

2.3.1 sort.c修改

2.4 特性总结✅

3. 堆排序

3.1 代码实现

3.2 特性总结

4. 选择排序和插入排序性能对比️

后语⭕️

---

前言❌

Hey young fella! Welcome to my channel!  前面一篇博客，我们学习了插入排序的2种实现方法。今天也是类似的，今天我们将一起学习选择排序的2种实现：直接选择排序+堆排序。

---

1. 基本思想⚠️

每一次从待排序的数据元素中选出最小（或最大）的一个元素，存放在序列的起始位置，直到全部待排序的数据元素排完 。

2. 直接选择排序

2.1 思路分析✳️

目标：实现升序

思路：暴力选取

把数据遍历一遍，依次将最大（小）的，次大（小）的，次次大（小）的放到对应的前三位（每次选取都要遍历一遍），然后重复上述步骤，直到最后就剩下1个数据

当然，我们也可以优化一下：
第一次遍历选出最大的和最小的放到头和尾的位置
第二次遍历选出次大的和次小的放到头+1和尾-1的位置
......

2.2 代码实现❎

2.2.1 sort.h

#pragma once
#include
#include

//打印
void PrintArray(int* a, int n);
//直接插入排序
void SelectSort(int* a, int n);

---

2.2.2 sort.c

#include"sort.h"
//打印
void PrintArray(int* a, int n)
{
	for (int i = 0; i < n; i++)
		printf("%d ", a[i]);
	printf("\n");
}

//交换
void Swap(int* p1, int* p2)
{
	int tmp = *p1;
	*p1 = *p2;
	*p2 = tmp;
}

//直接插入排序
void SelectSort(int* a, int n)
{
	int begin = 0, end = n - 1,i;
	while (begin < end)
	{
		int min = begin, max = begin;
		//一次排2个min和max
		for (i = begin + 1; i <=end; i++)
		{
			if (a[i] < a[min])
				min = i;
			if (a[i] > a[max])
				max = i;
		}
		Swap(&a[begin], &a[min]);
		Swap(&a[end], &a[max]);

		begin++;
		end--;
	}
}

---

2.2.3 test.c

#include"sort.h"
//直接插入排序
void testSelectSort()
{
    int a[] = { 3,2,6,8,9,7,5,10,1,4 };
    int n = sizeof(a) / sizeof(int);
    SelectSort(a, n);
    PrintArray(a, n);
}
int main()
{
    testSelectSort();
	return 0;
}

---

---

2.3 问题解决❇️

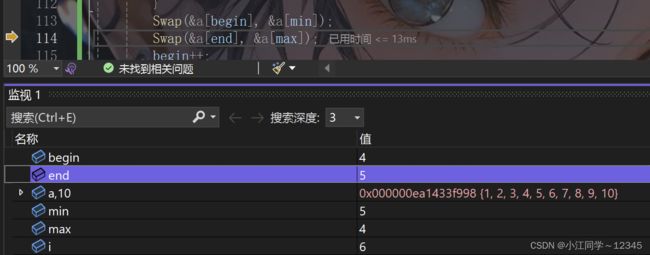
我们发现，排序结果怎么有点不对呢？5，6的顺序怎么反了？

通过调试，我们发现最后一次排序的时候，交换那里出现了问题：max和begin位置重合了
min和begin交换完之后，排序是正确的；但是，end和max又交换了一次，导致顺序出错

所以，我们需要判断一下max和begin是否重合，重合了就不交换max和end；没重合就交换

2.3.1 sort.c修改

//交换
void Swap(int* p1, int* p2)
{
	int tmp = *p1;
	*p1 = *p2;
	*p2 = tmp;
}

//直接插入排序
void SelectSort(int* a, int n)
{
	int begin = 0, end = n - 1,i;
	while (begin < end)
	{
		int min = begin, max = begin;
		//一次排2个min和max
		for (i = begin + 1; i <=end; i++)
		{
			if (a[i] < a[min])
				min = i;
			if (a[i] > a[max])
				max = i;
		}
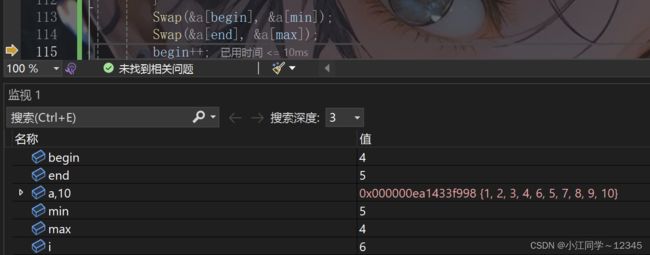
		Swap(&a[begin], &a[min]);
		//判断max和begin位置是否重合
		if (begin != max)
			Swap(&a[end], &a[max]);

		begin++;
		end--;
	}
}

---

2.4 特性总结✅

1. 直接选择排序思考非常好理解，但是效率不是很好（后面会性能对比）。实际中很少使用

2. 时间复杂度：O(N^2)

最好的情况下也是O(N^2)：接近有序or有序
但是，我们不知道有序还是无序，还是需要遍历并且选出max和min交换--->O(N^2)

3. 空间复杂度：O(1)

4. 稳定性：不稳定

3. 堆排序

之前的博客我们详细介绍过了堆排序的实现，这里就不再详述了，直接上代码。需要详述的小伙伴可以点击下方链接：

Note3---初阶二叉树～～-CSDN博客文章浏览阅读1.1k次，点赞59次，收藏51次。这篇博客，我们一起来了解并学习数据结构中的初阶的二叉树的概念和性质；以及堆和堆堆应用二叉树的知识点和内容比较多，友友们一定要有耐心看完（跳到自己需要的部分也是OK的）。https://blog.csdn.net/2301_79184587/article/details/135033457

3.1 代码实现

3.1.1 sort.h

//堆排序
void HeapSort(int* a, int n);

---

3.1.2 sort.c

//向下调整
void AdjustDown(int* a, int size, int parent)
{
	int child = parent * 2 + 1;
	while (child < size)
	{
		if (a[child + 1] > a[child] && child + 1 < size)
			child++;
		if (a[child] > a[parent])
		{
			Swap(&a[child], &a[parent]);
			parent = child;
			child = 2 * parent + 1;
		}
		else
			break;
	}
}

//堆排序
void HeapSort(int* a, int n)
{
	//建大堆
	for (int i = (n - 1 - 1) / 2; i >= 0; i--)
	{
		AdjustDown(a, n, i);
	}
	int end = n - 1;
	while (end > 0)
	{
		Swap(&a[0], &a[end]);
		AdjustDown(a, end, 0);
		end--;
	}
}

---

3.1.3 test.c

//堆排序
void testHeapSort()
{
    int a[] = { 3,2,6,8,9,7,5,10,1,4 };
    int n = sizeof(a) / sizeof(int);
    HeapSort(a, n);
    PrintArray(a, n);
}
int main()
{
    testHeapSort();
	return 0;
}

---

3.2 特性总结

1. 堆排序使用堆来选数，效率就高了很多。

2. 时间复杂度：O(N*logN)

3. 空间复杂度：O(1)

4. 稳定性：不稳定

4. 选择排序和插入排序性能对比️

// 测试排序的性能对比
void TestOP()
{
	srand(time(0));
	const int N = 100000;
	int* a1 = (int*)malloc(sizeof(int) * N);
	int* a2 = (int*)malloc(sizeof(int) * N);
	int* a3 = (int*)malloc(sizeof(int) * N);
	int* a4 = (int*)malloc(sizeof(int) * N);
	int* a5 = (int*)malloc(sizeof(int) * N);
	int* a6 = (int*)malloc(sizeof(int) * N);
	for (int i = 0; i < N; ++i)
	{
		a1[i] = rand()+i;
		a2[i] = a1[i];
		a3[i] = a1[i];
		a4[i] = a1[i];
		a5[i] = a1[i];
		a6[i] = a1[i];
    }
    int begin1 = clock();
    InsertSort(a1, N);
    int end1 = clock();
    int begin2 = clock();
    ShellSort(a2, N);
    int end2 = clock();
    int begin3 = clock();
    SelectSort(a3, N);
    int end3 = clock();
    int begin4 = clock();
    HeapSort(a4, N);
    int end4 = clock();
    int begin5 = clock();
    //QuickSort(a5, 0, N - 1);
    int end5 = clock();
    int begin6 = clock();
    //MergeSort(a6, N);
    int end6 = clock();
    printf("InsertSort:%d\n", end1 - begin1);
    printf("ShellSort:%d\n", end2 - begin2);
    printf("SelectSort:%d\n", end3 - begin3);
    printf("HeapSort:%d\n", end4 - begin4);
    printf("QuickSort:%d\n", end5 - begin5);
    printf("MergeSort:%d\n", end6 - begin6);
    free(a1);
    free(a2);
    free(a3);
    free(a4);
    free(a5);
    free(a6);
}
int main()
{
    TestOP();
	return 0;
}

---

---

我们发现，10万数据的时候，堆排序和希尔排序相差不大；100万个数据时，希尔排序的时间复杂度远超于堆排序的时间复杂度

后语⭕️

今天，我们学习了选择排序的实现和性能对比以及他们的特性总结。希望小伙伴们自己也可以练习一下，加强记忆和理解。

下一篇博客，我们将一起学习交换排序的相关知识点！请大家多多期待

本次的分享到这里就结束了！！！

PS：小江目前只是个新手小白。欢迎大家在评论区讨论哦！有问题也可以讨论的！期待大家的互动！！！

公主/王子殿下，请给我点赞+收藏⭐️+关注➕（这对我真的很重要！！！）

---