Codeforces Round 917 (Div. 2)(A~D)
A - Least Product
Solution
观察发现,对于 a i < 0 a_i<0 ai<0,操作后 a i a_i ai 不会变得更小, a i > 0 a_i>0 ai>0,操作后 a i a_i ai 不会变得更大。所以,当 ∏ i = 1 n a i ≥ 0 \prod_{i=1}^na_i\ge 0 ∏i=1nai≥0 时,不可能变得更大; ∏ i = 1 n a i < 0 \prod_{i=1}^na_i<0 ∏i=1nai<0 时,最优即为将 1 1 1 个数变为 0 0 0,这样可以保证操作次数最少。
A t t e n t i o n : \mathrm{Attention: } Attention: 不能将所有数相乘,因为乘积会超级大(最多也就 9 100 9^{100} 9100 位吧),所以需要记录负数的个数和是否有 0 0 0,来进行判断
Code
#include B - Erase First or Second Letter
Solution
暴力出奇迹,打表出省一
Way 1(打表找规律)
打表也需要技巧:
第 1 1 1 步:写出暴力代码
S = ' ' + S;
int Cnt = 0;
map<string, int> Vis;
queue<string> Q;
Q.push(S);
while (Q.size())
{
string S2 = Q.front();
Q.pop();
if (Vis.count(S2) || S2.size() == 1) continue;
Vis[S2] = 1;
Cnt ++;
string S3 = S2;
S3.erase(1, 1);
Q.push(S3);
S3 = S2;
if (S3.size() > 2) S3.erase(2, 1);
Q.push(S3);
}
cout << Cnt << endl;
第 2 2 2 步:观察如果整个序列无重复元素的时候的值: ( 1 + n ) ⋅ n 2 \frac{(1+n)\cdot n}{2} 2(1+n)⋅n
第 3 3 3 步:观察对于一个无重复元素的序列,加入重复元素的变化(难点)
通过,不断的尝试,可以发现规律:对于字母 a a a,如有重复数字,则为 n n n − - − ∑ i = 1 n i − P a − 1 [ S i = a ] \sum_{i=1}^n i-P_a-1[S_i=a] ∑i=1ni−Pa−1[Si=a],其中 P a P_a Pa 为字母 a a a 第一次出现的下标, [ ] [] [] 表示条件。
最后,通过容斥原理得,最终值为 ( 1 + n ) ⋅ n 2 − ∑ i = a z ∑ j = 1 n j − P i − 1 [ S j = i ] \frac{(1+n)\cdot n}{2}-\sum_{i=a}^z\sum_{j=1}^n j-P_i-1[S_j=i] 2(1+n)⋅n−∑i=az∑j=1nj−Pi−1[Sj=i]
由于 n n n 比较大,故通过前缀和优化即可。
Code 1
#include 方法很土,但是如果大家想要用总数 − - − 重复的数,那么可以借鉴一下,不过我不会证(*/ω\*)
Way 2(思维)
考虑对于 1 1 1 个字符串:bcdaaaabcdaaaa
- D e l \mathrm{Del} Del
b, D e l \mathrm{Del} Delc, … \dots … 共 14 14 14 种 - 保留
b, D e l \mathrm{Del} Delc, D e l \mathrm{Del} Deld, … \dots … 共 13 13 13 种 - D e l \mathrm{Del} Del
b,保留c, D e l \mathrm{Del} Deld, D e l \mathrm{Del} Dela, … \dots … 共 12 12 12 种 - D e l \mathrm{Del} Del
b, D e l \mathrm{Del} Delc,保留d, D e l \mathrm{Del} Dela, D e l \mathrm{Del} Dela, … \dots … 共 11 11 11 种
大家可以发现此时相加已经是 50 50 50 了(样例 4 4 4)
这说明,只有当该字符为第 1 1 1 次出现时,才会对答案产生 n − i + 1 n-i+1 n−i+1 的贡献。
P r o o f : \mathrm{Proof:} Proof: 若总共操作 k k k 次删第 1 1 1 个字符得到一个非第一次出现的字符 x x x(位置为 i i i),那么这个操作等价于不断地删第 1 1 1 个字符直到当前字符串的第 1 1 1 个字符是第一次出现的字符 x x x,然后不断地删第 2 2 2 个字符直到删掉第 i i i 字符。此时前后 2 2 2 种操作得到的字符串是一样的,所以 i i i 能得到的所有字符串,都能由第一次出现字符 x x x 的位置通过操作来得到。故,会计算重复。
综上所述,最终的值为: ∑ i = a z n − P i + 1 \sum_{i=a}^z n-P_i+1 ∑i=azn−Pi+1
其中, P i P_i Pi 为字符 i i i 出现的下标。
Code 2
#include C - Watering an Array
Solution
性质:当 a a a 数组全部第 1 1 1 次变为 0 0 0 后,之后一定是加 1 1 1 次,统计 1 1 1 次。
P r o o f : \mathrm{Proof:} Proof: 因为对于全 0 0 0 的数组,无论怎么加,一定都不会超过 1 1 1。故倒不如加 1 1 1 次,位置 1 1 1 一定满足,再统计即可。
所以,我们只需要考虑初始的 a a a 数组加多少次,才能价值最高。变为全 0 0 0 数组之后的价值就是 ⌊ 剩余的天数 2 ⌋ \lfloor\frac{剩余的天数}{2}\rfloor ⌊2剩余的天数⌋, 2 2 2 个权值相加即可。
这里,直接枚举即可,具体看代码吧!
Code
#include D - Yet Another Inversions Problem
Solution
首先,考虑对于每 k k k 个数内部有多少个逆序对,通过归并排序或树状数组即可求出。
那么,由于 q q q 是一个 0 ∼ k − 1 0\sim k-1 0∼k−1 的排列,所以 0 ∼ k − 1 0\sim k-1 0∼k−1 一定是都有的。下面,就需要求出对于任意 2 2 2 个数 p i , p j p_i,p_j pi,pj 能够产生多少个逆序对。
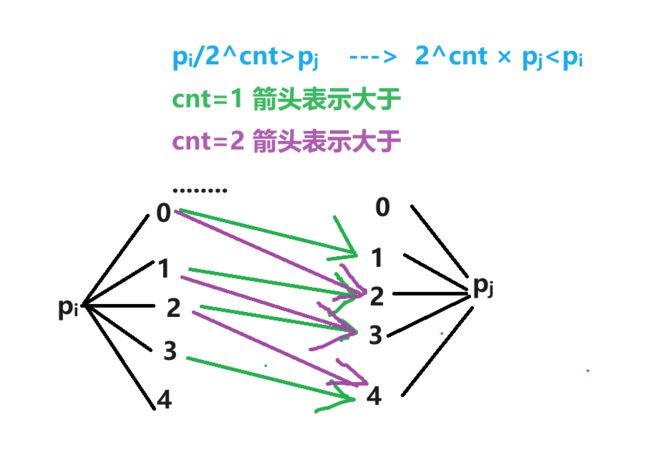
由于,对于每 1 1 1 个 p i p_i pi,都可以分解为 k k k 个: p i , 2 p i , 4 p i , 8 p i … p_i,2p_i,4p_i,8p_i\dots pi,2pi,4pi,8pi…。所以,可以考虑 乘 2 2 2 或 除 2 2 2 来判断,考虑对 p i p_i pi 不断除 2 2 2,如果除 2 2 2 之后还是大于 p j p_j pj,那么个数会多 k − c n t k-cnt k−cnt( c n t cnt cnt 表示除了多少次)
如图所示, k = 5 k=5 k=5, c n t cnt cnt 其实就意味着比当前倍数大 2 c n t 2^{cnt} 2cnt 的 p j p_j pj 还大,那符合条件的当前倍数的个数为 k − c n t k-cnt k−cnt,故贡献为 k − c n t k-cnt k−cnt。
对应着,还有不断乘 2 2 2,这就是再找 2 k p i > 2 k − c n t p j 2^kp_i>2^{k-cnt}p_j 2kpi>2k−cntpj,与上面的方法类似,箭头只是不断朝右上方罢了,大家可以自己画画,最后的贡献还是 k − c n t k-cnt k−cnt
不过,这样是 O ( n 2 ) O(n^2) O(n2),所以考虑优化。
观察可知,在枚举 p j p_j pj 的时候浪费了时间,所以可以用树状数组存储 p j p_j pj,然后可以做到 O ( log n ) O(\log n) O(logn) 的时间复杂度查询,那么贡献就变为了:个数 × \times × ( k − c n t ) (k-cnt) (k−cnt)
具体看代码吧(qwq)
Code
#include