Python算法题集_从前序与中序遍历序列构造二叉树

本文为Python算法题集之一的代码示例

题105：从前序与中序遍历序列构造二叉树

1. 示例说明

• 给定两个整数数组 preorder 和 inorder ，其中 preorder 是二叉树的先序遍历， inorder 是同一棵树的中序遍历，请构造二叉树并返回其根节点。

示例 1:

  输入: preorder = [3,9,20,15,7], inorder = [9,3,15,20,7]
输出: [3,9,20,null,null,15,7]

示例 2:

  输入: preorder = [-1], inorder = [-1]
输出: [-1]

提示:

• 1 <= preorder.length <= 3000
  • inorder.length == preorder.length
  • -3000 <= preorder[i], inorder[i] <= 3000
  • preorder 和 inorder 均 无重复 元素
  • inorder 均出现在 preorder
• preorder 保证 为二叉树的前序遍历序列
  • inorder 保证 为二叉树的中序遍历序列

---

2. 题目解析

- 题意分解

1. 先序遍历（Preorder Traversal）是常见的二叉树遍历方式，其顺序为：根节点 -> 左子树 -> 右子树【根|左|右】
2. 中序遍历（Inorder Traversal）是另一种二叉树遍历方式，其顺序为：左子树 -> 根节点 -> 右子树【左|根|右】
3. 本题有两个核心部分，1为preorder、inorder数组的根节点区分和左子树|右子树节点分离，2是生成链表
4. 基本的设计思路在于preorder次序为根|左|右，可以分离出根节点，然后依据inorder次序为左|根|右的特点，拆分出左子树、右子树

- 优化思路

1. 通常优化：减少循环层次

2. 通常优化：增加分支，减少计算集

3. 通常优化：采用内置算法来提升计算速度

4. 分析题目特点，分析最优解

   1. 可以考虑使用递归、迭代分别实现

   2. 先序遍历的第一个节点为二叉树的根节点

   3. 先序遍历根节点向左子树到底，中序遍历最左下节点为第一个，因此先序遍历列表递归左子树，遇到中序遍历的第一个节点截止，可依此解题

---

- 测量工具

• 本地化测试说明：LeetCode网站测试运行时数据波动很大【可把页面视为功能测试】，因此需要本地化测试解决数据波动问题
• CheckFuncPerf（本地化函数用时和内存占用测试模块）已上传到CSDN，地址：Python算法题集_检测函数用时和内存占用的模块
• 本题本地化超时测试用例自己生成，详见【最优算法章节】

---

3. 代码展开

1) 标准求解【先序生成+中序定位+递归】

使用先序列表生成根节点，用中序列表定位左右子树，递归展开

页面功能测试，马马虎虎，超过57%

import CheckFuncPerf as cfp

class Solution:
 def buildTree_base(self, preorder, inorder):
     if not preorder and not inorder:
         return
     root=TreeNode(preorder[0])
     irootidx=inorder.index(preorder[0])
     root.left=self.buildTree_base(preorder[1:irootidx+1],inorder[:irootidx])
     root.right=self.buildTree_base(preorder[irootidx+1:],inorder[irootidx+1:])
     return root

aSolution = Solution()
aroot = generate_symmetry_binary_tree(idepth, icountlist)
prelist = preorderTraversal(aroot)
inlist = inorderTraversal(aroot)
result = cfp.getTimeMemoryStr(Solution.buildTree_base, aSolution, prelist.copy(), inlist.copy())
print(result['msg'], '执行结果 = {}'.format(result['result'].val))

# 运行结果
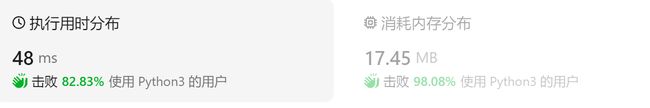
函数 buildTree_base 的运行时间为 137.60 ms；内存使用量为 10036.00 KB 执行结果 = 1

2) 改进版一【先序队列+中序队列+递归】

使用先序列表和中序列表作为队列，利用先序最左节点为中序第一个节点的特点，递归展开

页面功能测试，性能良好，超过83%

import CheckFuncPerf as cfp

class Solution:
 def buildTree_ext1(self, preorder, inorder):
     def build(rootval):
         if inorder and inorder[0] != rootval:
             root = TreeNode(preorder.pop(0))
             root.left = build(root.val)
             inorder.pop(0)
             root.right = build(rootval)
             return root
     return build(None)

aSolution = Solution()
aroot = generate_symmetry_binary_tree(idepth, icountlist)
prelist = preorderTraversal(aroot)
inlist = inorderTraversal(aroot)
result = cfp.getTimeMemoryStr(Solution.buildTree_ext1, aSolution, prelist.copy(), inlist.copy())
print(result['msg'], '执行结果 = {}'.format(result['result'].val))

# 运行结果
函数 buildTree_ext1 的运行时间为 791.85 ms；内存使用量为 10332.00 KB 执行结果 = 1

3) 改进版二【先序堆栈+中序堆栈+递归】

还是利用先序最左节点为中序第一个节点的特点进行递归展开，但是由于list的pop(0)【队列】性能远远低于pop(-1)【堆栈】，因此先将先序列表和中序列表反转，然后使用堆栈方式实现

页面功能测试，性能良好，超过82%

import CheckFuncPerf as cfp

class Solution:
 def buildTree_ext2(self, preorder, inorder):
     preorder.reverse()
     inorder.reverse()
     def build(rootval):
         if inorder and inorder[-1] != rootval:
             root = TreeNode(preorder.pop())
             root.left = build(root.val)
             inorder.pop()
             root.right = build(rootval)
             return root
     return build(None)
 
aSolution = Solution()
aroot = generate_symmetry_binary_tree(idepth, icountlist)
prelist = preorderTraversal(aroot)
inlist = inorderTraversal(aroot)
result = cfp.getTimeMemoryStr(Solution.buildTree_ext2, aSolution, prelist.copy(), inlist.copy())
print(result['msg'], '执行结果 = {}'.format(result['result'].val))

# 运行结果
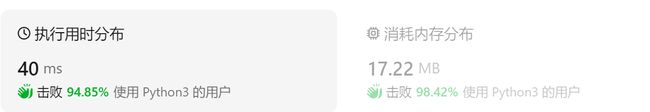
函数 buildTree_ext2 的运行时间为 147.61 ms；内存使用量为 8196.00 KB 执行结果 = 1

4) 改进版三【迭代+先序循环+辅助堆栈】

使用辅助堆栈实现迭代算法，以先序列表为主循环，堆栈和中序列表定位右子树的根节点

页面功能测试，性能优越，超越94%

import CheckFuncPerf as cfp

class Solution:
 def buildTree_ext3(self, preorder, inorder):
     if not preorder:
         return None
     root = TreeNode(preorder[0])
     stack = [root]
     inorderIndex = 0
     for iIdx in range(1, len(preorder)):
         preorderVal = preorder[iIdx]
         tmpnode = stack[-1]
         if tmpnode.val != inorder[inorderIndex]:
             tmpnode.left = TreeNode(preorderVal)
             stack.append(tmpnode.left)
         else:
             while stack and stack[-1].val == inorder[inorderIndex]:
                 tmpnode = stack.pop()
                 inorderIndex += 1
             tmpnode.right = TreeNode(preorderVal)
             stack.append(tmpnode.right)
     return root

aSolution = Solution()
aroot = generate_symmetry_binary_tree(idepth, icountlist)
prelist = preorderTraversal(aroot)
inlist = inorderTraversal(aroot)
result = cfp.getTimeMemoryStr(Solution.buildTree_ext2, aSolution, prelist.copy(), inlist.copy())
print(result['msg'], '执行结果 = {}'.format(result['result'].val))

# 运行结果
函数 buildTree_ext3 的运行时间为 113.70 ms；内存使用量为 8196.00 KB 执行结果 = 1

---

4. 最优算法

根据本地日志分析，最优算法为第4种方式【迭代+先序循环+辅助堆栈】buildTree_ext3

def inorderTraversal(root):
    if not root:
        return []
    list_stack = []
    list_node = []
    while root or list_stack:
        if root:
            list_stack.append(root)
            root = root.left
        else:
            curnode = list_stack.pop()
            list_node.append(curnode.val)
            root = curnode.right
    return list_node
def preorderTraversal(root):
    if root is None:
        return []
    list_node = []
    stack = [root]
    while stack:
        node = stack.pop()
        list_node.append(node.val)
        if node.right:
            stack.append(node.right)
        if node.left:
            stack.append(node.left)
    return list_node
def generate_symmetry_binary_tree(ilevel, icountlist):
    if ilevel <= 0:
        return None
    root = TreeNode(icountlist[0])
    icountlist[0] += 1
    left = generate_symmetry_binary_tree(ilevel - 1, icountlist)
    right = generate_symmetry_binary_tree(ilevel - 1, icountlist)
    root.left = left
    root.right = right
    return root
idepth, icountlist = 16, []
icountlist.append(1)
aroot = generate_symmetry_binary_tree(idepth, icountlist)
aSolution = Solution()
prelist = preorderTraversal(aroot)
inlist = inorderTraversal(aroot)
print(f'length of list = {len(prelist)}')
result = cfp.getTimeMemoryStr(Solution.buildTree_base, aSolution, prelist.copy(), inlist.copy())
print(result['msg'], '执行结果 = {}'.format(result['result'].val))
result = cfp.getTimeMemoryStr(Solution.buildTree_ext1, aSolution, prelist.copy(), inlist.copy())
print(result['msg'], '执行结果 = {}'.format(result['result'].val))
result = cfp.getTimeMemoryStr(Solution.buildTree_ext2, aSolution, prelist.copy(), inlist.copy())
print(result['msg'], '执行结果 = {}'.format(result['result'].val))
result = cfp.getTimeMemoryStr(Solution.buildTree_ext3, aSolution, prelist.copy(), inlist.copy())
print(result['msg'], '执行结果 = {}'.format(result['result'].val))

# 算法本地速度实测比较
length of list = 65535
函数 buildTree_base 的运行时间为 137.60 ms；内存使用量为 10036.00 KB 执行结果 = 1
函数 buildTree_ext1 的运行时间为 791.85 ms；内存使用量为 10332.00 KB 执行结果 = 1
函数 buildTree_ext2 的运行时间为 147.61 ms；内存使用量为 8196.00 KB 执行结果 = 1
函数 buildTree_ext3 的运行时间为 113.70 ms；内存使用量为 8196.00 KB 执行结果 = 1

一日练，一日功，一日不练十日空

may the odds be ever in your favor ~