数据结构(二):链表、链队列
上一篇博文中主要总结线性表的顺序存储结构实现,比如顺序表、顺序队列和顺序栈。具体可以参考上篇博文
http://blog.csdn.net/lg1259156776/article/details/46993591
下面要进行学习和总结的是线性表的链式存储结构实现,比如链表和链队列。
顺序存储结构的优缺点
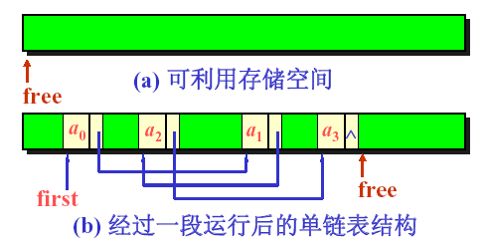
链表
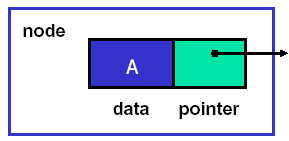
单向链表
typedef struct LNode
{
Datatype data;
struct LNode *next;
}
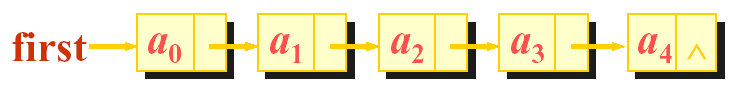
单向链表的存贮映像
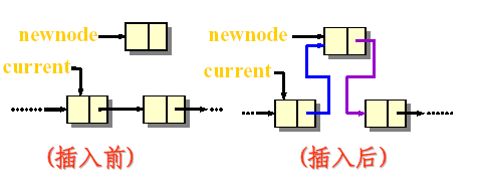
对应的指针操作
newnode->next= current->next;
current->next = newnode{
LNode *q;
q=new LNode;
If (q==NULL){错误信息}
q->data=item;
q->next=ptr->next;
ptr->next=q;
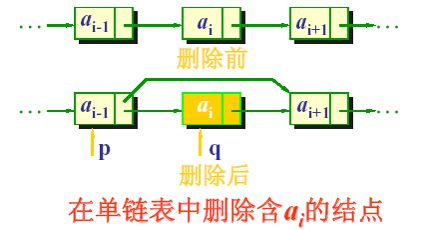
q=p->next;
If(q!=NULL){p.next=q.next;Deleteq;}
单链表的定义
多个类表达一个概念(单链表):链表结点(ListNode)、链表(List)
Typedef struct
{
LNode *front;
int Size;//并非必要的成员
} LList;
{
Lnode *p=L;
int k=0;
while(p){k++,p=p->next;}
return k;
}//时间复杂度O(n)
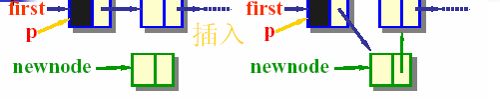
关于插入的讨论需要查找前驱,时间复杂度为O(n),如果指向第一个结点,要修改头指针
Void LListInsertB(LList *L,LNode *p,LNode *s)
{
LNode *q;
if(p==L){s->next=L;L=s;}
else { q=L;
while(q->next!=p)q=q->next;
q->next=s;s->next=p;
}
}
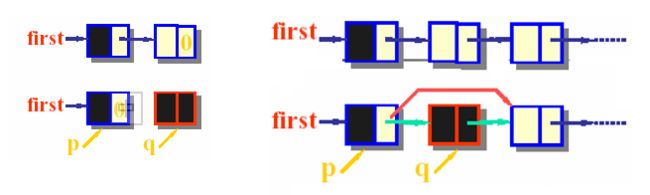
带表头结点的单链表
设置表头结点的目的是统一空表与非空表的操作,简化链表操作的实现
带表头结点的单链表插入Newnode->next=p->next ; p->next=newnode
从带表头结点的单链表中删除第一个结点
q= p->link;p->link = q->link;delete q;
{
int i;LNode *ptr;
如果pos不合法,退出
否则
ptr=NextNode(L->front);
for(i=0;i<pos;i++)
ptr=NextNode(ptr);
ptr->data=item
循环链表
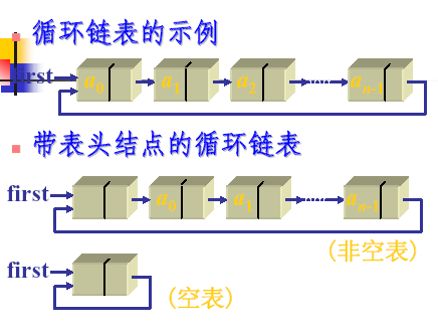
特殊链表
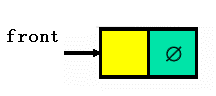
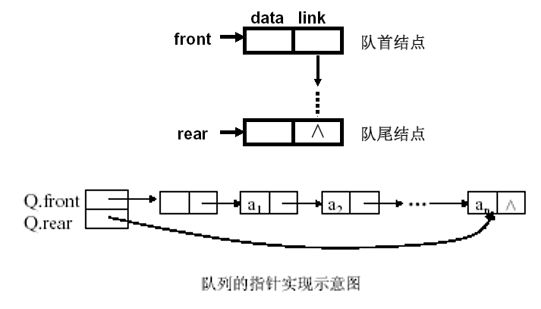
链表队列
Typedefstruct
{
LNode *front;
LNode *rear;
}LQuene;
{
Q->front=new LNode;
if(Q->front==NULL){err;}
SetNode(Q->front);
Q->rear=Q->front;
}
{
InsertAfter(Q->rear,item);
Q->rear =NextNode(Q->rear) ;
}
{
if (EMPTY( Q ) ){error;}
else{
temp = Q->front->next ;
Q->front->next =temp->next ;
delete temp ;
if ( Q->front->next == NULL )
Q->rear = Q->front ;
}
}
多项式的存储表示
静态数组
int degree;
float coef[maxDegree+1];动态数组
typedef struct
{
int degree;
float *coef;
}Polynomial;
构造函数:
VoidSetPoly ( Polynomial *poly,int sz ) {
Poly->degree= sz;
Poly->coef= new float [degree + 1];
}
以上两种存储表示适用于指数连续排列的多项式。但对于指数不全的多项式不经济
多项式的链表表示
在多项式的链表表示中每个结点三个数据成员
*************************************************************************************************************************************
2015-7-23