- C#中使用split分割字符串
互联网打工人no1
c#
1、用字符串分隔:usingSystem.Text.RegularExpressions;stringstr="aaajsbbbjsccc";string[]sArray=Regex.Split(str,"js",RegexOptions.IgnoreCase);foreach(stringiinsArray)Response.Write(i.ToString()+"");输出结果:aaabbbc
- WPF中的ComboBox控件几种数据绑定的方式
互联网打工人no1
wpfc#
一、用字典给ItemsSource赋值(此绑定用的地方很多,建议熟练掌握)在XMAL中:在CS文件中privatevoidBindData(){DictionarydicItem=newDictionary();dicItem.add(1,"北京");dicItem.add(2,"上海");dicItem.add(3,"广州");cmb_list.ItemsSource=dicItem;cmb_l
- python八股文面试题分享及解析(1)
Shawn________
python
#1.'''a=1b=2不用中间变量交换a和b'''#1.a=1b=2a,b=b,aprint(a)print(b)结果:21#2.ll=[]foriinrange(3):ll.append({'num':i})print(11)结果:#[{'num':0},{'num':1},{'num':2}]#3.kk=[]a={'num':0}foriinrange(3):#0,12#可变类型,不仅仅改变
- Redis系列:Geo 类型赋能亿级地图位置计算
Ly768768
redisbootstrap数据库
1前言我们在篇深刻理解高性能Redis的本质的时候就介绍过Redis的几种基本数据结构,它是基于不同业务场景而设计的:动态字符串(REDIS_STRING):整数(REDIS_ENCODING_INT)、字符串(REDIS_ENCODING_RAW)双端列表(REDIS_ENCODING_LINKEDLIST)压缩列表(REDIS_ENCODING_ZIPLIST)跳跃表(REDIS_ENCODI
- SpringBlade dict-biz/list 接口 SQL 注入漏洞
文章永久免费只为良心
oracle数据库
SpringBladedict-biz/list接口SQL注入漏洞POC:构造请求包查看返回包你的网址/api/blade-system/dict-biz/list?updatexml(1,concat(0x7e,md5(1),0x7e),1)=1漏洞概述在SpringBlade框架中,如果dict-biz/list接口的后台处理逻辑没有正确地对用户输入进行过滤或参数化查询(PreparedSta
- 一文掌握python常用的list(列表)操作
程序员neil
pythonpython开发语言
目录一、创建列表1.直接创建列表:2.使用list()构造器3.使用列表推导式4.创建空列表二、访问列表元素1.列表支持通过索引访问元素,索引从0开始:2.还可以使用切片操作访问列表的一部分:三、修改列表元素四、添加元素1.append():在末尾添加元素2.insert():在指定位置插入元素五、删除元素1.del:删除指定位置的元素2.remove():删除指定值的第一个匹配项3.pop():
- python中的深拷贝与浅拷贝
anshejd70787
python
深拷贝和浅拷贝浅拷贝的时候,修改原来的对象,浅拷贝的对象不会发生改变。1、对象的赋值对象的赋值实际上是对象之间的引用:当创建一个对象,然后将这个对象赋值给另外一个变量的时候,python并没有拷贝这个对象,而只是拷贝了这个对象的引用。当对对象做赋值或者是参数传递或者作为返回值的时候,总是传递原始对象的引用,而不是一个副本。如下所示:>>>aList=["kel","abc",123]>>>bLis
- 用Python实现读取统计单词个数
程序媛了了
python游戏java
完整实例代码:fromcollectionsimportCounterdefpythonit():danci={}withopen("pythonit.txt","r",encoding="utf-8")asf:foriinf:words=i.strip().split()forwordinwords:ifwordnotindanci:danci[word]=1else:danci[word]+=
- 2024.8.22 Python,链表两数之和,链表快速反转,二叉树的深度,二叉树前中后序遍历,N叉树递归遍历,翻转二叉树
RaidenQ
python链表开发语言
1.链表两数之和输入:l1=[2,4,3],l2=[5,6,4]输出:[7,0,8]解释:342+465=807.示例2:输入:l1=[0],l2=[0]输出:[0]示例3:输入:l1=[9,9,9,9,9,9,9],l2=[9,9,9,9]输出:[8,9,9,9,0,0,0,1]昨天的这个题,用自己的办法写的麻烦的要死,然后刚才一看chat归类的办法,感觉自己像个智障。classListNode
- ArrayList 源码解析
程序猿进阶
Java基础ArrayListListjava面试性能优化架构设计idea
ArrayList是Java集合框架中的一个动态数组实现,提供了可变大小的数组功能。它继承自AbstractList并实现了List接口,是顺序容器,即元素存放的数据与放进去的顺序相同,允许放入null元素,底层通过数组实现。除该类未实现同步外,其余跟Vector大致相同。每个ArrayList都有一个容量capacity,表示底层数组的实际大小,容器内存储元素的个数不能多于当前容量。当向容器中添
- Vue中table合并单元格用法
weixin_30613343
javascriptViewUI
地名结果人名性别{{item.name}}已完成未完成{{item.groups[0].name}}{{item.groups[0].sex}}{{item.groups[son].name}}{{item.groups[son].sex}}exportdefault{data(){return{list:[{name:'地名1',result:'1',groups:[{name:'张三',sex
- Android应用性能优化
轻口味
Android
Android手机由于其本身的后台机制和硬件特点,性能上一直被诟病,所以软件开发者对软件本身的性能优化就显得尤为重要;本文将对Android开发过程中性能优化的各个方面做一个回顾与总结。Cache优化ListView缓存:ListView中有一个回收器,Item滑出界面的时候View会回收到这里,需要显示新的Item的时候,就尽量重用回收器里面的View;每次在getView函数中inflate新
- tiff批量转png
诺有缸的高飞鸟
opencv图像处理pythonopencv图像处理
目录写在前面代码完写在前面1、本文内容tiff批量转png2、平台/环境opencv,python3、转载请注明出处:https://blog.csdn.net/qq_41102371/article/details/132975023代码importnumpyasnpimportcv2importosdeffindAllFile(base):file_list=[]forroot,ds,fsin
- 《 C++ 修炼全景指南:四 》揭秘 C++ List 容器背后的实现原理,带你构建自己的双向链表
Lenyiin
技术指南C++修炼全景指南c++list链表stl
本篇博客,我们将详细讲解如何从头实现一个功能齐全且强大的C++List容器,并深入到各个细节。这篇博客将包括每一步的代码实现、解释以及扩展功能的探讨,目标是让初学者也能轻松理解。一、简介1.1、背景介绍在C++中,std::list是一个基于双向链表的容器,允许高效的插入和删除操作,适用于频繁插入和删除操作的场景。与动态数组不同,list允许常数时间内的插入和删除操作,支持双向遍历。这篇文章将详细
- 02-Cesium聚合分析EntityCluster完整代码
fxshy
htmlcssjavascript
1.完整代码Document-->-->Cesium.Ion.defaultAccessToken='eyJhbGciOiJIUzI1NiIsInR5cCI6IkpXVCJ9.eyJqdGkiOiJhZjZkZDAwZC1mNTFhLTRhOTEtOGExNi00MzRhNGIzMDdlNDQiLCJpZCI6MTA1MTUzLCJpYXQiOjE2NjA4MDg0Njd9.qajeJtc4-kp
- 03-Cesium自定义着色器完整代码以及注释
fxshy
着色器javascript
1.效果展示2.完整代码自定义着色器完整代码#map{position:absolute;width:100%;height:100%;top:0;left:0;right:0;bottom:0;}Cesium.Ion.defaultAccessToken='eyJhbGciOiJIUzI1NiIsInR5cCI6IkpXVCJ9.eyJqdGkiOiJhZjZkZDAwZC1mNTFhLTRhO
- 代码随想录Day 41|动态规划之买卖股票问题,leetcode题目121. 买卖股票的最佳时机、122. 买卖股票的最佳时机Ⅱ、123. 买卖股票的最佳时机Ⅲ
LluckyYH
动态规划leetcode算法数据结构
提示:DDU,供自己复习使用。欢迎大家前来讨论~文章目录买卖股票的最佳时机相关题目题目一:121.买卖股票的最佳时机解题思路:题目二:122.买卖股票的最佳时机II解题思路:题目三:123.买卖股票的最佳时机III解题思路总结买卖股票的最佳时机相关题目题目一:121.买卖股票的最佳时机[[121.买卖股票的最佳时机](https://leetcode.cn/problems/combination
- Android实现监听事件的方法
Amy木婉清
1.通过内部类实现2.通过匿名内部类实现3.通过事件源所在类实现4.通过外部类实现5.布局文件中onclick属性(针对点击事件)1.通过内部类实现代码:privateButtonmBtnEvent;//oncreate中mBtnEvent.setOnClickListener(newOnClick());//内部类实现监听classOnClickimplementsView.OnClickLis
- leetcode中等.数组(21-40)python
九日火
pythonleetcode
80.RemoveDuplicatesfromSortedArrayII(m-21)Givenasortedarraynums,removetheduplicatesin-placesuchthatduplicatesappearedatmosttwiceandreturnthenewlength.Donotallocateextraspaceforanotherarray,youmustdoth
- uniapp实现动态标记效果详细步骤【前端开发】
2401_85123349
uni-app
第二个点在于实现将已经被用户标记的内容在下一次获取后刷新它的状态为已标记。这是什么意思呢?比如说上面gif图中的这些人物对象,有一些已被该用户添加为关心,那么当用户下一次进入该页面时,这些已经被添加关心的对象需要以“红心”状态显现出来。这个点的难度还不算大,只需要在每一次获取后端的内容后对标记对象进行状态更新即可。II.动态标记效果实现思路和步骤首先,整体的思路是利用动态类名对不同的元素进行选择。
- IO虚拟化 - virtio-vring的三个组成结构【转】
xidianjiapei001
#虚拟化技术
1.初始化三个结构vring_new_virtqueue函数中初始化virtqueue的各种字段的初始值vq->vq.callback=callback;vq->vq.vdev=vdev;vq->vq.name=name;vq->notify=notify;vq->broken=false;vq->last_used_idx=0;vq->num_added=0;list_add_tail(&vq-
- leetcode刷题day13|二叉树Part01(递归遍历、迭代遍历、统一迭代、层序遍历)
小冉在学习
leetcode算法职场和发展
递归遍历思路:使用递归的方式比较简单。1、递归函数的传参:因为最后输出一个数组,所以需要传入根节点和一个容器,本来想写数组,但发现长度不能确定,所以选择list。2、终止条件:当访问的节点为空时,return3、递归函数的逻辑:先访问一个节点,递归访问其他节点144.二叉树的前序遍历代码如下:classSolution{publicListpreorderTraversal(TreeNoderoo
- leetcode021-合并两个有序链表
陆阳226
问题描述将两个升序链表合并为一个新的升序链表并返回。新链表是通过拼接给定的两个链表的所有节点组成的。示例:输入:1->2->4,1->3->4输出:1->1->2->3->4->4解答递归法:每一层减去一个较小的节点,直到某个链表为null递归结束。publicstaticListNodesolution(ListNodel1,ListNodel2){if(l1==null){returnl2;}
- 设计模式】Listener模式和Visitor模式的区别
不爱洗脚的小滕
设计模式访问者模式javagolang
文章目录前言一、介绍Listener模式Visitor模式二、代码实现2.1Listener模式的Java实现2.2Listener模式的Go实现2.3Visitor模式的Java实现2.4Visitor模式的Go实现三、总结前言在软件设计中,设计模式是解决特定问题的通用解决方案。Listener模式和Visitor模式是两种常见的行为设计模式,它们在不同的场景下提供了解决问题的有效方法。本文将详
- 如何区分Python中数据类型可变还是不可变
秸秆混凝烧结工程师
关键字改变元素值,内存地址发生改变,被称为数据内型不可变如string,元组,存储数据类型单一,不能同时存在两个数据类型,新增元素后,表容量,元素个数,元素存储区ID改变,典型的内置元素一体存储法;改变元素值,但是内存地址不改变就是可变数据内型,如list,存储元素可以不同,删除,新增,插入,表序列不改变,扩展表容量时,对象地址ID不变,属于顺序表的,分离式存储结构,外置元素法,python中不可
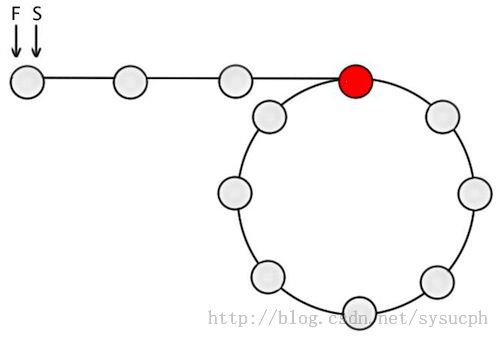
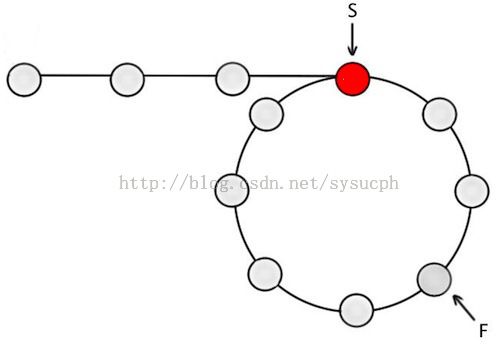
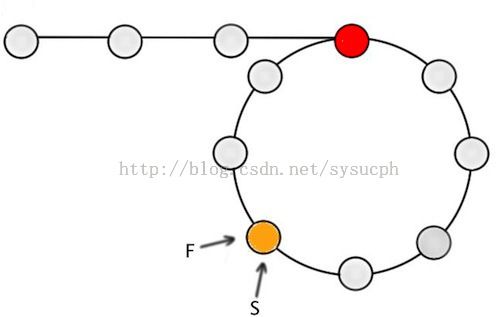
- 每日一题《leetcode--LCR 022.环形链表||》
Peace & Love487
题目分享leetcode链表算法笔记数据结构
https://leetcode.cn/problems/c32eOV/我们使用两个指针,fast与slow。它们起始都位于链表的头部。随后slow指针每次向后移动一个位置,而fast指针向后移动两个位置。如果链表中存在环,则fast指针最终将再次与slow指针在环中相遇。structListNode*detectCycle(structListNode*head){structListNode*
- LCR 078. 合并 K 个升序链表
装B且挨揍の
LeetCode链表算法数据结构经验分享笔记java
https://leetcode.cn/problems/vvXgSW/description/https://leetcode.cn/problems/vvXgSW/description/解题思路方法一:每个链表维护一个索引,每次找到值最小的节点,索引加一。可以采用优先队列实现。/***Definitionforsingly-linkedlist.*publicclassListNode{*i
- python字符串相等怎么表示_python怎样判断字符串相等
weixin_39993989
python字符串相等怎么表示
python字符串如何判断相等1.is来判断groupName=params['groupName']##groupName的值是'url'reqBody['dim']=groupNameprint("reqBody_dim-SummaryListHandler",reqBody['dim'])##('reqBody_dim-SummaryListHandler',u'url')print("re
- linux gcc 格式,Linux下gcc与gdb简介
神奇的战士
linuxgcc格式
gcc编译器可以将C、C++等语言源程序、汇编程序编译、链接成可执行程序。gdb是GNU开发的一个Unix/Linux下强大的程序调试工具。linux下没有后缀名的概念。但gcc根据文件的后缀来区别输入文件的类别:.cC语言源代码文件.a由目标文件构成的库文件.C、.cc、.cppC++源码文件.h头文件.i经过预处理之后的C语言文件.ii经过预处理之后的C++文件.o编译后的目标文件.s汇编源码
- Python中判断两个字符串的内容是否相同
songyuc
《Python学习笔记》Python
1前言今天在划分数据集的时候,需要判断两个字符串的内容是否相同,这个之前查过,不过好像忘记了,所以想着再记录一下~2Python中判断两个字符串的内容是否相同使用“==”符号进行判断,这个判断是根据字符串中字符的ASCII进行判断的;在判断字符串内容是否相同时,不能使用“is”进行判断,因为is是判断变量的内存ID(即使用函数id(a)获得变量的内存ID)是否相同;
- 继之前的线程循环加到窗口中运行
3213213333332132
javathreadJFrameJPanel
之前写了有关java线程的循环执行和结束,因为想制作成exe文件,想把执行的效果加到窗口上,所以就结合了JFrame和JPanel写了这个程序,这里直接贴出代码,在窗口上运行的效果下面有附图。
package thread;
import java.awt.Graphics;
import java.text.SimpleDateFormat;
import java.util
- linux 常用命令
BlueSkator
linux命令
1.grep
相信这个命令可以说是大家最常用的命令之一了。尤其是查询生产环境的日志,这个命令绝对是必不可少的。
但之前总是习惯于使用 (grep -n 关键字 文件名 )查出关键字以及该关键字所在的行数,然后再用 (sed -n '100,200p' 文件名),去查出该关键字之后的日志内容。
但其实还有更简便的办法,就是用(grep -B n、-A n、-C n 关键
- php heredoc原文档和nowdoc语法
dcj3sjt126com
PHPheredocnowdoc
<!doctype html>
<html lang="en">
<head>
<meta charset="utf-8">
<title>Current To-Do List</title>
</head>
<body>
<?
- overflow的属性
周华华
JavaScript
<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://www.w3.org/TR/xhtml1/DTD/xhtml1-transitional.dtd">
<html xmlns="http://www.w3.org/1999/xhtml&q
- 《我所了解的Java》——总体目录
g21121
java
准备用一年左右时间写一个系列的文章《我所了解的Java》,目录及内容会不断完善及调整。
在编写相关内容时难免出现笔误、代码无法执行、名词理解错误等,请大家及时指出,我会第一时间更正。
&n
- [简单]docx4j常用方法小结
53873039oycg
docx
本代码基于docx4j-3.2.0,在office word 2007上测试通过。代码如下:
import java.io.File;
import java.io.FileInputStream;
import ja
- Spring配置学习
云端月影
spring配置
首先来看一个标准的Spring配置文件 applicationContext.xml
<?xml version="1.0" encoding="UTF-8"?>
<beans xmlns="http://www.springframework.org/schema/beans"
xmlns:xsi=&q
- Java新手入门的30个基本概念三
aijuans
java新手java 入门
17.Java中的每一个类都是从Object类扩展而来的。 18.object类中的equal和toString方法。 equal用于测试一个对象是否同另一个对象相等。 toString返回一个代表该对象的字符串,几乎每一个类都会重载该方法,以便返回当前状态的正确表示.(toString 方法是一个很重要的方法) 19.通用编程:任何类类型的所有值都可以同object类性的变量来代替。
- 《2008 IBM Rational 软件开发高峰论坛会议》小记
antonyup_2006
软件测试敏捷开发项目管理IBM活动
我一直想写些总结,用于交流和备忘,然都没提笔,今以一篇参加活动的感受小记开个头,呵呵!
其实参加《2008 IBM Rational 软件开发高峰论坛会议》是9月4号,那天刚好调休.但接着项目颇为忙,所以今天在中秋佳节的假期里整理了下.
参加这次活动是一个朋友给的一个邀请书,才知道有这样的一个活动,虽然现在项目暂时没用到IBM的解决方案,但觉的参与这样一个活动可以拓宽下视野和相关知识.
- PL/SQL的过程编程,异常,声明变量,PL/SQL块
百合不是茶
PL/SQL的过程编程异常PL/SQL块声明变量
PL/SQL;
过程;
符号;
变量;
PL/SQL块;
输出;
异常;
PL/SQL 是过程语言(Procedural Language)与结构化查询语言(SQL)结合而成的编程语言PL/SQL 是对 SQL 的扩展,sql的执行时每次都要写操作
- Mockito(三)--完整功能介绍
bijian1013
持续集成mockito单元测试
mockito官网:http://code.google.com/p/mockito/,打开documentation可以看到官方最新的文档资料。
一.使用mockito验证行为
//首先要import Mockito
import static org.mockito.Mockito.*;
//mo
- 精通Oracle10编程SQL(8)使用复合数据类型
bijian1013
oracle数据库plsql
/*
*使用复合数据类型
*/
--PL/SQL记录
--定义PL/SQL记录
--自定义PL/SQL记录
DECLARE
TYPE emp_record_type IS RECORD(
name emp.ename%TYPE,
salary emp.sal%TYPE,
dno emp.deptno%TYPE
);
emp_
- 【Linux常用命令一】grep命令
bit1129
Linux常用命令
grep命令格式
grep [option] pattern [file-list]
grep命令用于在指定的文件(一个或者多个,file-list)中查找包含模式串(pattern)的行,[option]用于控制grep命令的查找方式。
pattern可以是普通字符串,也可以是正则表达式,当查找的字符串包含正则表达式字符或者特
- mybatis3入门学习笔记
白糖_
sqlibatisqqjdbc配置管理
MyBatis 的前身就是iBatis,是一个数据持久层(ORM)框架。 MyBatis 是支持普通 SQL 查询,存储过程和高级映射的优秀持久层框架。MyBatis对JDBC进行了一次很浅的封装。
以前也学过iBatis,因为MyBatis是iBatis的升级版本,最初以为改动应该不大,实际结果是MyBatis对配置文件进行了一些大的改动,使整个框架更加方便人性化。
- Linux 命令神器:lsof 入门
ronin47
lsof
lsof是系统管理/安全的尤伯工具。我大多数时候用它来从系统获得与网络连接相关的信息,但那只是这个强大而又鲜为人知的应用的第一步。将这个工具称之为lsof真实名副其实,因为它是指“列出打开文件(lists openfiles)”。而有一点要切记,在Unix中一切(包括网络套接口)都是文件。
有趣的是,lsof也是有着最多
- java实现两个大数相加,可能存在溢出。
bylijinnan
java实现
import java.math.BigInteger;
import java.util.regex.Matcher;
import java.util.regex.Pattern;
public class BigIntegerAddition {
/**
* 题目:java实现两个大数相加,可能存在溢出。
* 如123456789 + 987654321
- Kettle学习资料分享,附大神用Kettle的一套流程完成对整个数据库迁移方法
Kai_Ge
Kettle
Kettle学习资料分享
Kettle 3.2 使用说明书
目录
概述..........................................................................................................................................7
1.Kettle 资源库管
- [货币与金融]钢之炼金术士
comsci
金融
自古以来,都有一些人在从事炼金术的工作.........但是很少有成功的
那么随着人类在理论物理和工程物理上面取得的一些突破性进展......
炼金术这个古老
- Toast原来也可以多样化
dai_lm
androidtoast
Style 1: 默认
Toast def = Toast.makeText(this, "default", Toast.LENGTH_SHORT);
def.show();
Style 2: 顶部显示
Toast top = Toast.makeText(this, "top", Toast.LENGTH_SHORT);
t
- java数据计算的几种解决方法3
datamachine
javahadoopibatisr-languer
4、iBatis
简单敏捷因此强大的数据计算层。和Hibernate不同,它鼓励写SQL,所以学习成本最低。同时它用最小的代价实现了计算脚本和JAVA代码的解耦,只用20%的代价就实现了hibernate 80%的功能,没实现的20%是计算脚本和数据库的解耦。
复杂计算环境是它的弱项,比如:分布式计算、复杂计算、非数据
- 向网页中插入透明Flash的方法和技巧
dcj3sjt126com
htmlWebFlash
将
Flash 作品插入网页的时候,我们有时候会需要将它设为透明,有时候我们需要在Flash的背面插入一些漂亮的图片,搭配出漂亮的效果……下面我们介绍一些将Flash插入网页中的一些透明的设置技巧。
一、Swf透明、无坐标控制 首先教大家最简单的插入Flash的代码,透明,无坐标控制: 注意wmode="transparent"是控制Flash是否透明
- ios UICollectionView的使用
dcj3sjt126com
UICollectionView的使用有两种方法,一种是继承UICollectionViewController,这个Controller会自带一个UICollectionView;另外一种是作为一个视图放在普通的UIViewController里面。
个人更喜欢第二种。下面采用第二种方式简单介绍一下UICollectionView的使用。
1.UIViewController实现委托,代码如
- Eos平台java公共逻辑
蕃薯耀
Eos平台java公共逻辑Eos平台java公共逻辑
Eos平台java公共逻辑
>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>
蕃薯耀 2015年6月1日 17:20:4
- SpringMVC4零配置--Web上下文配置【MvcConfig】
hanqunfeng
springmvc4
与SpringSecurity的配置类似,spring同样为我们提供了一个实现类WebMvcConfigurationSupport和一个注解@EnableWebMvc以帮助我们减少bean的声明。
applicationContext-MvcConfig.xml
<!-- 启用注解,并定义组件查找规则 ,mvc层只负责扫描@Controller -->
<
- 解决ie和其他浏览器poi下载excel文件名乱码
jackyrong
Excel
使用poi,做传统的excel导出,然后想在浏览器中,让用户选择另存为,保存用户下载的xls文件,这个时候,可能的是在ie下出现乱码(ie,9,10,11),但在firefox,chrome下没乱码,
因此必须综合判断,编写一个工具类:
/**
*
* @Title: pro
- 挥洒泪水的青春
lampcy
编程生活程序员
2015年2月28日,我辞职了,离开了相处一年的触控,转过身--挥洒掉泪水,毅然来到了兄弟连,背负着许多的不解、质疑——”你一个零基础、脑子又不聪明的人,还敢跨行业,选择Unity3D?“,”真是不自量力••••••“,”真是初生牛犊不怕虎•••••“,••••••我只是淡淡一笑,拎着行李----坐上了通向挥洒泪水的青春之地——兄弟连!
这就是我青春的分割线,不后悔,只会去用泪水浇灌——已经来到
- 稳增长之中国股市两点意见-----严控做空,建立涨跌停版停牌重组机制
nannan408
对于股市,我们国家的监管还是有点拼的,但始终拼不过飞流直下的恐慌,为什么呢?
笔者首先支持股市的监管。对于股市越管越荡的现象,笔者认为首先是做空力量超过了股市自身的升力,并且对于跌停停牌重组的快速反应还没建立好,上市公司对于股价下跌没有很好的利好支撑。
我们来看美国和香港是怎么应对股灾的。美国是靠禁止重要股票做空,在
- 动态设置iframe高度(iframe高度自适应)
Rainbow702
JavaScriptiframecontentDocument高度自适应局部刷新
如果需要对画面中的部分区域作局部刷新,大家可能都会想到使用ajax。
但有些情况下,须使用在页面中嵌入一个iframe来作局部刷新。
对于使用iframe的情况,发现有一个问题,就是iframe中的页面的高度可能会很高,但是外面页面并不会被iframe内部页面给撑开,如下面的结构:
<div id="content">
<div id=&quo
- 用Rapael做图表
tntxia
rap
function drawReport(paper,attr,data){
var width = attr.width;
var height = attr.height;
var max = 0;
&nbs
- HTML5 bootstrap2网页兼容(支持IE10以下)
xiaoluode
html5bootstrap
<!DOCTYPE html>
<html>
<head lang="zh-CN">
<meta charset="UTF-8">
<meta http-equiv="X-UA-Compatible" content="IE=edge">