poj2528--Mayor's posters(线段树+离散化)
Mayor's posters
| Time Limit: 1000MS | Memory Limit: 65536K | |
| Total Submissions: 41785 | Accepted: 12164 |
Description
The citizens of Bytetown, AB, could not stand that the candidates in the mayoral election campaign have been placing their electoral posters at all places at their whim. The city council has finally decided to build an electoral wall for placing the posters and introduce the following rules:
They have built a wall 10000000 bytes long (such that there is enough place for all candidates). When the electoral campaign was restarted, the candidates were placing their posters on the wall and their posters differed widely in width. Moreover, the candidates started placing their posters on wall segments already occupied by other posters. Everyone in Bytetown was curious whose posters will be visible (entirely or in part) on the last day before elections.
Your task is to find the number of visible posters when all the posters are placed given the information about posters' size, their place and order of placement on the electoral wall.
- Every candidate can place exactly one poster on the wall.
- All posters are of the same height equal to the height of the wall; the width of a poster can be any integer number of bytes (byte is the unit of length in Bytetown).
- The wall is divided into segments and the width of each segment is one byte.
- Each poster must completely cover a contiguous number of wall segments.
They have built a wall 10000000 bytes long (such that there is enough place for all candidates). When the electoral campaign was restarted, the candidates were placing their posters on the wall and their posters differed widely in width. Moreover, the candidates started placing their posters on wall segments already occupied by other posters. Everyone in Bytetown was curious whose posters will be visible (entirely or in part) on the last day before elections.
Your task is to find the number of visible posters when all the posters are placed given the information about posters' size, their place and order of placement on the electoral wall.
Input
The first line of input contains a number c giving the number of cases that follow. The first line of data for a single case contains number 1 <= n <= 10000. The subsequent n lines describe the posters in the order in which they were placed. The i-th line among the n lines contains two integer numbers l
i and ri which are the number of the wall segment occupied by the left end and the right end of the i-th poster, respectively. We know that for each 1 <= i <= n, 1 <= l
i <= ri <= 10000000. After the i-th poster is placed, it entirely covers all wall segments numbered l
i, l
i+1 ,... , ri.
Output
For each input data set print the number of visible posters after all the posters are placed.
The picture below illustrates the case of the sample input.
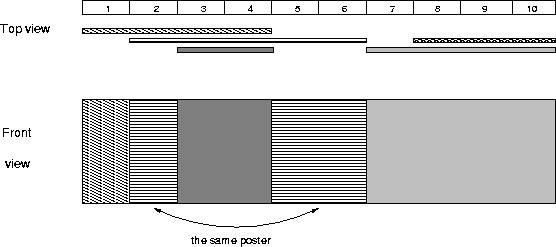
The picture below illustrates the case of the sample input.

Sample Input
1 5 1 4 2 6 8 10 3 4 7 10
Sample Output
4
题意:给出一面墙,给出n张海报贴在墙上,每张海报都覆盖一个范围,问最后可以看到多少张海报
海报覆盖的范围很大,直接使用数组存不下,但是只有最多10000张海报,也就是说最多出现20000个点,所以可以使用离散化,将每个点离散后,重新对给出控制的区间,这样区间最大就是1到20000.可以直接使用线段树,成段更新,每次更新一个颜色,最后遍历所有的段,将还可以遍历的颜色储存,统计
将-1 定义为没有颜色,0代表有多种颜色,1到m代表各自的颜色,线段树只要在0时向下深入,其他的直接统计颜色。
但是这个题中的离散化有问题,会挤掉中间的颜色,不过poj好像也是这么做的,没有考虑中间的颜色。
如 1 ,10 1,4 7,10 这三组数的正确结果应该是还可以看到3种颜色,但是如果直接排列点的话就会挤掉5到6这一种颜色。
真正的这种成段的离散化,一定要将两个节点中还有值的话,要多加一个节点,代表两个节点中间的值。
#include <cstdio>
#include <cstring>
#include <algorithm>
using namespace std;
#define maxn 30000
#define lmin 1
#define rmax n
#define lson l,(l+r)/2,rt<<1
#define rson (l+r)/2+1,r,rt<<1|1
#define root lmin,rmax,1
#define now l,r,rt
#define int_now int l,int r,int rt
int cl[maxn<<2] , lazy[maxn<<2] , a[maxn] ;
struct node{
int id1 , id2 , k ;
}p[maxn];
bool cmp(node a,node b)
{
return a.k < b.k ;
}
void push_up(int_now)
{
if( !cl[rt<<1] || !cl[rt<<1|1] || cl[rt<<1] != cl[rt<<1|1] )
cl[rt] = 0 ;
else
cl[rt] = cl[rt<<1|1] ;
}
void push_down(int_now)
{
if( lazy[rt] != -1 )
{
lazy[rt<<1] = lazy[rt<<1|1] = lazy[rt] ;
cl[rt<<1] = cl[rt<<1|1] = lazy[rt] ;
lazy[rt] = -1 ;
}
}
void update(int ll,int rr,int x,int_now)
{
if( ll > r || rr < l )
return ;
if( ll <= l && r <= rr )
{
cl[rt] = lazy[rt] = x ;
return ;
}
push_down(now);
update(ll,rr,x,lson);
update(ll,rr,x,rson);
push_up(now);
}
void query(int ll,int rr,int_now,int *a)
{
if( cl[rt] == -1 )
return ;
else if(cl[rt] > 0)
{
a[ cl[rt] ] = 1 ;
return ;
}
push_down(now);
query(ll,rr,lson,a);
query(ll,rr,rson,a);
}
int main()
{
int t , i , n , m , l , r , x ;
scanf("%d", &t);
while(t--)
{
scanf("%d", &m);
for(i = 0 ; i < m ; i++)
{
scanf("%d %d", &p[i].k, &p[i+m].k);
p[i].id1 = i ;
p[i+m].id1 = i+m ;
}
sort(p,p+2*m,cmp);
int temp = -1 ;
n = 0 ;
for(i = 0 ; i < 2*m ; i++)
{
if( p[i].k == temp )
p[i].id2 = n ;
else
{
p[i].id2 = ++n ;
temp = p[i].k ;
}
a[ p[i].id1 ] = p[i].id2 ;
}
memset(cl,-1,sizeof(cl));
memset(lazy,-1,sizeof(lazy));
for(i = 0 ; i < m ; i++)
{
update(a[i],a[i+m],i+1,root);
}
memset(a,0,sizeof(a));
query(1,n,root,a);
int num = 0;
for(i = 1 ; i <= m ; i++)
if(a[i])
num++ ;
printf("%d\n", num);
}
return 0;
}