POJ 2318 TOYS + 2398 Toy Storage（计算几何叉积）

POJ 2318 TOYS ：http://poj.org/problem?id=2318

Language: Default TOYS Time Limit: 2000MS   Memory Limit: 65536K Total Submissions: 13267   Accepted: 6416 Description Calculate the number of toys that land in each bin of a partitioned toy box.  Mom and dad have a problem - their child John never puts his toys away when he is finished playing with them. They gave John a rectangular box to put his toys in, but John is rebellious and obeys his parents by simply throwing his toys into the box. All the toys get mixed up, and it is impossible for John to find his favorite toys.  John's parents came up with the following idea. They put cardboard partitions into the box. Even if John keeps throwing his toys into the box, at least toys that get thrown into different bins stay separated. The following diagram shows a top view of an example toy box.    For this problem, you are asked to determine how many toys fall into each partition as John throws them into the toy box. Input The input file contains one or more problems. The first line of a problem consists of six integers, n m x1 y1 x2 y2. The number of cardboard partitions is n (0 < n <= 5000) and the number of toys is m (0 < m <= 5000). The coordinates of the upper-left corner and the lower-right corner of the box are (x1,y1) and (x2,y2), respectively. The following n lines contain two integers per line, Ui Li, indicating that the ends of the i-th cardboard partition is at the coordinates (Ui,y1) and (Li,y2). You may assume that the cardboard partitions do not intersect each other and that they are specified in sorted order from left to right. The next m lines contain two integers per line, Xj Yj specifying where the j-th toy has landed in the box. The order of the toy locations is random. You may assume that no toy will land exactly on a cardboard partition or outside the boundary of the box. The input is terminated by a line consisting of a single 0. Output The output for each problem will be one line for each separate bin in the toy box. For each bin, print its bin number, followed by a colon and one space, followed by the number of toys thrown into that bin. Bins are numbered from 0 (the leftmost bin) to n (the rightmost bin). Separate the output of different problems by a single blank line. Sample Input 5 6 0 10 60 0 3 1 4 3 6 8 10 10 15 30 1 5 2 1 2 8 5 5 40 10 7 9 4 10 0 10 100 0 20 20 40 40 60 60 80 80 5 10 15 10 25 10 35 10 45 10 55 10 65 10 75 10 85 10 95 10 0 Sample Output 0: 2 1: 1 2: 1 3: 1 4: 0 5: 1 0: 2 1: 2 2: 2 3: 2 4: 2 Hint As the example illustrates, toys that fall on the boundary of the box are "in" the box.

代码实现：

#include <iostream>
#include <cstdio>
#include <cstring>

using namespace std;

struct Point
{
    int x,y;
};
struct line
{
    Point a,b;
}line[5050];

int cnt[5050];

int Multi(Point p1,Point p2,Point p0)
{
    return (p1.x-p0.x)*(p2.y-p0.y)-(p2.x-p0.x)*(p1.y-p0.y);
}

void BSearch(Point a,int n)
{
    int l,r,mid;

    l=0;
    r=n-1;
    while(l<r)
    {
        mid=(l+r)>>1;
        if(Multi(a,line[mid].a,line[mid].b)>0)
            l=mid+1;
        else r=mid;
    }
    if(Multi(a,line[l].a,line[l].b)<0)
        cnt[l]++;
    else cnt[l+1]++;
}

int main()
{
    int n,m,x1,y1,x2,y2;
    int t1,t2;
    Point a;
    while(scanf("%d",&n)!=EOF)
    {
        if(n==0)
            break;
        scanf("%d%d%d%d%d",&m,&x1,&y1,&x2,&y2);
        for(int i=0;i<n;i++)
        {
            scanf("%d%d",&t1,&t2);
            line[i].a.x=t1;
            line[i].a.y=y1;
            line[i].b.x=t2;
            line[i].b.y=y2;
        }
        memset(cnt,0,sizeof(cnt));
        for(int i=0;i<m;i++)
        {
            scanf("%d%d",&a.x,&a.y);
            BSearch(a,n);
        }
        for(int i=0;i<=n;i++)
        {
            printf("%d: %d\n",i,cnt[i]);
        }
        printf("\n");
    }
    return 0;
}

POJ 2398 Toy Storage ：http://poj.org/problem?id=2398

Toy Storage

Time Limit: 1000MS		Memory Limit: 65536K
Total Submissions: 5095		Accepted: 3023

Description

Mom and dad have a problem: their child, Reza, never puts his toys away when he is finished playing with them. They gave Reza a rectangular box to put his toys in. Unfortunately, Reza is rebellious and obeys his parents by simply throwing his toys into the box. All the toys get mixed up, and it is impossible for Reza to find his favorite toys anymore. 
Reza's parents came up with the following idea. They put cardboard partitions into the box. Even if Reza keeps throwing his toys into the box, at least toys that get thrown into different partitions stay separate. The box looks like this from the top: 

We want for each positive integer t, such that there exists a partition with t toys, determine how many partitions have t, toys.

Input

The input consists of a number of cases. The first line consists of six integers n, m, x1, y1, x2, y2. The number of cardboards to form the partitions is n (0 < n <= 1000) and the number of toys is given in m (0 < m <= 1000). The coordinates of the upper-left corner and the lower-right corner of the box are (x1, y1) and (x2, y2), respectively. The following n lines each consists of two integers Ui Li, indicating that the ends of the ith cardboard is at the coordinates (Ui, y1) and (Li, y2). You may assume that the cardboards do not intersect with each other. The next m lines each consists of two integers Xi Yi specifying where the ith toy has landed in the box. You may assume that no toy will land on a cardboard. 

A line consisting of a single 0 terminates the input.

Output

For each box, first provide a header stating "Box" on a line of its own. After that, there will be one line of output per count (t > 0) of toys in a partition. The value t will be followed by a colon and a space, followed the number of partitions containing t toys. Output will be sorted in ascending order of t for each box.

Sample Input

4 10 0 10 100 0
20 20
80 80
60 60
40 40
5 10
15 10
95 10
25 10
65 10
75 10
35 10
45 10
55 10
85 10
5 6 0 10 60 0
4 3
15 30
3 1
6 8
10 10
2 1
2 8
1 5
5 5
40 10
7 9
0

Sample Output

Box
2: 5
Box
1: 4
2: 1

代码实现：

#include <iostream>
#include <cstdio>
#include <cstring>
#include <algorithm>

using namespace std;

struct Point
{
    int x,y;
    Point(){}
    Point(int _x,int _y)
    {
        x=_x;
        y=_y;
    }
    Point operator -(const Point &b)const
    {
        return Point(x-b.x,y-b.y);
    }
    int operator *(const Point &b)const
    {
        return x*b.x+y*b.y;
    }
    int operator ^(const Point &b)const
    {
        return x*b.y-y*b.x;
    }
};

struct Line
{
    Point s,e;
    Line(){}
    Line(Point _s,Point _e)
    {
        s=_s;
        e=_e;
    }
};

int xmult(Point p0,Point p1,Point p2)
{
    return (p1-p0)^(p2-p0);
}

const int MAXN=5050;
Line line[MAXN];
int ans[MAXN];
int num[MAXN];

bool cmp(Line a,Line b)
{
    return a.s.x<b.s.x;
}

int main()
{
    int n,m,x1,y1,x2,y2;
    int ui,li;
    while(scanf("%d",&n)!=EOF)
    {
        if(n==0)
        {
            break;
        }
        else
        {
            scanf("%d%d%d%d%d",&m,&x1,&y1,&x2,&y2);
            for(int i=0;i<n;i++)
            {
                scanf("%d%d",&ui,&li);
                line[i] = Line(Point(ui,y1) , Point(li,y2));
            }
            line[n]=Line(Point(x2,y1),Point(x2,y2));
            sort(line,line+n+1,cmp);
            int x,y;
            Point p;
            memset(ans,0,sizeof(ans));
            while(m--)
            {
                scanf("%d%d",&x,&y);
                p=Point(x,y);
                int l=0,r=n;
                int tmp;
                while(l<=r)
                {
                    int mid=(l+r)/2;
                    if(xmult(p,line[mid].s,line[mid].e)<0)
                    {
                        tmp=mid;
                        r=mid-1;
                    }
                    else l=mid+1;
                }
                ans[tmp]++;
            }
            for(int i=1;i<=n;i++)
            {
                num[i]=0;
            }
            for( int i=0;i<=n;i++)
            {
                if(ans[i]>0)
                    num[ans[i]]++;
            }
            printf("Box\n");
            for(int i=1;i<=n;i++)
            {
                if(num[i]>0)
                {
                    printf("%d: %d\n",i,num[i]);
                }
            }
        }
    }
    return 0;
}

Language: Default TOYS Time Limit: 2000MS   Memory Limit: 65536K Total Submissions: 13267   Accepted: 6416 Description Calculate the number of toys that land in each bin of a partitioned toy box.  Mom and dad have a problem - their child John never puts his toys away when he is finished playing with them. They gave John a rectangular box to put his toys in, but John is rebellious and obeys his parents by simply throwing his toys into the box. All the toys get mixed up, and it is impossible for John to find his favorite toys.  John's parents came up with the following idea. They put cardboard partitions into the box. Even if John keeps throwing his toys into the box, at least toys that get thrown into different bins stay separated. The following diagram shows a top view of an example toy box. 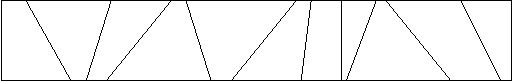   For this problem, you are asked to determine how many toys fall into each partition as John throws them into the toy box. Input The input file contains one or more problems. The first line of a problem consists of six integers, n m x1 y1 x2 y2. The number of cardboard partitions is n (0 < n <= 5000) and the number of toys is m (0 < m <= 5000). The coordinates of the upper-left corner and the lower-right corner of the box are (x1,y1) and (x2,y2), respectively. The following n lines contain two integers per line, Ui Li, indicating that the ends of the i-th cardboard partition is at the coordinates (Ui,y1) and (Li,y2). You may assume that the cardboard partitions do not intersect each other and that they are specified in sorted order from left to right. The next m lines contain two integers per line, Xj Yj specifying where the j-th toy has landed in the box. The order of the toy locations is random. You may assume that no toy will land exactly on a cardboard partition or outside the boundary of the box. The input is terminated by a line consisting of a single 0. Output The output for each problem will be one line for each separate bin in the toy box. For each bin, print its bin number, followed by a colon and one space, followed by the number of toys thrown into that bin. Bins are numbered from 0 (the leftmost bin) to n (the rightmost bin). Separate the output of different problems by a single blank line. Sample Input 5 6 0 10 60 0 3 1 4 3 6 8 10 10 15 30 1 5 2 1 2 8 5 5 40 10 7 9 4 10 0 10 100 0 20 20 40 40 60 60 80 80 5 10 15 10 25 10 35 10 45 10 55 10 65 10 75 10 85 10 95 10 0 Sample Output 0: 2 1: 1 2: 1 3: 1 4: 0 5: 1 0: 2 1: 2 2: 2 3: 2 4: 2 Hint As the example illustrates, toys that fall on the boundary of the box are "in" the box.