- 第一场雪
岁月静好_nx
早晨起来,外面白茫茫的一片,总算是下雪了,这还是今年第一场雪呢!走在路上,踩着雪“咯吱咯吱”的,空气很湿润。树上、草坪上、屋顶上都落了白白的一层,天上还零星漂着几点雪。慢慢走在路上,呼吸着清新的空气,感受着冬天的美好,心情也好多了。
- 山东大学小树林支教调研团青青仓木队——翟晓楠
山东大学青青仓木队
过了半年,又一次启程,又一次回到支教的初心之地。比起上一次的试探与不安,我更多了一丝稳重与熟练。心境、处境也都随着半个学期的过去而变得不同,半个学期中,身体上的,心理上的,太多的逆境让我变得步履维艰,曲曲折折,弯弯绕绕,我仿佛打不起精神,没有胃口,没有动力。感觉走的不顺畅的时候,支教这个旅程,给了我力量。自告奋勇承担起队长这一职务的我,从组织时的复杂和困难的经历,协调各种问题,从无到有,和校长和队
- 2018/02/12
Tracy_zhang
人生并不在于获取,更在于放得下。放下一粒种子,收获一棵大树;放下一处烦恼,收获一个惊喜;放下一种偏见,收获一种幸福;放下一种执著,收获一种自在。放下既是一种理性抉择,也是一种豁达美。只要看得开放得下,何愁没有快乐的春莺在啼鸣,何愁没有快乐的泉溪在歌唱,何愁没有快乐的鲜花绽放!
- 春季养肝正当时
dxn悟
重温快乐2023年2月4日立春。春天来了,春暖花开,小鸟欢唱,那在这样的季节我们如何养肝呢?自然界的春季对应中医五行的木,人体五脏肝属木,“木曰曲直”,是以树干曲曲直直地向上、向外伸长舒展的生发姿态,来形容具有生长、升发、条达、舒畅等特征的食物及现象。根据中医天人相应的理念,肝五行属木,喜条达,主疏泄,与春天相应,所以春天最适合养肝。养肝首先要少生气,因为肝喜条达恶抑郁。人体五志肝为怒,生气发怒最
- 每日算法&面试题,大厂特训二十八天——第二十天(树)
肥学
⚡算法题⚡面试题每日精进java算法数据结构
目录标题导读算法特训二十八天面试题点击直接资料领取导读肥友们为了更好的去帮助新同学适应算法和面试题,最近我们开始进行专项突击一步一步来。上一期我们完成了动态规划二十一天现在我们进行下一项对各类算法进行二十八天的一个小总结。还在等什么快来一起肥学进行二十八天挑战吧!!特别介绍小白练手专栏,适合刚入手的新人欢迎订阅编程小白进阶python有趣练手项目里面包括了像《机器人尬聊》《恶搞程序》这样的有趣文章
- Python爬虫解析工具之xpath使用详解
eqa11
python爬虫开发语言
文章目录Python爬虫解析工具之xpath使用详解一、引言二、环境准备1、插件安装2、依赖库安装三、xpath语法详解1、路径表达式2、通配符3、谓语4、常用函数四、xpath在Python代码中的使用1、文档树的创建2、使用xpath表达式3、获取元素内容和属性五、总结Python爬虫解析工具之xpath使用详解一、引言在Python爬虫开发中,数据提取是一个至关重要的环节。xpath作为一门
- 2018-12-29
枫叶红时总多离别
2018年12月29日星期六昨天老师就告诉我们,今天下午不用上课,是图书漂流活动会。我觉得很兴奋,好期待。到了下午,我帮好忙就到外面去买书,刚一出去,就有一大帮的大哥哥、大姐姐围着我问要不要买书,买一本书送一颗糖。我看到了一本《小老虎比上树》的书,问大姐姐多少钱,大姐姐说这本书原价13块,现在便宜4块钱也就是9块钱卖给你,我就把一张10块钱给她找,她找了我一块钱。我现在想想我今天只带了10块钱,现
- 似乎老是忘记什么东西
灰台
S带上了耳机,眼前的一切都与她隔绝开来。虽是初春的好天气,花都开的正鲜艳,行人也都驻足欣赏,还有不少怀着好心情的年轻人在花树下打闹。不过S似乎并不在意这些,连耳机传来的rap也没有调动起她的兴致。一瞬间,心脏好像变成了黑洞,“啊,我身边还有几个人呢,似乎没有了吧”。阳光的温度覆盖到了脖子上,S抬头看了看开满花的树,“我妈好像还挺喜欢花的”,S随手拍了一张照片,微信发到自己一家三口的群里。过了一会,
- 《在战“疫”中成长致敬生活》观后感
梅子刘的刀
(作者:周晨)今天上午,我看了“我是接班人”网络大课堂《在战役中成长致敬生活》。有很多人拿出自己攒下的钱,默默地捐给了武汉,有几千块钱的、有几万块钱的,也有十几万块钱的。连小朋友也把自己的压岁钱捐给了武汉。有名环卫工人把自己五年的积蓄全部捐给了武汉。有名外卖小哥为医护人员买鞋子送吃的。还有已经治愈出院的新型肺炎病人捐了400毫升的血浆。还有位叫大树的叔叔,虽然他没有钱,但是他地里有蔬菜,捐了几大卡
- 2019-08-16
希望在东方
《春游荣华山》春游荣华山,乍暖还寒。青苔路,石阶险。山路弯上弯!为寻古寺往幽探。细雨已润江南岸,初春芳草现。老树新芽冒枝端,人间又过到新年。今游荣华山,树茂参天,古寺悠闲。细雨飘落发端!三眼井旁,投币许心愿,并祷一世安然。更喜大女明事端,应心安,放开颜。修竹静默,雨中吐心愿。待得春风浩吹时,春笋节节攀。图片发自App图片发自App图片发自App
- 一颗小桃树
李蓉乐平市湾头中小学
当“凹”同“洼”的时侯,才读(wa,平声),他不叫贾平洼(贾,原名贾平娃),非要写作贾平凹。为了表示对他的尊重,对文学的尊重,对文化人的尊重。如果不是帮闺蜜的儿子修改作文,我也不会发现贾平凹叫贾平娃。以下是摘选他的文章《一棵小桃树》:可我的小桃树儿,一颗“仙桃”的种子,却开得太白了,太淡了,那瓣片儿单薄得似纸做的,没有肉的感觉,没有粉的感觉,像患了重病的少女,苍白白的脸,又偏苦涩涩地笑着。雨还在下
- 安居士/海滨:落雪无声
海滨公园
安居士/海滨:落雪无声落雪无声作者:安居士/海滨安居士/海滨:落雪无声寒风凛冽裹挟着尘埃横扫大地上所有河流山脉落木萧萧枯叶瑟瑟虎吼龙吟一夜劲舞狂歌驱散多日不散的雾霾安居士/海滨:落雪无声你从浩渺天宇翩然飞来内心深藏着纯洁温柔的爱飘飘洒洒纷纷扬扬而来宛若一群美丽的仙女下凡袅袅婷婷尽显万千仪态安居士/海滨:落雪无声我从迷离的梦境里醒来临窗远眺山河间表里澄澈天地茫茫白雪皑皑琼林玉枝银桃挤挤挨挨似千树万树
- 祭坛随笔
阿门不热
街角右拐,便是北宋的祠堂。平日里冉冉的佛香被雨水打湿了,一地枯黄的银杏显得平静哀伤,如同一地被踩碎的阳光。我喜欢在这样的阴暗里吞噬古代的讯息,那遥远的来自过去的历史风潮。谢却茶扉,轻轻地抚上墙壁,寒风不御,无数深浅的纹路交织在心底,如同一把古琴不堪重负的尾音。寂寞锁朱门,香客们已是三三两两,巨大的雨帘让天空失掉了颜色,灰蒙蒙掉在阁楼一角,沉稳不惊地暗下去,再暗下去......古树上红色的挂牌像一块
- 2024.8.22 Python,链表两数之和,链表快速反转,二叉树的深度,二叉树前中后序遍历,N叉树递归遍历,翻转二叉树
RaidenQ
python链表开发语言
1.链表两数之和输入:l1=[2,4,3],l2=[5,6,4]输出:[7,0,8]解释:342+465=807.示例2:输入:l1=[0],l2=[0]输出:[0]示例3:输入:l1=[9,9,9,9,9,9,9],l2=[9,9,9,9]输出:[8,9,9,9,0,0,0,1]昨天的这个题,用自己的办法写的麻烦的要死,然后刚才一看chat归类的办法,感觉自己像个智障。classListNode
- 山海师秘录(草稿小段)
白淼清流
“阿弱,山北头还有两树好梨!我们给你留着呢,你快去采了吧,我们玩会儿就回家了。”几个伙伴都望着宁虚,其中一个胖胖喊道。宁虚望过去:“知道啦!你们趁着路好走,赶紧回家吧,这天一会儿怕是要下雨!”说罢,宁虚紧了紧后背的竹筐,迈步向山上走去。今天是村里采梨的最后一天,最多倒晚饭时,剩下的梨子便不许村民再采摘,熟透了便掉到地上,当做下次结果的肥料。宁虚顺着工匠铺出的简陋石道,却没有去同伴所说的山北头,而是
- 《 C++ 修炼全景指南:九 》打破编程瓶颈!掌握二叉搜索树的高效实现与技巧
Lenyiin
C++修炼全景指南技术指南c++算法stl
摘要本文详细探讨了二叉搜索树(BinarySearchTree,BST)的核心概念和技术细节,包括插入、查找、删除、遍历等基本操作,并结合实际代码演示了如何实现这些功能。文章深入分析了二叉搜索树的性能优势及其时间复杂度,同时介绍了前驱、后继的查找方法等高级功能。通过自定义实现的二叉搜索树类,读者能够掌握其实际应用,此外,文章还建议进一步扩展为平衡树(如AVL树、红黑树)以优化极端情况下的性能退化。
- 我家纱窗上全是杨树毛子
viiiiiiiiito
1“所以你脖…嘶…子上的伤不是你自己抓出来的喽?”永河喜欢在说话说到一半的时候吸烟,这总让他产生一些惊人的断句。”恩,不是给你说了么,方易在厕所门口就和别人打起来了,从厕所一路打到酒吧门口,他说我是去劝架,被误伤的。”“后来呢?”“后来就打车回家了啊。”“我是说打…嘶…架,赢了输了?“”完全不记得了,方易连他打的是谁都不知道,我看我这浑身疼的,估计是输了。“”垃圾,要不是我赶飞机昨天我们肯定…”“
- 《 C++ 修炼全景指南:十 》自平衡的艺术:深入了解 AVL 树的核心原理与实现
Lenyiin
C++修炼全景指南技术指南c++数据结构stl
摘要本文深入探讨了AVL树(自平衡二叉搜索树)的概念、特点以及实现细节。我们首先介绍了AVL树的基本原理,并详细分析了其四种旋转操作,包括左旋、右旋、左右双旋和右左双旋,阐述了它们在保持树平衡中的重要作用。接着,本文从头到尾详细描述了AVL树的插入、删除和查找操作,配合完整的代码实现和详尽的注释,使读者能够全面理解这些操作的执行过程。此外,我们还提供了AVL树的遍历方法,包括中序、前序和后序遍历,
- 【树一线性代数】005入门
Owlet_woodBird
算法
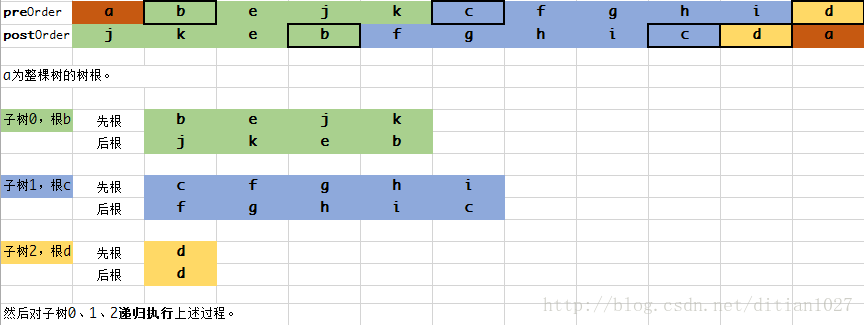
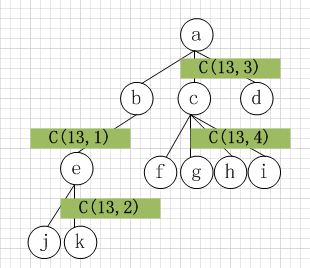
Index本文稍后补全,推荐阅读:https://blog.csdn.net/weixin_60702024/article/details/141874376分析实现总结本文稍后补全,推荐阅读:https://blog.csdn.net/weixin_60702024/article/details/141874376已知非空二叉树T的结点值均为正整数,采用顺序存储方式保存,数据结构定义如下:t
- 学点警句
处世须待春风
一个人只要知道自己去哪里,全世界都会给他让步。—爱默生—你日渐平庸,甘于平庸,将继续平庸。—《以自己的方式过一生》—不怕面对阴影,因为知道身后有阳光。那些你很冒险的梦,我陪你去疯。《你是最好的自己》—其实人跟树是一样的,越是向往高处的阳光,它的根就越要伸向黑暗的地底。—尼采—
- 中学生父母的修养
再简单不过了
我是一个中学生的父母,我有许多心情,偶尔彷徨,偶尔愤怒,偶尔欣喜,偶尔还会感伤,我彷徨的是。他仿佛瞬间长大了,失去了我的掌控,从而愤怒他不再和我那么亲近,第一次告诉我说,你根本就不懂我,欣喜的是,我经常看到的小一些人,已经以我察觉不到的速度慢慢的蜕变成一棵树,虽然不够枝繁叶茂,但是已经有很多分支,绿叶还有开叉了,我感觉自己并没有老去,但孩子却已经有自己的世界。他会说,不准随便进他的房间,不要乱问班
- 《流年一曲成殇》连载47
方冷颜
第四十七章青葱岁月宋曲殇躺在床上,看着寝室的灯已经熄灭了,在上铺的好处大概就是可以看到窗外的景。军训基地在郊区,所以自然环境特别的好,是没有受到污染的地区,晚上真的可以看到满天繁星。一轮弯月挂在天空,月光透过窗户,照在窗外的柏树上,那挺拔的柏树像守卫银河系的战士。宋晟波的舞,就像电影的回放,让她大吃一惊。生活中似乎充满着惊喜,发现,就是一种惊喜。付流年的歌究竟是什么?她好想找个有网络的地方搜一番。
- 酒店床装车出货
臧冰
一百多套的酒店床、圆床,床垫终于出货了,可惜还没装完,明天将继续出货,辛苦了各位小伙伴们!图片发自App图片发自App图片发自App图片发自App图片发自App图片发自App图片发自App图片发自App我是两个孩子的宝妈,经营着一间软体家具厂,“伊力威斯”是我们的品牌。这是我的第178篇原创日记。栽一棵树最好的时间是十年前跟今天,写日记亦是如此,抓住今天,我们将收获更精彩的人生!
- leetcode-617. 合并二叉树
manba_
leetcodehot100leetcode算法
题目描述给你两棵二叉树:root1和root2。想象一下,当你将其中一棵覆盖到另一棵之上时,两棵树上的一些节点将会重叠(而另一些不会)。你需要将这两棵树合并成一棵新二叉树。合并的规则是:如果两个节点重叠,那么将这两个节点的值相加作为合并后节点的新值;否则,不为null的节点将直接作为新二叉树的节点。返回合并后的二叉树。注意:合并过程必须从两个树的根节点开始。示例1:输入:root1=[1,3,2,
- 轻风拂柳《春意萦怀》之六
轻风拂柳
图/来自网络轻风拂柳《春意萦怀》之六轻风拂柳《春意萦怀》原韵烂熳芳林赏丽容,春光明媚盼相逢。娇桃绽蕊仙姿艳,淑杏凝脂玉色浓。对对黄莺穿树影,双双彩蝶逐花踪。风情小雅灵犀有,景美难将笔墨封。图/来自网络步轻风拂柳《春意萦怀》原韵(一)诗·时就三月阳春思丽容,花红柳绿也相逢。不歆桃蕊风姿艳,只慕书斋墨色浓。期翼共窗难觅影,时望携手苦寻踪。天涯海角君何有?一颗痴心哪日封?(二)诗·大漠孤烟滴翠丛林展媚容
- 《爱情》
杜文霞
杜文霞坚持原创分享第39天(20190214)图片发自App对爱情的认识我越来越清晰了。真正的爱情是成年人的游戏,双方在关系中是平等的。就像舒婷《致橡树》中写的:我如果爱你——绝不学痴情的鸟儿,为绿荫重复单调的歌曲;必须是你近旁的一株木棉,作为树的形象和你站在一起。我们共享雾霭、流岚、虹霓。仿佛永远分离,却又终身相依。爱情中的爱是相互的,是爱与被爱的流动,不是控制和占有。如果一方总觉得另一方“应该
- 《我的职业是小说家》
simple梦
《我的职业是小说家》:《我的职业是小说家》是村上春树前所未有的自传性作品,历时六年完成。一个人,写作三十五年,十三部长篇小说,超过五十种语言译本。虽然拥有享誉世界的知名度,但关于村上春树,许多事情始终包裹在神秘的面纱中:他是怎样下定决心走上职业小说家之路?对他来说,人生中幸福的事是什么?究竟如何看待芥川奖与诺贝尔文学奖……小说家看似风光,却是份孤独的职业。三十五年来,村上春树在孤独中编织着美妙动人
- 因为付出,所以精彩
江南雨1
新年第一天,我哪里都没有去。就在家里读书写字,想一想我的人生很平淡:童年是不懂忧虑的。小时候在家里,有父母长辈的疼爱。六岁上的学,那年祖父过世了。祖母继续疼着我,天天给我讲故事,在物质匮乏的年代还能给我做骨头粥、蒸鸡蛋之类的美食。父母虽然贫困,但是只要我需要的学习资料都会给我买,我是1981年开始读小学一年级,小学四五年级的时候父亲就给我订阅了《中国少年报》。家里有不少果树,每年都有梨子、龙眼、番
- 种树的最好时光
山药君123
总想着什么时候开个头。对,到底什么时候开个头呢?今天拖明天拖,一晃几个月过去了,再一晃,一年两年过去了。一回头,呦,过去这么长时间了,要是当初早点开始肯定早就怎么怎么样……你看,人总是做的少,想的美。种树的最好时光是十年前,其次是现在。择日不如撞日,就今天吧!不然真要等到七老八十再来感叹蹉跎了多少光阴?说不上想写点什么,权当碎碎念吧。最近要试着调整生活作息了,目标是早睡早起,加油!
- leetcode刷题day19|二叉树Part07(235. 二叉搜索树的最近公共祖先、701.二叉搜索树中的插入操作、450.删除二叉搜索树中的节点)
小冉在学习
leetcode算法数据结构
235.二叉搜索树的最近公共祖先思路:二叉搜索树首先考虑中序遍历。根据二叉搜索树的特性,如果p,q分别在中间节点的左右两边,该中间节点一定是最近公共祖先,如果在同一侧,则递归这一侧即可。递归三部曲:1、传入参数:根节点,p,q,返回节点。2、终止条件:因为p,q一定存在,所以不会遍历到树的最底层,因此可以不写终止条件3、递归逻辑:如果p,q均小于root的值,递归调用左子树;如果p,q均大于roo
- 开发者关心的那些事
圣子足道
ios游戏编程apple支付
我要在app里添加IAP,必须要注册自己的产品标识符(product identifiers)。产品标识符是什么?
产品标识符(Product Identifiers)是一串字符串,它用来识别你在应用内贩卖的每件商品。App Store用产品标识符来检索产品信息,标识符只能包含大小写字母(A-Z)、数字(0-9)、下划线(-)、以及圆点(.)。你可以任意排列这些元素,但我们建议你创建标识符时使用
- 负载均衡器技术Nginx和F5的优缺点对比
bijian1013
nginxF5
对于数据流量过大的网络中,往往单一设备无法承担,需要多台设备进行数据分流,而负载均衡器就是用来将数据分流到多台设备的一个转发器。
目前有许多不同的负载均衡技术用以满足不同的应用需求,如软/硬件负载均衡、本地/全局负载均衡、更高
- LeetCode[Math] - #9 Palindrome Number
Cwind
javaAlgorithm题解LeetCodeMath
原题链接:#9 Palindrome Number
要求:
判断一个整数是否是回文数,不要使用额外的存储空间
难度:简单
分析:
题目限制不允许使用额外的存储空间应指不允许使用O(n)的内存空间,O(1)的内存用于存储中间结果是可以接受的。于是考虑将该整型数反转,然后与原数字进行比较。
注:没有看到有关负数是否可以是回文数的明确结论,例如
- 画图板的基本实现
15700786134
画图板
要实现画图板的基本功能,除了在qq登陆界面中用到的组件和方法外,还需要添加鼠标监听器,和接口实现。
首先,需要显示一个JFrame界面:
public class DrameFrame extends JFrame { //显示
- linux的ps命令
被触发
linux
Linux中的ps命令是Process Status的缩写。ps命令用来列出系统中当前运行的那些进程。ps命令列出的是当前那些进程的快照,就是执行ps命令的那个时刻的那些进程,如果想要动态的显示进程信息,就可以使用top命令。
要对进程进行监测和控制,首先必须要了解当前进程的情况,也就是需要查看当前进程,而 ps 命令就是最基本同时也是非常强大的进程查看命令。使用该命令可以确定有哪些进程正在运行
- Android 音乐播放器 下一曲 连续跳几首歌
肆无忌惮_
android
最近在写安卓音乐播放器的时候遇到个问题。在MediaPlayer播放结束时会回调
player.setOnCompletionListener(new OnCompletionListener() {
@Override
public void onCompletion(MediaPlayer mp) {
mp.reset();
Log.i("H
- java导出txt文件的例子
知了ing
javaservlet
代码很简单就一个servlet,如下:
package com.eastcom.servlet;
import java.io.BufferedOutputStream;
import java.io.IOException;
import java.net.URLEncoder;
import java.sql.Connection;
import java.sql.Resu
- Scala stack试玩, 提高第三方依赖下载速度
矮蛋蛋
scalasbt
原文地址:
http://segmentfault.com/a/1190000002894524
sbt下载速度实在是惨不忍睹, 需要做些配置优化
下载typesafe离线包, 保存为ivy本地库
wget http://downloads.typesafe.com/typesafe-activator/1.3.4/typesafe-activator-1.3.4.zip
解压r
- phantomjs安装(linux,附带环境变量设置) ,以及casperjs安装。
alleni123
linuxspider
1. 首先从官网
http://phantomjs.org/下载phantomjs压缩包,解压缩到/root/phantomjs文件夹。
2. 安装依赖
sudo yum install fontconfig freetype libfreetype.so.6 libfontconfig.so.1 libstdc++.so.6
3. 配置环境变量
vi /etc/profil
- JAVA IO FileInputStream和FileOutputStream,字节流的打包输出
百合不是茶
java核心思想JAVA IO操作字节流
在程序设计语言中,数据的保存是基本,如果某程序语言不能保存数据那么该语言是不可能存在的,JAVA是当今最流行的面向对象设计语言之一,在保存数据中也有自己独特的一面,字节流和字符流
1,字节流是由字节构成的,字符流是由字符构成的 字节流和字符流都是继承的InputStream和OutPutStream ,java中两种最基本的就是字节流和字符流
类 FileInputStream
- Spring基础实例(依赖注入和控制反转)
bijian1013
spring
前提条件:在http://www.springsource.org/download网站上下载Spring框架,并将spring.jar、log4j-1.2.15.jar、commons-logging.jar加载至工程1.武器接口
package com.bijian.spring.base3;
public interface Weapon {
void kil
- HR看重的十大技能
bijian1013
提升能力HR成长
一个人掌握何种技能取决于他的兴趣、能力和聪明程度,也取决于他所能支配的资源以及制定的事业目标,拥有过硬技能的人有更多的工作机会。但是,由于经济发展前景不确定,掌握对你的事业有所帮助的技能显得尤为重要。以下是最受雇主欢迎的十种技能。 一、解决问题的能力 每天,我们都要在生活和工作中解决一些综合性的问题。那些能够发现问题、解决问题并迅速作出有效决
- 【Thrift一】Thrift编译安装
bit1129
thrift
什么是Thrift
The Apache Thrift software framework, for scalable cross-language services development, combines a software stack with a code generation engine to build services that work efficiently and s
- 【Avro三】Hadoop MapReduce读写Avro文件
bit1129
mapreduce
Avro是Doug Cutting(此人绝对是神一般的存在)牵头开发的。 开发之初就是围绕着完善Hadoop生态系统的数据处理而开展的(使用Avro作为Hadoop MapReduce需要处理数据序列化和反序列化的场景),因此Hadoop MapReduce集成Avro也就是自然而然的事情。
这个例子是一个简单的Hadoop MapReduce读取Avro格式的源文件进行计数统计,然后将计算结果
- nginx定制500,502,503,504页面
ronin47
nginx 错误显示
server {
listen 80;
error_page 500/500.html;
error_page 502/502.html;
error_page 503/503.html;
error_page 504/504.html;
location /test {return502;}}
配置很简单,和配
- java-1.二叉查找树转为双向链表
bylijinnan
二叉查找树
import java.util.ArrayList;
import java.util.List;
public class BSTreeToLinkedList {
/*
把二元查找树转变成排序的双向链表
题目:
输入一棵二元查找树,将该二元查找树转换成一个排序的双向链表。
要求不能创建任何新的结点,只调整指针的指向。
10
/ \
6 14
/ \
- Netty源码学习-HTTP-tunnel
bylijinnan
javanetty
Netty关于HTTP tunnel的说明:
http://docs.jboss.org/netty/3.2/api/org/jboss/netty/channel/socket/http/package-summary.html#package_description
这个说明有点太简略了
一个完整的例子在这里:
https://github.com/bylijinnan
- JSONUtil.serialize(map)和JSON.toJSONString(map)的区别
coder_xpf
jqueryjsonmapval()
JSONUtil.serialize(map)和JSON.toJSONString(map)的区别
数据库查询出来的map有一个字段为空
通过System.out.println()输出 JSONUtil.serialize(map): {"one":"1","two":"nul
- Hibernate缓存总结
cuishikuan
开源sshjavawebhibernate缓存三大框架
一、为什么要用Hibernate缓存?
Hibernate是一个持久层框架,经常访问物理数据库。
为了降低应用程序对物理数据源访问的频次,从而提高应用程序的运行性能。
缓存内的数据是对物理数据源中的数据的复制,应用程序在运行时从缓存读写数据,在特定的时刻或事件会同步缓存和物理数据源的数据。
二、Hibernate缓存原理是怎样的?
Hibernate缓存包括两大类:Hib
- CentOs6
dalan_123
centos
首先su - 切换到root下面1、首先要先安装GCC GCC-C++ Openssl等以来模块:yum -y install make gcc gcc-c++ kernel-devel m4 ncurses-devel openssl-devel2、再安装ncurses模块yum -y install ncurses-develyum install ncurses-devel3、下载Erang
- 10款用 jquery 实现滚动条至页面底端自动加载数据效果
dcj3sjt126com
JavaScript
无限滚动自动翻页可以说是web2.0时代的一项堪称伟大的技术,它让我们在浏览页面的时候只需要把滚动条拉到网页底部就能自动显示下一页的结果,改变了一直以来只能通过点击下一页来翻页这种常规做法。
无限滚动自动翻页技术的鼻祖是微博的先驱:推特(twitter),后来必应图片搜索、谷歌图片搜索、google reader、箱包批发网等纷纷抄袭了这一项技术,于是靠滚动浏览器滚动条
- ImageButton去边框&Button或者ImageButton的背景透明
dcj3sjt126com
imagebutton
在ImageButton中载入图片后,很多人会觉得有图片周围的白边会影响到美观,其实解决这个问题有两种方法
一种方法是将ImageButton的背景改为所需要的图片。如:android:background="@drawable/XXX"
第二种方法就是将ImageButton背景改为透明,这个方法更常用
在XML里;
<ImageBut
- JSP之c:foreach
eksliang
jspforearch
原文出自:http://www.cnblogs.com/draem0507/archive/2012/09/24/2699745.html
<c:forEach>标签用于通用数据循环,它有以下属性 属 性 描 述 是否必须 缺省值 items 进行循环的项目 否 无 begin 开始条件 否 0 end 结束条件 否 集合中的最后一个项目 step 步长 否 1
- Android实现主动连接蓝牙耳机
gqdy365
android
在Android程序中可以实现自动扫描蓝牙、配对蓝牙、建立数据通道。蓝牙分不同类型,这篇文字只讨论如何与蓝牙耳机连接。
大致可以分三步:
一、扫描蓝牙设备:
1、注册并监听广播:
BluetoothAdapter.ACTION_DISCOVERY_STARTED
BluetoothDevice.ACTION_FOUND
BluetoothAdapter.ACTION_DIS
- android学习轨迹之四:org.json.JSONException: No value for
hyz301
json
org.json.JSONException: No value for items
在JSON解析中会遇到一种错误,很常见的错误
06-21 12:19:08.714 2098-2127/com.jikexueyuan.secret I/System.out﹕ Result:{"status":1,"page":1,&
- 干货分享:从零开始学编程 系列汇总
justjavac
编程
程序员总爱重新发明轮子,于是做了要给轮子汇总。
从零开始写个编译器吧系列 (知乎专栏)
从零开始写一个简单的操作系统 (伯乐在线)
从零开始写JavaScript框架 (图灵社区)
从零开始写jQuery框架 (蓝色理想 )
从零开始nodejs系列文章 (粉丝日志)
从零开始编写网络游戏
- jquery-autocomplete 使用手册
macroli
jqueryAjax脚本
jquery-autocomplete学习
一、用前必备
官方网站:http://bassistance.de/jquery-plugins/jquery-plugin-autocomplete/
当前版本:1.1
需要JQuery版本:1.2.6
二、使用
<script src="./jquery-1.3.2.js" type="text/ja
- PLSQL-Developer或者Navicat等工具连接远程oracle数据库的详细配置以及数据库编码的修改
超声波
oracleplsql
在服务器上将Oracle安装好之后接下来要做的就是通过本地机器来远程连接服务器端的oracle数据库,常用的客户端连接工具就是PLSQL-Developer或者Navicat这些工具了。刚开始也是各种报错,什么TNS:no listener;TNS:lost connection;TNS:target hosts...花了一天的时间终于让PLSQL-Developer和Navicat等这些客户
- 数据仓库数据模型之:极限存储--历史拉链表
superlxw1234
极限存储数据仓库数据模型拉链历史表
在数据仓库的数据模型设计过程中,经常会遇到这样的需求:
1. 数据量比较大; 2. 表中的部分字段会被update,如用户的地址,产品的描述信息,订单的状态等等; 3. 需要查看某一个时间点或者时间段的历史快照信息,比如,查看某一个订单在历史某一个时间点的状态, 比如,查看某一个用户在过去某一段时间内,更新过几次等等; 4. 变化的比例和频率不是很大,比如,总共有10
- 10点睛Spring MVC4.1-全局异常处理
wiselyman
spring mvc
10.1 全局异常处理
使用@ControllerAdvice注解来实现全局异常处理;
使用@ControllerAdvice的属性缩小处理范围
10.2 演示
演示控制器
package com.wisely.web;
import org.springframework.stereotype.Controller;
import org.spring