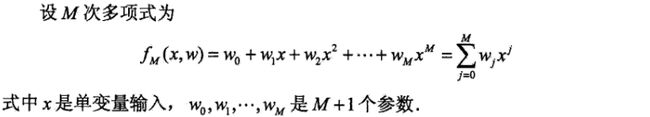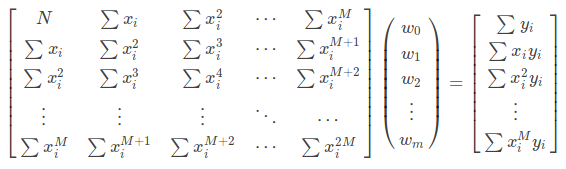- 小白零基础学数学建模系列-引言与课程目录
川川菜鸟
数学建模小白到精通系列数学建模
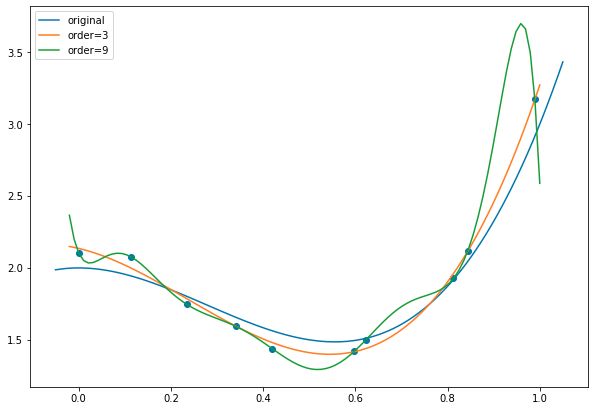
目录引言一、我们的专辑包含哪些内容?第一周:数学建模基础与工具第二周:高级数学建模技巧与应用第三周:机器学习基础与数据处理第四周:监督学习与无监督学习算法第五周:神经网络二、学完本专辑能收获到什么?三、适合什么样的人群学习?四、如何学习本专辑?课程目录第1周:数学建模基础与工具第1天:数学建模入门介绍第2天:数学建模工具介绍第3天:线性回归与曲线拟合第4天:线性规划第5天:动态规划第2周:高级数学
- 深入解析深度学习中的过拟合与欠拟合诊断、解决与工程实践
古月居GYH
深度学习人工智能
一、引言:模型泛化能力的核心挑战在深度学习模型开发中,欠拟合与过拟合是影响泛化能力的两个核心矛盾。据GoogleBrain研究统计,工业级深度学习项目中有63%的失败案例与这两个问题直接相关。本文将从基础概念到工程实践,系统解析其本质特征、诊断方法及解决方案,并辅以可复现的代码案例。二、核心概念与通熟易懂解释简单而言,欠拟合是指模型不能在训练集上获得足够低的误差。换句换说,就是模型复杂度低,模型在
- CSP-J备考冲刺必刷题(C++) | AcWing 1253 家谱
热爱编程的通信人
c++开发语言
本文分享的必刷题目是从蓝桥云课、洛谷、AcWing等知名刷题平台精心挑选而来,并结合各平台提供的算法标签和难度等级进行了系统分类。题目涵盖了从基础到进阶的多种算法和数据结构,旨在为不同阶段的编程学习者提供一条清晰、平稳的学习提升路径。欢迎大家订阅我的专栏:算法题解:C++与Python实现!附上汇总贴:算法竞赛备考冲刺必刷题(C++)|汇总【题目来源】Acwing:1253.家谱-AcWing题库
- 常见的应用对应的传输层协议和端口号
数据链路摸索者
网路安全网络
常见的应用对应的传输层协议和端口号如下:服务端口号协议http80TCPhttps443TCPFTP21(控制)TCP20(数据)TCPTFTP69UDPTelent23TCPSSH22TCPSMTP25TCPPOP3110TCPSNMP161UDPNTP123UDPDNS53TCP+UDPDHCP67和68UDPOracle数据库1521TCP终端服务3389TCP
- Python接口自动化
花落同学
Python自动化从入门到放弃python自动化
4接口自动化4.1使用python实现接口自动化如果不了解接口测试可参考https://ke.qq.com/course/4092904使用Python的request库实现接口测试:importjsonimportrequests#使用session管理:#1.可以自动关联set-cookie里面的内容#2.可以加快与服务器的连接速度session=requests.session()#auth
- python实现接口自动化
一只小H呀の
python自动化开发语言
代码实现自动化相关理论代码编写脚本和工具实现脚本区别是啥?代码:优点:代码灵活方便缺点:学习成本高工具:优点:易上手缺点:灵活度低,有局限性。总结:功能脚本:工具自动化脚本:代码代码接口自动化怎么做的?第一步:python+request+unittest;具体描述?第二步:封装、调用、数据驱动、日志、报告;详细举例:第三步:api\scripts\data\log\report\until…脚本
- 如何通过Python实现自动化任务:从入门到实践
小弟有话说1.0
python自动化开发语言
在当今快节奏的数字化时代,自动化技术正逐渐成为提高工作效率的利器。无论是处理重复性任务,还是管理复杂的工作流程,自动化都能为我们节省大量时间和精力。本文将以Python为例,带你从零开始学习如何实现自动化任务,并通过一个实际案例展示其强大功能。一、为什么选择Python实现自动化?Python作为一种简单易学、功能强大的编程语言,已经成为自动化领域的首选工具。以下是Python在自动化中的几大优势
- 用Python修改Word文档字体
在数字化办公场景中,Word文档作为主流文件格式承载着大量商务文书与学术资料。传统手动调整字体格式的操作模式存在显著局限性:当面对批量文档处理、动态内容生成或企业级模板维护时,逐一手工修改不仅效率低下,更难以保障格式规范的统一性。通过Python实现文档字体的程序化控制,能够有效构建自动化处理流程,在确保排版精准度的同时,显著提升文档批量化操作能力。本文将介绍如何使用Python修改Word文档段
- 源码篇:python生成《蔬菜店销售数据分析报告》案例
IT小本本
pythonpython数据分析开发语言
本文将通过Python实现一个完整的蔬菜销售数据分析项目,涵盖数据生成、清洗、分析及可视化全流程。我们将利用模拟数据生成技术创建90天的销售记录,通过Pandas进行数据处理,结合Matplotlib和Seaborn实现多样化的可视化图表,并最终生成动态交互报告。一、数据生成:模拟真实销售场景为了模拟真实的蔬菜销售数据,我们设计了包含10种蔬菜(白菜、土豆、西红柿等)的90天销售记录。数据生成逻辑
- CSP-J备考冲刺必刷题(C++) | AcWing 11 背包问题求方案数
热爱编程的通信人
c++算法开发语言
本文分享的必刷题目是从蓝桥云课、洛谷、AcWing等知名刷题平台精心挑选而来,并结合各平台提供的算法标签和难度等级进行了系统分类。题目涵盖了从基础到进阶的多种算法和数据结构,旨在为不同阶段的编程学习者提供一条清晰、平稳的学习提升路径。欢迎大家订阅我的专栏:算法题解:C++与Python实现!附上汇总贴:算法竞赛备考冲刺必刷题(C++)|汇总【题目来源】AcWing:11.背包问题求方案数-AcWi
- 用Python实现SFM
薄辉
pythonopencv计算机视觉人工智能图像处理
SFM(结构化光流法)是一种用于解决三维重建问题的方法,它可以根据许多二维图像和它们之间的相对位置,估计出三维场景的深度和摄像机的姿态。在Python中,你可以使用OpenCV库来实现SFM。下面是一个简单的例子,展示了如何使用OpenCV库的cv2.sfm_create函数来实现SFM:importcv2#读入图像,存入列表images中images=[]foriinrange(1,11):im
- 关于神经网络中的正则化
文弱_书生
乱七八糟神经网络人工智能深度学习
神经网络训练中的正则化正则化(Regularization)是神经网络训练中的一个关键技术,主要用于防止模型过拟合(overfitting),提高泛化能力。1.为什么需要正则化?在神经网络训练过程中,模型的目标是找到能在训练数据上表现良好的参数,同时也能泛化到未见过的数据。如果一个模型过于复杂(如参数过多、层数过深),它可能会记住训练数据中的噪声,而不是学习数据的本质模式。这种情况称为过拟合(ov
- 算法基础——蓝桥杯(python实现,实际上大多数用c++更明白易懂)(第一部分,共12个小题)
New_Teen
算法蓝桥杯python
1.成绩统计问题描述:编写一个程序,建立一个字典,每个字典包含姓名、学号、英语成绩、数学成绩和C++成绩,并通过字典操作平均分最高的学生和平均分最低的学生并且输出。输入格式:输入n+1行,第一行输入一个正整数n,表示学生数量;接下来的n行每行输入5个数据,分别表示姓名、学号、英语成绩、数学成绩和C++成绩。注意成绩有可能会有小数。输出格式:输出两行,第一行输出平均成绩最高的学生姓名。第二行输出平均
- 【机器学习】模型拟合
CH3_CH2_CHO
什么?!是机器学习!!机器学习人工智能欠拟合过拟合
1、欠拟合1.1现象欠拟合是机器学习和统计建模中的一种常见问题,表现为模型无法充分捕捉数据中的潜在规律和模式。无论是训练数据还是测试数据,模型的预测误差都居高不下。在实际应用中,欠拟合的模型往往显得过于简单和粗糙,无法对数据进行有效的拟合和描述。1.2原因模型过于简单是导致欠拟合的主要原因:例如,使用直线去拟合具有明显曲线趋势的数据,或者使用低阶多项式去拟合高阶的复杂函数关系。这种情况下,模型的表
- python自定义函数的参数有多种类型_Python实现自定义函数的5种常见形式分析
weixin_39632728
Python自定义函数是以def开头,空一格之后是这个自定义函数的名称,名称后面是一对括号,括号里放置形参列表,结束括号后面一定要有冒号“:”,函数的执行体程序代码也要有适当的缩排。Python自定义函数的通用语法是:def函数名称(形参列表):执行体程序代码Python自定义函数的5种常见形式:1、标准自定义函数:形参列表是标准的tuple数据类型>>>defabvedu_add(x,y):pr
- Python:区块链 Blockchain 入门的技术指南
拾荒的小海螺
Pythonpython区块链开发语言
1、简述区块链(Blockchain)是一种去中心化、不可篡改的分布式账本技术,最初因比特币而广为人知。如今,区块链已发展成为一种可以应用于金融、供应链管理、智能合约等多个领域的技术。本文将简要介绍区块链的基本概念和原理,并通过Python实现一个简化的区块链原型,帮助您快速上手区块链的实践。2、基本原理区块链是一种链式结构,由多个“区块”串联而成。每个区块中包含若干交易信息,并通过加密哈希指向前
- python实现一个通讯录,拥有添加联系人,删除联系人,修改联系人,查询联系人,查找通讯录,退出功能
新手懒羊哥
python开发语言
print('-'*25)#输出25个横杠print('-'*25)print("欢迎使用通讯录")print("1.添加联系人")print("2.查看通讯录")print("3.删除联系人")print("4.修改联系人")print("5.查找联系人")print("6.退出")print('-'*25)list1=[0]*10all_user=[]whileTrue:choose=inpu
- 用 Python 实现每秒百万级请求
weixin_33719619
python网络后端
本文讲的是用Python实现每秒百万级请求,用Python可以每秒发出百万个请求吗?这个问题终于有了肯定的回答。许多公司抛弃Python拥抱其他语言就为了提高性能节约服务器成本。但是没必要啊。Python也可以胜任。Python社区近来针对性能做了很多优化。CPython3.6新的字典实现方式提升了解释器的总体性能。得益于更快的调用约定和字典查询缓存,CPython3.7会更快。对于计算密集型工作
- 【数学建模】灰色关联分析模型详解与应用
烟锁池塘柳0
数学建模数学建模算法
灰色关联分析模型详解与应用文章目录灰色关联分析模型详解与应用引言灰色系统理论简介灰色关联分析基本原理灰色关联分析计算步骤1.确定分析序列2.数据无量纲化处理3.计算关联系数4.计算关联度灰色关联分析应用实例实例:某企业生产效率影响因素分析灰色关联分析在各领域的应用灰色关联分析的Python实现灰色关联分析的局限性结论引言在数据分析领域,我们经常面临样本量少、信息不完全、数据不确定性高的情况。传统的
- 人生重开模拟器 -deepseek版
Cccc吃吃吃
python开发语言
人生重开模拟器是一个有趣的文字类游戏,玩家可以通过选择不同的选项来体验不同的人生轨迹。下面是一个简单的Python实现,模拟了人生重开的过程。玩家可以通过输入数字来选择不同的选项,游戏会根据选择生成不同的人生结局。```pythonimportrandomdefprint_intro():print("欢迎来到人生重开模拟器!")print("你将重新开始你的人生,通过不同的选择体验不同的人生轨迹
- 量子密码学技术架构解析与程序员视角
算法
量子计算威胁模型分析传统公钥密码体系(RSA/ECC)的安全假设基于:大数分解问题的计算复杂度(RSA)椭圆曲线离散对数问题(ECC)有限域离散对数问题(DSA)Shor算法的时间复杂度为O((logN)^3),当量子比特数达到阈值时:2048位RSA可在8小时内破解(理论值)ECC-256的破解时间将降至多项式级别Grover算法对对称密码的影响:AES-256的有效安全性降至2^128哈希函数
- 神经网络基础之正则化
硬水果糖
人工智能神经网络人工智能机器学习
引言:正则化(Regularization)是机器学习中一种用于防止模型过拟合技术。核心思想是通过在模型损失函数中添加一个惩罚项(PenaltyTerm),对模型的复杂度进行约束,从而提升模型在新数据上的泛化能力。一、正则化目的防止过拟合:当模型过于复杂(例如神经网络层数过多、参数过多)时,容易在训练数据上“记忆”噪声或细节,导致在测试数据上表现差。简化模型:正则化通过限制模型参数的大小或数量,迫
- 推特关键词爬虫Python实现最新版(2025.2.20)
才华是浅浅的耐心
爬虫python开发语言
引言随着各类自媒体平台的兴起,数据挖掘和分析变得尤为重要。推特作为全球最大的自媒体平台,越来越来越多的人需要通过爬取其内容进行分析。然后自从马斯克接手推特之后,推特api不可再用,推特的反爬力度也在逐渐增强。今天小编就分享一个推特爬虫的教程。描述这篇文章主要通过关键词爬取帖子内容信息以及帖子作者主页相关信息,用户也可根据自己需要的时间段进行筛选。推特可支持筛选多种语言,我这里先展示中文和英文的。字
- 求解一次最佳平方逼近多项式
F_D_Z
数理数值分析一次最佳平方逼近多项式
例设f(x)=1+x2f(x)=\sqrt{1+x^2}f(x)=1+x2,求[0,1][0,1][0,1]上的一个一次最佳平方逼近多项式。解:d0=∫011+x2dx=12ln(1+2)+22≈1.147d_0=\int_{0}^{1}\sqrt{1+x^2}dx=\frac{1}{2}\ln(1+\sqrt{2})+\frac{\sqrt{2}}{2}\approx1.147d0=∫011+
- 勒让德多项式
F_D_Z
数理数值分析勒让德多项式Legendre
勒让德多项式(Legendre)当区间为[−1,1][-1,1][−1,1],权函数ρ(x)=1ρ(x)=1ρ(x)=1时,由1,x,...,xn,...{1,x,...,x^n,...}1,x,...,xn,...正交化得到的多项式称为勒让德多项式,并用P0(x),P1(x),...,Pn(x),...P_0(x),P_1(x),...,P_n(x),...P0(x),P1(x),...,Pn(
- Spring Boot 应用的接口访问从 HTTP 改为 HTTPS
day day day ...
httpspringboothttps
LINUX1.生成SSL证书使用工具(如`keytool`或`openssl`)生成SSL证书。以下以`keytool`为例:keytool-genkeypair-aliasmydomain-keyalgRSA-keysize2048-storetypePKCS12-keystorekeystore.p12-validity3650-输入密码并填写证书信息。-生成的`keystore.p12`文件
- 如何用Python批量将CSV文件编码转换为UTF-8并转为Excel格式?
字节王德发
pythonpythonexcel开发语言
在处理数据时,CSV文件格式常常用作数据的交换格式。不过,很多情况下我们会遇到编码问题,特别是当文件不是UTF-8编码时。为了更好地处理这些文件,可能需要将它们转换为UTF-8编码,并且将其转换为Excel格式,这样可以方便后续的数据分析和使用。今天就来聊聊如何用Python实现这一过程。准备工作:安装必要的库我们需要确保安装了所需的Python库。主要用到的库有pandas和openpyxl。p
- 数据增强:扩充数据集提升模型泛化能力
AI天才研究院
计算AI大模型企业级应用开发实战ChatGPT计算科学神经计算深度学习神经网络大数据人工智能大型语言模型AIAGILLMJavaPython架构设计AgentRPA
1.背景介绍1.1.数据增强的重要性在机器学习领域,模型的泛化能力至关重要。一个泛化能力强的模型能够在未见数据上表现良好,而过拟合的模型则会在训练数据上表现出色,但在新数据上表现糟糕。数据增强是一种有效提升模型泛化能力的技术,它通过对现有数据进行各种变换,人为地扩充数据集,从而增加训练数据的数量和多样性。1.2.数据增强的应用场景数据增强广泛应用于各种机器学习任务中,包括:图像识别:对图像进行旋转
- 数据增强:扩充数据集,提升模型的鲁棒性
AI天才研究院
DeepSeekR1&大数据AI人工智能大模型LLM大模型落地实战指南计算科学神经计算深度学习神经网络大数据人工智能大型语言模型AIAGILLMJavaPython架构设计AgentRPA
数据增强:扩充数据集,提升模型的鲁棒性1.背景介绍1.1数据集的重要性在机器学习和深度学习领域中,数据集是训练模型的基础。高质量的数据集对于构建准确、鲁棒的模型至关重要。然而,在现实世界中,获取大量高质量的数据通常是一个巨大的挑战。数据采集过程耗时耗力,而且成本高昂。此外,某些领域的数据存在隐私和安全问题,难以获取。1.2数据集不足的挑战当数据集规模有限时,模型很容易过拟合,无法很好地推广到新的、
- 机器学习之KMeans算法
知舟不叙
机器学习算法kmeans
文章目录引言1.KMeans算法简介2.KMeans算法的数学原理3.KMeans算法的步骤3.1初始化簇中心3.2分配数据点3.3更新簇中心3.4停止条件4.KMeans算法的优缺点4.1优点4.2缺点5.KMeans算法的应用场景5.1图像分割5.2市场细分5.3文档聚类5.4异常检测6.Python实现KMeans算法7.总结引言KMeans算法是机器学习中最经典的无监督学习算法之一,广泛应
- mondb入手
木zi_鸣
mongodb
windows 启动mongodb 编写bat文件,
mongod --dbpath D:\software\MongoDBDATA
mongod --help 查询各种配置
配置在mongob
打开批处理,即可启动,27017原生端口,shell操作监控端口 扩展28017,web端操作端口
启动配置文件配置,
数据更灵活
- 大型高并发高负载网站的系统架构
bijian1013
高并发负载均衡
扩展Web应用程序
一.概念
简单的来说,如果一个系统可扩展,那么你可以通过扩展来提供系统的性能。这代表着系统能够容纳更高的负载、更大的数据集,并且系统是可维护的。扩展和语言、某项具体的技术都是无关的。扩展可以分为两种:
1.
- DISPLAY变量和xhost(原创)
czmmiao
display
DISPLAY
在Linux/Unix类操作系统上, DISPLAY用来设置将图形显示到何处. 直接登陆图形界面或者登陆命令行界面后使用startx启动图形, DISPLAY环境变量将自动设置为:0:0, 此时可以打开终端, 输出图形程序的名称(比如xclock)来启动程序, 图形将显示在本地窗口上, 在终端上输入printenv查看当前环境变量, 输出结果中有如下内容:DISPLAY=:0.0
- 获取B/S客户端IP
周凡杨
java编程jspWeb浏览器
最近想写个B/S架构的聊天系统,因为以前做过C/S架构的QQ聊天系统,所以对于Socket通信编程只是一个巩固。对于C/S架构的聊天系统,由于存在客户端Java应用,所以直接在代码中获取客户端的IP,应用的方法为:
String ip = InetAddress.getLocalHost().getHostAddress();
然而对于WEB
- 浅谈类和对象
朱辉辉33
编程
类是对一类事物的总称,对象是描述一个物体的特征,类是对象的抽象。简单来说,类是抽象的,不占用内存,对象是具体的,
占用存储空间。
类是由属性和方法构成的,基本格式是public class 类名{
//定义属性
private/public 数据类型 属性名;
//定义方法
publ
- android activity与viewpager+fragment的生命周期问题
肆无忌惮_
viewpager
有一个Activity里面是ViewPager,ViewPager里面放了两个Fragment。
第一次进入这个Activity。开启了服务,并在onResume方法中绑定服务后,对Service进行了一定的初始化,其中调用了Fragment中的一个属性。
super.onResume();
bindService(intent, conn, BIND_AUTO_CREATE);
- base64Encode对图片进行编码
843977358
base64图片encoder
/**
* 对图片进行base64encoder编码
*
* @author mrZhang
* @param path
* @return
*/
public static String encodeImage(String path) {
BASE64Encoder encoder = null;
byte[] b = null;
I
- Request Header简介
aigo
servlet
当一个客户端(通常是浏览器)向Web服务器发送一个请求是,它要发送一个请求的命令行,一般是GET或POST命令,当发送POST命令时,它还必须向服务器发送一个叫“Content-Length”的请求头(Request Header) 用以指明请求数据的长度,除了Content-Length之外,它还可以向服务器发送其它一些Headers,如:
- HttpClient4.3 创建SSL协议的HttpClient对象
alleni123
httpclient爬虫ssl
public class HttpClientUtils
{
public static CloseableHttpClient createSSLClientDefault(CookieStore cookies){
SSLContext sslContext=null;
try
{
sslContext=new SSLContextBuilder().l
- java取反 -右移-左移-无符号右移的探讨
百合不是茶
位运算符 位移
取反:
在二进制中第一位,1表示符数,0表示正数
byte a = -1;
原码:10000001
反码:11111110
补码:11111111
//异或: 00000000
byte b = -2;
原码:10000010
反码:11111101
补码:11111110
//异或: 00000001
- java多线程join的作用与用法
bijian1013
java多线程
对于JAVA的join,JDK 是这样说的:join public final void join (long millis )throws InterruptedException Waits at most millis milliseconds for this thread to die. A timeout of 0 means t
- Java发送http请求(get 与post方法请求)
bijian1013
javaspring
PostRequest.java
package com.bijian.study;
import java.io.BufferedReader;
import java.io.DataOutputStream;
import java.io.IOException;
import java.io.InputStreamReader;
import java.net.HttpURL
- 【Struts2二】struts.xml中package下的action配置项默认值
bit1129
struts.xml
在第一部份,定义了struts.xml文件,如下所示:
<!DOCTYPE struts PUBLIC
"-//Apache Software Foundation//DTD Struts Configuration 2.3//EN"
"http://struts.apache.org/dtds/struts
- 【Kafka十三】Kafka Simple Consumer
bit1129
simple
代码中关于Host和Port是割裂开的,这会导致单机环境下的伪分布式Kafka集群环境下,这个例子没法运行。
实际情况是需要将host和port绑定到一起,
package kafka.examples.lowlevel;
import kafka.api.FetchRequest;
import kafka.api.FetchRequestBuilder;
impo
- nodejs学习api
ronin47
nodejs api
NodeJS基础 什么是NodeJS
JS是脚本语言,脚本语言都需要一个解析器才能运行。对于写在HTML页面里的JS,浏览器充当了解析器的角色。而对于需要独立运行的JS,NodeJS就是一个解析器。
每一种解析器都是一个运行环境,不但允许JS定义各种数据结构,进行各种计算,还允许JS使用运行环境提供的内置对象和方法做一些事情。例如运行在浏览器中的JS的用途是操作DOM,浏览器就提供了docum
- java-64.寻找第N个丑数
bylijinnan
java
public class UglyNumber {
/**
* 64.查找第N个丑数
具体思路可参考 [url] http://zhedahht.blog.163.com/blog/static/2541117420094245366965/[/url]
*
题目:我们把只包含因子
2、3和5的数称作丑数(Ugly Number)。例如6、8都是丑数,但14
- 二维数组(矩阵)对角线输出
bylijinnan
二维数组
/**
二维数组 对角线输出 两个方向
例如对于数组:
{ 1, 2, 3, 4 },
{ 5, 6, 7, 8 },
{ 9, 10, 11, 12 },
{ 13, 14, 15, 16 },
slash方向输出:
1
5 2
9 6 3
13 10 7 4
14 11 8
15 12
16
backslash输出:
4
3
- [JWFD开源工作流设计]工作流跳跃模式开发关键点(今日更新)
comsci
工作流
既然是做开源软件的,我们的宗旨就是给大家分享设计和代码,那么现在我就用很简单扼要的语言来透露这个跳跃模式的设计原理
大家如果用过JWFD的ARC-自动运行控制器,或者看过代码,应该知道在ARC算法模块中有一个函数叫做SAN(),这个函数就是ARC的核心控制器,要实现跳跃模式,在SAN函数中一定要对LN链表数据结构进行操作,首先写一段代码,把
- redis常见使用
cuityang
redis常见使用
redis 通常被认为是一个数据结构服务器,主要是因为其有着丰富的数据结构 strings、map、 list、sets、 sorted sets
引入jar包 jedis-2.1.0.jar (本文下方提供下载)
package redistest;
import redis.clients.jedis.Jedis;
public class Listtest
- 配置多个redis
dalan_123
redis
配置多个redis客户端
<?xml version="1.0" encoding="UTF-8"?><beans xmlns="http://www.springframework.org/schema/beans" xmlns:xsi=&quo
- attrib命令
dcj3sjt126com
attr
attrib指令用于修改文件的属性.文件的常见属性有:只读.存档.隐藏和系统.
只读属性是指文件只可以做读的操作.不能对文件进行写的操作.就是文件的写保护.
存档属性是用来标记文件改动的.即在上一次备份后文件有所改动.一些备份软件在备份的时候会只去备份带有存档属性的文件.
- Yii使用公共函数
dcj3sjt126com
yii
在网站项目中,没必要把公用的函数写成一个工具类,有时候面向过程其实更方便。 在入口文件index.php里添加 require_once('protected/function.php'); 即可对其引用,成为公用的函数集合。 function.php如下:
<?php /** * This is the shortcut to D
- linux 系统资源的查看(free、uname、uptime、netstat)
eksliang
netstatlinux unamelinux uptimelinux free
linux 系统资源的查看
转载请出自出处:http://eksliang.iteye.com/blog/2167081
http://eksliang.iteye.com 一、free查看内存的使用情况
语法如下:
free [-b][-k][-m][-g] [-t]
参数含义
-b:直接输入free时,显示的单位是kb我们可以使用b(bytes),m
- JAVA的位操作符
greemranqq
位运算JAVA位移<<>>>
最近几种进制,加上各种位操作符,发现都比较模糊,不能完全掌握,这里就再熟悉熟悉。
1.按位操作符 :
按位操作符是用来操作基本数据类型中的单个bit,即二进制位,会对两个参数执行布尔代数运算,获得结果。
与(&)运算:
1&1 = 1, 1&0 = 0, 0&0 &
- Web前段学习网站
ihuning
Web
Web前段学习网站
菜鸟学习:http://www.w3cschool.cc/
JQuery中文网:http://www.jquerycn.cn/
内存溢出:http://outofmemory.cn/#csdn.blog
http://www.icoolxue.com/
http://www.jikexue
- 强强联合:FluxBB 作者加盟 Flarum
justjavac
r
原文:FluxBB Joins Forces With Flarum作者:Toby Zerner译文:强强联合:FluxBB 作者加盟 Flarum译者:justjavac
FluxBB 是一个快速、轻量级论坛软件,它的开发者是一名德国的 PHP 天才 Franz Liedke。FluxBB 的下一个版本(2.0)将被完全重写,并已经开发了一段时间。FluxBB 看起来非常有前途的,
- java统计在线人数(session存储信息的)
macroli
javaWeb
这篇日志是我写的第三次了 前两次都发布失败!郁闷极了!
由于在web开发中常常用到这一部分所以在此记录一下,呵呵,就到备忘录了!
我对于登录信息时使用session存储的,所以我这里是通过实现HttpSessionAttributeListener这个接口完成的。
1、实现接口类,在web.xml文件中配置监听类,从而可以使该类完成其工作。
public class Ses
- bootstrp carousel初体验 快速构建图片播放
qiaolevip
每天进步一点点学习永无止境bootstrap纵观千象
img{
border: 1px solid white;
box-shadow: 2px 2px 12px #333;
_width: expression(this.width > 600 ? "600px" : this.width + "px");
_height: expression(this.width &
- SparkSQL读取HBase数据,通过自定义外部数据源
superlxw1234
sparksparksqlsparksql读取hbasesparksql外部数据源
关键字:SparkSQL读取HBase、SparkSQL自定义外部数据源
前面文章介绍了SparSQL通过Hive操作HBase表。
SparkSQL从1.2开始支持自定义外部数据源(External DataSource),这样就可以通过API接口来实现自己的外部数据源。这里基于Spark1.4.0,简单介绍SparkSQL自定义外部数据源,访
- Spring Boot 1.3.0.M1发布
wiselyman
spring boot
Spring Boot 1.3.0.M1于6.12日发布,现在可以从Spring milestone repository下载。这个版本是基于Spring Framework 4.2.0.RC1,并在Spring Boot 1.2之上提供了大量的新特性improvements and new features。主要包含以下:
1.提供一个新的sprin
![]() 方程得到
方程得到