- 数据结构:链表、栈、队列
小朱在敲代码
数据结构链表c语言ubuntuvscode
目录链表、栈、队列链表空间使用的区别链表类型单链表节点定义单链表示例双链表节点定义双链表使用栈(Stack)LIFO栈定义栈的使用中缀表达式转后缀表达式(逆波兰表达式)队列(queue)FIFO队列的定义队列的使用链表、栈、队列计算机科学中的数据结构是算法设计的基础。本文将详细介绍链表、栈和队列这三种常见的数据结构,并重点分析链表的各种形式及其操作方法。链表链表是一种线性数据结构,与数组不同的是,
- 经典数据结构-栈计算逆波兰表达式
翔山代码
算法数据结构
用栈计算逆波兰表达式的基本思路是:按顺序遍历整个表达式,若遇到操作数(假设都是二元运算符)则入栈;若遇到操作符(+-*/)连续弹出两个操作数并执行相应的运算,然后将其运算结果入栈。重复以上过程,直到表达式遍历完,栈内只剩下一个操作数时,那就是最终的运算结果,弹出打印即可。importjava.util.Stack;publicclassSolution17{publicstaticintevalu
- 表达式求值相关算法
hatlonely
实现对一个数学表达式的求值,例如:1+2*(3+4)这个表达式的值为15这个问题主要要分为如下几个步骤:语法分析:将字符串表达式转化为数字和操作符的token数组,['1','+','2','*','(','3','+','4',')']转逆波兰表达式:将中缀表达式转后缀表达式,['1','2','3','4','+','*','+']逆波兰表达式求值:15逆波兰表达式转二叉树:条件表达式中,二叉
- 【Leetcode】150. 逆波兰表达式求值
飞哥不鸽
leetcode150FunctionBiFunction
今天做了一道leetcode,题目不算难,基本就是stack的入栈出栈,但我看了一圈别人的代码,感觉不够优雅,所以我想分享一下自己的写法,主要就是用Map和BiFunction优化4个ifelseJava中,函数是能以对象的形式表达。例如(Integera)->{returna+1;},这就是个自增的函数,我们可以将它赋值给FunctionincreateFunc,也就是Functionincre
- 代码随想录算法训练营第11天|● 20. 有效的括号● 1047. 删除字符串中的所有相邻重复项● 150. 逆波兰表达式求值
strive340
数据结构开发语言
20.有效的括号思路:栈的经典应用,利用栈的先进后出特性,当遇到左括号,则把相应的右括号压入栈中,遇到右括号,再把栈顶元素pop出来对比即刻.但是要分清楚3种边界情况。第一种:遍历过程中,栈中提出的右括号和当前的左括号不匹配,则错误第二种:遍历过程中,当遇到右括号,但是栈中元素是空的,则说明没有相应的左括号,错误.因为每压入栈的右括号,都是因为遍历到了左括号,可以把栈看作一个存入期望括号的栈,比如
- 代码随想录算法训练营第10天—栈和队列02 | ● 20. 有效的括号 ● 1047. 删除字符串中的所有相邻重复项 ● 150. 逆波兰表达式求值
日拱一卒的吾
Leetcode代码随想录(Python)算法python
20.有效的括号题目链接/文章讲解/视频讲解:https://programmercarl.com/0020.%E6%9C%89%E6%95%88%E7%9A%84%E6%8B%AC%E5%8F%B7.html考点栈我的思路利用标志位记录遇到了什么字符,遇到左括号加1,右括号减1,最后判断结果是否为0这种思路解决不了先出现右括号,再出现左括号的情况(需要额外添加逻辑判断)视频讲解关键点总结使用栈结
- [C++13]:stack && queue && priority_queue 模拟实现
随风飘扬@
c++开发语言算法模拟
stack&&queue&&priority_queue模拟实现一.stack1.概念:2.使用:3.模拟实现:一些题目:1.最小栈:2.栈的压入弹出序列:3.逆波兰表达式求值:二.queue1.概念:2.使用:3.模拟实现:一个题目:1.层序遍历:GIF解析三.priority_queue1.概念:2.一个题目:思路一:建堆+堆元素删除思路二:优化3.模拟实现:4.仿函数的应用:1.priori
- 算法学习——LeetCode力扣栈与队列篇2
拉依达不拉胯
LeetCode算法学习算法学习leetcodec++c语言
算法学习——LeetCode力扣栈与队列篇2150.逆波兰表达式求值150.逆波兰表达式求值-力扣(LeetCode)描述给你一个字符串数组tokens,表示一个根据逆波兰表示法表示的算术表达式。请你计算该表达式。返回一个表示表达式值的整数。注意:有效的算符为‘+’、‘-’、‘*’和‘/’。每个操作数(运算对象)都可以是一个整数或者另一个表达式。两个整数之间的除法总是向零截断。表达式中不含除零运算
- 《剑指 Offer》专项突破版 - 面试题 36 : 详解后缀表达式(C++ 实现)
melonyzzZ
数据结构c++开发语言数据结构面试leetcode
题目链接:LCR036.逆波兰表达式求值-力扣(LeetCode)题目:后缀表达式是一种算术表达式,它的操作符在操作数的后面。输入一个用字符串数组表示的后缀表达式,请输出该后缀表达式的计算结果。假设输入的一定是有效的后缀表达式。例如,后缀表达式["2","1","3","*","+"]对应的算术表达式是"2+1*3",因此输出它的计算结果5。分析:后缀表达式又叫逆波兰表达式(ReversePoli
- DAY11之有效的括号、删除字符串中的所有相邻重复项和逆波兰表达式求值
佳佳1515
算法
有效的括号20.有效的括号-力扣(LeetCode)由于栈结构的特殊性,非常适合做对称匹配类的题目。首先要弄清楚,字符串里的括号不匹配有几种情况。一些同学,在面试中看到这种题目上来就开始写代码,然后就越写越乱。建议在写代码之前要分析好有哪几种不匹配的情况,如果不在动手之前分析好,写出的代码也会有很多问题。先来分析一下这里有三种不匹配的情况,第一种情况,字符串里左方向的括号多余了,所以不匹配。第二种
- [源码和报告分享]基于C++的表达式计算求值
ggdd5151
功能要求为了实现表达式求值,本项目要求首先读入表达式(包括括号)并创建对应二叉树,其次对二叉树进行前序遍历,中序遍历,后序遍历,输出对应的波兰表达式,中缀表达式和逆波兰表达式。1参考博文和源码下载地址:https://write-bug.com/article/1400.html
- 代码随想录算法训练营day11|| 20. 有效的括号、1047. 删除字符串中的所有相邻重复项、150. 逆波兰表达式求值
轻舟未过万重山ing
代码随想录算法
栈与队列part02:20.有效的括号思路:由于栈结构的特殊性,非常适合做对称匹配类的题目。首先要弄清楚,字符串里的括号不匹配有几种情况。一些同学,在面试中看到这种题目上来就开始写代码,然后就越写越乱。建议在写代码之前要分析好有哪几种不匹配的情况,如果不在动手之前分析好,写出的代码也会有很多问题。先来分析一下这里有三种不匹配的情况,第一种情况,字符串里左方向的括号多余了,所以不匹配。2.第二种情况
- 【栈】【字符串和int类型转化】Leetcode 150 逆波兰表达式求值
苹果就叫不呐呐
Leetcodeleetcode算法java职场和发展程序人生
【栈】【字符串和int类型转化】Leetcode150逆波兰表达式求值解法1栈---------------题目链接Leetcode150逆波兰表达式求值-------------------解法1栈字符串转化为int类型数据:Integer.parseInt(s)Long.parseLong(s)Double.parseDouble(s)判断字符串相等s1.equals(s2)创建栈Stack(
- 「数据结构」栈:题解
Ice_Sugar_7
Java数据结构数据结构java开发语言
个人主页:Ice_Sugar_7所属专栏:Java数据结构欢迎点赞收藏加关注哦!栈简介栈的常用方法栈的应用逆波兰表达式求值出栈入栈顺序匹配最小栈问题简介栈是一种特殊的线性表,它只允许在固定的一端进行插入和删除元素操作概念区分栈、虚拟机栈、栈帧有什么区别?栈是一种数据结构虚拟机栈是JVM划分的一块内存栈帧是调用方法时,在虚拟机中给这个方法开辟的一块内存因为它的结构比较简单,所以本文中我们就不专门去实
- 150. 逆波兰表达式求值
ToxicantC
leetcode数据结构算法
给你一个字符串数组tokens,表示一个根据逆波兰表示法表示的算术表达式。请你计算该表达式。返回一个表示表达式值的整数。注意:有效的算符为'+'、'-'、'*'和'/'。每个操作数(运算对象)都可以是一个整数或者另一个表达式。两个整数之间的除法总是向零截断。表达式中不含除零运算。输入是一个根据逆波兰表示法表示的算术表达式。答案及所有中间计算结果可以用32位整数表示。示例1:输入:tokens=["
- 代码随想录算法训练营第十一天|● 20. 有效的括号 ● 1047. 删除字符串中的所有相邻重复项 ● 150. 逆波兰表达式求值
一枚清澈愚蠢的研究生
letcode算法
仅做学习笔记,详细请访问代码随想录栈提供push和pop等等接口,所有元素必须符合先进后出规则,所以栈不提供走访功能,也不提供迭代器(iterator)。不像是set或者map提供迭代器iterator来遍历所有元素栈是以底层容器完成其所有的工作,对外提供统一的接口,底层容器是可插拔的(也就是说我们可以控制使用哪种容器来实现栈的功能)。所以STL中栈往往不被归类为容器,而被归类为container
- 代码随想录算法训练营第十一天|20. 有效的括号、1047. 删除字符串中的所有相邻重复项、150. 逆波兰表达式求值
walkerLing
算法leetcodejava数据结构
20.有效的括号刷题https://leetcode.cn/problems/valid-parentheses/description/文章讲解https://programmercarl.com/0020.%E6%9C%89%E6%95%88%E7%9A%84%E6%8B%AC%E5%8F%B7.html#%E7%AE%97%E6%B3%95%E5%85%AC%E5%BC%80%E8%AF%B
- day11算法补卡|栈与队列02|Leetcode20有效括号、1047删除字符串中的所有相邻重复项 、150逆波兰表达式求值
lala....
算法leetcode
Leetcode20:有效括号题目链接:https://leetcode.cn/problems/valid-parentheses/description/题目分析:使用栈实现,如果栈为空,直接入栈;如果栈不为空且栈顶元素与即将遍历字符匹配,则对应栈顶元素出栈;如不匹配,则对元素直接入栈Java实现代码:importjava.util.Stack;//leetcodesubmitregionbe
- 代码随想录算法训练营第十一天:20. 有效的括号● 1047. 删除字符串中的所有相邻重复项● 150. 逆波兰表达式求值
孤馆深沉
算法
20.有效的括号括号匹配三种错误情况:左右括号类型不匹配;右括号比左括号多,右括号会遇到空栈;左括号比右括号多,多的左括号会留在栈内。classSolution{public:stackst;boolisValid(strings){for(inti=0;i='0'&&tokens[i][0]<='9')||(tokens[i][0]=='-')){ss.push(stoll(tokens[i])
- 随想录刷题笔记 —栈与队列篇2 20有效括号 1047删除字符串相邻重复项 150逆波兰表达式求值
XWHQQ7426
随想录刷题笔记算法java
20有效括号给定一个只包括'(',')','{','}','[',']'的字符串s,判断字符串是否有效使用栈来做括号匹配,如果是左括号则入栈,如果是右括号则出栈,在遇到右括号出栈时比较括号类型是否相同即可。classSolution{publicbooleanisValid(Strings){Stackstack=newStackstack=newStackstack=newStack<>();f
- 数据结构与算法学习01之前缀表达式(波兰表达式)、中缀表达式、后缀表达式(逆波兰表达式)
zh成长之路
数据结构算法java堆栈
前缀表达式(波兰表达式)概念:前缀表达式的运算符位于操作符之前举例:(3+4)*5-6,对应的前缀表达式就是-*+3456前缀表达式的计算机求值:从右至左扫描表达式,遇到数字时,将数字压入堆栈,遇到运算符时,弹出栈顶的两个数,用运算付对它们做相应的计算(栈顶元素和次顶元素),并将结果入栈:重复上述过程知道表达式最左端,最后运算得出的值即为表达式的结果。例如:(3+4)*5-6对应的前缀表达式就是-
- 数据结构----栈的概念、模拟实现、栈的使用、栈的应用、有关栈的算法题
码农小张~
数据结构数据结构算法
文章目录1.栈(Stack)的概念2.栈的模拟实现3.栈的使用4.栈的应用场景4.1.改变元素的序列4.2.将递归转化为循环4.3.150.逆波兰表达式求值4.4.20.括号匹配4.5JZ31栈的压入、弹出序列4.6155.最小的栈5.概念区分1.栈(Stack)的概念栈:一种特殊的线性表,其只允许在固定的一端进行插入和删除元素操作。进行数据插入和删除操作的一端称为栈顶,另一端称为栈底。栈中的数据
- Leetcode的AC指南 —— 栈与队列 :150. 逆波兰表达式求值
知之_知之
leetcode的AC指南leetcode算法go
摘要:**Leetcode的AC指南——栈与队列:150.逆波兰表达式求值**。题目介绍:给你一个字符串数组tokens,表示一个根据逆波兰表示法表示的算术表达式。请你计算该表达式。返回一个表示表达式值的整数。文章目录一、题目**进阶:**二、解析(go语言版)1、栈三、其他语言版本JavaPythonC++一、题目题目介绍:给你一个字符串数组tokens,表示一个根据逆波兰表示法表示的算术表达式
- 汪都能理解的逆波兰计算器(C++实现)
vernzhao
简介EXPLANATION逆波兰表示法(ReversePolishnotation,RPN)也称作后缀表示法,与之对应的是波兰表示法(Polishnotation),也就是前缀表示法。之所以使用“波兰”来命名,是因为发明者的名字太难发音logicianJanŁukasiewicz,只好以国籍来命名。实际意义由于逆波兰表达式在计算时不需要将表达式括起来,进行计算时输入较少。此外,用户在使用时也可以更
- 算法day10
TOMOT77
算法golang数据结构
算法day1020有效的括号1047删除字符串中的所有相邻重复性150逆波兰表达式求值20有效的括号拿到这个题的想法,首先我在想我能不能用数组的操作来扫描做。后来想想,如果这样做那特判也太多了,不好做。然后第二个想法就是用栈来做,我之前看过有类似的算法题,但是具体细节怎样我忘了。这里我就之间看题解学习了。看完解题我回来了:括号匹配时使用栈解决的经典问题,题意要求:就是和我们平时写代码的顺序一样,有
- 力扣150 逆波兰表达式求值 Java版本
蓑 羽
LeetCodeleetcodejavapython
题目描述给你一个字符串数组tokens,表示一个根据逆波兰表示法表示的算术表达式。请你计算该表达式。返回一个表示表达式值的整数。注意:有效的算符为‘+’、‘-’、‘*’和‘/’。每个操作数(运算对象)都可以是一个整数或者另一个表达式。两个整数之间的除法总是向零截断。表达式中不含除零运算。输入是一个根据逆波兰表示法表示的算术表达式。答案及所有中间计算结果可以用32位整数表示。示例1:输入:token
- 栈和队列-逆波兰表达式求值
hema12138
数据结构和算法leetcode算法
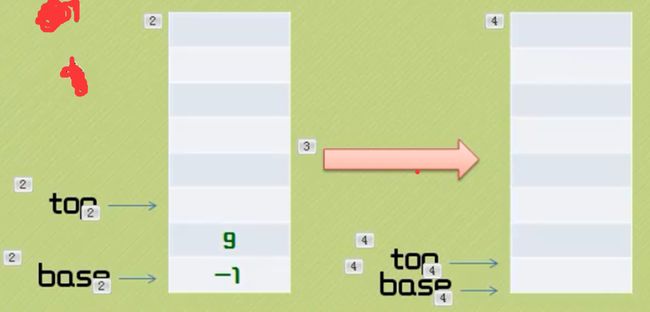
Problem:150.逆波兰表达式求值文章目录题目思路复杂度Code题目输入一个根据逆波兰表达式表示的四则运算表达式,输出计算结果。注意除法是向零取整。示例1:输入:tokens=[“2”,“1”,“+”,“3”,““];输出:9示例2:输入:tokens=[“10”,“6”,“9”,“3”,”+“,”-11","”,“/”,“*”,“17”,“+”,“5”,“+”];输出:22思路逆波兰表达式
- 表达式求值问题数据结构课程设计
WHAT'S YOUR INSIGHT?
c++数据结构c++栈算法二叉树
完整代码在最后~~1需求分析1.1问题描述表达式求值是程序设计语言编译中的一个最基本问题,就是将一个表达式转化为逆波兰表达式并求值。具体要求是以字符序列的形式从终端输入语法正确的、不含变量的整数表达式,并利用给定的优先关系实现对算术四则混合表达式的求值,并演示在求值过程中运算符栈、操作数栈、输入字符和主要操作变化过程。要把一个表达式翻译成正确求值的一个机器指令序列,或者直接对表达式求值,首先要能正
- 算法练习-逆波兰表达式求值(思路+流程图+代码)
Yamai Yuzuru
算法编程笔记java算法数据结构leetcode
难度参考难度:中等分类:栈与队列难度与分类由我所参与的培训课程提供,但需要注意的是,难度与分类仅供参考。且所在课程未提供测试平台,故实现代码主要为自行测试的那种,以下内容均为个人笔记,旨在督促自己认真学习。题目根据逆波兰表示法,求表达式的值。有效的运算符包括+,·,*,/。每个运算对像可以是整数,也可以是另一个逆波兰表达式。说明:整数除法只保留整数部分。给定逆波兰表达式总是有效的。换句话说,表达式
- 代码随想录算法训练营第十一天|20.有效的括号、1047.删除字符串中的所有相邻重复项、150.逆波兰表达式求值
萌帅帅小明
算法leetcodec#数据结构
20.有效的括号publicclassSolution{publicboolIsValid(strings){Stacka=newStack();for(inti=0;is1=newStack();Stackns=newStack();char[]c=s.ToCharArray();stringans="";for(inti=0;i=1){if(c[i]==s1.Peek()){s1.Pop();
- 戴尔笔记本win8系统改装win7系统
sophia天雪
win7戴尔改装系统win8
戴尔win8 系统改装win7 系统详述
第一步:使用U盘制作虚拟光驱:
1)下载安装UltraISO:注册码可以在网上搜索。
2)启动UltraISO,点击“文件”—》“打开”按钮,打开已经准备好的ISO镜像文
- BeanUtils.copyProperties使用笔记
bylijinnan
java
BeanUtils.copyProperties VS PropertyUtils.copyProperties
两者最大的区别是:
BeanUtils.copyProperties会进行类型转换,而PropertyUtils.copyProperties不会。
既然进行了类型转换,那BeanUtils.copyProperties的速度比不上PropertyUtils.copyProp
- MyEclipse中文乱码问题
0624chenhong
MyEclipse
一、设置新建常见文件的默认编码格式,也就是文件保存的格式。
在不对MyEclipse进行设置的时候,默认保存文件的编码,一般跟简体中文操作系统(如windows2000,windowsXP)的编码一致,即GBK。
在简体中文系统下,ANSI 编码代表 GBK编码;在日文操作系统下,ANSI 编码代表 JIS 编码。
Window-->Preferences-->General -
- 发送邮件
不懂事的小屁孩
send email
import org.apache.commons.mail.EmailAttachment;
import org.apache.commons.mail.EmailException;
import org.apache.commons.mail.HtmlEmail;
import org.apache.commons.mail.MultiPartEmail;
- 动画合集
换个号韩国红果果
htmlcss
动画 指一种样式变为另一种样式 keyframes应当始终定义0 100 过程
1 transition 制作鼠标滑过图片时的放大效果
css
.wrap{
width: 340px;height: 340px;
position: absolute;
top: 30%;
left: 20%;
overflow: hidden;
bor
- 网络最常见的攻击方式竟然是SQL注入
蓝儿唯美
sql注入
NTT研究表明,尽管SQL注入(SQLi)型攻击记录详尽且为人熟知,但目前网络应用程序仍然是SQLi攻击的重灾区。
信息安全和风险管理公司NTTCom Security发布的《2015全球智能威胁风险报告》表明,目前黑客攻击网络应用程序方式中最流行的,要数SQLi攻击。报告对去年发生的60亿攻击 行为进行分析,指出SQLi攻击是最常见的网络应用程序攻击方式。全球网络应用程序攻击中,SQLi攻击占
- java笔记2
a-john
java
类的封装:
1,java中,对象就是一个封装体。封装是把对象的属性和服务结合成一个独立的的单位。并尽可能隐藏对象的内部细节(尤其是私有数据)
2,目的:使对象以外的部分不能随意存取对象的内部数据(如属性),从而使软件错误能够局部化,减少差错和排错的难度。
3,简单来说,“隐藏属性、方法或实现细节的过程”称为——封装。
4,封装的特性:
4.1设置
- [Andengine]Error:can't creat bitmap form path “gfx/xxx.xxx”
aijuans
学习Android遇到的错误
最开始遇到这个错误是很早以前了,以前也没注意,只当是一个不理解的bug,因为所有的texture,textureregion都没有问题,但是就是提示错误。
昨天和美工要图片,本来是要背景透明的png格式,可是她却给了我一个jpg的。说明了之后她说没法改,因为没有png这个保存选项。
我就看了一下,和她要了psd的文件,还好我有一点
- 自己写的一个繁体到简体的转换程序
asialee
java转换繁体filter简体
今天调研一个任务,基于java的filter实现繁体到简体的转换,于是写了一个demo,给各位博友奉上,欢迎批评指正。
实现的思路是重载request的调取参数的几个方法,然后做下转换。
- android意图和意图监听器技术
百合不是茶
android显示意图隐式意图意图监听器
Intent是在activity之间传递数据;Intent的传递分为显示传递和隐式传递
显式意图:调用Intent.setComponent() 或 Intent.setClassName() 或 Intent.setClass()方法明确指定了组件名的Intent为显式意图,显式意图明确指定了Intent应该传递给哪个组件。
隐式意图;不指明调用的名称,根据设
- spring3中新增的@value注解
bijian1013
javaspring@Value
在spring 3.0中,可以通过使用@value,对一些如xxx.properties文件中的文件,进行键值对的注入,例子如下:
1.首先在applicationContext.xml中加入:
<beans xmlns="http://www.springframework.
- Jboss启用CXF日志
sunjing
logjbossCXF
1. 在standalone.xml配置文件中添加system-properties:
<system-properties> <property name="org.apache.cxf.logging.enabled" value=&
- 【Hadoop三】Centos7_x86_64部署Hadoop集群之编译Hadoop源代码
bit1129
centos
编译必需的软件
Firebugs3.0.0
Maven3.2.3
Ant
JDK1.7.0_67
protobuf-2.5.0
Hadoop 2.5.2源码包
Firebugs3.0.0
http://sourceforge.jp/projects/sfnet_findbug
- struts2验证框架的使用和扩展
白糖_
框架xmlbeanstruts正则表达式
struts2能够对前台提交的表单数据进行输入有效性校验,通常有两种方式:
1、在Action类中通过validatexx方法验证,这种方式很简单,在此不再赘述;
2、通过编写xx-validation.xml文件执行表单验证,当用户提交表单请求后,struts会优先执行xml文件,如果校验不通过是不会让请求访问指定action的。
本文介绍一下struts2通过xml文件进行校验的方法并说
- 记录-感悟
braveCS
感悟
再翻翻以前写的感悟,有时会发现自己很幼稚,也会让自己找回初心。
2015-1-11 1. 能在工作之余学习感兴趣的东西已经很幸福了;
2. 要改变自己,不能这样一直在原来区域,要突破安全区舒适区,才能提高自己,往好的方面发展;
3. 多反省多思考;要会用工具,而不是变成工具的奴隶;
4. 一天内集中一个定长时间段看最新资讯和偏流式博
- 编程之美-数组中最长递增子序列
bylijinnan
编程之美
import java.util.Arrays;
import java.util.Random;
public class LongestAccendingSubSequence {
/**
* 编程之美 数组中最长递增子序列
* 书上的解法容易理解
* 另一方法书上没有提到的是,可以将数组排序(由小到大)得到新的数组,
* 然后求排序后的数组与原数
- 读书笔记5
chengxuyuancsdn
重复提交struts2的token验证
1、重复提交
2、struts2的token验证
3、用response返回xml时的注意
1、重复提交
(1)应用场景
(1-1)点击提交按钮两次。
(1-2)使用浏览器后退按钮重复之前的操作,导致重复提交表单。
(1-3)刷新页面
(1-4)使用浏览器历史记录重复提交表单。
(1-5)浏览器重复的 HTTP 请求。
(2)解决方法
(2-1)禁掉提交按钮
(2-2)
- [时空与探索]全球联合进行第二次费城实验的可能性
comsci
二次世界大战前后,由爱因斯坦参加的一次在海军舰艇上进行的物理学实验 -费城实验
至今给我们大家留下很多迷团.....
关于费城实验的详细过程,大家可以在网络上搜索一下,我这里就不详细描述了
在这里,我的意思是,现在
- easy connect 之 ORA-12154: TNS: 无法解析指定的连接标识符
daizj
oracleORA-12154
用easy connect连接出现“tns无法解析指定的连接标示符”的错误,如下:
C:\Users\Administrator>sqlplus username/
[email protected]:1521/orcl
SQL*Plus: Release 10.2.0.1.0 – Production on 星期一 5月 21 18:16:20 2012
Copyright (c) 198
- 简单排序:归并排序
dieslrae
归并排序
public void mergeSort(int[] array){
int temp = array.length/2;
if(temp == 0){
return;
}
int[] a = new int[temp];
int
- C语言中字符串的\0和空格
dcj3sjt126com
c
\0 为字符串结束符,比如说:
abcd (空格)cdefg;
存入数组时,空格作为一个字符占有一个字节的空间,我们
- 解决Composer国内速度慢的办法
dcj3sjt126com
Composer
用法:
有两种方式启用本镜像服务:
1 将以下配置信息添加到 Composer 的配置文件 config.json 中(系统全局配置)。见“例1”
2 将以下配置信息添加到你的项目的 composer.json 文件中(针对单个项目配置)。见“例2”
为了避免安装包的时候都要执行两次查询,切记要添加禁用 packagist 的设置,如下 1 2 3 4 5
- 高效可伸缩的结果缓存
shuizhaosi888
高效可伸缩的结果缓存
/**
* 要执行的算法,返回结果v
*/
public interface Computable<A, V> {
public V comput(final A arg);
}
/**
* 用于缓存数据
*/
public class Memoizer<A, V> implements Computable<A,
- 三点定位的算法
haoningabc
c算法
三点定位,
已知a,b,c三个顶点的x,y坐标
和三个点都z坐标的距离,la,lb,lc
求z点的坐标
原理就是围绕a,b,c 三个点画圆,三个圆焦点的部分就是所求
但是,由于三个点的距离可能不准,不一定会有结果,
所以是三个圆环的焦点,环的宽度开始为0,没有取到则加1
运行
gcc -lm test.c
test.c代码如下
#include "stdi
- epoll使用详解
jimmee
clinux服务端编程epoll
epoll - I/O event notification facility在linux的网络编程中,很长的时间都在使用select来做事件触发。在linux新的内核中,有了一种替换它的机制,就是epoll。相比于select,epoll最大的好处在于它不会随着监听fd数目的增长而降低效率。因为在内核中的select实现中,它是采用轮询来处理的,轮询的fd数目越多,自然耗时越多。并且,在linu
- Hibernate对Enum的映射的基本使用方法
linzx0212
enumHibernate
枚举
/**
* 性别枚举
*/
public enum Gender {
MALE(0), FEMALE(1), OTHER(2);
private Gender(int i) {
this.i = i;
}
private int i;
public int getI
- 第10章 高级事件(下)
onestopweb
事件
index.html
<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://www.w3.org/TR/xhtml1/DTD/xhtml1-transitional.dtd">
<html xmlns="http://www.w3.org/
- 孙子兵法
roadrunners
孙子兵法
始计第一
孙子曰:
兵者,国之大事,死生之地,存亡之道,不可不察也。
故经之以五事,校之以计,而索其情:一曰道,二曰天,三曰地,四曰将,五
曰法。道者,令民于上同意,可与之死,可与之生,而不危也;天者,阴阳、寒暑
、时制也;地者,远近、险易、广狭、死生也;将者,智、信、仁、勇、严也;法
者,曲制、官道、主用也。凡此五者,将莫不闻,知之者胜,不知之者不胜。故校
之以计,而索其情,曰
- MySQL双向复制
tomcat_oracle
mysql
本文包括:
主机配置
从机配置
建立主-从复制
建立双向复制
背景
按照以下简单的步骤:
参考一下:
在机器A配置主机(192.168.1.30)
在机器B配置从机(192.168.1.29)
我们可以使用下面的步骤来实现这一点
步骤1:机器A设置主机
在主机中打开配置文件 ,
- zoj 3822 Domination(dp)
阿尔萨斯
Mina
题目链接:zoj 3822 Domination
题目大意:给定一个N∗M的棋盘,每次任选一个位置放置一枚棋子,直到每行每列上都至少有一枚棋子,问放置棋子个数的期望。
解题思路:大白书上概率那一张有一道类似的题目,但是因为时间比较久了,还是稍微想了一下。dp[i][j][k]表示i行j列上均有至少一枚棋子,并且消耗k步的概率(k≤i∗j),因为放置在i+1~n上等价与放在i+1行上,同理