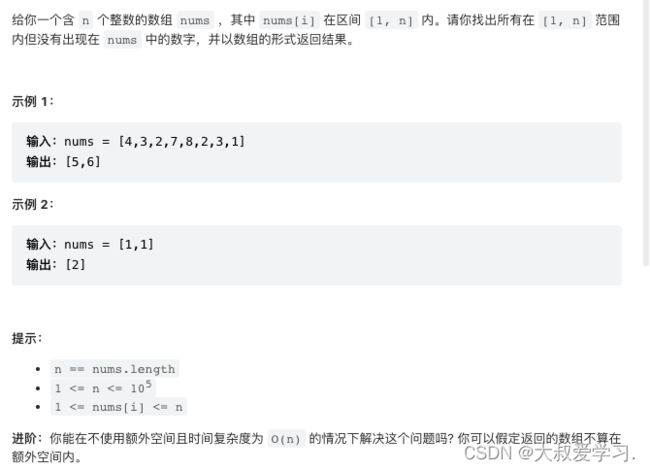
Leetcode 算法面试冲刺 热题 HOT 100 刷题（406 416 437 438 448）（六十九）

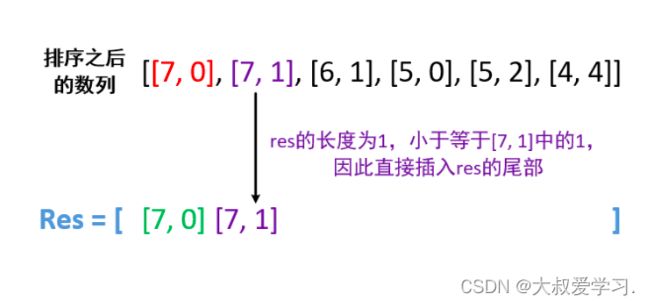
406. 根据身高重建队列

一句话理解：就是先把这些人按照个头，从大到小排列，然后我们再按照第2个数进行index插入。原理就是，比如第3个人要插入队列时，前面已经排好的2个人，身高都大于等于他。他只插在index位置，就是前面有几个人比他高。

class Solution:
    def reconstructQueue(self, people: List[List[int]]) -> List[List[int]]:
        res = []
        people = sorted(people, key = lambda x: (-x[0], x[1]))
        for p in people:
            if len(res) <= p[1]:
                res.append(p)
            elif len(res) > p[1]:
                res.insert(p[1], p)
        return res

下面是我写的代码：

class Solution:
    def reconstructQueue(self, people: List[List[int]]) -> List[List[int]]:
        # 根据第一个1元素的负数，当第一个元素负数相等，根据第二个元素正数排序
        people.sort(key = lambda x : (-x[0], x[1]))
        res = []
        for p, ind in people:
            if len(res) < ind:
                res.append([p, ind])
            else:
                res.insert(ind, [p, ind])
        return res

416. 分割等和子集

437. 路径总和 III

知道要用回溯，但是具体写不出来，一个是root不一定是定点，而是每一个点都可能是root。这里没想明白。

# Definition for a binary tree node.
# class TreeNode:
#     def __init__(self, val=0, left=None, right=None):
#         self.val = val
#         self.left = left
#         self.right = right
class Solution:
    def pathSum(self, root: Optional[TreeNode], targetSum: int) -> int:
        ps = []
        cnt = 0
        def backtrack(root, ps, cnt):
            if not root: return 0
            if sum(ps) == targetSum:
                cnt += 1
                ps.pop()
                return
            ps.append(root.val)
            backtrack(root.left, ps, cnt)
            backtrack(root.right, ps, cnt)
        backtrack(root, ps, cnt)
        return cnt

class Solution:
    def pathSum(self, root: TreeNode, targetSum: int) -> int:
        def rootSum(root, targetSum):
            if root is None:
                return 0

            ret = 0
            if root.val == targetSum:
                ret += 1

            ret += rootSum(root.left, targetSum - root.val)
            ret += rootSum(root.right, targetSum - root.val)
            return ret
        
        if root is None:
            return 0
            
        ret = rootSum(root, targetSum)
        ret += self.pathSum(root.left, targetSum)
        ret += self.pathSum(root.right, targetSum)
        return ret

下面是我写的：

# Definition for a binary tree node.
# class TreeNode:
#     def __init__(self, val=0, left=None, right=None):
#         self.val = val
#         self.left = left
#         self.right = right
class Solution:
    def pathSum(self, root: Optional[TreeNode], targetSum: int) -> int:
        
        def helper(root, targetSum):
            if not root: return 0
            res = 0
            if root.val == targetSum:
                res += 1
            res += helper(root.left, targetSum - root.val)
            res += helper(root.right, targetSum - root.val)
            return res

        if not root: return 0
        res = helper(root, targetSum)
        res += self.pathSum(root.left, targetSum)
        res += self.pathSum(root.right, targetSum)
        return res

需要二刷。

438. 找到字符串中所有字母异位词

写了一个常规方法，超时了：

class Solution:
    def findAnagrams(self, s: str, p: str) -> List[int]:
        res = []
        length = len(p)
        for i in range(len(s) - length + 1):
            tmp_s = s[i: i + length]
            tmp = "".join(sorted(tmp_s))
            if tmp == p:
                res.append(i)
        return res

根据思路自己写的：

class Solution:
    def findAnagrams(self, s: str, p: str) -> List[int]:
        len_s, len_p = len(s), len(p)
        if len_s < len_p:
            return []

        s_cnt = [0] * 26
        p_cnt = [0] * 26
        for i in range(len_p):
            s_cnt[ord(s[i]) - 97] += 1
            p_cnt[ord(p[i]) - 97] += 1

        res = []
        if s_cnt == p_cnt:
            res.append(0)

        for i in range(len_s - len_p):
            s_cnt[ord(s[i]) - 97] -= 1
            s_cnt[ord(s[i + len_p]) - 97] += 1

            if s_cnt == p_cnt:
                res.append(i + 1)
        
        return res
    

448. 找到所有数组中消失的数字

简单题，写了个暴力法，超时了

class Solution:
    def findDisappearedNumbers(self, nums: List[int]) -> List[int]:
        n = len(nums)
        res = []
        for i in range(1, n + 1):
            if i not in nums:
                res.append(i)
        return res

class Solution:
    def findDisappearedNumbers(self, nums: List[int]) -> List[int]:
        n = len(nums)
        for num in nums:
            x = (num - 1) % n
            nums[x] += n
        
        ret = [i + 1 for i, num in enumerate(nums) if num <= n]
        return ret

哈希的方法：

class Solution:
    def findDisappearedNumbers(self, nums: List[int]) -> List[int]:
        dic = {}
        for num in nums:
            if num in dic:
                dic[num] += 1
            else:
                dic[num] = 0
        
        res = []
        for i in range(1, len(nums) + 1):
            if i not in dic:
                res.append(i)
        return res