重学数据结构与算法
学习数据结构与算法的目的：
优化时间复杂度与空间复杂度 优化时间复杂度与空间复杂度 优化时间复杂度与空间复杂度
教程总纲： 暴力解法(模拟)、算法优化(递归/二分/排序/DP)、时刻转换(数据结构)
-
- 1.时间复杂度的核心方法论
- 2.增删查——选取数据结构的基本方法
- 3.线性表——如何完成基本增删查
- 4.栈——先进后出的增删查
- 5.队列——先进先出的增删查
- 6.数组——基于索引的查找
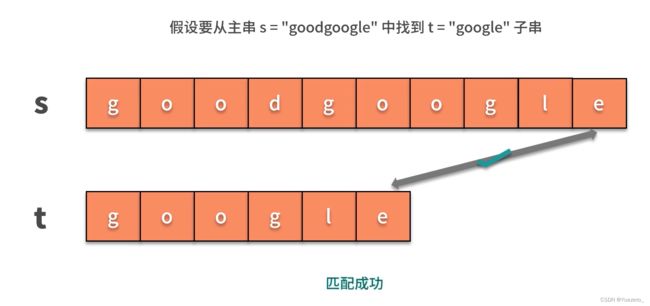
- 7.字符串——字符串匹配与操作
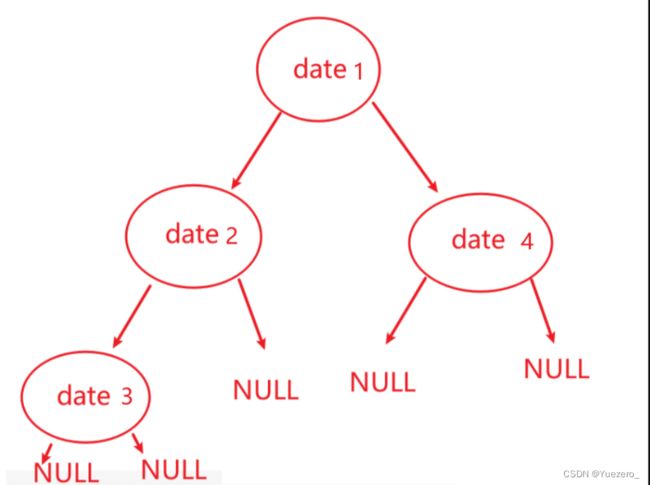
- 8.树&二叉树——分支与层次关系
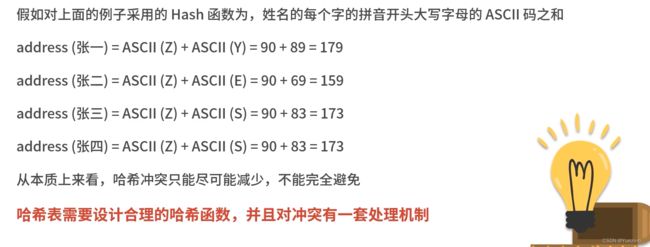
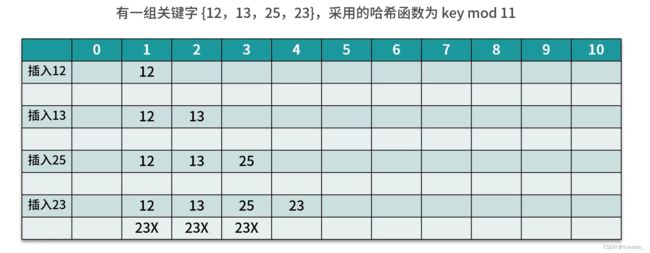
- 9.哈希表——高效查找的利器
- 10.递归——解决汉诺塔问题
- 11.分治——利用分治快速完成数据查找
- 12.排序——经典排序算法解析
1.时间复杂度的核心方法论
空间是廉价的，时间是昂贵的
相较于空间复杂度(投入金钱 增加算力)，时间复杂度(消耗时间)更为重要！
降低时间与空间复杂度的方法：
时刻转换：选用合适的数据结构，进一步降低时间复杂度
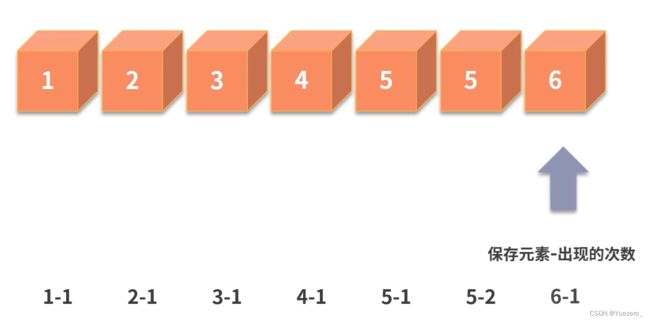
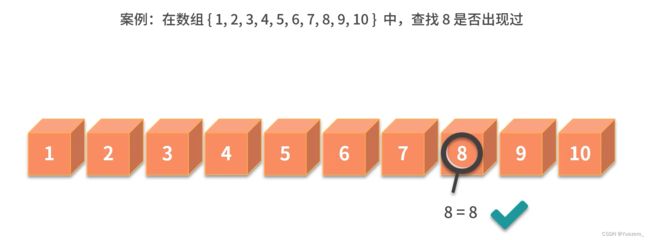
例.输入数组 a = [1,2,3,4,5,5,6] 中查找出现次数最多的数值。
暴力解法是：两层for遍历，维护一个最大次数time_max，对每个元素计算出现次数time_tmp，与time_max进行对比，时间复杂度是 0 ( n 2 ) 0(n^2) 0(n2)
int main(){
vector<int> a={1,2,3,4,5,5,6};
int val_max=-1,time_max=0,time_tmp=0;
for(int i=0;i<a.size();i++){
time_tmp=0;
for(int j=0;j<a.size();j++)
if(a[i]==a[j]) time_tmp++;
if(time_tmp>time_max){
time_max=time_tmp;
val_max=a[i];
}
}
cout<<val_max<<" "<<time_max<<endl;
return 0;
}
优化思想：如何仅用单层for循环完成，用hash思想，引入k-v字典数据结构map，一次for保存每个元素出现的次数，再求每个元素次数的最大值，时间复杂度是 0 ( 2 n ) 0(2n) 0(2n)。
int main(){
vector<int> a={1,2,3,4,5,5,6};
map<int ,int> num_cnt;
int val_max,time_max=0;
for(int i=0;i<a.size();i++){
num_cnt[a[i]]++; //counting the number of times a[i occurs in the vector a.
}
for(auto it:num_cnt){ //iterating over the map and printing the max time a[i] occurs for each element.
if(time_max < it.second){
val_max=it.first; //assigning the maximum value from the map to val_max.
time_max=it.second; //assigning the maximum count from the map to time_max.
}
}
cout<<val_max<<" "<<time_max<<endl;
return 0;
}
2.增删查——选取数据结构的基本方法
当你不知道用什么数据结构的时候：
分析需要对数据进行了哪些操作，根据数据操作，选取合适的数据结构 分析需要对数据进行了哪些操作，根据数据操作，选取合适的数据结构 分析需要对数据进行了哪些操作，根据数据操作，选取合适的数据结构
还用上面的例子介绍：
对于统计次数最多的元素，我们需要对数据结构进行以下操作：
具体的：
所以
3.线性表——如何完成基本增删查
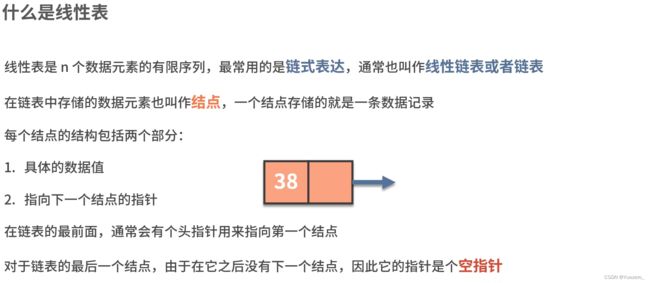
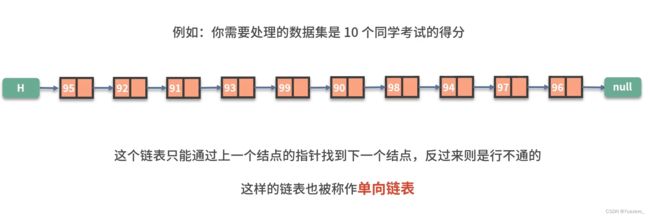
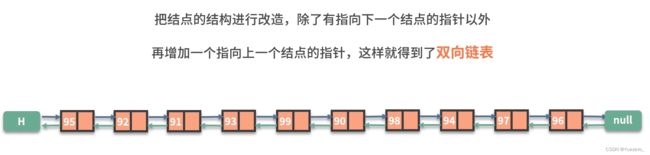
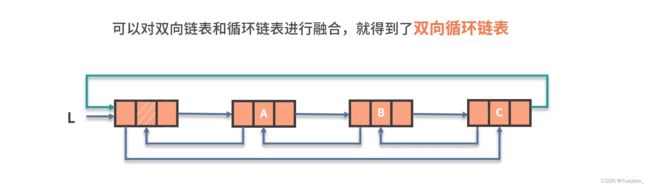
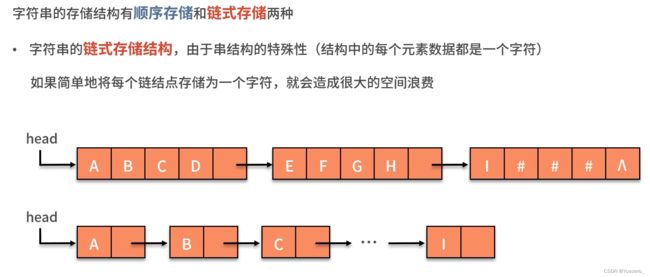
实际上，有线性存储(数组)和链式存储(链表)两种结构，这里仅介绍链式存储。
单向链表：
循环链表：
双向链表：
双向循环链表：
线性表增删查：其他链表的操作与单向链表雷同，仅介绍单向链表
增加操作：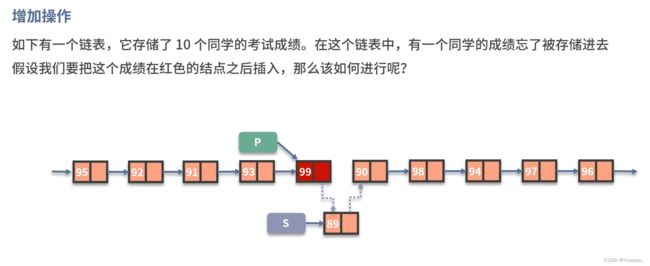
删除操作：
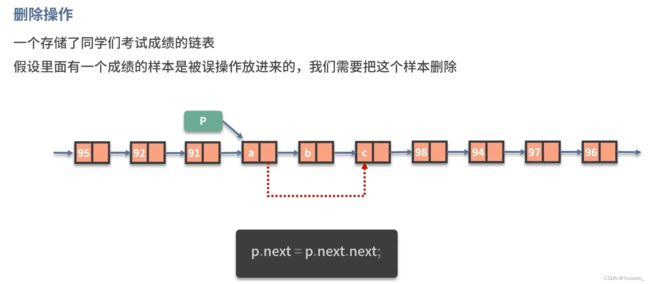
查找操作：
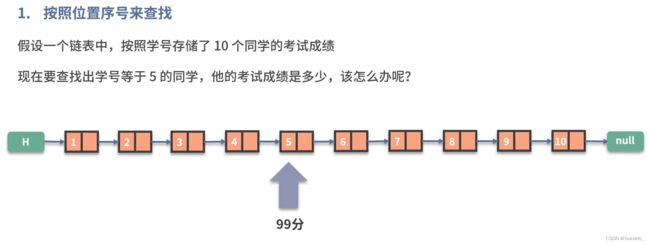
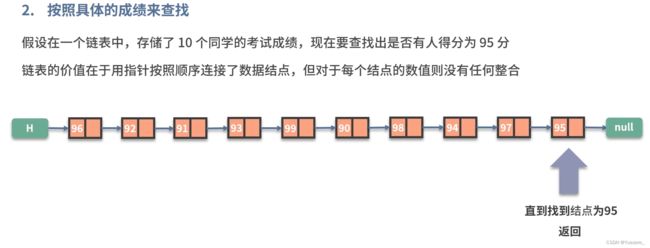
总结：
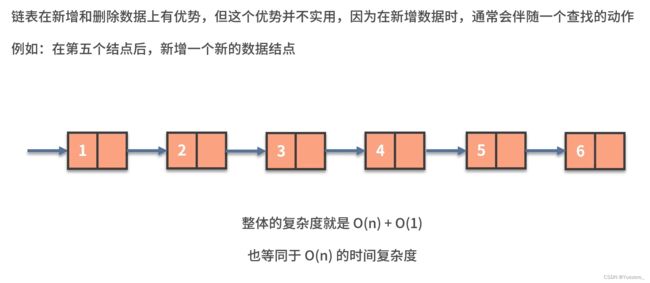
链表的查找速度慢 ( 无法用 i n d e x ) O ( n ) ，但插入和删除 ( 改变指针 ) 方便 O ( 1 ) 链表的查找速度慢(无法用index)O(n)，但插入和删除(改变指针)方便O(1) 链表的查找速度慢(无法用index)O(n)，但插入和删除(改变指针)方便O(1)
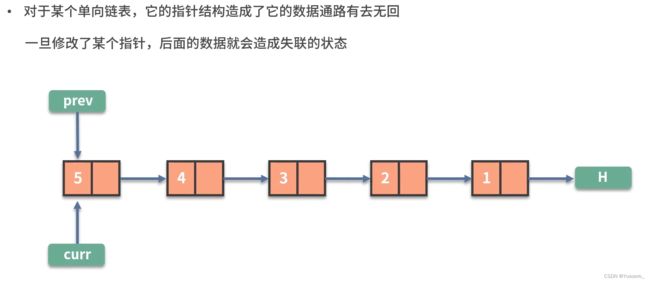
链表的问题常常围绕数据顺序的处理：链表反转，快慢指针
例1.
为此，我们使用3个指针prev、curr、next，分别指向 新链表头节点、旧链表转换节点、旧链表转换节点的下一个，完成旧链表向链表逐个节点的转换。
#include例2.
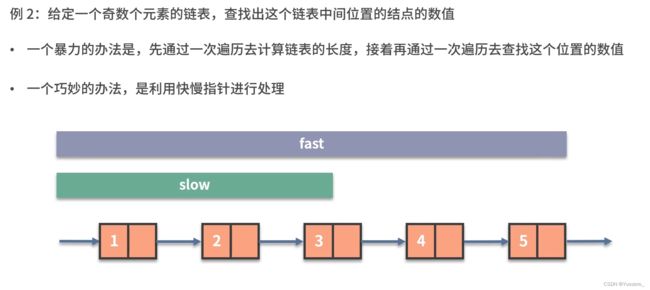
slow走1步，fast走两步。（因为fast一次走两步，所以要防止fast到fast.next.next为空，所以while的判断条件是3个）
fast到达终点时，slow到达中点。
#include例3.
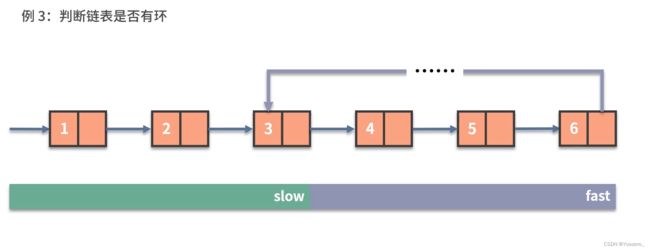
基本思想是利用两个指针，一个快指针和一个慢指针，分别从链表头部开始遍历，快指针每次走两步，慢指针每次走一步，若快指针追上了慢指针，则说明链表存在环路；否则，当快指针到达链表尾部时，结束遍历，slow永远不可能和fast相等，链表不存在环路。
#include4.栈——先进后出的增删查
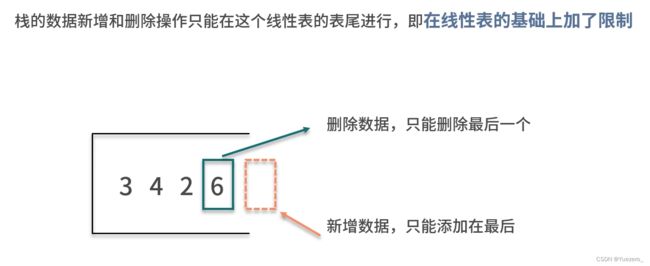
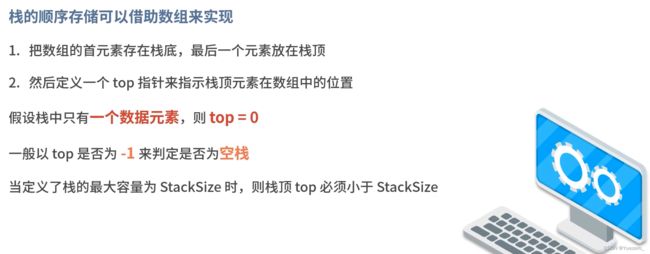
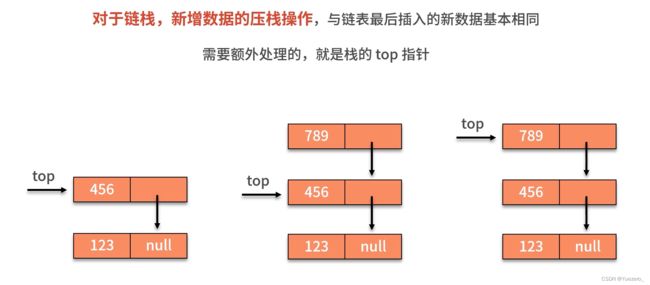
顺序栈：
推荐：用vector模拟栈时，仅允许在线性表尾部（栈顶）插入删除数据，push_back()和pop_back()。
不推荐：也可以用数组模拟。
链栈： 不需要头指针，进维护一个栈顶top指针。
例1.
#include例2.
#include总结：
5.队列——先进先出的增删查
头指针front，尾指针rear
链队： 头节点仅用来表示队列(data=number)，不用了存储数据
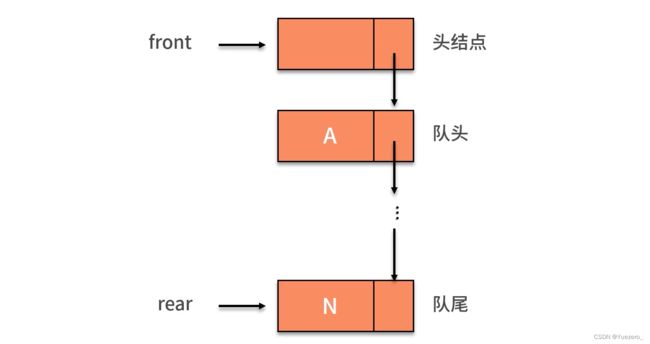
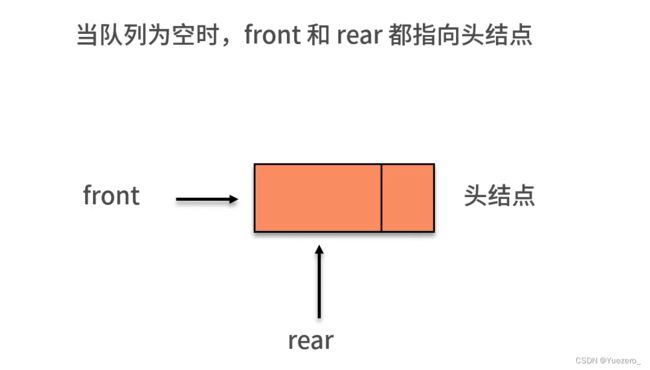
头节点的意义：给空链表的front 和rear指针一个指向，防止变成野指针。
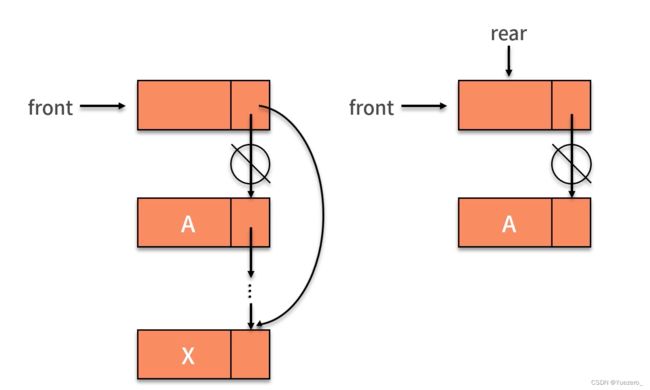
顺序队列： 数组模拟，队尾插入时间复杂度为O(1)，队头删除，后面的所有元素前移，时间复杂度为O(n)，如果仅通过移动fornt指针的方式，会造成假溢出，空间不足的情况。
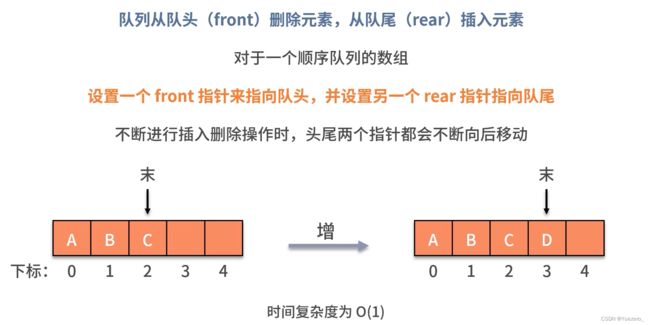
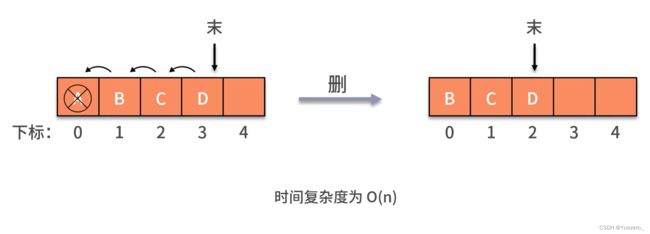
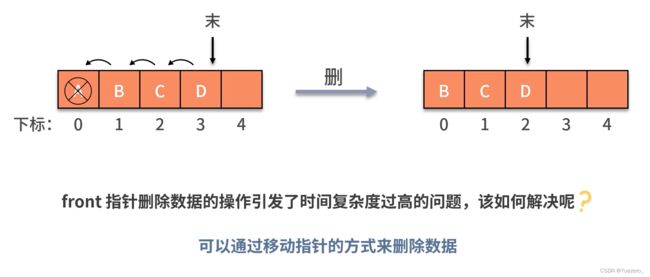
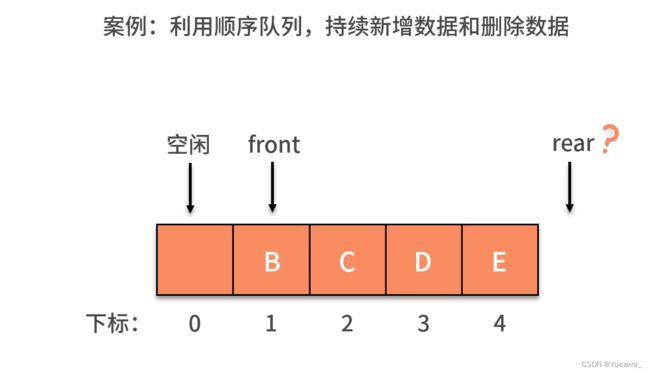
实际上，上述两种解决方法都不好，假溢出最优的解决办法是构造循环队列
循环队列：
例1.
总结：
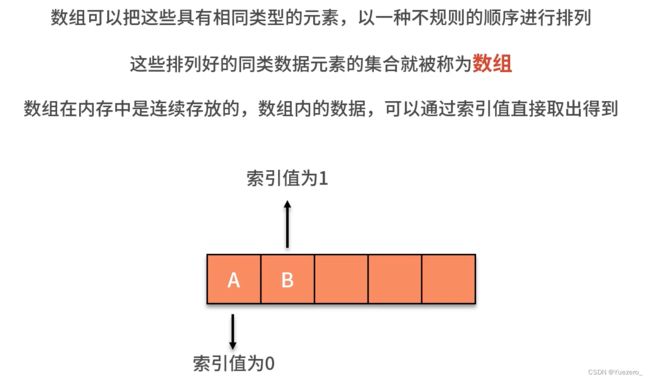
6.数组——基于索引的查找
增加：
删除：
查找;
总结：
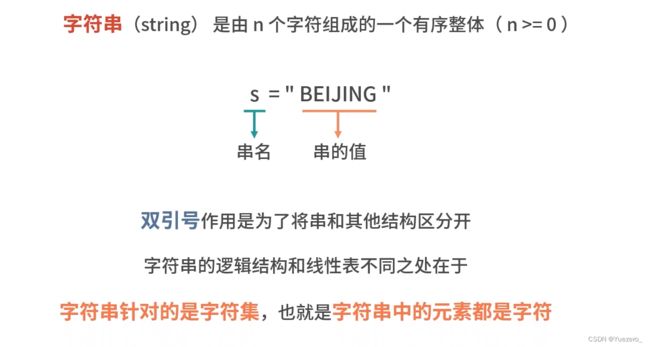
7.字符串——字符串匹配与操作
插入：
删除：
字符串匹配：
暴力匹配：
例题. 可以暴力也，可以动态规划
暴力法：
#include例题.
#include总结：面试笔试常考字符串匹配！暴力->KMP
8.树&二叉树——分支与层次关系
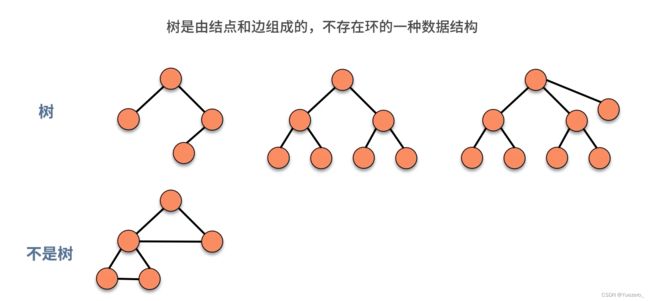
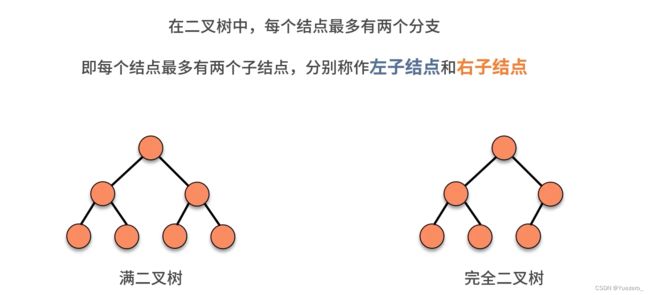
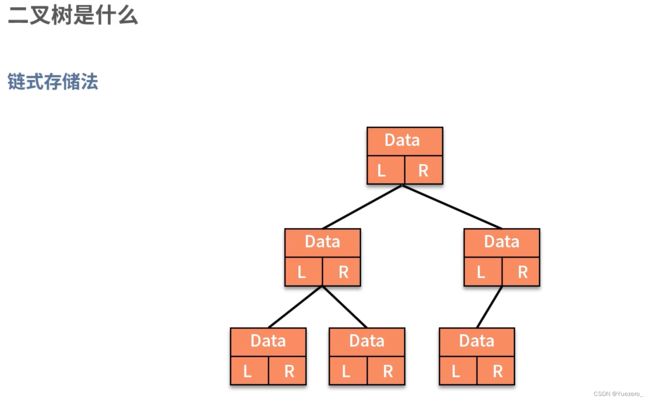
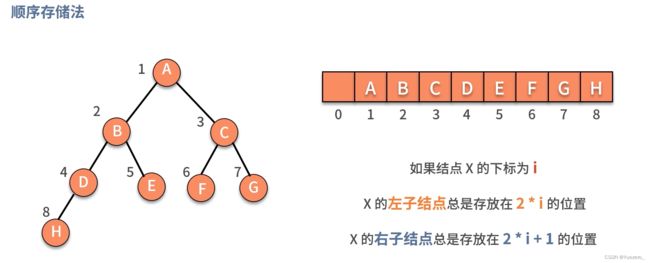
非完全二叉树，使用顺序存储会浪费大量的存储空间！
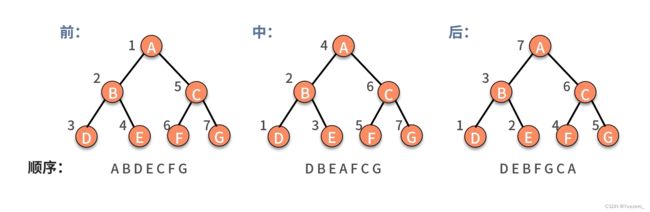
递归实现二叉树的前、中、后序遍历
class node{ public: string val; node* left; node* right;};
void PreOrder(node* NODE){
if(NODE==NULL)return;
cout<<NODE->val<<" ";
PreOrder(NODE->left);
PreOrder(NODE->right);
}
void InOrder(node* NODE){
if(NODE==NULL)return;
InOrder(NODE->left);
cout<<NODE->val<<" ";
InOrder(NODE->right);
}
void PostOrder(node* NODE){
if(NODE==NULL)return;
PostOrder(NODE->left);
PostOrder(NODE->right);
cout<<NODE->val<<" ";
}
二叉查找树（二次排序树）： 左小右大，左子树上所有结点的关键字均小于根结点的关键字；右子树上的所有结点的关键字均大于根结点的关键字。中序遍历是有序数列！
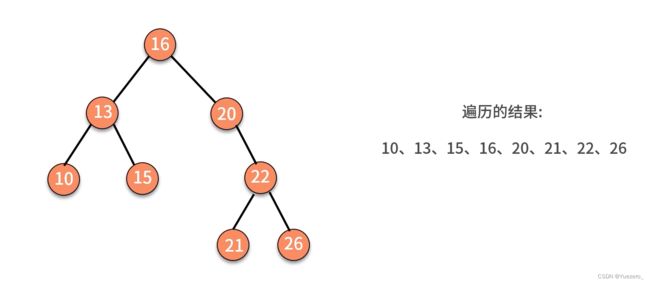
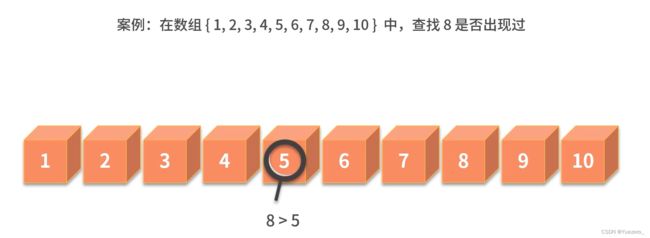
利用二次排序树的性质，可以实现二分查找，加快查找速度！！！
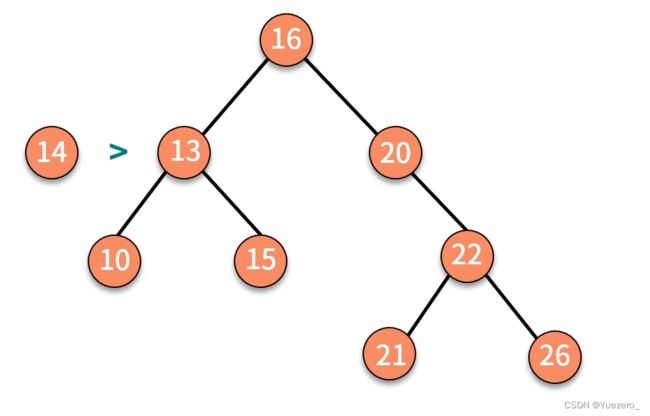
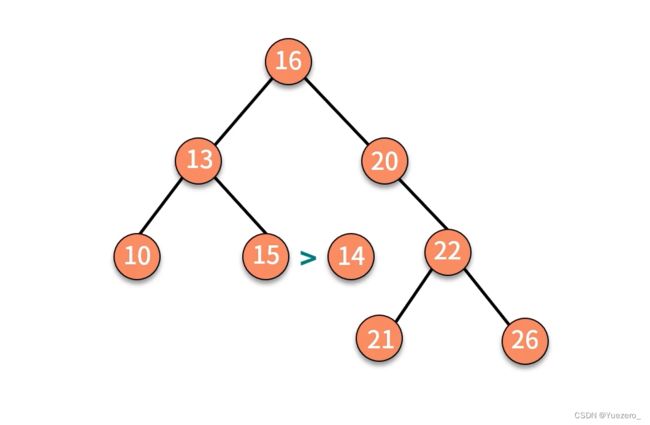
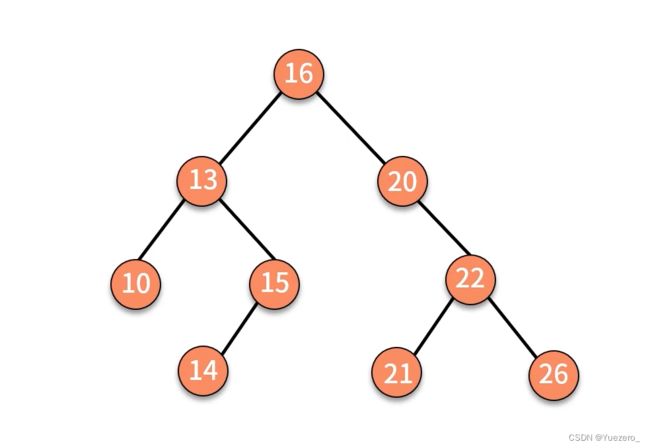
二叉排序树的插入：
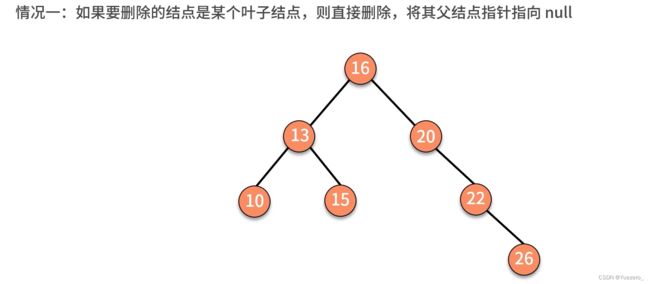
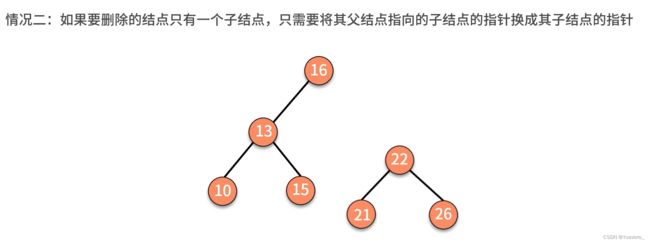
二叉排序树删除：
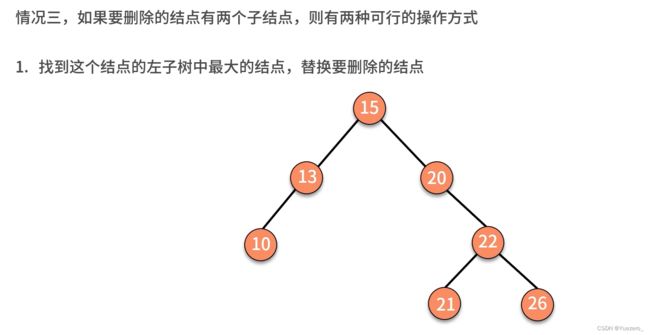
例题.
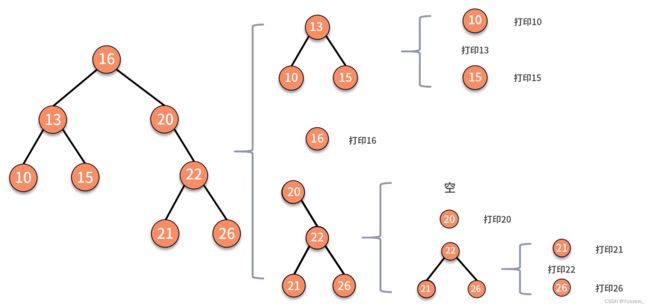
可以暴力搜索，也可以用字典树，层次遍历到叶子节点的路径。
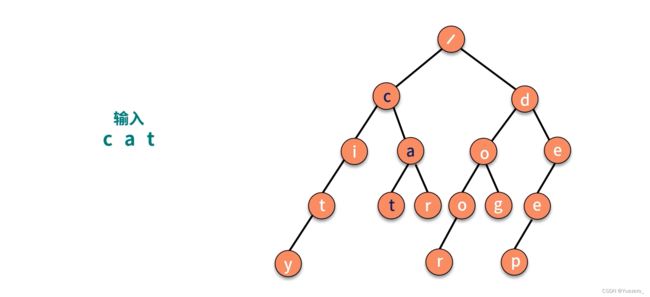
例题.层次遍历，维护OPEN表(队列)，不断扩展队首子节点，加入队列。
#include
总结：
9.哈希表——高效查找的利器
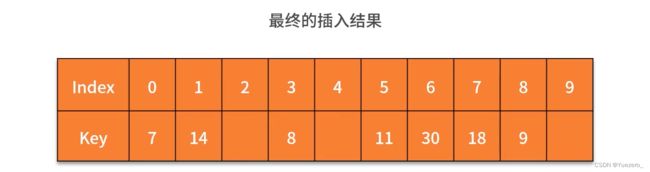
哈希冲突： 不同对象的哈希地址相同（键值对的值相同）
哈希函数设计： 下面几个方法都可能出现哈希冲突
冲突解决：
线性探测法（沿占用地址逐个向下遍历寻找未占用的地址存放）
链地址法（将相同哈希地址的记录存放在同一条链表上）
总结：优点（CUDR飞快）、缺点(处理输入顺序敏感的问题时，会破坏序列的构造顺序)
如C++的map
例子.
例题.
#include总结：
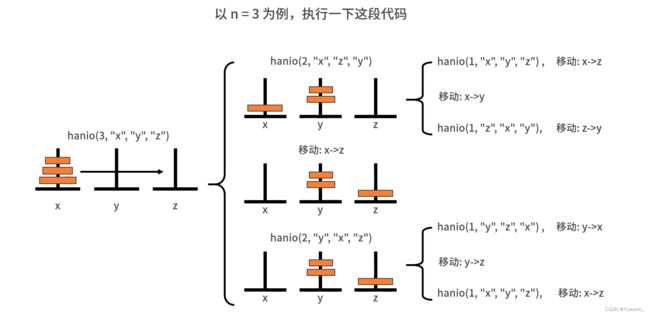
10.递归——解决汉诺塔问题
例子.中序遍历
例子.汉诺塔问题
总结：
11.分治——利用分治快速完成数据查找
分治需要使用递归
每轮递归的包括：分解问题、解决问题、合并结果
例子.
可以递归实现，也可以循环实现
#include例题.
总结：
12.排序——经典排序算法解析
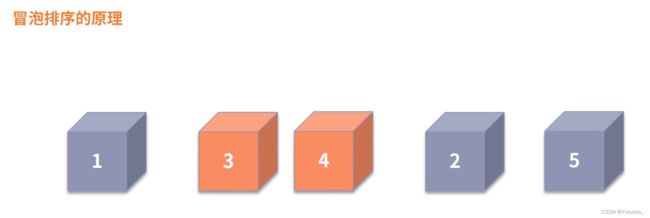
冒泡排序： 相邻元素两两比较，逆序对交换，每轮将1个大的元素交换到最后，经过多轮迭代完成排序。稳定：元素相等时不做交换
#include插入排序： 维护一个排好序的序列，不断为每个未插入的元素，与序列元素比较，找到合适的插入位置。稳定排序。
#include
归并排序： 将待排序序列从中点位置分成左右两个子序列，分别递归调用归并排序函数，直到子序列长度为1。对两个已经有序的子序列进行合并，得到一个新的有序序列。 稳定排序