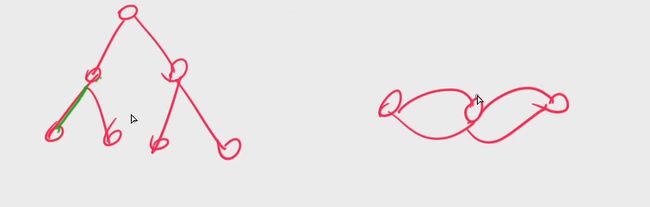第三章 图论 No.10无向图的双连通分量

定义

无向图有两种双连通分量

1. 边双连通分量，e-DCC
2. 点双连通分量，v-DCC

桥：删除这条无向边后，图变得不连通，这条边被称为桥
边双连通分量：极大的不含有桥的连通区域，说明无论删除e-DCC中的哪条边，e-DCC依旧连通 （该连通分量的任意边属于原图中的某条环）。此外，任意两点之间一定包含两条不相交（无公共边）的路径

割点：删除该点（与该点相关的边）后，图变得不连通，这个点被称为割点
点双连通分量：极大的不含有割点的连通区域

一些性质：

1. 每个割点至少属于两个连通分量
2. 任何两个割点之间的边不一定是桥，任何桥两边的端点不一定是割点，两者没有必然联系，一个点连通分量也不一定是边连通分量

---

Tarjan求e-DCC

无向图不存在横叉边
和有向图的强连通分量类似，引入dfn和low两个数组
如何找到桥？判断x->y的y是否能走到x之前（祖先节点），如果能走到，x和y在一个环中，删除这条边，其他点依然是连通的
所以x->y为桥：dfn[x] < low[y]

如何找到所有边的双连通分量？

1. 删除所有桥
2. 或者用stk辅助，若dfn[x] == low[x]，说明x出发一定走不到x的祖先节点，那么x和其父节点之间的边是桥，此时还在stk中的点为e-DCC的节点

这里使用第二种方式，模板：

void tarjan(int x, int from)
{
    dfn[x] = low[x] = ++ tp;
    stk[ ++ tt] = x;
    for (int i = h[x]; i != -1; i = ne[i])
    {
        int y = e[i];
        if (!dfn[y])
        {
            tarjan(y, i);
            low[x] = min(low[x], low[y]);
        }
        else if (i != (from ^ 1))
            low[x] = min(low[x], dfn[y]);
        if (dfn[x] < low[y])
                st[i] = st[i ^ 1] = true;
    }
    if (dfn[x] == low[x])
    {
        int y;
        cnt ++ ;
        do {
            y = stk[tt -- ];
            id[y] = cnt;
        } while (x != y);
    }
}

由于无向图要存储两条有向边，并且从数组的0下标开始存储，所以0，1、2，3…这样一对的边是互相反向的边，即i和i ^ 1为反向边
为什么与有向图的强连通分量不同，边双连通分量不需要使用st数组以标记栈中的元素？
因为无向图不存在横叉边的概念，就不会出现：x->y而y的dfn小于x，因为在无向图中y一定会向x遍历

---

Tarjan求v-DCC

如何求割点？low[y] >= dfn[x]，删除x节点后，y就是一颗独立的子树

1. 如果x不是根节点，那么x是一个割点
2. 如果x是根节点，至少有两个y满足以上关系

求割点的板子：

void tarjan(int x)
{
    dfn[x] = low[x] = ++ tp;
    int t = 0;
    for (int i = h[x]; i != -1; i = ne[i])
    {
        int y = e[i];
        if (!dfn[y])
        {
            tarjan(y);
            low[x] = min(low[x], low[y]);
            if (low[y] >= dfn[x]) t ++ ;
        }
        else low[x] = min(low[x], dfn[y]);
    }
    
    if (x != root) t ++ ;
    ans = max(ans, t);
}

将每个v-DCC向其包含的割点连一条边
缩点后，边的数量不会增加，点的数量可能会增加到两倍

tarjan求v-DCC与割点的板子：
一个孤立的点也是一个v-DCC，这里需要特判
若满足条件：low[y] >= dfn[x]，那么y的子树与x一起就是一个v-DCC
对于该节点是否是割点还需要特判，若该节点为根，并且与该节点相连的连通块数量为1，那么该节点就不是一个割点

void tarjan(int x)
{
	dfn[x] = low[x] = ++ tp;
	stk[ ++ tt ] = x;

	if (x == root && h[x] == -1)
	{
		++ dcnt;
		dcc[dcnt].push_back(x);
		return;
	}
	int t = 0; // 与x相连的连通块数量
	for (int i = h[x]; i != -1; i = ne[i])
	{
		int y = e[i];
		if (!dfn[y])
		{
			tarjan(y);
			low[x] = min(low[x], low[y]);
			if (low[y] >= dfn[x])
			{
				dcnt ++, t ++ ;
				if (x != root || t > 1) st[x] = true; // x为割点
				int u;
				do {
					u = stk[tt -- ];
					dcc[dcnt].push_back(u);
				} while (u != y);
				dcc[dcnt].push_back(x);
			}
		}
		else low[x] = min(low[x], dfn[y]);
	}
}

---

395. 冗余路径

395. 冗余路径 - AcWing题库

任意两点间都存在两条没有重复边的路径，等价于整个图是一个双连通分量

将无向连通图的边双连通分量缩点后，得到的结构是一颗树，因为边双连通分量是不包含桥的结构，缩点后，图中只含有桥，即删除任意一条边后，图成为两个连通块，这是一个树结构

为了满足题意，需要向这颗树中添加边，使之成为边连通分量，那么要加几条边？

连接所有叶子节点，使这颗树结构成为双连通分量，至少需要加$[(cnt+1)/2]$然后再下取整的边数，也就是将每个叶子节点相连，使环满足双连通分量的性质

注意cnt为1时需要特判

#include 
#include 
using namespace std;

const int N = 5010, M = 10010;
int h[N], e[M], ne[M], idx;
int dfn[N], low[N], tp, cnt;
int stk[N], tt, id[N];
bool st[N]; int d[N];
int n, m;

void add(int x, int y)
{
    e[idx] = y, ne[idx] = h[x], h[x] = idx ++ ;
}

void tarjan(int x, int from)
{
    dfn[x] = low[x] = ++ tp;
    stk[ ++ tt] = x;
    for (int i = h[x]; i != -1; i = ne[i])
    {
        int y = e[i];
        if (!dfn[y])
        {
            tarjan(y, i);
            low[x] = min(low[x], low[y]);
            if (dfn[x] < low[y])
                st[i] = st[i ^ 1] = true;
        }
        else if (i != (from ^ 1))
            low[x] = min(low[x], dfn[y]);
    }
    if (dfn[x] == low[x])
    {
        int y;
        cnt ++ ;
        do {
            y = stk[tt -- ];
            id[y] = cnt;
        } while (x != y);
    }
}

int main()
{
    memset(h, -1, sizeof(h));
    scanf("%d%d", &n, &m);
    int x, y;
    for (int i = 0; i < m; ++ i )
    {
        scanf("%d%d", &x, &y);
        add(x, y), add(y, x);
    }
    
    tarjan(1, -1);
    for (int i = 0; i < idx; i ++ )
        if (st[i])
            d[id[e[i]]] ++ ;
    
    int res = 0;
    for (int i = 1; i <= cnt; ++ i )
        if (d[i] == 1) res ++ ;
        
    if (cnt == 1) puts("0");
    else printf("%d\n", (res + 1) / 2);
    return 0;
}

debug：^的优先级小于!=

可以不使用st数组标记桥：

#include 
#include 
using namespace std;

const int N = 5010, M = 10010;
int h[N], e[M], ne[M], idx;
int dfn[N], low[N], tp, cnt;
int stk[N], tt, id[N];
int d[N];
int n, m;

void add(int x, int y)
{
    e[idx] = y, ne[idx] = h[x], h[x] = idx ++ ;
}

void tarjan(int x, int from)
{
    dfn[x] = low[x] = ++ tp;
    stk[ ++ tt] = x;
    for (int i = h[x]; i != -1; i = ne[i])
    {
        int y = e[i];
        if (!dfn[y])
        {
            tarjan(y, i);
            low[x] = min(low[x], low[y]);
        }
        else if (i != (from ^ 1))
            low[x] = min(low[x], dfn[y]);
    }
    if (dfn[x] == low[x])
    {
        int y;
        cnt ++ ;
        do {
            y = stk[tt -- ];
            id[y] = cnt;
        } while (x != y);
    }
}

int main()
{
    memset(h, -1, sizeof(h));
    scanf("%d%d", &n, &m);
    int x, y;
    for (int i = 0; i < m; ++ i )
    {
        scanf("%d%d", &x, &y);
        add(x, y), add(y, x);
    }
    
    tarjan(1, -1);
    
    
    for (int x = 1; x <= n; ++ x )
        for (int i = h[x]; i != -1; i = ne[i])
        {
            int y = e[i];
            int a = id[x], b = id[y];
            if (a != b) d[a] ++ ;
        }
        
    int res = 0;
    for (int i = 1; i <= cnt; i ++ )
        if (d[i] == 1) res ++ ;
    
    if (cnt == 1) puts("0");
    else printf("%d\n", (res + 1) / 2);
    return 0;
}

---

1183. 电力

1183. 电力 - AcWing题库

枚举所有割点，判断删除哪个割点后剩余的连通块数量最大
剩余的连通块数量为ans + cnt - 1
由于题目给定的图并不是一个连通图，所以可能存在多个连通块，cnt为连通块数量
枚举所有割点只能在一个连通块中枚举，此时其他连通块的数量为cnt - 1
又因为ans为删除割点后，剩余连通块最多的值，所以答案为ans + cnt - 1

这题的点编号从0开始

#include 
#include 
using namespace std;

const int N = 10010, M = 30010;
int h[N], e[M], ne[M], idx;
int dfn[N], low[N], tp, cnt;
int ans, n, m, root;

void add(int x, int y)
{
    e[idx] = y, ne[idx] = h[x], h[x] = idx ++ ;
}

void tarjan(int x)
{
    dfn[x] = low[x] = ++ tp;
    int t = 0;
    for (int i = h[x]; i != -1; i = ne[i])
    {
        int y = e[i];
        if (!dfn[y])
        {
            tarjan(y);
            low[x] = min(low[x], low[y]);
            if (low[y] >= dfn[x]) t ++ ;
        }
        else low[x] = min(low[x], dfn[y]);
    }
    
    if (x != root) t ++ ;
    ans = max(ans, t);
}

int main()
{
    while (scanf("%d%d", &n, &m), n | m)
    {
        memset(h, -1, sizeof(h));
        memset(dfn, 0, sizeof(dfn));
        idx = tp = cnt = ans = 0;
        int x, y;
        for (int i = 0; i < m; ++ i )
        {
            scanf("%d%d", &x, &y);
            add(x, y), add(y, x);
        }
        for (root = 0; root < n; ++ root)
        {
            if (!dfn[root]) 
            {
                cnt ++ ;
                tarjan(root);
            }
        }
        printf("%d\n", ans + cnt - 1);
    }
    return 0;
}

debug：dfn数组没有置空

---

396. 矿场搭建

396. 矿场搭建 - AcWing题库

对于图中的每个连通块，分情况讨论：

1. 若连通块无割点，那么任意设置两个救援点即可
2. 若连通块中有割点，缩点：将每个割点依然看成一个点，将每个v-DCC向其包含的割点连线

• 缩点后得到一棵树，对于叶子节点，需要建立救援点。因为只有一个点与其相连，若该点坍塌，需要在内部建立救援点。假设内部节点数量为cnt，方案数为cnt-1个，去除割点
• 对于非叶子节点，无需建立救援点，因为无论与之相连的哪个割点坍塌，该节点都能走到叶子节点，而叶子节点已经建立救援点

#include 
#include 
#include 
using namespace std;

typedef unsigned long long ULL;
const int N = 1010, M = 1010;
int h[N], e[M], ne[M], idx;
vector<int> dcc[N];
int dcnt, root;
int dfn[N], low[N], tp;
int stk[N], tt;
bool st[N];
int n, m;

void add(int x, int y)
{
    e[idx] = y, ne[idx] = h[x], h[x] = idx ++ ;
}

void tarjan(int x)
{
    low[x] = dfn[x] = ++ tp;
    stk[ ++ tt ] = x;
    if (x == root && h[x] == -1)
    {
        dcnt ++ ;
        dcc[dcnt].push_back(x);
        return;
    }
    int t = 0;
    for (int i = h[x]; i != -1; i = ne[i])
    {
        int y = e[i];
        if (!dfn[y])
        {
            tarjan(y);
            low[x] = min(low[x], low[y]);
            if (low[y] >= dfn[x])
            {
                t ++, dcnt ++ ;
                if (x != root || t > 1) st[x] = true;
                int u;
                do {
                    u = stk[tt -- ];
                    dcc[dcnt].push_back(u);
                } while (u != y);
                dcc[dcnt].push_back(x);
            }
        }
        else low[x] = min(low[x], dfn[y]);
    }
}

int main()
{
    int T = 1;
    while (scanf("%d", &m), m)
    {
        for (int i = 0; i < N; ++ i ) dcc[i].clear();
        memset(h, -1, sizeof(h));
        memset(dfn, 0, sizeof(dfn));
        memset(st, false, sizeof(st));
        tp = dcnt = idx = tt = n = 0;
        for (int i = 0; i < m; ++ i )
        {
            int x, y;
            scanf("%d%d", &x, &y);
            add(x, y), add(y, x);
            n = max(n, x), n = max(n, y);
        }
        for (root = 1; root <= n; ++ root )
            if (!dfn[root]) tarjan(root);
        
        ULL sum = 1; int ans = 0;
        for (int i = 1; i <= dcnt; ++ i )
        {
            int t = 0;
            for (int j = 0; j < dcc[i].size(); ++ j )
                if (st[dcc[i][j]]) 
                    t ++ ;
            if (t == 0) 
            {
                if (dcc[i].size() > 1) ans += 2, sum *= ((ULL)dcc[i].size() * (dcc[i].size() - 1)) / 2;
                else ans ++ ;
            }
            else if (t == 1) ans += 1, sum *= (dcc[i].size() - 1);
        }
        printf("Case %d: %d %llu\n", T ++ , ans, sum);
    }
    return 0;
}

debug：由于多组测试数据，没有初始化干净所有元素
最后统计救援点数量以及方案总数时，没有对孤立点进行特判