- 【从零开始学习计算机科学】数字逻辑(五) Verilog HDL语言
贫苦游商
学习fpga开发数字逻辑verilogHDL硬件开发逻辑电路
【从零开始学习计算机科学】数字逻辑(五)VerilogHDL语言VerilogHDL语言8位全加器8位计数器2位比较器三态驱动器VerilogHDL模块的结构模块声明。端口定义。信号类型。功能描述verilog描述级别verilog关键字verilog标识符编写VerilogHDL源代码的标准数据类型常量变量nets型变量register型变量reg型变量运算符及表达式算术运算符逻辑运算符位运算符
- java手机小游戏源码_Java手机版数独小游戏(J2me)JAVA游戏源码下载
weixin_39748773
java手机小游戏源码
数独游戏,相信朋友们都知道的,以前也经常玩的,用VB、VC++和Delphi版编写的都在网上宣布过,今天放出一个鉴于Java的J2me手机版的,大致看一下截图,这是在Java模拟机运行的界面,带有Java源码,学习J2me编程的朋友有资料看了。Java手机版数独小游戏(J2me)(1folders,2files,1.38KB,754.03KBintotal.)源码(1folders,2f
- java课程 数独 文库_JAVA版数独小游戏源码(课程设计)
weixin_39640543
java课程数独文库
【实例简介】JAVA版数独小游戏源码(课程设计)【实例截图】【核心代码】Sudoku├──bin│└──com│└──marssoft│└──sudoku│├──Sudoku.class│├──bean││├──Base.class││├──Member.class││└──Number.class│├──display││├──LineFrame$RePaint.class││└──LineFr
- 如何用python实现数独游戏【附源码】
helloshili2011
python专栏java前端服务器
一、第一次用python实现数独游戏的代码:defprint_board(board):forrowinboard:print("".join(map(str,row)))defis_valid_move(board,row,col,num):#Checkifthenumberisalreadyintherowifnuminboard[row]:returnFalse#Checkifthenumb
- 代码随想录第二十五天|回溯算法part05--332.重新安排行程、51.N皇后、37.解数独
Aqua Cheng.
代码随想录算法训练营一刷算法java数据结构leetcode
刷题小记:三道困难题,理解成本不低,推荐结合题解视频进行理解。回溯问题的本质是暴力搜索,在面对过于复杂的问题时,要把握事物的主要矛盾,即应当先实现基本思路,再考虑剪枝(次要矛盾),否则可能不但没成功剪枝,反倒“枝横叶乱”。332.重新安排行程(332.重新安排行程)题目分析:给定一个航线列表List>tickets,其中tickets[i]=[fromi,toi]表示飞机出发和降落的机场地点。请对
- 引用相关的知识点
C小白˃ʍ˂
c++引用
一、引用的概念和声明什么是引用?就是给变量起一个别名(外号)语法:&=;&在这里代表引用变量说明符;&一符多义:①位运算中的位与运算符②引用变量说明符③取地址运算符引用时的注意事项:1.引用类型必须与被引用变量的数据类型一致2.被引用变量名必须是已被定义过的3.声明引用时必须进行初始化,指明它是哪个变量的引用4.引用不是值,不占内存空间5.引用只能被初始化一次对引用的修改实际就是对被引用变量的修改
- Java 运算符
Mr_One_Zhang
学习JAVAjavapython算法
计算机的最基本用途之一就是执行数学运算,作为一门计算机语言,Java也提供了一套丰富的运算符来操纵变量。我们可以把运算符分成以下几组:算术运算符关系运算符位运算符逻辑运算符赋值运算符其他运算符算术运算符算术运算符用在数学表达式中,它们的作用和在数学中的作用一样。下表列出了所有的算术运算符。表格中的实例假设整数变量A的值为10,变量B的值为20:操作符描述例子+加法-相加运算符两侧的值A+B等于30
- 数独游戏开发与优化:使用 Tkinter 实现数独界面和智能生成算法
壹屋安源
算法python数独游戏编程
文章目录介绍在这里插入图片描述代码解读1.数独生成器(`SudokuGenerator`)2.数独验证与优化3.数独界面(`SudokuGame`)4.创建数独网格5.计时器与历史记录优化与体验总结示例获取代码介绍在本篇博文中,我们将带您逐步实现一个数独游戏,使用Python的Tkinter库进行图形界面设计,并结合先进的数独生成和验证算法,确保每局游戏都能提供一个有挑战性的体验。我们不仅优化了数
- 算法题笔记(自用)——Python
CodeNerd影
算法笔记数据结构
目录一.进制&位运算&ASCAII二.format格式化输出1.基本用法2.位置参数3.格式化数字4.对齐和填充5.格式化二进制、八进制、十六进制6.格式化百分比7.格式化科学计数法8.格式化字符串字面量(f-string)三.字符串使用join()方法拼接字符串列表。使用find()查找子字符串的位置(返回第一个匹配的索引,未找到返回-1)。使用index()查找子字符串的位置(未找到会抛出异常
- 【0009】Python运算符详解
程序员张小厨
【007】PythonpythonPython运算符运算符Python算术运算符Python逻辑运算符Python关系运算符Python比较运算符
如果你觉得我的文章写的不错,请关注我哟,请点赞、评论,收藏此文章,谢谢!本文内容体系结构如下:运算符是用于执行各种运算的符号,例如加法、减法、乘法等。Python中的运算符可以分为算术运算符、赋值运算符、比较运算符、逻辑运算符、位运算符、成员运算符和身份运算符等。本文将详细讲解各个运算符的使用。一、算术运算符Python中的算术运算符主要用于执行数值计算,包括整数和浮点数的加减乘除等基本运算。具体
- tcl常用命令笔记--运算符&条件语句
芯火相传
tcl常用命令笔记笔记
运算符运算符是告诉编译器执行特定的数学或逻辑操作的符号,类型有:算术运算符,关系运算符,逻辑运算符,位运算符,三元运算符。算术运算符运算符描述+两个操作数相加-第一个操作数减第二个操作数*两个操作数相乘/分子除以分母%取余关系运算符运算符描述==检查前后是否相等,若相等则为1!=检查前后是否不相等,若不相等则为1>检查前操作数的值是否大于后操作数的值,若大于则为1=检查前操作数的值是否大于或等于后
- 位操作----位运算符详解(按位与(&),按位或(|),按位异或(^),按位取反(~)以及左移(<<)右移(>>))
什么半岛铁盒
c语言学习方法
前言此文主要为了本人复习知识在学习位操作时,我们首先要清楚的是:1.位运算的符操作数只能是整型和字符型对象2.在计算机中数据都是以二进制存储的(位操作就是直接对二进制进行操作)原码、反码、补码,这是学习位操作必不可少的知识一、原码、反码、补码整数以二进制补码的形式存储在内存中,对于正数的:原码、反码、补码都相同,以下规则针对负整数。原码:根据数值写出的二进制序列反码:以原码为基础,原码符号位不变其
- 如何判断字符串中所有字符是否全都不同?
钰云空间
java算法开发语言
文章目录概要解法一:利用Set不可重复性代码解析优点与缺点解法二:位运算解决代码解析优点与缺点结论概要在编程中,检查一个字符串中所有字符是否全都不同是一个常见问题。以下是两种常见的解决方案:使用集合(Set)和位运算(BitManipulation)。每种方法都有其优点和适用场景。本文将详细介绍这两种方法,包括其实现代码和分析解法一:利用Set不可重复性使用集合(Set)是一种直观且易于理解的方法
- 蓝桥杯备赛Day10 位运算
爱coding的橙子
蓝桥杯蓝桥杯算法c++
位运算1.要点与:&或:|异或:^非:~异或运算性质:(1)x^x=0(2)x^0=x(3)a^b^b=a(1,2推出)(4)a^b=c->a=b^c(两侧同异或b)位运算按补码计算正数的补码就是正数本身;负数的补码=负数的绝对值正数补码取反+1正数右移要用unsignedint最后才会变0(int高位补1)(1)将一个数乘(除)2的非负整数次幂x>i(除以2的i次方)(2)判断数字奇偶性:x&1
- ~ !和^=1这三个取反运算符的区别
Moonnnn.
c语言开发语言学习笔记
1.三者的关系和区别~(按位取反)~是按位取反运算符,它对操作数的每一位进行取反操作,即将0变为1,1变为0。例如,在二进制中,~00000010的结果是11111101。如果是有符号整数,按位取反后的结果还会涉及到补码等概念。它主要用于对整数的二进制位进行操作,常用于位运算相关的场景,如设置或清除特定的位标志等。!(逻辑取反)!是逻辑取反运算符,它用于对布尔值进行取反操作。如果操作数为真(非零值
- Python 从基础到进阶(一套打通)
浪子西科
Pythonpython开发语言
文章目录一、Python入门1.1Python简介1.2安装PythonWindowsLinuxmacOS1.3第一个Python程序交互式环境脚本文件二、Python基础语法2.1变量和数据类型变量数据类型数字类型字符串类型(str)布尔类型(bool)2.2运算符算术运算符比较运算符逻辑运算符位运算符2.3控制流语句条件语句循环语句`for`循环`while`循环三、Python数据结构3.1
- 【leetcode刷题版】回溯算法
学废了wuwu
算法leetcodepython
系列文章目录文章目录系列文章目录背景知识一、组合二、组合优化三、电话号码的字母组合四、组合总和五、组合总和Ⅱ六、分割回文串七、复原IP地址八、子集九、子集(需要去重)十、非递减子序列十一、全排列十一、全排列Ⅱ十二、重新安排行程(难)十三、N皇后十四、解数独背景知识回溯算法是一种通过试错来解决问题的算法。它会在解决问题的过程中剪枝,以避免无效搜索。在Python中实现回溯算法通常涉及以下几个步骤:定
- c++基础知识易忘易混 —— 6_函数、位运算和运算符 【复习笔记】
wanjiazhongqi
复习笔记笔记c++c语言
1.传引用调用引用特性:1.引用在定义时必须初始化2.一个变量可以有多个引用3.同一个引用不能给多个变量传引用调用不会拷贝实参,效率更高2.函数重载指在同一个作用域中可以有多个同名函数,函数名称相同,参数列表不同参数列表不同:参数的数量、类型或顺序至少有一个不同。函数返回类型不影响函数重载3.10进制转2进制1.用要转换的10进制数除以2,得到商和余数2.再将商除以2,又得到一个商和余数3.重复上
- 汇编的使用总结
¥ 多多¥
汇编
一、汇编的组成1、汇编指令(指令集)数据处理指令:数据搬移指令数据移位指令位运算指令算术运算指令比较指令跳转指令内存读写指令状态寄存器传送指令异常产生指令等2、伪指令不是汇编指令,但是可以起到指令的作用,伪指令也会占用一定内存空间3、伪操作不占用指令内存,能够在程序编译过程中起到编译引导作用的内容4、注释二、汇编指令2.1基本格式{}{s},,注释:opcode:指令的功能码,用来表示当前指令的作
- 单入单出队列性能优化(Lock-Free)
落樱弥城
c++基础知识性能优化开发语言c++多线程
摘要:文中首先介绍了有锁线程安全循环队列的基本实现,然后探讨了使用原子变量实现Lock-Free队列的优势,能够减少线程之间的数据竞争。接着,介绍了数据对齐的策略,以降低伪共享的概率,随后引入了索引缓存来减少索引访问冲突的影响。最后,文中提出了使用位运算替代模运算来优化循环队列的性能。关键字:单入单出队列,Lock-Free,伪共享,索引缓存,性能优化 CppCon中有一个单入单出Lock-Fr
- 【六】Golang 运算符
张胤尘
Golanggolang开发语言后端
欢迎来到张胤尘的技术站技术如江河,汇聚众志成。代码似星辰,照亮行征程。开源精神长,传承永不忘。携手共前行,未来更辉煌文章目录运算符算术运算符注意事项关系运算符逻辑运算符注意事项位运算符常见操作检查特定位是否为1(检查整数的奇偶性)设置特定位为1清除特定位交换变量值快速乘除注意事项赋值运算符基本赋值运算符复合赋值运算符指针运算符注意事项优先级运算符golang中支持运算符:算术运算符、关系运算符、逻
- 蓝桥杯学习大纲
ん贤
蓝桥杯算法数据结构
(致酷德与热爱算法、编程的小伙伴们)在查阅了相当多的资料后,发现没有那篇博客、文章很符合我们备战蓝桥杯的学习路径。所以,干脆自己整理一篇,欢迎大家补充!一、蓝桥必备高频考点我们以此为重点学习方向:1.基础算法枚举模拟贪心递归分治构造前缀和差分2.搜索与排序线性搜索二分法BFSDFS回溯剪枝深搜优化记忆化搜索位运算冒泡排序归并排序快速排序桶排序3.动态规划编辑距离最长不重复子串整数背包矩阵连乘最长公
- 快速幂(竞赛必备)
ん贤
蓝桥杯算法c++c语言
一、概念:快速幂是一种高效的指数运算方法,通过指数折半或二进制位运算减少计算次数。它的核心思想是利用二进制表示法或指数折半来加速计算,从而避免大量的循环操作。二、学习路径:了解基本概念掌握暴力解法、快速幂(二进制)、快速幂(指数折半)快速幂于库函数中pow()的区别。进行如下题目练习,以达到掌握目的:数的次幂(基础)->小数第n位(进阶)->堆的计数(综合)->乘法逆元(拓展)三、用法:快速幂可有
- 学习疯狂JAVA讲义——运算符与位运算符
红鲤鱼与绿鲤鱼与哈士奇
学习java开发语言
笔记备忘,方便以后忘了查询(如有错误,敬请指点)★变量:-变量的定义、赋值(简单值、表达式)-8个基本类型:byte、short、int、long、float、double、char、boolean★运算符▲算数运算符(7个):+、-、*、/、%++:将单个变量的值加1放在变量之后:表示先用变量的值,再自加放在变量之前:表示先自加,再用变量的值--:将单个变量的值减1放在变量之后:表示先用变量的值
- 从C语言的角度重构数据结构系列(十三)-位运算
文宇肃然
数据结构常见算法原理讲解C语言数据结构
位运算简介位运算位运算就是基于整数的二进制表示进行的运算。由于计算机内部就是以二进制来存储数据,位运算是相当快的。基本的位运算共6种,分别为按位与、按位或、按位异或、按位取反、左移和右移。运算运算符数学符号表示解释与&&、and只有两个对应位都为1时才为1或||、or只要两个对应位中有一个1时就为1异或^、xor只有两个对应位不同时才为1左移假设要将一个无符号整数乘以2。可以简单地将所有位向左边移
- 检查数独是否有效
想名真难
数据结构和算法
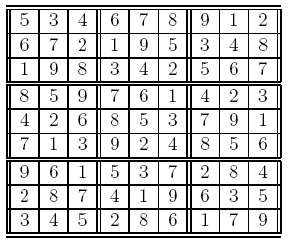
判断一个9x9的数独是否有效。只需要根据以下规则,验证已经填入的数字是否有效即可。数字1-9在每一行只能出现一次。数字1-9在每一列只能出现一次。数字1-9在每一个以粗实线分隔的3x3宫内只能出现一次。上图是一个部分填充的有效的数独。数独部分空格内已填入了数字,空白格用'.'表示。示例1:输入:[["5","3",".",".","7",".",".",".","."],["6",".",".",
- 【学习】验证数独的正确性
小飞哥咯咯咯
学习学习
源于面试的一个问题,在leetcode里也有这道题,参考站内的一篇文章。首先此问题的分析需要满足三个约束条件:每行不能有重复的数每列不能有重复的数每个3*3的方格中不能有重复的数其中前两个约束条件都是容易满足的,关键在第三个。使用三个与数独相同尺寸的二维数组,作为visited的tag,分别记录行、列和3*3方格的数据。关键在于上述的16行代码,接下来将详细分析:首先i/3和j/3会将当前的位置映
- 力扣每日一练之字符串Day6
京与旧铺
LeetCode刷起来leetcodejava算法
力扣每日一练之字符串Day6前面的话大家好!本篇文章将介绍2周搞定数据结构的题,本文将以三道题作为背景,介绍经典的数独以及排序算法,展示语言为java(博主学习语言为java)。今天呢,是博主开始刷力扣的第五天,如果有想要开始准备自己的算法面试的同学,可以跟着我的脚步一起,共同进步。大家都是并肩作战的伙伴,一起努力奋力前行,路漫漫其修远兮,吾将上下而求索,相信我们一定都可以拿到自己期望的offer
- C语言的一些容易忘的语法细节(长期记录)
嵌入式Tlog
c语言开发语言单片机iotmcu物联网
C语言的一些冷门语法c语言|和||c语言|和||在C语言中,|和||是两种不同的运算符,分别用于位运算和逻辑运算。以下是它们的核心区别和用法:|(按位或)用途:对两个整数的二进制位进行逐位比较。规则:对应二进制位中至少有一个为1,则结果为1;否则为0。示例:inta=5;//二进制0101intb=3;//二进制0011intc=a|b;//结果0111(十进制7)特点:操作数必须是整数类型(如i
- LeetCode第37题_解数独
@蓝莓果粒茶
算法leetcode算法职场和发展c#unity数据结构游戏
LeetCode第37题:解数独题目描述编写一个程序,通过填充空格来解决数独问题。数独的解法需遵循如下规则:数字1-9在每一行只能出现一次。数字1-9在每一列只能出现一次。数字1-9在每一个以粗实线分隔的3x3宫内只能出现一次。(请参考示例图)数独部分空格内已填入了数字,空白格用‘.’表示。难度困难题目链接点击在LeetCode中查看题目示例示例1:输入:board=[["5","3",".","
- ztree设置禁用节点
3213213333332132
JavaScriptztreejsonsetDisabledNodeAjax
ztree设置禁用节点的时候注意,当使用ajax后台请求数据,必须要设置为同步获取数据,否者会获取不到节点对象,导致设置禁用没有效果。
$(function(){
showTree();
setDisabledNode();
});
- JVM patch by Taobao
bookjovi
javaHotSpot
在网上无意中看到淘宝提交的hotspot patch,共四个,有意思,记录一下。
7050685:jsdbproc64.sh has a typo in the package name
7058036:FieldsAllocationStyle=2 does not work in 32-bit VM
7060619:C1 should respect inline and
- 将session存储到数据库中
dcj3sjt126com
sqlPHPsession
CREATE TABLE sessions (
id CHAR(32) NOT NULL,
data TEXT,
last_accessed TIMESTAMP NOT NULL,
PRIMARY KEY (id)
);
<?php
/**
* Created by PhpStorm.
* User: michaeldu
* Date
- Vector
171815164
vector
public Vector<CartProduct> delCart(Vector<CartProduct> cart, String id) {
for (int i = 0; i < cart.size(); i++) {
if (cart.get(i).getId().equals(id)) {
cart.remove(i);
- 各连接池配置参数比较
g21121
连接池
排版真心费劲,大家凑合看下吧,见谅~
Druid
DBCP
C3P0
Proxool
数据库用户名称 Username Username User
数据库密码 Password Password Password
驱动名
- [简单]mybatis insert语句添加动态字段
53873039oycg
mybatis
mysql数据库,id自增,配置如下:
<insert id="saveTestTb" useGeneratedKeys="true" keyProperty="id"
parameterType=&
- struts2拦截器配置
云端月影
struts2拦截器
struts2拦截器interceptor的三种配置方法
方法1. 普通配置法
<struts>
<package name="struts2" extends="struts-default">
&
- IE中页面不居中,火狐谷歌等正常
aijuans
IE中页面不居中
问题是首页在火狐、谷歌、所有IE中正常显示,列表页的页面在火狐谷歌中正常,在IE6、7、8中都不中,觉得可能那个地方设置的让IE系列都不认识,仔细查看后发现,列表页中没写HTML模板部分没有添加DTD定义,就是<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://www.w3
- String,int,Integer,char 几个类型常见转换
antonyup_2006
htmlsql.net
如何将字串 String 转换成整数 int?
int i = Integer.valueOf(my_str).intValue();
int i=Integer.parseInt(str);
如何将字串 String 转换成Integer ?
Integer integer=Integer.valueOf(str);
如何将整数 int 转换成字串 String ?
1.
- PL/SQL的游标类型
百合不是茶
显示游标(静态游标)隐式游标游标的更新和删除%rowtyperef游标(动态游标)
游标是oracle中的一个结果集,用于存放查询的结果;
PL/SQL中游标的声明;
1,声明游标
2,打开游标(默认是关闭的);
3,提取数据
4,关闭游标
注意的要点:游标必须声明在declare中,使用open打开游标,fetch取游标中的数据,close关闭游标
隐式游标:主要是对DML数据的操作隐
- JUnit4中@AfterClass @BeforeClass @after @before的区别对比
bijian1013
JUnit4单元测试
一.基础知识
JUnit4使用Java5中的注解(annotation),以下是JUnit4常用的几个annotation: @Before:初始化方法 对于每一个测试方法都要执行一次(注意与BeforeClass区别,后者是对于所有方法执行一次)@After:释放资源 对于每一个测试方法都要执行一次(注意与AfterClass区别,后者是对于所有方法执行一次
- 精通Oracle10编程SQL(12)开发包
bijian1013
oracle数据库plsql
/*
*开发包
*包用于逻辑组合相关的PL/SQL类型(例如TABLE类型和RECORD类型)、PL/SQL项(例如游标和游标变量)和PL/SQL子程序(例如过程和函数)
*/
--包用于逻辑组合相关的PL/SQL类型、项和子程序,它由包规范和包体两部分组成
--建立包规范:包规范实际是包与应用程序之间的接口,它用于定义包的公用组件,包括常量、变量、游标、过程和函数等
--在包规
- 【EhCache二】ehcache.xml配置详解
bit1129
ehcache.xml
在ehcache官网上找了多次,终于找到ehcache.xml配置元素和属性的含义说明文档了,这个文档包含在ehcache.xml的注释中!
ehcache.xml : http://ehcache.org/ehcache.xml
ehcache.xsd : http://ehcache.org/ehcache.xsd
ehcache配置文件的根元素是ehcahe
ehcac
- java.lang.ClassNotFoundException: org.springframework.web.context.ContextLoaderL
白糖_
javaeclipsespringtomcatWeb
今天学习spring+cxf的时候遇到一个问题:在web.xml中配置了spring的上下文监听器:
<listener>
<listener-class>org.springframework.web.context.ContextLoaderListener</listener-class>
</listener>
随后启动
- angular.element
boyitech
AngularJSAngularJS APIangular.element
angular.element
描述: 包裹着一部分DOM element或者是HTML字符串,把它作为一个jQuery元素来处理。(类似于jQuery的选择器啦) 如果jQuery被引入了,则angular.element就可以看作是jQuery选择器,选择的对象可以使用jQuery的函数;如果jQuery不可用,angular.e
- java-给定两个已排序序列,找出共同的元素。
bylijinnan
java
import java.util.ArrayList;
import java.util.Arrays;
import java.util.List;
public class CommonItemInTwoSortedArray {
/**
* 题目:给定两个已排序序列,找出共同的元素。
* 1.定义两个指针分别指向序列的开始。
* 如果指向的两个元素
- sftp 异常,有遇到的吗?求解
Chen.H
javajcraftauthjschjschexception
com.jcraft.jsch.JSchException: Auth cancel
at com.jcraft.jsch.Session.connect(Session.java:460)
at com.jcraft.jsch.Session.connect(Session.java:154)
at cn.vivame.util.ftp.SftpServerAccess.connec
- [生物智能与人工智能]神经元中的电化学结构代表什么?
comsci
人工智能
我这里做一个大胆的猜想,生物神经网络中的神经元中包含着一些化学和类似电路的结构,这些结构通常用来扮演类似我们在拓扑分析系统中的节点嵌入方程一样,使得我们的神经网络产生智能判断的能力,而这些嵌入到节点中的方程同时也扮演着"经验"的角色....
我们可以尝试一下...在某些神经
- 通过LAC和CID获取经纬度信息
dai_lm
laccid
方法1:
用浏览器打开http://www.minigps.net/cellsearch.html,然后输入lac和cid信息(mcc和mnc可以填0),如果数据正确就可以获得相应的经纬度
方法2:
发送HTTP请求到http://www.open-electronics.org/celltrack/cell.php?hex=0&lac=<lac>&cid=&
- JAVA的困难分析
datamachine
java
前段时间转了一篇SQL的文章(http://datamachine.iteye.com/blog/1971896),文章不复杂,但思想深刻,就顺便思考了一下java的不足,当砖头丢出来,希望引点和田玉。
-----------------------------------------------------------------------------------------
- 小学5年级英语单词背诵第二课
dcj3sjt126com
englishword
money 钱
paper 纸
speak 讲,说
tell 告诉
remember 记得,想起
knock 敲,击,打
question 问题
number 数字,号码
learn 学会,学习
street 街道
carry 搬运,携带
send 发送,邮寄,发射
must 必须
light 灯,光线,轻的
front
- linux下面没有tree命令
dcj3sjt126com
linux
centos p安装
yum -y install tree
mac os安装
brew install tree
首先来看tree的用法
tree 中文解释:tree
功能说明:以树状图列出目录的内容。
语 法:tree [-aACdDfFgilnNpqstux][-I <范本样式>][-P <范本样式
- Map迭代方式,Map迭代,Map循环
蕃薯耀
Map循环Map迭代Map迭代方式
Map迭代方式,Map迭代,Map循环
>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>
蕃薯耀 2015年
- Spring Cache注解+Redis
hanqunfeng
spring
Spring3.1 Cache注解
依赖jar包:
<!-- redis -->
<dependency>
<groupId>org.springframework.data</groupId>
<artifactId>spring-data-redis</artifactId>
- Guava中针对集合的 filter和过滤功能
jackyrong
filter
在guava库中,自带了过滤器(filter)的功能,可以用来对collection 进行过滤,先看例子:
@Test
public void whenFilterWithIterables_thenFiltered() {
List<String> names = Lists.newArrayList("John"
- 学习编程那点事
lampcy
编程androidPHPhtml5
一年前的夏天,我还在纠结要不要改行,要不要去学php?能学到真本事吗?改行能成功吗?太多的问题,我终于不顾一切,下定决心,辞去了工作,来到传说中的帝都。老师给的乘车方式还算有效,很顺利的就到了学校,赶巧了,正好学校搬到了新校区。先安顿了下来,过了个轻松的周末,第一次到帝都,逛逛吧!
接下来的周一,是我噩梦的开始,学习内容对我这个零基础的人来说,除了勉强完成老师布置的作业外,我已经没有时间和精力去
- 架构师之流处理---------bytebuffer的mark,limit和flip
nannan408
ByteBuffer
1.前言。
如题,limit其实就是可以读取的字节长度的意思,flip是清空的意思,mark是标记的意思 。
2.例子.
例子代码:
String str = "helloWorld";
ByteBuffer buff = ByteBuffer.wrap(str.getBytes());
Sy
- org.apache.el.parser.ParseException: Encountered " ":" ": "" at line 1, column 1
Everyday都不同
$转义el表达式
最近在做Highcharts的过程中,在写js时,出现了以下异常:
严重: Servlet.service() for servlet jsp threw exception
org.apache.el.parser.ParseException: Encountered " ":" ": "" at line 1,
- 用Java实现发送邮件到163
tntxia
java实现
/*
在java版经常看到有人问如何用javamail发送邮件?如何接收邮件?如何访问多个文件夹等。问题零散,而历史的回复早已经淹没在问题的海洋之中。
本人之前所做过一个java项目,其中包含有WebMail功能,当初为用java实现而对javamail摸索了一段时间,总算有点收获。看到论坛中的经常有此方面的问题,因此把我的一些经验帖出来,希望对大家有些帮助。
此篇仅介绍用
- 探索实体类存在的真正意义
java小叶檀
POJO
一. 实体类简述
实体类其实就是俗称的POJO,这种类一般不实现特殊框架下的接口,在程序中仅作为数据容器用来持久化存储数据用的
POJO(Plain Old Java Objects)简单的Java对象
它的一般格式就是
public class A{
private String id;
public Str