- 数字转换(dp+数论)
小崔的技术博客
算法
题意:如果一个数x的约数之和y(不包括他本身)比他本身小,那么x可以变成y,y也可以变成x。例如,4可以变为3,1可以变为7。限定所有数字变换在不超过n的正整数范围内进行,求不断进行数字变换且不出现重复数字的最多变换步数。思路:可以将每个数与能到达的数之间连一条边,这样就会形成一个森林,而题目要求的就是在森林中找一棵树的最大直径。问题转换为求树的最大直径:第一步:用筛法的变形求每个数的约数之和第二
- 基础算法--欧拉函数
不会搬砖的淡水鱼
基础算法算法java数据结构
欧拉函数(Euler’stotientfunction),也称为费马函数,是一个与正整数相关的数论函数,用符号φ(n)表示。欧拉函数φ(n)定义为小于或等于n的正整数中与n互质的数的个数。RSA加密算法(Rivest-Shamir-Adleman)就是通过欧拉函数进行公钥加密。具体而言,对于给定的正整数n,欧拉函数φ(n)计算满足以下条件的k的个数:1≤k≤n,且k与n互质(即k和n的最大公约数为
- CSS语言的数论算法
宇瞳月
包罗万象golang开发语言后端
CSS语言的数论算法引言数论作为数学的一个重要分支,主要研究整数及其性质。数论的基本问题包括素数的性质、最大公约数、最小公倍数、同余等,同时数论在密码学、计算机科学等领域具有广泛的应用。而CSS(层叠样式表)本身是一种样式表语言,用于控制HTML文档的样式和布局,虽然CSS本身并不能直接进行复杂的数论运算,但它可以和JavaScript等编程语言结合使用,实现数论算法的可视化与交互。本文将探讨数论
- 小凯的疑惑(数论 )
vir02
算法数据结构c++
#includeusingnamespacestd;typedeflonglongll;intmain(){//请在此输入您的代码lla,b;cin>>a>>b;llN=a*b-a-b;cout<<N;return0;}如果a和b互素,那么a*b-a-b是最大无法被表示的金额
- 论当今的精神状态...(2025.3.14)
VU-zFaith870
日常随笔模拟退火算法
好无聊好烦喏,字符串、线段树、深搜宽搜、DP还有数论...无语。最近OI那边又有西安多校集训的消息,13天的集训,多少是长点。不去是OI的溃败,去了就是whk的惨退。挺纠结,跟家长聊聊吧,大抵是不同意i,我也不打算去,现在OI是有点紧张,但文化成绩别退啊,很难受...我还是习惯在学校安然自得地静心学习,闲暇时放松身心,焦虑时做些心理工作(去找心理老师不错),迷茫时还有身边的一切。因为我眷恋这里..
- CF576A Vasya and Petya‘s Game 题解
W9095
算法学习笔记c++
CF576AVasyaandPetya’sGame数论思维题。根据唯一分解定理,可以知道,如果一个数的各个质因数的数量确定了,这个数也就确定了。每次询问的中,如果xxx是yyy的倍数,证明xxx中含yyy的所有质因数。我们可以枚举质数,判定xxx能否整除这个质数,就可以判断xxx是否含有这个质因数。但是这还不能完全确定xxx,因为这样只能确定是否有某个质因数,而不能确定质因数的数量。为了确定质因数
- leetcode 2024春招冲刺百题计划——动态规划+数论
云深沐子兮
leetcode算法
不打算充钱第一次用java写,有点不熟悉。。。还是用c+stl爽。没写完,不定期更新。在忙八股,先发出来吧,万一有人需要呢先更数论和动态规划目录动态规划篇数论篇动态规划篇70.爬楼梯一眼斐波那契数列。想更进一步可以找一下矩阵写法。classSolution{publicintclimbStairs(intn){if(n==1)return1;elseif(n==2)return2;intsum=0
- 数论-1智乃的数字
幽影欧门
数论c++牛客
链接:登录—专业IT笔试面试备考平台_牛客网题目描述如果一个奇数满足以下两个条件之一:以555结尾各个数位相加的和是333的倍数则称它是一个"智数"前555个"智数"分别为{3,5,9,15,21}\{3,5,9,15,21\}{3,5,9,15,21}现在智乃想要你给升序排序第kkk个"智数"输入描述:第一行输入一个正整数T(1≤T≤105)T(1\leqT\leq10^5)T(1≤T≤105)
- 素数筛介绍,C++实现
非德77
c++算法开发语言密码学
一、素数在数学的奇妙世界里,素数是一个独特而又基础的概念。素数,也被称为质数,是指在大于1的自然数中,除了1和它自身外,不能被其他自然数整除的数。例如,2、3、5、7、11等都是素数,而4(能被2整除)、6(能被2和3整除)等则不是。素数在数学领域中具有举足轻重的地位,是数论等众多数学分支的核心研究对象。在计算机科学领域,素数也有着广泛的应用,比如在密码学中,RSA加密算法就依赖于大素数的性质来保
- 算法竞赛备赛——【数论】快速幂
Aurora_wmroy
算法竞赛备赛算法c++数据结构蓝桥杯
快速幂计算a的b次方时间复杂度:O(logb)#includeusingnamespacestd;constintN=1e5+9;usingll=longlong;#definemod998244353llksm(lla,llb){llres=1;//a=2b=13--1101while(b){//res=2a=2^2b=6//体现倍增思想if(b&1)res=res*a%mod;//res=2a
- php 常用bc函数
任性不起来了
phpbc函数
bcadd—加法,2个任意精度数字的加法计算bcsub—减法bcmul—乘法bcdiv—除法bcpow—乘方bcmod—取模bcsqrt—求二次方根bccomp—比较两个任意精度的数字,返回一个整数的结果:若两数相等返回0,左数大返回1,否则返回-1bcpowmod—求高精度数字乘方求模,数论里非常常用bcscale—设置所有bc数学函数的默认小数点保留位数—比较两个高精度数字,返回-1,0,1
- 【算法学习之路】4.简单数论(4)
零零时
算法学习之路算法学习c++开发语言数据结构数学高精度
简单数论(4)前言三.高精度1.什么是高精度2.解决办法精度乘除法一.精度乘法1.数据的存储2.步骤3.例题:高精度乘法二.精度除法1.例子2.步骤3.例题:高精度除法前言我会将一些常用的算法以及对应的题单给写完,形成一套完整的算法体系,以及大量的各个难度的题目,目前算法也写了几篇,滑动窗口的题单正在更新,其他的也会陆陆续续的更新,希望大家点赞收藏我会尽快更新的!!!三.高精度1.什么是高精度对运
- 欧拉定理
GocNeverGiveUp
数论基础
今天上午近代史和英语又看了看数论,看到了这个费马-欧拉定理,之前还真没见过,只是知道欧拉函数打表欧拉函数φ欧拉定理是用来阐述素数模下,指数同余的性质。欧拉定理:对于正整数N,代表小于等于N的与N互质的数的个数,记作φ(N)例如φ(8)=4,因为与8互质且小于等于8的正整数有4个,它们是:1,3,5,7欧拉定理还有几个引理,具体如下:①:如果n为某一个素数p,则φ(p)=p-1;①很好证明:因为素数
- 【数论 二分查找】P7588 双重素数(2021 CoE-II A)|普及
闻缺陷则喜何志丹
#洛谷普及算法c++洛谷数学二分查找数论位和
本文涉及的基础知识点C++二分查找数论:质数、最大公约数、菲蜀定理双重素数(2021CoE-IIA)题目描述素数(质数)是指在大于111的自然数中,除了111和它本身以外不再有其他因数的自然数。定义双重素数为这样的素数:它的各位数字之和也是一个素数。给定一个闭区间,试确定在该区间内双重素数的个数。输入格式输入包含多组测试数据。输入第一行包含一个整数TTT,表示测试数据的组数。接下来每行一组测试数据
- 【算法】初等数论
非 白
算法开发语言java
初等数论模取余,遵循尽可能让商向0靠近的原则,结果的正负和左操作数相同取模,遵循尽可能让商向负无穷靠近的原则,结果的正负和右操作数相同7/(-3)=-2.3,产生了两个商-2和-3,取余语言中取-2,导致余数为1;取模语言中取-3,导致余数为-2java中%是取余幂1、暴力幂思想:直接将a连续乘以b遍时间复杂度:O(n)空间复杂度:O(1)//求a^bpubliclongpow(inta,intb
- python | math --- 数学函数
黄佳俊、
Pythonpython
这些函数不适用于复数;如果你需要计算复数,请使用cmath模块中的同名函数。常用数论与表示函数math.ceil(x)返回x的上限,即大于或者等于x的最小整数。如果x不是一个浮点数,则委托x.__ceil__(),返回一个Integral类的值。math.fabs(x)返回x的绝对值。math.factorial(x)以一个整数返回x的阶乘。如果x不是整数或为负数时则将引发ValueError。3
- poj 1142 Smith Numbers(数论:欧拉函数变形)
殷华
数学/数论
给定一个数n找出大于n的最小smith数smith数定义如下:一个数n为smith数当且仅当它的所有质因子各位数之和等于n的所有位数之和且n不是素数那么给定一个n,我们就可以每次+1判断是否为smith数这道题唯一的难点就在于找到一个数的所有素数因子套用欧拉函数变形即可375ms代码如下:#include#include#defineLLlonglongLLn;intget_ans(LLn){in
- 探索约数:试除法,约数之和,最大公约数
Lostgreen
数据结构&算法算法最大公约数
引言约数(Divisor)是数论中的基本概念之一,指能够整除某个数的整数。约数在数学、计算机科学和密码学中有着广泛的应用。本文将详细介绍约数的相关知识,包括试除法求约数、最大公约数算法(如辗转相除法和更相减损术),并阐明这些算法的原理和步骤。1.试除法求约数1.1算法原理试除法是一种简单直观的求约数的方法。对于一个数nnn,如果ddd是nnn的约数,则nnn能被ddd整除。通过遍历1到n\sqrt
- ACM培训4
ZIZIZIZIZ()
算法笔记
学习总结--基础数论大多为模板一、GCD(最大公约数)①辗转相除法longlonggcd(longa,longb){longlongr;while(b!=0){r=a%b;a=b;b=r;}returna;}②扩展欧几里得算法intexgcd(inta,intb,int&x,int&y){if(b==0){x=1;y=0;returnaa;}intans=exgcd(b,a%b,x,y);intk
- 【数论】—— 素数
Tom_wsc
数论算法
素数定义因数只有111和这个数本身的数被称作素数。注意:111既不是素数也不是合数,222是最小的素数。两个关于素数的定理唯一分解定理对于任意大于111的整数xxx,都可以分解成若干个素数的乘积:x=p1a1×p2a2×p3a3×⋯×pnan(ai∈Z+)x=p_1^{a_1}\timesp_2^{a_2}\timesp_3^{a_3}\times\cdots\timesp_n^{a_n}(a_i
- 【运行别超时】最近小何去在我们学校的比赛中遇到一个有意思的题,答案做出来了,但运行总是超时。这怎么解决呢?来看看吧。
小浩~
c语言
题目内容如下:小C最近在研究数论,他发现质数有太多美妙的性质了,于是他想要统计一下一段区域里的数有多少是质数,请你编程帮他解决这个问题吧。输入格式:第一行一个正整数t,表示数据组数。(1≤t≤105)接下来t行,每行两个正整数l,r,表示区间的左右端点。(1≤l≤r≤106)输出格式:每组数据输出一个整数,表示闭区间[l,r]中的质数数量输入样例:21326输出样例:在这里给出相应的输出。例如:2
- 2025年日祭
JeremyHe1209
笔记
本文将同步发表于洛谷(暂无法访问)、CSDN与Github个人博客(暂未发布)本蒟自2025.2.8开始半停课。任务计划(站外题与专题)数了一下,通过人数比较高的题,也就是我准备补的题,刚好差不多100道题。于是……摆烂百题计划开始!(糖丸了)(2025.2.8)NetworkNetworkofSchoolsDP优化——矩阵数论——容斥、二项式反演DP优化——斜率优化数据结构——左偏树数据结构——
- 解析数论基础:第三十三章 零点分布(二)
AI天才研究院
计算AI大模型企业级应用开发实战DeepSeekR1&大数据AI人工智能大模型计算科学神经计算深度学习神经网络大数据人工智能大型语言模型AIAGILLMJavaPython架构设计AgentRPA
解析数论基础:第三十三章零点分布(二)作者:禅与计算机程序设计艺术/ZenandtheArtofComputerProgramming关键词:解析数论、黎曼ζ函数、零点分布、素数定理、蒙哥马利猜想、配对相关函数、随机矩阵理论1.背景介绍1.1问题的由来解析数论是现代数学的重要分支,它利用复变函数论等分析学的方法研究数论问题。其中一个核心课题就是研究黎曼ζ函数的性质,特别是它的零点分布。这个问题不仅
- 【密码学基础】RSA加密算法
Mr.zwX
隐私计算及密码学基础密码学安全
1RSA介绍RSA是一种非对称加密算法,即加密和解密时用到的密钥不同。加密密钥是公钥,可以公开;解密密钥是私钥,必须保密保存。基于一个简单的数论事实:两个大质数相乘很容易,但想要对其乘积进行因式分解却极其困难,因此可以将乘积公开作为加密密钥,即公钥;而两个大质数组合成私钥。2密钥对的生成step1生成N(公钥和私钥的一部分)首先选取两个互为质数的数ppp和qqq(p≠q,gcd(p,q)=1p\n
- 数论问题79一一研究成果
李扩继
数据分析深度学习学习方法算法数学建模
(豆包智能搜索一一李扩继)李扩继是一位在数学研究尤其是哥德巴赫猜想研究领域有一定成果的中学老师,以下是关于他的具体介绍:①研究经历:2006年承担咸阳市教研室的立项课题《角谷猜想的研究》,虽未完成角谷猜想的证明,但在意外灵感下开始对哥德巴赫猜想展开持续性研究工作。②发表论文:研究哥德巴赫猜想发表了多篇文章,如2008年的《哥德巴赫猜想的证明》、2010年的《哥德巴赫猜想的“1+1”证明》、2017
- 【算法学习之路】4.简单数论(2)
零零时
算法学习之路算法学习数据结构笔记经验分享
简单数论(2)前言二.快速幂1.什么是快速幂2.前置知识2.1进制转化2.2短除法2.3普通转换法3.快速幂3.1原理3.2代码4.拓展4.1模运算法则4.2题目前言我会将一些常用的算法以及对应的题单给写完,形成一套完整的算法体系,以及大量的各个难度的题目,目前算法也写了几篇,滑动窗口的题单正在更新,其他的也会陆陆续续的更新,希望大家点赞收藏我会尽快更新的!!!二.快速幂1.什么是快速幂快速幂是一
- 数论问题77一一3x+1问题
李扩继
深度学习学习方法算法数学建模数据分析
3X+1问题,也被称为考拉兹猜想、角谷猜想等,是数学领域一个著名的未解决问题,以下是关于它的介绍:问题表述对于任意一个正整数X,如果X是奇数,则将其变为3X+1;如果X是偶数,则将其变为X/2。不断重复这个过程,最终是否无论初始值X是多少,都会经过有限次变换后最终得到1。例如,取X=5,它是奇数,进行3X+1操作得到3×5+1=16;16是偶数,进行X/2操作得到16÷2=8,接着8÷2=4,4÷
- 数论问题76一一容斥原理
李扩继
深度学习数学建模大数据学习方法算法
容斥原理是一种计数方法,用于计算多个集合的并集中元素的个数,以避免重复计算。以下是其基本内容及相关公式:两个集合的容斥原理若有集合A和集合B,那么A与B的并集中元素的个数等于A集合元素个数加上B集合元素个数,再减去A与B交集的元素个数,即|AUB|=|A|+|B|-|A∧B|。例如,一个班级中喜欢数学的有30人,喜欢语文的有25人,既喜欢数学又喜欢语文的有10人。那么喜欢数学或语文的人数为30+2
- 【数论】Acwing质数与约数
九年义务漏网鲨鱼
算法python算法数论质数约数
质数质数的判定(试除法)除了开方的数,其他因数都是成对出现的defis_prime(x):if(x<2)returnFalseforiinrange(2,int(x/i)+1):if(x%iW==0):returnFalsereturnTrue分解质因数defdivide(x):foriinrange(2,int(x/i)+1):if(x%i==0):s=0while(x%i==0):x//=is
- 数论(三)——约数(约数个数,约数和,公约数)
DearLife丶
#数学知识算法gcd约数欧几里德算法
目录试除法求约数求约数个数约数之和欧几里得算法试除法求约数试除法求一个数的所有约数,思路与判断质数的思路一样,优化的方法也是一样的,这里就不再赘述,没有看过我之前关于质数的博客可以点这里。从小到大枚举所有约数,但是我们只需要枚举每一对儿中较小的一个就可以了。时间复杂度:O(sqrt(n))vectorget_divisors(intn){vectorres;//vector数组存储一个数的所有约数
- 关于旗正规则引擎规则中的上传和下载问题
何必如此
文件下载压缩jsp文件上传
文件的上传下载都是数据流的输入输出,大致流程都是一样的。
一、文件打包下载
1.文件写入压缩包
string mainPath="D:\upload\"; 下载路径
string tmpfileName=jar.zip; &n
- 【Spark九十九】Spark Streaming的batch interval时间内的数据流转源码分析
bit1129
Stream
以如下代码为例(SocketInputDStream):
Spark Streaming从Socket读取数据的代码是在SocketReceiver的receive方法中,撇开异常情况不谈(Receiver有重连机制,restart方法,默认情况下在Receiver挂了之后,间隔两秒钟重新建立Socket连接),读取到的数据通过调用store(textRead)方法进行存储。数据
- spark master web ui 端口8080被占用解决方法
daizj
8080端口占用sparkmaster web ui
spark master web ui 默认端口为8080,当系统有其它程序也在使用该接口时,启动master时也不会报错,spark自己会改用其它端口,自动端口号加1,但为了可以控制到指定的端口,我们可以自行设置,修改方法:
1、cd SPARK_HOME/sbin
2、vi start-master.sh
3、定位到下面部分
- oracle_执行计划_谓词信息和数据获取
周凡杨
oracle执行计划
oracle_执行计划_谓词信息和数据获取(上)
一:简要说明
在查看执行计划的信息中,经常会看到两个谓词filter和access,它们的区别是什么,理解了这两个词对我们解读Oracle的执行计划信息会有所帮助。
简单说,执行计划如果显示是access,就表示这个谓词条件的值将会影响数据的访问路径(表还是索引),而filter表示谓词条件的值并不会影响数据访问路径,只起到
- spring中datasource配置
g21121
dataSource
datasource配置有很多种,我介绍的一种是采用c3p0的,它的百科地址是:
http://baike.baidu.com/view/920062.htm
<!-- spring加载资源文件 -->
<bean name="propertiesConfig"
class="org.springframework.b
- web报表工具FineReport使用中遇到的常见报错及解决办法(三)
老A不折腾
finereportFAQ报表软件
这里写点抛砖引玉,希望大家能把自己整理的问题及解决方法晾出来,Mark一下,利人利己。
出现问题先搜一下文档上有没有,再看看度娘有没有,再看看论坛有没有。有报错要看日志。下面简单罗列下常见的问题,大多文档上都有提到的。
1、repeated column width is largerthan paper width:
这个看这段话应该是很好理解的。比如做的模板页面宽度只能放
- mysql 用户管理
墙头上一根草
linuxmysqluser
1.新建用户 //登录MYSQL@>mysql -u root -p@>密码//创建用户mysql> insert into mysql.user(Host,User,Password) values(‘localhost’,'jeecn’,password(‘jeecn’));//刷新系统权限表mysql>flush privileges;这样就创建了一个名为:
- 关于使用Spring导致c3p0数据库死锁问题
aijuans
springSpring 入门Spring 实例Spring3Spring 教程
这个问题我实在是为整个 springsource 的员工蒙羞
如果大家使用 spring 控制事务,使用 Open Session In View 模式,
com.mchange.v2.resourcepool.TimeoutException: A client timed out while waiting to acquire a resource from com.mchange.
- 百度词库联想
annan211
百度
<!DOCTYPE html>
<html>
<head>
<meta http-equiv="Content-Type" content="text/html; charset=UTF-8">
<title>RunJS</title&g
- int数据与byte之间的相互转换实现代码
百合不是茶
位移int转bytebyte转int基本数据类型的实现
在BMP文件和文件压缩时需要用到的int与byte转换,现将理解的贴出来;
主要是要理解;位移等概念 http://baihe747.iteye.com/blog/2078029
int转byte;
byte转int;
/**
* 字节转成int,int转成字节
* @author Administrator
*
- 简单模拟实现数据库连接池
bijian1013
javathreadjava多线程简单模拟实现数据库连接池
简单模拟实现数据库连接池
实例1:
package com.bijian.thread;
public class DB {
//private static final int MAX_COUNT = 10;
private static final DB instance = new DB();
private int count = 0;
private i
- 一种基于Weblogic容器的鉴权设计
bijian1013
javaweblogic
服务器对请求的鉴权可以在请求头中加Authorization之类的key,将用户名、密码保存到此key对应的value中,当然对于用户名、密码这种高机密的信息,应该对其进行加砂加密等,最简单的方法如下:
String vuser_id = "weblogic";
String vuse
- 【RPC框架Hessian二】Hessian 对象序列化和反序列化
bit1129
hessian
任何一个对象从一个JVM传输到另一个JVM,都要经过序列化为二进制数据(或者字符串等其他格式,比如JSON),然后在反序列化为Java对象,这最后都是通过二进制的数据在不同的JVM之间传输(一般是通过Socket和二进制的数据传输),本文定义一个比较符合工作中。
1. 定义三个POJO
Person类
package com.tom.hes
- 【Hadoop十四】Hadoop提供的脚本的功能
bit1129
hadoop
1. hadoop-daemon.sh
1.1 启动HDFS
./hadoop-daemon.sh start namenode
./hadoop-daemon.sh start datanode
通过这种逐步启动的方式,比start-all.sh方式少了一个SecondaryNameNode进程,这不影响Hadoop的使用,其实在 Hadoop2.0中,SecondaryNa
- 中国互联网走在“灰度”上
ronin47
管理 灰度
中国互联网走在“灰度”上(转)
文/孕峰
第一次听说灰度这个词,是任正非说新型管理者所需要的素质。第二次听说是来自马化腾。似乎其他人包括马云也用不同的语言说过类似的意思。
灰度这个词所包含的意义和视野是广远的。要理解这个词,可能同样要用“灰度”的心态。灰度的反面,是规规矩矩,清清楚楚,泾渭分明,严谨条理,是决不妥协,不转弯,认死理。黑白分明不是灰度,像彩虹那样
- java-51-输入一个矩阵,按照从外向里以顺时针的顺序依次打印出每一个数字。
bylijinnan
java
public class PrintMatrixClockwisely {
/**
* Q51.输入一个矩阵,按照从外向里以顺时针的顺序依次打印出每一个数字。
例如:如果输入如下矩阵:
1 2 3 4
5 6 7 8
9
- mongoDB 用户管理
开窍的石头
mongoDB用户管理
1:添加用户
第一次设置用户需要进入admin数据库下设置超级用户(use admin)
db.addUsr({user:'useName',pwd:'111111',roles:[readWrite,dbAdmin]});
第一个参数用户的名字
第二个参数
- [游戏与生活]玩暗黑破坏神3的一些问题
comsci
生活
暗黑破坏神3是有史以来最让人激动的游戏。。。。但是有几个问题需要我们注意
玩这个游戏的时间,每天不要超过一个小时,且每次玩游戏最好在白天
结束游戏之后,最好在太阳下面来晒一下身上的暗黑气息,让自己恢复人的生气
&nb
- java 二维数组如何存入数据库
cuiyadll
java
using System;
using System.Linq;
using System.Text;
using System.Windows.Forms;
using System.Xml;
using System.Xml.Serialization;
using System.IO;
namespace WindowsFormsApplication1
{
- 本地事务和全局事务Local Transaction and Global Transaction(JTA)
darrenzhu
javaspringlocalglobaltransaction
Configuring Spring and JTA without full Java EE
http://spring.io/blog/2011/08/15/configuring-spring-and-jta-without-full-java-ee/
Spring doc -Transaction Management
http://docs.spring.io/spri
- Linux命令之alias - 设置命令的别名,让 Linux 命令更简练
dcj3sjt126com
linuxalias
用途说明
设置命令的别名。在linux系统中如果命令太长又不符合用户的习惯,那么我们可以为它指定一个别名。虽然可以为命令建立“链接”解决长文件名的问 题,但对于带命令行参数的命令,链接就无能为力了。而指定别名则可以解决此类所有问题【1】。常用别名来简化ssh登录【见示例三】,使长命令变短,使常 用的长命令行变短,强制执行命令时询问等。
常用参数
格式:alias
格式:ali
- yii2 restful web服务[格式响应]
dcj3sjt126com
PHPyii2
响应格式
当处理一个 RESTful API 请求时, 一个应用程序通常需要如下步骤 来处理响应格式:
确定可能影响响应格式的各种因素, 例如媒介类型, 语言, 版本, 等等。 这个过程也被称为 content negotiation。
资源对象转换为数组, 如在 Resources 部分中所描述的。 通过 [[yii\rest\Serializer]]
- MongoDB索引调优(2)——[十]
eksliang
mongodbMongoDB索引优化
转载请出自出处:http://eksliang.iteye.com/blog/2178555 一、概述
上一篇文档中也说明了,MongoDB的索引几乎与关系型数据库的索引一模一样,优化关系型数据库的技巧通用适合MongoDB,所有这里只讲MongoDB需要注意的地方 二、索引内嵌文档
可以在嵌套文档的键上建立索引,方式与正常
- 当滑动到顶部和底部时,实现Item的分离效果的ListView
gundumw100
android
拉动ListView,Item之间的间距会变大,释放后恢复原样;
package cn.tangdada.tangbang.widget;
import android.annotation.TargetApi;
import android.content.Context;
import android.content.res.TypedArray;
import andr
- 程序员用HTML5制作的爱心树表白动画
ini
JavaScriptjqueryWebhtml5css
体验效果:http://keleyi.com/keleyi/phtml/html5/31.htmHTML代码如下:
<!DOCTYPE html>
<html xmlns="http://www.w3.org/1999/xhtml"><head><meta charset="UTF-8" >
<ti
- 预装windows 8 系统GPT模式的ThinkPad T440改装64位 windows 7旗舰版
kakajw
ThinkPad预装改装windows 7windows 8
该教程具有普遍参考性,特别适用于联想的机器,其他品牌机器的处理过程也大同小异。
该教程是个人多次尝试和总结的结果,实用性强,推荐给需要的人!
缘由
小弟最近入手笔记本ThinkPad T440,但是特别不能习惯笔记本出厂预装的Windows 8系统,而且厂商自作聪明地预装了一堆没用的应用软件,消耗不少的系统资源(本本的内存为4G,系统启动完成时,物理内存占用比
- Nginx学习笔记
mcj8089
nginx
一、安装nginx 1、在nginx官方网站下载一个包,下载地址是:
http://nginx.org/download/nginx-1.4.2.tar.gz
2、WinSCP(ftp上传工
- mongodb 聚合查询每天论坛链接点击次数
qiaolevip
每天进步一点点学习永无止境mongodb纵观千象
/* 18 */
{
"_id" : ObjectId("5596414cbe4d73a327e50274"),
"msgType" : "text",
"sendTime" : ISODate("2015-07-03T08:01:16.000Z"
- java术语(PO/POJO/VO/BO/DAO/DTO)
Luob.
DAOPOJODTOpoVO BO
PO(persistant object) 持久对象
在o/r 映射的时候出现的概念,如果没有o/r映射,就没有这个概念存在了.通常对应数据模型(数据库),本身还有部分业务逻辑的处理.可以看成是与数据库中的表相映射的java对象.最简单的PO就是对应数据库中某个表中的一条记录,多个记录可以用PO的集合.PO中应该不包含任何对数据库的操作.
VO(value object) 值对象
通
- 算法复杂度
Wuaner
Algorithm
Time Complexity & Big-O:
http://stackoverflow.com/questions/487258/plain-english-explanation-of-big-o
http://bigocheatsheet.com/
http://www.sitepoint.com/time-complexity-algorithms/
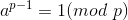
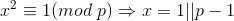
![]() ,显然
,显然![]() (q,m为任意整数)是成立的,所以
(q,m为任意整数)是成立的,所以![]() ,显然
,显然![]() .
.![]() ,
,![]() ……,
……,![]() .
.