- MATLAB学习笔记(六):MATLAB数学建模
向上的车轮
MATLAB数学建模matlab学习数学软件
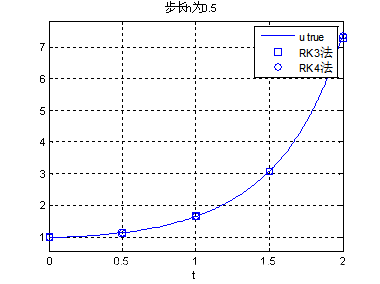
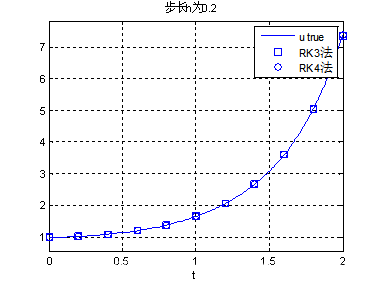
MATLAB是数学建模的强大工具,其丰富的函数库和可视化能力可以高效解决各类数学建模问题。以下是MATLAB数学建模的完整指南,涵盖建模流程、常用方法、代码示例及实际应用。一、数学建模的基本流程问题分析•明确目标(预测、优化、分类等)•确定变量与约束条件•选择数学模型类型(连续/离散、确定性/随机性)。模型构建•建立数学方程(微分方程、代数方程、统计模型等)。•确定参数与初始条件。模型求解•解析解
- Matlab学习笔记:square函数的用法
手树
matlab数字信号处理信号处理
square函数用于产生周期性矩形脉冲信号如图代码为:t=-10:0.01:10;f_0=square(t);plot(t,f_0,'LineWidth',2);一般square调用形式为y=square(t,duty)duty指占空比,在这里调用时,可以理解为,在一个周期里幅值为1的脉冲的占比,默认50%而square函数的默认周期为2*pi故想要去自己想要的周期则需要先让2*pi.*t然后除以
- MATLAB学习之旅:数据建模与仿真应用
软考和人工智能学堂
matlab学金融matlab学习开发语言
在MATLAB的学习之旅中,我们已经积累了丰富的基础知识和实用的编程技巧。从前面的学习中,我们对MATLAB的基础操作、数据处理、统计分析等方面都有了深入的了解。如今,我们将迈向一个充满创造力和实用性的阶段——数据建模与仿真应用。这部分内容将使我们能够利用MATLAB强大的功能,构建各种现实世界中的模型,并通过仿真来验证和分析这些模型的行为。数据建模的基础:定义模型结构和参数数据建模是构建真实世界
- MATLAB学习笔记——linspace
小小仙人板板
编程学习笔记matlab学习笔记
作用:返回一个等间隔的行向量,包含在指定范围内均匀分布的点标准格式:linspace(star,end,n)star:线段的起始值end:线段的结束值n:在线段上生成的点的数量(包含起始点和终止点)注:n不是无穷大eg.linspace(0,1,5);
- MATLAB学习笔记
Thomas Bob
matlab学习笔记
MATLAB学习笔记MATLAB软件使用MATLAB桌面文件命令行工作区菜单MATLAB文件的建立与保存M文件脚本文件(Script.m)脚本是一个包含多行连续的MATLAB命令和函数调用的文件。可类比于c语言中的源文件(main.c)可以单独执行可处理工作区中的数据函数文件(Function.m)函数是可接受输入参数并返回输出参数的文件。可类比于c语言中的一个函数一般不能单独执行,但可以被脚本调
- MATLAB学习笔记4
好好学习的不知名程序员
matlab笔记学习
1.变量及其操作1.1变量命名和赋值%创建变量myVariable=10;myVariable2='Hello,MATLAB!';1.2数组和矩阵%创建向量vector=[1,2,3,4];%创建矩阵matrix=[1,2,3;4,5,6;7,8,9];%访问元素element=matrix(1,2);%获取第一行第二列的元素%修改元素matrix(1,2)=10;%矩阵操作anotherMatr
- Matlab学习路线(本人)比较简洁易懂,不像教科书那样喜欢装高大上(不讲人话)
硕硕不想秃头
学习matlab笔记
(本人小白一枚,能力有限。如有改进意见十分欢迎评论或私信)第一天学习MATLAB,先学习了通过deepseek推荐的https://ww2.mathworks.cn/,matlab入门和经典MATLAB书籍,大概一上午就可以学完。MATLAB是美国MathWorks公司出品的商业数学软件,用于算法开发、数据可视化、数据分析以及数值计算的高级技术计算语言和交互式环境。20世纪70年代,美国新墨西哥大
- Matlab学习笔记五十:循环语句和条件语句的用法
freexyn
Matlab扫盲速成小短篇matlab学习笔记
1.说明循环语句:for…end,while…end条件语句:if…end,switch…case…end其中if语句语法还可以是:for…else…end,for…elseif…else…end2.简单for程序实例forx=1:5%循环遍历1~5y=x+5end[1:5]+5%对于简单循环运算,矩阵运算方式本身就可以实现3.简单if程序实例if1<2%判断条件是否满足,若不满足跳转到下一个分支
- 数字信号处理matlab学习(一)
Plan-C-
信号处理matlab学习
时域离散信号的产生一、实验用函数1、stem功能:绘制二维图形。调用格式:stem(n,x);n为横轴,x为纵轴的线性图形。2、length功能:计算某一变量的长度或采样点数。调用格式:N=length(t);计算时间向量t的个数并赋给变量N。3、axis功能:限定图形坐标的范围。
- MATLAB学习之旅:数据插值与曲线拟合
Evaporator Core
matlabmatlab学习算法
在MATLAB的奇妙世界里,我们已经走过了一段又一段的学习旅程。从基础的语法和数据处理,到如今,我们即将踏入数据插值与曲线拟合这片充满魅力的领域。这个领域就像是魔法中的艺术创作,能够让我们根据现有的数据点,构建出更加丰富的曲线和曲面,从而更好地理解和描述数据背后的规律。数据插值:用已知点填补空白数据插值是在给定的离散数据点之间,通过某种方法估算出中间未知点的方法。这就好比我们在一幅拼图中,有些碎片
- matlab学习路线
kyle~
matlabmatlab学习信息可视化
阶段1:基础入门(1-2周)目标:熟悉MATLAB界面、基础语法和简单操作。学习内容:环境与界面:了解MATLAB的桌面布局(命令窗口、工作区、编辑器、当前文件夹)。学习如何创建脚本(.m文件)和实时脚本(.mlx文件)。掌握常用快捷键(如Ctrl+R注释、F5运行脚本)。基础语法:变量定义与数据类型(数值、字符、逻辑、矩阵、cell数组、结构体)。矩阵操作(创建、索引、切片、拼接),例如:A=[
- sym和syms--Matlab学习
末时清
matlab学习算法
一、symsym是MATLAB中的一个函数,用于创建符号对象。符号对象允许你在MATLAB中进行符号计算和代数运算,而不仅仅是数值计算。使用符号对象,你可以表示符号表达式,求解方程,进行符号积分等。例如,你可以使用sym函数创建一个符号变量:symsx现在,变量x就被定义为一个符号变量,你可以使用它来构建符号表达式,比如:expr=x^2+3*x+2;这将创建一个符号表达式(x^2+3x+2)。然
- 小白入门MATLAB学习笔记
小mushroom
matlab学习笔记
绘图:使用MATLAB绘制三叶玫瑰线公式:玫瑰线的极坐标方程为:ρ=a*sin(nθ),ρ=a*cos(nθ)用直角坐标方程表示为:x=a*sin(nθ)*cos(θ),y=a*sin(nθ)*sin(θ)其中n为玫瑰线的叶数,我们绘制三叶玫瑰线,因而公式中取n=3代码:t=-2*pi:0.01:2*pi;x=sin(3*t).*cos(t);y=2*sin(3*t).*sin(t);z=3*si
- Day25_0.1基础学习MATLAB学习小技巧总结(25)——四维图形的可视化
非常规定义M
0.1基础学习MATLAB学习matlab开发语言SIMULINK数学建模
利用空闲时间把碎片化的MATLAB知识重新系统的学习一遍,为了在这个过程中加深印象,也为了能够有所足迹,我会把自己的学习总结发在专栏中,以便学习交流。参考书目:1、《MATLAB基础教程(第三版)(薛山)》2、《MATLABR2020a完全自学一本通》之前的章节都是基础的数据运算用法,对于功课来说更加重要的内容是建模、绘图、观察数据趋势,接下来我会结合自己的使用经验,来为大家分享绘图、建模使用的小
- MATLAB学习笔记5
好好学习的不知名程序员
matlab学习笔记
1.函数的创建与使用1.1创建简单的函数在MATLAB中,你可以创建自己的函数来执行特定任务。函数通常保存在一个`.m`文件中,文件名需要和函数名相同。创建一个计算两点之间距离的函数`distance.m`functiond=distance(x1,y1,x2,y2)%计算两点之间的欧几里得距离d=sqrt((x2-x1)^2+(y2-y1)^2);end调用函数:使用函数名并传入参数即可调用函数
- 第一章 matlab 学习入门之matlab基础
CN-JackZhang
matplotlibmatlab学习开发语言
matlab系列文章目录第一章matlab学习入门之matlab基础在这一章会学习到:数据类型(数值,字符串,结构,单元数组,函数句柄,映射容器)运算符与运算(算术运算符,关系运算符,逻辑运算符)字符串处理(构造,比较,查找,替换,类型转换)矩阵基础(构造矩阵,改变矩阵维度与大小,矩阵索引,获取矩阵属性信息)文章目录matlab系列文章目录第一章matlab学习入门之matlab基础前言一、数据类
- Matlab学习笔记7 高阶绘图
犀首公孫衍
画多个图x=logspace(-1,1,100);%这个语句表示从10的-1次方到1次方,100个数y=x.^2;subplot(2,2,1);%使用subplot语句可以在一个Figure中画多个子图plot(x,y);title('Plot');subplot(2,2,2);semilogx(x,y);title('Semilogx');subplot(2,2,3);semilogy(x,y)
- MATLAB学习笔记(二)| 数组类型及矩阵的运算
lightteng
matlabmatlab矩阵学习
数组矩阵定义方法:按行输入矩阵元素构造矩阵冒号运算符构造向量和矩阵x=1:10--->相当于x=12345678910y=1:2:10y从1到10取第2个数相当于y=13579x=初值:步长:终值3.linspace函数生成等间隔向量:x=linspace(初值,终值,向量长度)4.定义复数矩阵:A=[12;34];B=[56;78];c=complex(A,B)c是以A为实部,B为虚部生成的复数
- Matlab学习记录——变量函数
Dust_Just
MATLAB学习matlab
Matlab学习记录——变量函数文章目录Matlab学习记录——变量函数前言一、变量函数二、变量函数矩阵的引用学习记录未完待续这里也期待各位的指导前言CADN上关于MATLAB的数组、变量等的介绍非常多,但是在学习过程中,笔者发现有些东西没有介绍,在此记录。一、变量函数在各种理论推导中,我们常常要使用到没有明确定义的但是有关其他变量的函数。例如,仅关于x与y的函数f,但是f的具体表达式未知。后续推
- 符号变量函数的化简
Dust_Just
MATLAB学习matlab
MATLAB学习记录——符号变量函数的化简对于复杂的符号变量函数,我们大多希望他能有一个清晰简洁的表达式,以下记录我在学习过程中遇到的问题及解决方法。文章目录MATLAB学习记录——符号变量函数的化简前言一、simplify()函数1、简单应用simplify()2、simplify(X,'IgnoreAnalyticConstraints',true)3、simplify(X,'steps',v
- MATLAB学习笔记
宽容人厚载物
matlab学习笔记
第一章MATLAB简介第二章矩阵与数值数组2.1数组的创建直接输入法直接输入法就是在命令窗口中直接输入数组中的所有元素,在输入数组时必须遵循以下的规定。2.2.载入外部数据文件载入函数(loadfunction)可以读取由MATLAB早期版本生成的包含矩阵的二进制文件,或者读取包含数值数据的文本文件。文本文件中的数字应排列成矩形,每行只能包含矩阵的一行元素,元素与元素之间用空格分隔,各行元素的个数
- 菜鸟之MATLAB学习——FM0波形生成及FFT变换
洁仔爱吃冰淇淋
MATLAB学习笔记matlab
首先声明:我是MATLAB初学者,只做笔记记录。clc;closeall;fdata=160*1000;%数据速率160kT=1/fdata;%信号周期N_sample=10;%每个周期的采样点数dt=T/N_sample;d0_1=[ones(1,N_sample/2),-1*ones(1,N_sample/2)];d0_2=[-ones(1,N_sample/2),ones(1,N_sampl
- 菜鸟之MATLAB学习——SSB & DSB调制信号及其频谱分析
洁仔爱吃冰淇淋
MATLAB学习笔记matlab
clc;closeall;fm=1;fc=10;dt=0.001;T=5;t=0:dt:T-dt;A=2;mt=A*cos(2*pi*fm*t);s_ssb=real(hilbert(mt).*exp(j*2*pi*fc*t));s_dsb=mt.*cos(2*pi*fc*t);B=fm;subplot(311),plot(t,s_ssb);fs=1/dt;N=T/dt;df=fs/N;%%%%-
- 菜鸟之MATLAB学习——NRZ & RZ& sinc信号及其频谱分析
洁仔爱吃冰淇淋
MATLAB学习笔记matlab
本人MATLAB学习小白,仅做笔记记录和分享~~clc;closeall;Ts=1;N_sample=8;dt=Ts/N_sample;N=1000;t=0:dt:(N*N_sample-1)*dt;%码型构建%gt1=ones(1,N_sample);%1s时长高电平NRZ波形gt2=[ones(1,N_sample/2),zeros(1,N_sample/2)];%RZ波形mt3=sinc((
- 菜鸟之MATLAB学习——QPSK &OQPSK信号生成及频谱分析
洁仔爱吃冰淇淋
MATLAB学习笔记matlab学习开发语言
本人MATLAB学习小白,仅做笔记记录和分享~~%qpsk&&oqpskclc;closeall;Ts=1;fc=10;N_sample=16;N_sum=100;dt=1/fc/N_sample;t=0:dt:N_sum*Ts-dt;T=dt*length(t);d1=sign(randn(1,N_sum));d2=sign(randn(1,N_sum));gt=ones(1,fc*N_samp
- matlab解代矩阵代数方程,用matlab学习线性代数_线性方程组与矩阵代数.doc
weixin_39669204
matlab解代矩阵代数方程
用matlab学习线性代数_线性方程组与矩阵代数.doc用MATLAB学习线性代数线性方程组与矩阵代数实验目的熟悉线性方程组的解法和矩阵的基本运算及性质验证。MATLAB命令本练习中用到的MATLAB命令有INV,FLOOR,RAND,TIC,TOC,RREF,ABS,MAX,ROUND,SUM,EYE,TRIU,ONES,ZEROS。本练习引入的运算有,,,’,,\。其中和表示通常标量及矩阵的加
- MATLAB学习一本通 读书笔记二
chinxue2008
学习
变量名大小写敏感局部变量存在于该函数调用中,全局变量需要使用global进行声明汉宁窗,低版本用hann替代hanning1.文件只是一串排列的指令集合2.变量存在工作区,不关闭软件,不清除不会删除3.函数与文件的区别,函数变量为局部,执行完之后将被清除4.向量化,应用于matlab的循环执行语句?5.跨空间变量传递用asignin,我们用的是save为mat文件,再调用
- matlab学习笔记(七)
14077526
matlab学习开发语言
提示:文章写完后,目录可以自动生成,如何生成可参考右边的帮助文档matlab学习笔记(七)一、LTI系统的响应impulse()函数step()函数lsim()函数系统无初态时系统有初始状态时二、使用步骤A1:A2:A3一、LTI系统的响应matlab中有专门用于求解连续系统冲激响应和阶跃响应,并绘制其时域波形的函数impulse()和step()。如果系统输入为f(t),冲激响应为h(t),系统
- matlab显示函数表达式_从零开始的matlab学习笔记——(25)微分方程
weixin_39636333
matlab显示函数表达式matlab求图像自相关CSDNmatlab用辛普森公式求积分
matlab应用——求极限,求导,求积分,解方程,函数绘图,三维图像,拟合函数....更多内容尽在个人专栏:matlab学习之前我们学习过matlab的各种解方程的函数工具,这一节我们再来学习一种,(常)微分方程的求解工具。微积分的符号表示方法:我们先了解一下怎么用符号表示导数这个变量:符号计算中,用D代表求导Dy是一阶导数,D2y是二阶导数...以此类推此外,符号计算还支持赋特殊值操作y(0)=
- matlab数值微积分与方程数值求解,六、数值微积分与方程求解(MATLAB学习笔记)
卫星88
六、数值微积分与方程求解6.1数值微分与数值积分★高等数学是解析解,MATLAB求数值解。6.1.1数值差分与差商:6.1.2数值微分的实现:向前差分函数diff(),计算向量元素间差分。调用方法:diff(x,n):计算向量x的n阶向前差分。(一阶:dx(i)=x(i+1)-x(i))diff(A,n,dim):计算矩阵A的n阶差分,(默认)dim=1,按列计算。6.1.3数值积分可用牛顿-莱布
- web前段跨域nginx代理配置
刘正强
nginxcmsWeb
nginx代理配置可参考server部分
server {
listen 80;
server_name localhost;
- spring学习笔记
caoyong
spring
一、概述
a>、核心技术 : IOC与AOP
b>、开发为什么需要面向接口而不是实现
接口降低一个组件与整个系统的藕合程度,当该组件不满足系统需求时,可以很容易的将该组件从系统中替换掉,而不会对整个系统产生大的影响
c>、面向接口编口编程的难点在于如何对接口进行初始化,(使用工厂设计模式)
- Eclipse打开workspace提示工作空间不可用
0624chenhong
eclipse
做项目的时候,难免会用到整个团队的代码,或者上一任同事创建的workspace,
1.电脑切换账号后,Eclipse打开时,会提示Eclipse对应的目录锁定,无法访问,根据提示,找到对应目录,G:\eclipse\configuration\org.eclipse.osgi\.manager,其中文件.fileTableLock提示被锁定。
解决办法,删掉.fileTableLock文件,重
- Javascript 面向对面写法的必要性?
一炮送你回车库
JavaScript
现在Javascript面向对象的方式来写页面很流行,什么纯javascript的mvc框架都出来了:ember
这是javascript层的mvc框架哦,不是j2ee的mvc框架
我想说的是,javascript本来就不是一门面向对象的语言,用它写出来的面向对象的程序,本身就有些别扭,很多人提到js的面向对象首先提的是:复用性。那么我请问你写的js里有多少是可以复用的,用fu
- js array对象的迭代方法
换个号韩国红果果
array
1.forEach 该方法接受一个函数作为参数, 对数组中的每个元素
使用该函数 return 语句失效
function square(num) {
print(num, num * num);
}
var nums = [1,2,3,4,5,6,7,8,9,10];
nums.forEach(square);
2.every 该方法接受一个返回值为布尔类型
- 对Hibernate缓存机制的理解
归来朝歌
session一级缓存对象持久化
在hibernate中session一级缓存机制中,有这么一种情况:
问题描述:我需要new一个对象,对它的几个字段赋值,但是有一些属性并没有进行赋值,然后调用
session.save()方法,在提交事务后,会出现这样的情况:
1:在数据库中有默认属性的字段的值为空
2:既然是持久化对象,为什么在最后对象拿不到默认属性的值?
通过调试后解决方案如下:
对于问题一,如你在数据库里设置了
- WebService调用错误合集
darkranger
webservice
Java.Lang.NoClassDefFoundError: Org/Apache/Commons/Discovery/Tools/DiscoverSingleton
调用接口出错,
一个简单的WebService
import org.apache.axis.client.Call;import org.apache.axis.client.Service;
首先必不可
- JSP和Servlet的中文乱码处理
aijuans
Java Web
JSP和Servlet的中文乱码处理
前几天学习了JSP和Servlet中有关中文乱码的一些问题,写成了博客,今天进行更新一下。应该是可以解决日常的乱码问题了。现在作以下总结希望对需要的人有所帮助。我也是刚学,所以有不足之处希望谅解。
一、表单提交时出现乱码:
在进行表单提交的时候,经常提交一些中文,自然就避免不了出现中文乱码的情况,对于表单来说有两种提交方式:get和post提交方式。所以
- 面试经典六问
atongyeye
工作面试
题记:因为我不善沟通,所以在面试中经常碰壁,看了网上太多面试宝典,基本上不太靠谱。只好自己总结,并试着根据最近工作情况完成个人答案。以备不时之需。
以下是人事了解应聘者情况的最典型的六个问题:
1 简单自我介绍
关于这个问题,主要为了弄清两件事,一是了解应聘者的背景,二是应聘者将这些背景信息组织成合适语言的能力。
我的回答:(针对技术面试回答,如果是人事面试,可以就掌
- contentResolver.query()参数详解
百合不是茶
androidquery()详解
收藏csdn的博客,介绍的比较详细,新手值得一看 1.获取联系人姓名
一个简单的例子,这个函数获取设备上所有的联系人ID和联系人NAME。
[java]
view plain
copy
public void fetchAllContacts() {
- ora-00054:resource busy and acquire with nowait specified解决方法
bijian1013
oracle数据库killnowait
当某个数据库用户在数据库中插入、更新、删除一个表的数据,或者增加一个表的主键时或者表的索引时,常常会出现ora-00054:resource busy and acquire with nowait specified这样的错误。主要是因为有事务正在执行(或者事务已经被锁),所有导致执行不成功。
1.下面的语句
- web 开发乱码
征客丶
springWeb
以下前端都是 utf-8 字符集编码
一、后台接收
1.1、 get 请求乱码
get 请求中,请求参数在请求头中;
乱码解决方法:
a、通过在web 服务器中配置编码格式:tomcat 中,在 Connector 中添加URIEncoding="UTF-8";
1.2、post 请求乱码
post 请求中,请求参数分两部份,
1.2.1、url?参数,
- 【Spark十六】: Spark SQL第二部分数据源和注册表的几种方式
bit1129
spark
Spark SQL数据源和表的Schema
case class
apply schema
parquet
json
JSON数据源 准备源数据
{"name":"Jack", "age": 12, "addr":{"city":"beijing&
- JVM学习之:调优总结 -Xms -Xmx -Xmn -Xss
BlueSkator
-Xss-Xmn-Xms-Xmx
堆大小设置JVM 中最大堆大小有三方面限制:相关操作系统的数据模型(32-bt还是64-bit)限制;系统的可用虚拟内存限制;系统的可用物理内存限制。32位系统下,一般限制在1.5G~2G;64为操作系统对内存无限制。我在Windows Server 2003 系统,3.5G物理内存,JDK5.0下测试,最大可设置为1478m。典型设置:
java -Xmx355
- jqGrid 各种参数 详解(转帖)
BreakingBad
jqGrid
jqGrid 各种参数 详解 分类:
源代码分享
个人随笔请勿参考
解决开发问题 2012-05-09 20:29 84282人阅读
评论(22)
收藏
举报
jquery
服务器
parameters
function
ajax
string
- 读《研磨设计模式》-代码笔记-代理模式-Proxy
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
import java.lang.reflect.InvocationHandler;
import java.lang.reflect.Method;
import java.lang.reflect.Proxy;
/*
* 下面
- 应用升级iOS8中遇到的一些问题
chenhbc
ios8升级iOS8
1、很奇怪的问题,登录界面,有一个判断,如果不存在某个值,则跳转到设置界面,ios8之前的系统都可以正常跳转,iOS8中代码已经执行到下一个界面了,但界面并没有跳转过去,而且这个值如果设置过的话,也是可以正常跳转过去的,这个问题纠结了两天多,之前的判断我是在
-(void)viewWillAppear:(BOOL)animated
中写的,最终的解决办法是把判断写在
-(void
- 工作流与自组织的关系?
comsci
设计模式工作
目前的工作流系统中的节点及其相互之间的连接是事先根据管理的实际需要而绘制好的,这种固定的模式在实际的运用中会受到很多限制,特别是节点之间的依存关系是固定的,节点的处理不考虑到流程整体的运行情况,细节和整体间的关系是脱节的,那么我们提出一个新的观点,一个流程是否可以通过节点的自组织运动来自动生成呢?这种流程有什么实际意义呢?
这里有篇论文,摘要是:“针对网格中的服务
- Oracle11.2新特性之INSERT提示IGNORE_ROW_ON_DUPKEY_INDEX
daizj
oracle
insert提示IGNORE_ROW_ON_DUPKEY_INDEX
转自:http://space.itpub.net/18922393/viewspace-752123
在 insert into tablea ...select * from tableb中,如果存在唯一约束,会导致整个insert操作失败。使用IGNORE_ROW_ON_DUPKEY_INDEX提示,会忽略唯一
- 二叉树:堆
dieslrae
二叉树
这里说的堆其实是一个完全二叉树,每个节点都不小于自己的子节点,不要跟jvm的堆搞混了.由于是完全二叉树,可以用数组来构建.用数组构建树的规则很简单:
一个节点的父节点下标为: (当前下标 - 1)/2
一个节点的左节点下标为: 当前下标 * 2 + 1
&
- C语言学习八结构体
dcj3sjt126com
c
为什么需要结构体,看代码
# include <stdio.h>
struct Student //定义一个学生类型,里面有age, score, sex, 然后可以定义这个类型的变量
{
int age;
float score;
char sex;
}
int main(void)
{
struct Student st = {80, 66.6,
- centos安装golang
dcj3sjt126com
centos
#在国内镜像下载二进制包
wget -c http://www.golangtc.com/static/go/go1.4.1.linux-amd64.tar.gz
tar -C /usr/local -xzf go1.4.1.linux-amd64.tar.gz
#把golang的bin目录加入全局环境变量
cat >>/etc/profile<
- 10.性能优化-监控-MySQL慢查询
frank1234
性能优化MySQL慢查询
1.记录慢查询配置
show variables where variable_name like 'slow%' ; --查看默认日志路径
查询结果:--不用的机器可能不同
slow_query_log_file=/var/lib/mysql/centos-slow.log
修改mysqld配置文件:/usr /my.cnf[一般在/etc/my.cnf,本机在/user/my.cn
- Java父类取得子类类名
happyqing
javathis父类子类类名
在继承关系中,不管父类还是子类,这些类里面的this都代表了最终new出来的那个类的实例对象,所以在父类中你可以用this获取到子类的信息!
package com.urthinker.module.test;
import org.junit.Test;
abstract class BaseDao<T> {
public void
- Spring3.2新注解@ControllerAdvice
jinnianshilongnian
@Controller
@ControllerAdvice,是spring3.2提供的新注解,从名字上可以看出大体意思是控制器增强。让我们先看看@ControllerAdvice的实现:
@Target(ElementType.TYPE)
@Retention(RetentionPolicy.RUNTIME)
@Documented
@Component
public @interface Co
- Java spring mvc多数据源配置
liuxihope
spring
转自:http://www.itpub.net/thread-1906608-1-1.html
1、首先配置两个数据库
<bean id="dataSourceA" class="org.apache.commons.dbcp.BasicDataSource" destroy-method="close&quo
- 第12章 Ajax(下)
onestopweb
Ajax
index.html
<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://www.w3.org/TR/xhtml1/DTD/xhtml1-transitional.dtd">
<html xmlns="http://www.w3.org/
- BW / Universe Mappings
blueoxygen
BO
BW Element
OLAP Universe Element
Cube Dimension
Class
Charateristic
A class with dimension and detail objects (Detail objects for key and desription)
Hi
- Java开发熟手该当心的11个错误
tomcat_oracle
java多线程工作单元测试
#1、不在属性文件或XML文件中外化配置属性。比如,没有把批处理使用的线程数设置成可在属性文件中配置。你的批处理程序无论在DEV环境中,还是UAT(用户验收
测试)环境中,都可以顺畅无阻地运行,但是一旦部署在PROD 上,把它作为多线程程序处理更大的数据集时,就会抛出IOException,原因可能是JDBC驱动版本不同,也可能是#2中讨论的问题。如果线程数目 可以在属性文件中配置,那么使它成为
- 推行国产操作系统的优劣
yananay
windowslinux国产操作系统
最近刮起了一股风,就是去“国外货”。从应用程序开始,到基础的系统,数据库,现在已经刮到操作系统了。原因就是“棱镜计划”,使我们终于认识到了国外货的危害,开始重视起了信息安全。操作系统是计算机的灵魂。既然是灵魂,为了信息安全,那我们就自然要使用和推行国货。可是,一味地推行,是否就一定正确呢?
先说说信息安全。其实从很早以来大家就在讨论信息安全。很多年以前,就据传某世界级的网络设备制造商生产的交