- 斤斤计较的婚姻到底有多难?
白心之岂必有为
很多人私聊我会问到在哪个人群当中斤斤计较的人最多?我都会回答他,一般婚姻出现问题的斤斤计较的人士会非常多,以我多年经验,在婚姻落的一塌糊涂的人当中,斤斤计较的人数占比在20~30%以上,也就是说10个婚姻出现问题的斤斤计较的人有2-3个有多不减。在婚姻出问题当中,有大量的心理不平衡的、尖酸刻薄的怨妇。在婚姻中仅斤斤计较有两种类型:第一种是物质上的,另一种是精神上的。在物质与精神上抠门已经严重的影响
- 机器学习与深度学习间关系与区别
ℒℴѵℯ心·动ꦿ໊ོ꫞
人工智能学习深度学习python
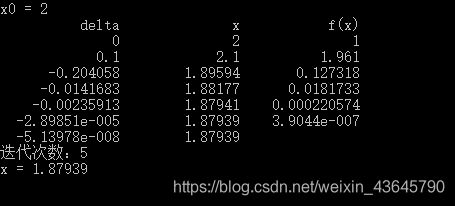
一、机器学习概述定义机器学习(MachineLearning,ML)是一种通过数据驱动的方法,利用统计学和计算算法来训练模型,使计算机能够从数据中学习并自动进行预测或决策。机器学习通过分析大量数据样本,识别其中的模式和规律,从而对新的数据进行判断。其核心在于通过训练过程,让模型不断优化和提升其预测准确性。主要类型1.监督学习(SupervisedLearning)监督学习是指在训练数据集中包含输入
- 【iOS】MVC设计模式
Magnetic_h
iosmvc设计模式objective-c学习ui
MVC前言如何设计一个程序的结构,这是一门专门的学问,叫做"架构模式"(architecturalpattern),属于编程的方法论。MVC模式就是架构模式的一种。它是Apple官方推荐的App开发架构,也是一般开发者最先遇到、最经典的架构。MVC各层controller层Controller/ViewController/VC(控制器)负责协调Model和View,处理大部分逻辑它将数据从Mod
- OC语言多界面传值五大方式
Magnetic_h
iosui学习objective-c开发语言
前言在完成暑假仿写项目时,遇到了许多需要用到多界面传值的地方,这篇博客来总结一下比较常用的五种多界面传值的方式。属性传值属性传值一般用前一个界面向后一个界面传值,简单地说就是通过访问后一个视图控制器的属性来为它赋值,通过这个属性来做到从前一个界面向后一个界面传值。首先在后一个界面中定义属性@interfaceBViewController:UIViewController@propertyNSSt
- 小丽成长记(四十三)
玲玲54321
小丽发现,即使她好不容易调整好自己的心态下一秒总会有不确定的伤脑筋的事出现,一个接一个的问题,人生就没有停下的时候,小问题不断出现。不过她今天看的书,她接受了人生就是不确定的,厉害的人就是不断创造确定性,在Ta的领域比别人多的确定性就能让自己脱颖而出,显示价值从而获得的比别人多的利益。正是这样的原因,因为从前修炼自己太少,使得她现在在人生道路上打怪起来困难重重,她似乎永远摆脱不了那种无力感,有种习
- 《投行人生》读书笔记
小蘑菇的树洞
《投行人生》----作者詹姆斯-A-朗德摩根斯坦利副主席40年的职业洞见-很短小精悍的篇幅,比较适合初入职场的新人。第一部分成功的职业生涯需要规划1.情商归为适应能力分享与协作同理心适应能力,更多的是自我意识,你有能力识别自己的情并分辨这些情绪如何影响你的思想和行为。2.对于初入职场的人的建议,细节,截止日期和数据很重要截止日期,一种有效的方法是请老板为你所有的任务进行优先级排序。和老板喝咖啡的好
- 绘本讲师训练营【24期】8/21阅读原创《独生小孩》
1784e22615e0
24016-孟娟《独生小孩》图片发自App今天我想分享一个蛮特别的绘本,讲的是一个特殊的群体,我也是属于这个群体,80后的独生小孩。这是一本中国绘本,作者郭婧,也是一个80厚。全书一百多页,均为铅笔绘制,虽然为黑白色调,但并不显得沉闷。全书没有文字,犹如“默片”,但并不影响读者对该作品的理解,反而显得神秘,梦幻,給读者留下想象的空间。作者在前蝴蝶页这样写到:“我更希望父母和孩子一起分享这本书,使他
- 向内而求
陈陈_19b4
10月27日,阴。阅读书目:《次第花开》。作者:希阿荣博堪布,是当今藏传佛家宁玛派最伟大的上师法王,如意宝晋美彭措仁波切颇具影响力的弟子之一。多年以来,赴海内外各地弘扬佛法,以正式授课、现场开示、发表文章等多种方法指导佛学弟子修行佛法。代表作《寂静之道》、《生命这出戏》、《透过佛法看世界》自出版以来一直是佛教类书籍中的畅销书。图片发自App金句:1.佛陀说,一切痛苦的根源在于我们长期以来对自身及外
- html 中如何使用 uniapp 的部分方法
某公司摸鱼前端
htmluni-app前端
示例代码:Documentconsole.log(window);效果展示:好了,现在就可以uni.使用相关的方法了
- 抖音乐买买怎么加入赚钱?赚钱方法是什么
测评君高省
你会在抖音买东西吗?如果会,那么一定要免费注册一个乐买买,抖音直播间,橱窗,小视频里的小黄车买东西都可以返佣金!省下来都是自己的,分享还可以赚钱乐买买是好省旗下的抖音返佣平台,乐买买分析社交电商的价值,乐买买属于今年难得的副业项目风口机会,2019年错过做好省的搞钱的黄金时期,那么2022年千万别再错过乐买买至于我为何转到高省呢?当然是高省APP佣金更高,模式更好,终端用户不流失。【高省】是一个自
- 水平垂直居中的几种方法(总结)
LJ小番茄
CSS_玄学语言htmljavascript前端csscss3
1.使用flexbox的justify-content和align-items.parent{display:flex;justify-content:center;/*水平居中*/align-items:center;/*垂直居中*/height:100vh;/*需要指定高度*/}2.使用grid的place-items:center.parent{display:grid;place-item
- 我的烦恼
余建梅
我的烦恼。女儿问我:“你给学生布置什么作文题目?”“《我的烦恼》。”“他们都这么大了,你觉得他们还有烦恼吗?”“有啊!每个人都会有自己烦恼。”“我不相信,大人是没有烦恼的,如果说一定有的话,你的烦恼和我写作业有关,而且是小烦恼。不像我,天天被你说,有这样的妈妈,烦恼是没完没了。”女儿愤愤不平。每个人都会有自己的烦恼,处在上有老下有小的年纪,烦恼多的数不完。想干好工作带好孩子,想孝顺父母又想经营好自
- Python中os.environ基本介绍及使用方法
鹤冲天Pro
#Pythonpython服务器开发语言
文章目录python中os.environos.environ简介os.environ进行环境变量的增删改查python中os.environ的使用详解1.简介2.key字段详解2.1常见key字段3.os.environ.get()用法4.环境变量的增删改查和判断是否存在4.1新增环境变量4.2更新环境变量4.3获取环境变量4.4删除环境变量4.5判断环境变量是否存在python中os.envi
- PHP环境搭建详细教程
好看资源平台
前端php
PHP是一个流行的服务器端脚本语言,广泛用于Web开发。为了使PHP能够在本地或服务器上运行,我们需要搭建一个合适的PHP环境。本教程将结合最新资料,介绍在不同操作系统上搭建PHP开发环境的多种方法,包括Windows、macOS和Linux系统的安装步骤,以及本地和Docker环境的配置。1.PHP环境搭建概述PHP环境的搭建主要分为以下几类:集成开发环境:例如XAMPP、WAMP、MAMP,这
- 下载github patch到本地
小米人er
我的博客gitpatch
以下是几种从GitHub上下载以.patch结尾的补丁文件的方法:通过浏览器直接下载打开包含该.patch文件的GitHub仓库。在仓库的文件列表中找到对应的.patch文件。点击该文件,浏览器会显示文件的内容,在页面的右上角通常会有一个“Raw”按钮,点击它可以获取原始文件内容。然后在浏览器中使用快捷键(如Ctrl+S或者Command+S)将原始文件保存到本地,选择保存的文件名并确保后缀为.p
- 基于社交网络算法优化的二维最大熵图像分割
智能算法研学社(Jack旭)
智能优化算法应用图像分割算法php开发语言
智能优化算法应用:基于社交网络优化的二维最大熵图像阈值分割-附代码文章目录智能优化算法应用:基于社交网络优化的二维最大熵图像阈值分割-附代码1.前言2.二维最大熵阈值分割原理3.基于社交网络优化的多阈值分割4.算法结果:5.参考文献:6.Matlab代码摘要:本文介绍基于最大熵的图像分割,并且应用社交网络算法进行阈值寻优。1.前言阅读此文章前,请阅读《图像分割:直方图区域划分及信息统计介绍》htt
- 关于提高复杂业务逻辑代码可读性的思考
编程经验分享
开发经验java数据库开发语言
目录前言需求场景常规写法拆分方法领域对象总结前言实际工作中大部分时间都是在写业务逻辑,一般都是三层架构,表示层(Controller)接收客户端请求,并对入参做检验,业务逻辑层(Service)负责处理业务逻辑,一般开发都是在这一层中写具体的业务逻辑。数据访问层(Dao)是直接和数据库交互的,用于查数据给业务逻辑层,或者是将业务逻辑层处理后的数据写入数据库。简单的增删改查接口不用多说,基本上写好一
- 山东大学小树林支教调研团青青仓木队——翟晓楠
山东大学青青仓木队
过了半年,又一次启程,又一次回到支教的初心之地。比起上一次的试探与不安,我更多了一丝稳重与熟练。心境、处境也都随着半个学期的过去而变得不同,半个学期中,身体上的,心理上的,太多的逆境让我变得步履维艰,曲曲折折,弯弯绕绕,我仿佛打不起精神,没有胃口,没有动力。感觉走的不顺畅的时候,支教这个旅程,给了我力量。自告奋勇承担起队长这一职务的我,从组织时的复杂和困难的经历,协调各种问题,从无到有,和校长和队
- 拥有断舍离的心态,过精简生活--《断舍离》读书笔记
爱吃丸子的小樱桃
不知不觉间房间里的东西越来越多,虽然摆放整齐,但也时常会觉得空间逼仄,令人心生烦闷。抱着断舍离的态度,我开始阅读《断舍离》这本书,希望从书中能找到一些有效的方法,帮助我实现空间、物品上的断舍离。《断舍离》是日本作家山下英子通过自己的经历、思考和实践总结而成的,整体内涵也从刚开始的私人生活哲学的“断舍离”升华成了“人生实践哲学”,接着又成为每个人都能实行的“改变人生的断舍离”,从“哲学”逐渐升华成“
- 如果做到轻松在股市赚钱?只要坚持这三个原则。
履霜之人
大A股里向来就有七亏二平一赚的说法,能赚钱的都是少数人。否则股市就成了慈善机构,人人都有钱赚,谁还要上班?所以说亏钱是正常的,或者说是应该的。那么那些赚钱的人又是如何做到的呢?普通人能不能找到捷径去分一杯羹呢?方法是有的,但要做到需要你有极高的自律。第一,控制仓位,散户最大的问题是追涨杀跌,只要涨起来,就把钱往股票上砸,然后被套,隔天跌的受不了,又一刀切,全部割肉。来来回回间,遍体鳞伤。所以散户首
- 高端密码学院笔记285
柚子_b4b4
高端幸福密码学院(高级班)幸福使者:李华第(598)期《幸福》之回归内在深层生命原动力基础篇——揭秘“激励”成长的喜悦心理案例分析主讲:刘莉一,知识扩充:成功=艰苦劳动+正确方法+少说空话。贪图省力的船夫,目标永远下游。智者的梦再美,也不如愚人实干的脚印。幸福早课堂2020.10.16星期五一笔记:1,重视和珍惜的前提是知道它的价值非常重要,当你珍惜了,你就真正定下来,真正的学到身上。2,大家需要
- 从0到500+,我是如何利用自媒体赚钱?
一列脚印
运营公众号半个多月,从零基础的小白到现在慢慢懂了一些运营的知识。做好公众号是很不容易的,要做很多事情;排版、码字、引流…通通需要自己解决,业余时间全都花费在这上面涨这么多粉丝是真的不容易,对比知乎大佬来说,我们这种没资源,没人脉,还没钱的小透明来说,想要一个月涨粉上万,怕是今天没睡醒(不过你有的方法,算我piapia打脸)至少我是清醒的,自己慢慢努力,实现我的万粉目标!大家快来围观、支持我吧!孩子
- 使用Apify加载Twitter消息以进行微调的完整指南
nseejrukjhad
twittereasyui前端python
#使用Apify加载Twitter消息以进行微调的完整指南##引言在自然语言处理领域,微调模型以适应特定任务是提升模型性能的常见方法。本文将介绍如何使用Apify从Twitter导出聊天信息,以便进一步进行微调。##主要内容###使用Apify导出推文首先,我们需要从Twitter导出推文。Apify可以帮助我们做到这一点。通过Apify的强大功能,我们可以批量抓取和导出数据,适用于各类应用场景。
- 深入理解 MultiQueryRetriever:提升向量数据库检索效果的强大工具
nseejrukjhad
数据库python
深入理解MultiQueryRetriever:提升向量数据库检索效果的强大工具引言在人工智能和自然语言处理领域,高效准确的信息检索一直是一个关键挑战。传统的基于距离的向量数据库检索方法虽然广泛应用,但仍存在一些局限性。本文将介绍一种创新的解决方案:MultiQueryRetriever,它通过自动生成多个查询视角来增强检索效果,提高结果的相关性和多样性。MultiQueryRetriever的工
- 数组去重
好奇的猫猫猫
整理自js中基础数据结构数组去重问题思考?如何去除数组中重复的项例如数组:[1,3,4,3,5]我们在做去重的时候,一开始想到的肯定是,逐个比较,外面一层循环,内层后一个与前一个一比较,如果是久不将当前这一项放进新的数组,挨个比较完之后返回一个新的去过重复的数组不好的实践方式上述方法效率极低,代码量还多,思考?有没有更好的方法这时候不禁一想当然有了!!!hashtable啊,通过对象的hash办法
- 有舍才有得
_清净_
为什么经常讲放下?放下就是让你要舍得、舍去。喜舍心就是把自己喜欢的,用慈悲心喜舍出去。这就锻炼了你们在人间,学会放下原本不舍得的东西或一些事物,学会舍出去,学会帮助别人,学会多付出。你今天付出了慈悲心、喜舍心,以后会得到更多的缘助力。缘助力是什么?——贵人缘啊。今天没有付出,不懂得付出,什么都只会想到自己,那你也得不到缘助力。慈悲喜舍就是用慈悲心去帮助别人,用喜舍心去付出,最后也会得到别人回报。别
- 【JS】执行时长(100分) |思路参考+代码解析(C++)
l939035548
JS算法数据结构c++
题目为了充分发挥GPU算力,需要尽可能多的将任务交给GPU执行,现在有一个任务数组,数组元素表示在这1秒内新增的任务个数且每秒都有新增任务。假设GPU最多一次执行n个任务,一次执行耗时1秒,在保证GPU不空闲情况下,最少需要多长时间执行完成。题目输入第一个参数为GPU一次最多执行的任务个数,取值范围[1,10000]第二个参数为任务数组长度,取值范围[1,10000]第三个参数为任务数组,数字范围
- Python快速入门 —— 第三节:类与对象
孤华暗香
Python快速入门python开发语言
第三节:类与对象目标:了解面向对象编程的基础概念,并学会如何定义类和创建对象。内容:类与对象:定义类:class关键字。类的构造函数:__init__()。类的属性和方法。对象的创建与使用。示例:classStudent:def__init__(self,name,age,major):self.name&#
- 走向以教育叙事为载体的教育叙事研究
666小飞鱼
今天我读了吴松超老师的《给教师的68条建写作建议》中的第23条《如何通过教育叙事走向研究》,吴老师在文中与我们分享了一个德育案例,这是一个反面的案例,意在告知我们在处理问题时,不能就考虑的点太窄,思考要全面。走向教育叙事研究,教师要有敏锐的“感知力”,这个感知力来自于背后专业知识的支撑,思维能力以及广阔的视野和见识等。所以对于同一件事处理方法不同,这个就是教师背后“敏锐力”的不同造成的,也就是说是
- 2022-11-17
无奇君
又去了一次社康,这次是急性支气管炎……太难了。半夜就猛咳,天天咳醒,还好他戴海绵耳塞睡吵不到他,要不然对他来说也是种煎熬。一累也会猛咳,希望这次是最后一次吃药,吃完就好。又想把头发剪短了,顺便染个色。可是刚刚去看人家还没开门,不是休息日老板好佛系。理发店是个夫妻店,一年多前刚搬来的时候老板还没对象呢,当时聊天老板就说希望能找个对象一起两个人守着店都比上班强。不久后再去他已经有对象了,而且在店里帮忙
- [星球大战]阿纳金的背叛
comsci
本来杰迪圣殿的长老是不同意让阿纳金接受训练的.........
但是由于政治原因,长老会妥协了...这给邪恶的力量带来了机会
所以......现代的地球联邦接受了这个教训...绝对不让某些年轻人进入学院
- 看懂它,你就可以任性的玩耍了!
aijuans
JavaScript
javascript作为前端开发的标配技能,如果不掌握好它的三大特点:1.原型 2.作用域 3. 闭包 ,又怎么可以说你学好了这门语言呢?如果标配的技能都没有撑握好,怎么可以任性的玩耍呢?怎么验证自己学好了以上三个基本点呢,我找到一段不错的代码,稍加改动,如果能够读懂它,那么你就可以任性了。
function jClass(b
- Java常用工具包 Jodd
Kai_Ge
javajodd
Jodd 是一个开源的 Java 工具集, 包含一些实用的工具类和小型框架。简单,却很强大! 写道 Jodd = Tools + IoC + MVC + DB + AOP + TX + JSON + HTML < 1.5 Mb
Jodd 被分成众多模块,按需选择,其中
工具类模块有:
jodd-core &nb
- SpringMvc下载
120153216
springMVC
@RequestMapping(value = WebUrlConstant.DOWNLOAD)
public void download(HttpServletRequest request,HttpServletResponse response,String fileName) {
OutputStream os = null;
InputStream is = null;
- Python 标准异常总结
2002wmj
python
Python标准异常总结
AssertionError 断言语句(assert)失败 AttributeError 尝试访问未知的对象属性 EOFError 用户输入文件末尾标志EOF(Ctrl+d) FloatingPointError 浮点计算错误 GeneratorExit generator.close()方法被调用的时候 ImportError 导入模块失
- SQL函数返回临时表结构的数据用于查询
357029540
SQL Server
这两天在做一个查询的SQL,这个SQL的一个条件是通过游标实现另外两张表查询出一个多条数据,这些数据都是INT类型,然后用IN条件进行查询,并且查询这两张表需要通过外部传入参数才能查询出所需数据,于是想到了用SQL函数返回值,并且也这样做了,由于是返回多条数据,所以把查询出来的INT类型值都拼接为了字符串,这时就遇到问题了,在查询SQL中因为条件是INT值,SQL函数的CAST和CONVERST都
- java 时间格式化 | 比较大小| 时区 个人笔记
7454103
javaeclipsetomcatcMyEclipse
个人总结! 不当之处多多包含!
引用 1.0 如何设置 tomcat 的时区:
位置:(catalina.bat---JAVA_OPTS 下面加上)
set JAVA_OPT
- 时间获取Clander的用法
adminjun
Clander时间
/**
* 得到几天前的时间
* @param d
* @param day
* @return
*/
public static Date getDateBefore(Date d,int day){
Calend
- JVM初探与设置
aijuans
java
JVM是Java Virtual Machine(Java虚拟机)的缩写,JVM是一种用于计算设备的规范,它是一个虚构出来的计算机,是通过在实际的计算机上仿真模拟各种计算机功能来实现的。Java虚拟机包括一套字节码指令集、一组寄存器、一个栈、一个垃圾回收堆和一个存储方法域。 JVM屏蔽了与具体操作系统平台相关的信息,使Java程序只需生成在Java虚拟机上运行的目标代码(字节码),就可以在多种平台
- SQL中ON和WHERE的区别
avords
SQL中ON和WHERE的区别
数据库在通过连接两张或多张表来返回记录时,都会生成一张中间的临时表,然后再将这张临时表返回给用户。 www.2cto.com 在使用left jion时,on和where条件的区别如下: 1、 on条件是在生成临时表时使用的条件,它不管on中的条件是否为真,都会返回左边表中的记录。
- 说说自信
houxinyou
工作生活
自信的来源分为两种,一种是源于实力,一种源于头脑.实力是一个综合的评定,有自身的能力,能利用的资源等.比如我想去月亮上,要身体素质过硬,还要有飞船等等一系列的东西.这些都属于实力的一部分.而头脑不同,只要你头脑够简单就可以了!同样要上月亮上,你想,我一跳,1米,我多跳几下,跳个几年,应该就到了!什么?你说我会往下掉?你笨呀你!找个东西踩一下不就行了吗?
无论工作还
- WEBLOGIC事务超时设置
bijian1013
weblogicjta事务超时
系统中统计数据,由于调用统计过程,执行时间超过了weblogic设置的时间,提示如下错误:
统计数据出错!
原因:The transaction is no longer active - status: 'Rolling Back. [Reason=weblogic.transaction.internal
- 两年已过去,再看该如何快速融入新团队
bingyingao
java互联网融入架构新团队
偶得的空闲,翻到了两年前的帖子
该如何快速融入一个新团队,有所感触,就记下来,为下一个两年后的今天做参考。
时隔两年半之后的今天,再来看当初的这个博客,别有一番滋味。而我已经于今年三月份离开了当初所在的团队,加入另外的一个项目组,2011年的这篇博客之后的时光,我很好的融入了那个团队,而直到现在和同事们关系都特别好。大家在短短一年半的时间离一起经历了一
- 【Spark七十七】Spark分析Nginx和Apache的access.log
bit1129
apache
Spark分析Nginx和Apache的access.log,第一个问题是要对Nginx和Apache的access.log文件进行按行解析,按行解析就的方法是正则表达式:
Nginx的access.log解析正则表达式
val PATTERN = """([^ ]*) ([^ ]*) ([^ ]*) (\\[.*\\]) (\&q
- Erlang patch
bookjovi
erlang
Totally five patchs committed to erlang otp, just small patchs.
IMO, erlang really is a interesting programming language, I really like its concurrency feature.
but the functional programming style
- log4j日志路径中加入日期
bro_feng
javalog4j
要用log4j使用记录日志,日志路径有每日的日期,文件大小5M新增文件。
实现方式
log4j:
<appender name="serviceLog"
class="org.apache.log4j.RollingFileAppender">
<param name="Encoding" v
- 读《研磨设计模式》-代码笔记-桥接模式
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
/**
* 个人觉得关于桥接模式的例子,蜡笔和毛笔这个例子是最贴切的:http://www.cnblogs.com/zhenyulu/articles/67016.html
* 笔和颜色是可分离的,蜡笔把两者耦合在一起了:一支蜡笔只有一种
- windows7下SVN和Eclipse插件安装
chenyu19891124
eclipse插件
今天花了一天时间弄SVN和Eclipse插件的安装,今天弄好了。svn插件和Eclipse整合有两种方式,一种是直接下载插件包,二种是通过Eclipse在线更新。由于之前Eclipse版本和svn插件版本有差别,始终是没装上。最后在网上找到了适合的版本。所用的环境系统:windows7JDK:1.7svn插件包版本:1.8.16Eclipse:3.7.2工具下载地址:Eclipse下在地址:htt
- [转帖]工作流引擎设计思路
comsci
设计模式工作应用服务器workflow企业应用
作为国内的同行,我非常希望在流程设计方面和大家交流,刚发现篇好文(那么好的文章,现在才发现,可惜),关于流程设计的一些原理,个人觉得本文站得高,看得远,比俺的文章有深度,转载如下
=================================================================================
自开博以来不断有朋友来探讨工作流引擎该如何
- Linux 查看内存,CPU及硬盘大小的方法
daizj
linuxcpu内存硬盘大小
一、查看CPU信息的命令
[root@R4 ~]# cat /proc/cpuinfo |grep "model name" && cat /proc/cpuinfo |grep "physical id"
model name : Intel(R) Xeon(R) CPU X5450 @ 3.00GHz
model name :
- linux 踢出在线用户
dongwei_6688
linux
两个步骤:
1.用w命令找到要踢出的用户,比如下面:
[root@localhost ~]# w
18:16:55 up 39 days, 8:27, 3 users, load average: 0.03, 0.03, 0.00
USER TTY FROM LOGIN@ IDLE JCPU PCPU WHAT
- 放手吧,就像不曾拥有过一样
dcj3sjt126com
内容提要:
静悠悠编著的《放手吧就像不曾拥有过一样》集结“全球华语世界最舒缓心灵”的精华故事,触碰生命最深层次的感动,献给全世界亿万读者。《放手吧就像不曾拥有过一样》的作者衷心地祝愿每一位读者都给自己一个重新出发的理由,将那些令你痛苦的、扛起的、背负的,一并都放下吧!把憔悴的面容换做一种清淡的微笑,把沉重的步伐调节成春天五线谱上的音符,让自己踏着轻快的节奏,在人生的海面上悠然漂荡,享受宁静与
- php二进制安全的含义
dcj3sjt126com
PHP
PHP里,有string的概念。
string里,每个字符的大小为byte(与PHP相比,Java的每个字符为Character,是UTF8字符,C语言的每个字符可以在编译时选择)。
byte里,有ASCII代码的字符,例如ABC,123,abc,也有一些特殊字符,例如回车,退格之类的。
特殊字符很多是不能显示的。或者说,他们的显示方式没有标准,例如编码65到哪儿都是字母A,编码97到哪儿都是字符
- Linux下禁用T440s,X240的一体化触摸板(touchpad)
gashero
linuxThinkPad触摸板
自打1月买了Thinkpad T440s就一直很火大,其中最让人恼火的莫过于触摸板。
Thinkpad的经典就包括用了小红点(TrackPoint)。但是小红点只能定位,还是需要鼠标的左右键的。但是自打T440s等开始启用了一体化触摸板,不再有实体的按键了。问题是要是好用也行。
实际使用中,触摸板一堆问题,比如定位有抖动,以及按键时会有飘逸。这就导致了单击经常就
- graph_dfs
hcx2013
Graph
package edu.xidian.graph;
class MyStack {
private final int SIZE = 20;
private int[] st;
private int top;
public MyStack() {
st = new int[SIZE];
top = -1;
}
public void push(i
- Spring4.1新特性——Spring核心部分及其他
jinnianshilongnian
spring 4.1
目录
Spring4.1新特性——综述
Spring4.1新特性——Spring核心部分及其他
Spring4.1新特性——Spring缓存框架增强
Spring4.1新特性——异步调用和事件机制的异常处理
Spring4.1新特性——数据库集成测试脚本初始化
Spring4.1新特性——Spring MVC增强
Spring4.1新特性——页面自动化测试框架Spring MVC T
- 配置HiveServer2的安全策略之自定义用户名密码验证
liyonghui160com
具体从网上看
http://doc.mapr.com/display/MapR/Using+HiveServer2#UsingHiveServer2-ConfiguringCustomAuthentication
LDAP Authentication using OpenLDAP
Setting
- 一位30多的程序员生涯经验总结
pda158
编程工作生活咨询
1.客户在接触到产品之后,才会真正明白自己的需求。
这是我在我的第一份工作上面学来的。只有当我们给客户展示产品的时候,他们才会意识到哪些是必须的。给出一个功能性原型设计远远比一张长长的文字表格要好。 2.只要有充足的时间,所有安全防御系统都将失败。
安全防御现如今是全世界都在关注的大课题、大挑战。我们必须时时刻刻积极完善它,因为黑客只要有一次成功,就可以彻底打败你。 3.
- 分布式web服务架构的演变
自由的奴隶
linuxWeb应用服务器互联网
最开始,由于某些想法,于是在互联网上搭建了一个网站,这个时候甚至有可能主机都是租借的,但由于这篇文章我们只关注架构的演变历程,因此就假设这个时候已经是托管了一台主机,并且有一定的带宽了,这个时候由于网站具备了一定的特色,吸引了部分人访问,逐渐你发现系统的压力越来越高,响应速度越来越慢,而这个时候比较明显的是数据库和应用互相影响,应用出问题了,数据库也很容易出现问题,而数据库出问题的时候,应用也容易
- 初探Druid连接池之二——慢SQL日志记录
xingsan_zhang
日志连接池druid慢SQL
由于工作原因,这里先不说连接数据库部分的配置,后面会补上,直接进入慢SQL日志记录。
1.applicationContext.xml中增加如下配置:
<bean abstract="true" id="mysql_database" class="com.alibaba.druid.pool.DruidDataSourc