- 机器学习与深度学习间关系与区别
ℒℴѵℯ心·动ꦿ໊ོ꫞
人工智能学习深度学习python
一、机器学习概述定义机器学习(MachineLearning,ML)是一种通过数据驱动的方法,利用统计学和计算算法来训练模型,使计算机能够从数据中学习并自动进行预测或决策。机器学习通过分析大量数据样本,识别其中的模式和规律,从而对新的数据进行判断。其核心在于通过训练过程,让模型不断优化和提升其预测准确性。主要类型1.监督学习(SupervisedLearning)监督学习是指在训练数据集中包含输入
- 绘本讲师训练营【24期】8/21阅读原创《独生小孩》
1784e22615e0
24016-孟娟《独生小孩》图片发自App今天我想分享一个蛮特别的绘本,讲的是一个特殊的群体,我也是属于这个群体,80后的独生小孩。这是一本中国绘本,作者郭婧,也是一个80厚。全书一百多页,均为铅笔绘制,虽然为黑白色调,但并不显得沉闷。全书没有文字,犹如“默片”,但并不影响读者对该作品的理解,反而显得神秘,梦幻,給读者留下想象的空间。作者在前蝴蝶页这样写到:“我更希望父母和孩子一起分享这本书,使他
- LLM 词汇表
落难Coder
LLMsNLP大语言模型大模型llama人工智能
Contextwindow“上下文窗口”是指语言模型在生成新文本时能够回溯和参考的文本量。这不同于语言模型训练时所使用的大量数据集,而是代表了模型的“工作记忆”。较大的上下文窗口可以让模型理解和响应更复杂和更长的提示,而较小的上下文窗口可能会限制模型处理较长提示或在长时间对话中保持连贯性的能力。Fine-tuning微调是使用额外的数据进一步训练预训练语言模型的过程。这使得模型开始表示和模仿微调数
- 将cmd中命令输出保存为txt文本文件
落难Coder
Windowscmdwindow
最近深度学习本地的训练中我们常常要在命令行中运行自己的代码,无可厚非,我们有必要保存我们的炼丹结果,但是复制命令行输出到txt是非常麻烦的,其实Windows下的命令行为我们提供了相应的操作。其基本的调用格式就是:运行指令>输出到的文件名称或者具体保存路径测试下,我打开cmd并且ping一下百度:pingwww.baidu.com>./data.txt看下相同目录下data.txt的输出:如果你再
- 回溯算法-重新安排行程
chirou_
算法数据结构图论c++图搜索
leetcode332.重新安排行程这题我还没自己ac过,只能现在凭着刚学完的热乎劲把我对题解的理解记下来。本题我认为对数据结构的考察比较多,用什么数据结构去存数据,去读取数据,都是很重要的。classSolution{private:unordered_map>targets;boolbacktracking(intticketNum,vector&result){//1.确定参数和返回值//2
- 展现思维导图魅力,不断挖掘人生宝藏
思维导图讲师Mandy
第13期最强思维导图训练营已经结束一周了,但是我依旧是感觉所有学员还在努力的学习,这些学员中有教师、学生、白领、公务员、宝妈等等,只要你努力,只要你想改变自己,任何行业,任何岗位都可以参与进来,28天足以让你见成效,在这28天中,我们的学员不仅仅是收获了一枚毕业证,最重要的是让自己的思维方式得到升级,今天的你为自己投资,明天的你就会感谢你今天的付出,我们来听一听来自13期最强思维导图训练营优秀学员
- C++ | Leetcode C++题解之第409题最长回文串
Ddddddd_158
经验分享C++Leetcode题解
题目:题解:classSolution{public:intlongestPalindrome(strings){unordered_mapcount;intans=0;for(charc:s)++count[c];for(autop:count){intv=p.second;ans+=v/2*2;if(v%2==1andans%2==0)++ans;}returnans;}};
- 渝婧感恩日记第68天
梁渝婧lydia
1.哇!我真是太幸福啦!感恩奇迹感恩训练营毕业典礼,让我能共振到同学们的喜悦和能量,感谢!感谢!感谢!2.哇!我真是太幸福啦!感恩每天早起,运动3公里!这个星期又做到连续三天,不间断!感谢亲爱的渝婧!你真的是非常的棒!加油,继续坚持!感谢!感谢!感谢!3.哇!我真是太幸福啦!感恩曾正波班主任给我们分享的艾宾浩斯的记忆曲线255学习法,让我蠢蠢欲试,感谢!感谢!感谢!4.哇!我真是太幸福啦!感恩胜利
- BART&BERT
Ambition_LAO
深度学习
BART和BERT都是基于Transformer架构的预训练语言模型。模型架构:BERT(BidirectionalEncoderRepresentationsfromTransformers)主要是一个编码器(Encoder)模型,它使用了Transformer的编码器部分来处理输入的文本,并生成文本的表示。BERT特别擅长理解语言的上下文,因为它在预训练阶段使用了掩码语言模型(MLM)任务,即
- 放松的一天
4da9b7687fa0
20190325总结起床07:20图片发自App睡觉:23:00天气:晴今日任务清单学习·信息·阅读•水滴阅读Day40Alice’sAdventuresinWonderlandChapter6.2图片发自App•BBC跟读训练营Day24图片发自App图片发自App图片发自App•潘多拉口语训练营Day6Wow.Whatabigboy!•文化知识学习今日无•阅读时间地狱健康·饮食·锻炼•饮食目标
- 人到中年的5大恐惧
不想独白的独白
这一段时间闭关在家,心里越来越没有底。全球疫情,全国疫情,一直在关心和自我调试中。但是,好像还是对自己的未来充满了无所适从。不想去做什么,也没有激情和兴趣去开始什么。人生过半,还有什么可以逆袭或改变的机会呢。不知道做什么的时候,去追剧,做美食,教育孩子,锻炼,花钱进什么什么读书训练营,打卡训练营,微信群,各种分享和共同体的群。但是还是没有任何的起色。就这样了吗。中午并不困,但是到了12点,还是习惯
- 我的一个小心愿,减肥20斤,有人一起吗
张晓晓ZXX
我现在体重141斤,163cm,想减到120以内,不想吃减肥药,不喝奶昔,也不想买健身卡,就是希望通过一些运动的aPP进行训练和适当的节食,有人一起的吗?3月12号,我73公斤,现在70.9公斤,是通过咕咚app训练来的,但一个人太孤单,有一起的吗?我想知道除了小时候坚持一个月练习写字帖把字写好了,还能做什么锻炼一下自己的毅力,我也想知道100天之后,我能不能也达到理想的体重。接下来100天,愿意
- 今天是总结
薛帅
今天来个最后一天的总结。为什么要学习写作技巧呢?就如同建房子,如果想要住的安全、舒服,我们要先打地基,建房子的框架,这样才能随意的装修。那么我们要怎么建好才能建好写作的地基呢?1走直路,少弯路01利他:能够给别人带来价值。02吸引:吸住读者的眼球。03打动:打动人心,引起共鸣。04说服:用数据说话。05刻意:通过有意识的训练。06修改:好的文章至上修改10遍。07模仿:10万+的文章必有成功的道理
- 字节二面
Redstone Monstrosity
前端面试
1.假设你是正在面试前端开发工程师的候选人,面试官让你详细说出你上一段实习过程的收获和感悟。在上一段实习过程中,我获得了宝贵的实践经验和深刻的行业洞察,以下是我的主要收获和感悟:一、专业技能提升框架应用熟练度:通过实际项目,我深入掌握了React、Vue等前端框架的使用,不仅提升了编码效率,还学会了如何根据项目需求选择合适的框架。问题解决能力:在实习期间,我遇到了许多预料之外的技术难题。通过查阅文
- 当你看不惯的东西越来越多时,证明你老了!
书影斑斓的简书
看国产电视剧,看到那些小鲜肉扮嫩耍酷面瘫的演技时,我总有一种冲进屏幕痛打对方一顿的冲动。什么玩意儿?!但是,多年训练出来的对方视角看问题的能力,又让我可以理智下来,思考其中的合理性。一部影视剧的投资人、导演不是看不出这些小鲜肉的白痴演技,但之所以依然启用这些油头粉面的小鲜肉,就是因为他们能带来无数年轻粉丝的追捧,进而带来收视率和收益。资本天然逐利,影视剧本来就是资本运作的产物罢了。你看不惯这些小鲜
- 3.1 损失函数和优化:损失函数
做只小考拉
用一个函数把W当做输入,然后看一下得分,定量地估计W的好坏,这个函数被称为“损失函数”。损失函数用于度量W的好坏。有了损失函数的概念后,就可以定量的衡量W到底是好还是坏,要找到一种有效的方法来从W的可行域里,找到W取何值时情况最不坏,,这个过程将会是一个优化过程。损失函数L_i定义:通过函数f给出预测的分数和真实的目标(或者说是标签y),可以定量的描述训练样本预测的好不好,最终的损失函数是在整个数
- Vicky的ScalersTalk第六轮新概念朗读持续力训练Day73 20210411
Vicky_b9de
练习材料:ModerncavemenPart-3ˈmɒdənˈkeɪvmənpɑːt-3Theyplungedintothelake,andafterloadingtheirgearonaninflatablerubberdinghy,letthecurrentcarrythemtotheotherside.Toprotectthemselvesfromtheicywater,theyhadtow
- 使用selenium调用firefox提示Profile Missing的问题解决
歪歪的酒壶
selenium测试工具python
在Ubuntu22.04环境中,使用python3运行selenium提示ProfileMissing,具体信息为:YourFirefoxprofilecannotbeloaded.Itmaybemissingorinaccessible在这个问题的环境中firefox浏览器工作正常。排查中,手动在命令行执行firefox可以打开浏览器,但是出现如下提示Gtk-Message:15:32:09.9
- 股票公众号怎么赚钱的?炒股公众号靠什么赚钱?
氧惠评测
股票公众号赚钱的方式主要有以下几种:别指望别人对你好,你没有价值,别人怎么可能会对你好,要知道:人生都是相互的,你要让自己有价值,只要你有价值了,水到自然渠成了。氧惠APP是与以往完全不同的抖客+淘客app!2024全新模式,我的直推也会放到你下面。主打:带货高补贴,深受各位带货团队长喜爱(训练营导师每天出单带货几万单)。注册即可享受高补贴+0撸+捡漏等带货新体验。送万元推广大礼包,教你如何1年做
- 【从问题中去学习k8s】k8s中的常见面试题(夯实理论基础)(二十八)
向往风的男子
k8s学习kubernetes容器
本站以分享各种运维经验和运维所需要的技能为主《python零基础入门》:python零基础入门学习《python运维脚本》:python运维脚本实践《shell》:shell学习《terraform》持续更新中:terraform_Aws学习零基础入门到最佳实战《k8》从问题中去学习k8s《docker学习》暂未更新《ceph学习》ceph日常问题解决分享《日志收集》ELK+各种中间件《运维日常》
- 必知|儿童能力训练中的能力到底是什么?
ll冰儿
今天我们来一起聊一下“能力”,我们做儿童能力训练,我们跟家长解释能力训练多么多么重要,很多家长反应很茫然,表示不太容易理解,训练能力难倒比学习知识更重要?这说明我们没有解释清楚什么是“能力”,它为什么会如此的重要,今天我们就来跟大白老师一起梳理一下能力的重要性,以及能力和知识的关系。我们采用类比的方式来形象的解释一下什么是能力:先来说说茅草屋,茅草屋一般很矮,没有谁会建造好几层的茅草屋吧?!我们再
- 吴恩达深度学习笔记(30)-正则化的解释
极客Array
正则化(Regularization)深度学习可能存在过拟合问题——高方差,有两个解决方法,一个是正则化,另一个是准备更多的数据,这是非常可靠的方法,但你可能无法时时刻刻准备足够多的训练数据或者获取更多数据的成本很高,但正则化通常有助于避免过拟合或减少你的网络误差。如果你怀疑神经网络过度拟合了数据,即存在高方差问题,那么最先想到的方法可能是正则化,另一个解决高方差的方法就是准备更多数据,这也是非常
- 南山演讲口才|教你如何克服演讲中的不良心理
韦先
演讲者在演讲中必须解除思想负担和心理压力,及时调节自己的心境和情绪,树立起必胜的自信心。1.缺乏信心的心理演讲者看到自己的某些弱点,如普通话说得不太标准、言语技巧训练不足等,常有这样的疑问:“我能行吗?”这个疑问本身会促使演讲者夸大自己的弱点,从而对演讲丧失信心。其实,缺点人人都有,在千百双眼睛注视你时,需要的是扬长避短,掩盖缺点几乎不可能。因此,演讲时应告诉自己:“我刻苦练习了,只要发挥出应有水
- Codeforces Round 972 (Div. 2) A-C 题解
AKDreamer_HeXY
Codeforces比赛题解c++算法动态规划数据结构贪心算法
本来以为B2难度会1900什么的,结果感觉1200还没有,先做的B1,后悔了QwQ关于我现场没切出C这件事……现场排名:A.SimplePalindrome题意构造一个长度为nnn的字符串,只包含aeiou五种字母,需要使得构造出来的字符串所包含的回文子序列数量最小思路当n≤5n\le5n≤5时,只要555个字母不重复出现都是最优情况当n>5n>5n>5时,可以证明:把相同字母放在一起是最优情况:
- 你会读书吗
阿杰说澄长
一上学那会,朋友W报名了一个快速阅读培训课。出于好奇,我拿着他的培训资料进行了一个月的自我训练,并一度深陷其中。材料主要是无规则的符号以及横跨A4纸的连线,通过视线快速移动,扩大视幅来提升信息的接受速度,又通过图案和符号锻炼大脑的视觉记忆,摆脱音读习惯。那一个月,我沉溺其中,每天用很多的时间练习。一个月后,我确实做到了快速阅读,以句群接受信息,一目一行。只是速度虽快,却读过无痕,该知道的全忘记了。
- 抖音返利平台有哪些?值得推荐抖音返利app有哪些?
氧惠超好用
随着抖音电商的崛起,越来越多的用户开始关注抖音返利平台希望通过这些平台在享受购物乐趣的同时,也能获得一定的返利优惠。那么,面对众多的抖音返利平台,哪个返利最高呢?本文将为您深入解析,助您找到最优选择。氧惠APP是与以往完全不同的抖客+淘客app!2024全新模式,我的直推也会放到你下面。主打:带货高补贴,深受各位带货团队长喜爱(训练营导师每天出单带货几万单)。注册即可享受高补贴+0撸+捡漏等带货新
- 2023-05-11 关于科研
姐弟的老师妈妈
越来越觉得,科研并没有想象中那么难。为何呢?科研的过程不难。随着对科研的进一步深入了解发现:科研其实就是将自己在工作中遇到的问题——解决问题的方法、过程——问题解决后的收获做一个完整的记录。这其实是我们在工作中一直都在做的事情。科研过程的记录难。用最少的字表达清楚自己的想法,应该是科研成果能够称得上是成果,并可能被推广的精髓所在。从提出问题开始:科研题目就是明确的方向——让自己和旁人都能通过看见题
- 2021年化工自动化控制仪表考试及化工自动化控制仪表考试技巧
女王219
安全生产模拟考试一点通安全生产一点通题库
题库来源:安全生产模拟考试一点通公众号小程序化工自动化控制仪表考试参考答案及化工自动化控制仪表考试试题解析是安全生产模拟考试一点通题库老师及化工自动化控制仪表操作证已考过的学员汇总,相对有效帮助化工自动化控制仪表考试技巧学员顺利通过考试。1、【单选题】辐射传热()任何介质做媒介。(A)A、不需要B、需要C、有时需要2、【单选题】同一密度的液体深度越深,压强()。(B)A、越小B、越大C、基本不变3
- 大模型训练数据库Common Crawl
WindyChanChan
数据集语言模型数据库
CommonCrawl介绍CommonCrawl是一个非营利组织,致力于通过大规模分布式爬虫系统定期抓取整个Web并将其存储在一个可公开访问的数据库中。CommonCrawl的数据收集和处理过程包括使用Python开源爬虫工具收集全球范围内的网站数据,并将其上传到CommonCrawl基金会的数据仓库中。该项目从2008年开始,至今已经积累了大量的原始网页数据、元数据和文本提取数据。这些数据
- 让你的孩子悄悄拔尖
水墨烟岚
帮助孩子找到适合自己的方法,相信我,只要正确地努力,孩子的成绩一定会进步!1.这些准备一定要有:都有一个错题本;都有一个好题本;新课之前一定先预习;先复习后做作业;做作业要计时(限时训练)。2.计划管理——有规律长计划,短安排在制定一个长期目标的同时,一定要制定一个短期学习目标,这个目标要切合自己的实际,通过努力是完全可以实现的。最重要的是,能管住自己,也就挡住了各种学习上的负面干扰,如此,那个“
- apache ftpserver-CentOS config
gengzg
apache
<server xmlns="http://mina.apache.org/ftpserver/spring/v1"
xmlns:xsi="http://www.w3.org/2001/XMLSchema-instance"
xsi:schemaLocation="
http://mina.apache.o
- 优化MySQL数据库性能的八种方法
AILIKES
sqlmysql
1、选取最适用的字段属性 MySQL可以很好的支持大数据量的存取,但是一般说来,数据库中的表越小,在它上面执行的查询也就会越快。因此,在创建表的时候,为了获得更好的 性能,我们可以将表中字段的宽度设得尽可能小。例如,在定义邮政编码这个字段时,如果将其设置为CHAR(255),显然给数据库增加了不必要的空间,甚至使用VARCHAR这种类型也是多余的,因为CHAR(6)就可以很
- JeeSite 企业信息化快速开发平台
Kai_Ge
JeeSite
JeeSite 企业信息化快速开发平台
平台简介
JeeSite是基于多个优秀的开源项目,高度整合封装而成的高效,高性能,强安全性的开源Java EE快速开发平台。
JeeSite本身是以Spring Framework为核心容器,Spring MVC为模型视图控制器,MyBatis为数据访问层, Apache Shiro为权限授权层,Ehcahe对常用数据进行缓存,Activit为工作流
- 通过Spring Mail Api发送邮件
120153216
邮件main
原文地址:http://www.open-open.com/lib/view/open1346857871615.html
使用Java Mail API来发送邮件也很容易实现,但是最近公司一个同事封装的邮件API实在让我无法接受,于是便打算改用Spring Mail API来发送邮件,顺便记录下这篇文章。 【Spring Mail API】
Spring Mail API都在org.spri
- Pysvn 程序员使用指南
2002wmj
SVN
源文件:http://ju.outofmemory.cn/entry/35762
这是一篇关于pysvn模块的指南.
完整和详细的API请参考 http://pysvn.tigris.org/docs/pysvn_prog_ref.html.
pysvn是操作Subversion版本控制的Python接口模块. 这个API接口可以管理一个工作副本, 查询档案库, 和同步两个.
该
- 在SQLSERVER中查找被阻塞和正在被阻塞的SQL
357029540
SQL Server
SELECT R.session_id AS BlockedSessionID ,
S.session_id AS BlockingSessionID ,
Q1.text AS Block
- Intent 常用的用法备忘
7454103
.netandroidGoogleBlogF#
Intent
应该算是Android中特有的东西。你可以在Intent中指定程序 要执行的动作(比如:view,edit,dial),以及程序执行到该动作时所需要的资料 。都指定好后,只要调用startActivity(),Android系统 会自动寻找最符合你指定要求的应用 程序,并执行该程序。
下面列出几种Intent 的用法
显示网页:
- Spring定时器时间配置
adminjun
spring时间配置定时器
红圈中的值由6个数字组成,中间用空格分隔。第一个数字表示定时任务执行时间的秒,第二个数字表示分钟,第三个数字表示小时,后面三个数字表示日,月,年,< xmlnamespace prefix ="o" ns ="urn:schemas-microsoft-com:office:office" />
测试的时候,由于是每天定时执行,所以后面三个数
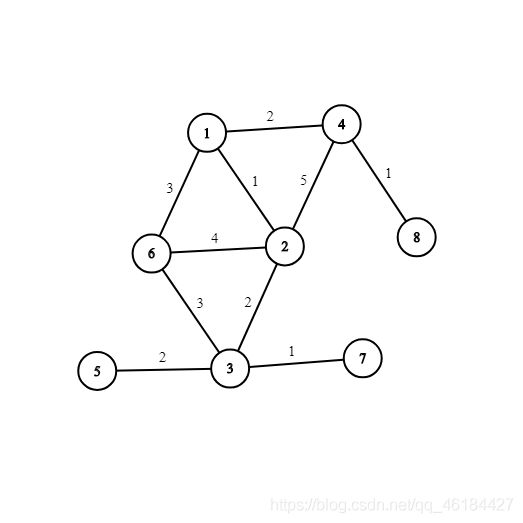
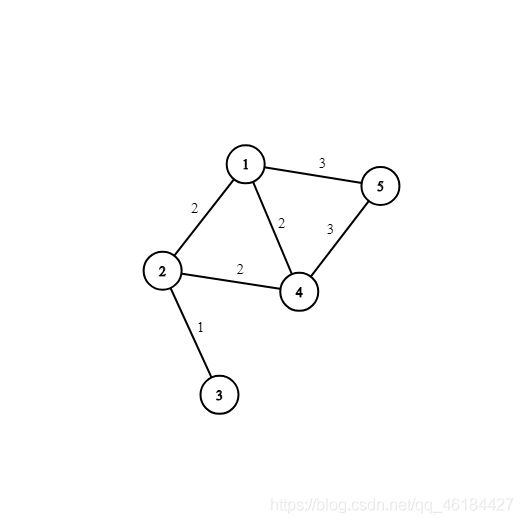
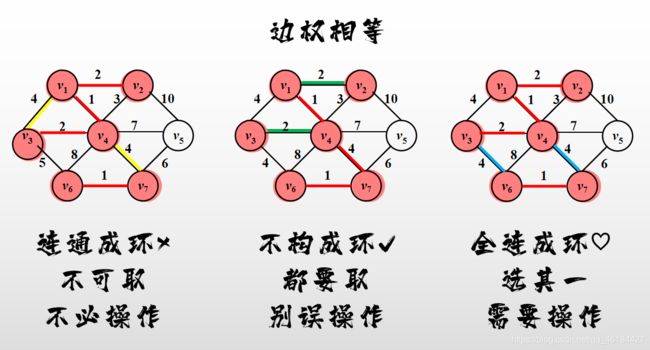
- POJ 2421 Constructing Roads 最小生成树
aijuans
最小生成树
来源:http://poj.org/problem?id=2421
题意:还是给你n个点,然后求最小生成树。特殊之处在于有一些点之间已经连上了边。
思路:对于已经有边的点,特殊标记一下,加边的时候把这些边的权值赋值为0即可。这样就可以既保证这些边一定存在,又保证了所求的结果正确。
代码:
#include <iostream>
#include <cstdio>
- 重构笔记——提取方法(Extract Method)
ayaoxinchao
java重构提炼函数局部变量提取方法
提取方法(Extract Method)是最常用的重构手法之一。当看到一个方法过长或者方法很难让人理解其意图的时候,这时候就可以用提取方法这种重构手法。
下面是我学习这个重构手法的笔记:
提取方法看起来好像仅仅是将被提取方法中的一段代码,放到目标方法中。其实,当方法足够复杂的时候,提取方法也会变得复杂。当然,如果提取方法这种重构手法无法进行时,就可能需要选择其他
- 为UILabel添加点击事件
bewithme
UILabel
默认情况下UILabel是不支持点击事件的,网上查了查居然没有一个是完整的答案,现在我提供一个完整的代码。
UILabel *l = [[UILabel alloc] initWithFrame:CGRectMake(60, 0, listV.frame.size.width - 60, listV.frame.size.height)]
- NoSQL数据库之Redis数据库管理(PHP-REDIS实例)
bijian1013
redis数据库NoSQL
一.redis.php
<?php
//实例化
$redis = new Redis();
//连接服务器
$redis->connect("localhost");
//授权
$redis->auth("lamplijie");
//相关操
- SecureCRT使用备注
bingyingao
secureCRT每页行数
SecureCRT日志和卷屏行数设置
一、使用securecrt时,设置自动日志记录功能。
1、在C:\Program Files\SecureCRT\下新建一个文件夹(也就是你的CRT可执行文件的路径),命名为Logs;
2、点击Options -> Global Options -> Default Session -> Edite Default Sett
- 【Scala九】Scala核心三:泛型
bit1129
scala
泛型类
package spark.examples.scala.generics
class GenericClass[K, V](val k: K, val v: V) {
def print() {
println(k + "," + v)
}
}
object GenericClass {
def main(args: Arr
- 素数与音乐
bookjovi
素数数学haskell
由于一直在看haskell,不可避免的接触到了很多数学知识,其中数论最多,如素数,斐波那契数列等,很多在学生时代无法理解的数学现在似乎也能领悟到那么一点。
闲暇之余,从图书馆找了<<The music of primes>>和<<世界数学通史>>读了几遍。其中素数的音乐这本书与软件界熟知的&l
- Java-Collections Framework学习与总结-IdentityHashMap
BrokenDreams
Collections
这篇总结一下java.util.IdentityHashMap。从类名上可以猜到,这个类本质应该还是一个散列表,只是前面有Identity修饰,是一种特殊的HashMap。
简单的说,IdentityHashMap和HashM
- 读《研磨设计模式》-代码笔记-享元模式-Flyweight
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
import java.util.ArrayList;
import java.util.Collection;
import java.util.HashMap;
import java.util.List;
import java
- PS人像润饰&调色教程集锦
cherishLC
PS
1、仿制图章沿轮廓润饰——柔化图像,凸显轮廓
http://www.howzhi.com/course/retouching/
新建一个透明图层,使用仿制图章不断Alt+鼠标左键选点,设置透明度为21%,大小为修饰区域的1/3左右(比如胳膊宽度的1/3),再沿纹理方向(比如胳膊方向)进行修饰。
所有修饰完成后,对该润饰图层添加噪声,噪声大小应该和
- 更新多个字段的UPDATE语句
crabdave
update
更新多个字段的UPDATE语句
update tableA a
set (a.v1, a.v2, a.v3, a.v4) = --使用括号确定更新的字段范围
- hive实例讲解实现in和not in子句
daizj
hivenot inin
本文转自:http://www.cnblogs.com/ggjucheng/archive/2013/01/03/2842855.html
当前hive不支持 in或not in 中包含查询子句的语法,所以只能通过left join实现。
假设有一个登陆表login(当天登陆记录,只有一个uid),和一个用户注册表regusers(当天注册用户,字段只有一个uid),这两个表都包含
- 一道24点的10+种非人类解法(2,3,10,10)
dsjt
算法
这是人类算24点的方法?!!!
事件缘由:今天晚上突然看到一条24点状态,当时惊为天人,这NM叫人啊?以下是那条状态
朱明西 : 24点,算2 3 10 10,我LX炮狗等面对四张牌痛不欲生,结果跑跑同学扫了一眼说,算出来了,2的10次方减10的3次方。。我草这是人类的算24点啊。。
然后么。。。我就在深夜很得瑟的问室友求室友算
刚出完题,文哥的暴走之旅开始了
5秒后
- 关于YII的菜单插件 CMenu和面包末breadcrumbs路径管理插件的一些使用问题
dcj3sjt126com
yiiframework
在使用 YIi的路径管理工具时,发现了一个问题。 <?php
- 对象与关系之间的矛盾:“阻抗失配”效应[转]
come_for_dream
对象
概述
“阻抗失配”这一词组通常用来描述面向对象应用向传统的关系数据库(RDBMS)存放数据时所遇到的数据表述不一致问题。C++程序员已经被这个问题困扰了好多年,而现在的Java程序员和其它面向对象开发人员也对这个问题深感头痛。
“阻抗失配”产生的原因是因为对象模型与关系模型之间缺乏固有的亲合力。“阻抗失配”所带来的问题包括:类的层次关系必须绑定为关系模式(将对象
- 学习编程那点事
gcq511120594
编程互联网
一年前的夏天,我还在纠结要不要改行,要不要去学php?能学到真本事吗?改行能成功吗?太多的问题,我终于不顾一切,下定决心,辞去了工作,来到传说中的帝都。老师给的乘车方式还算有效,很顺利的就到了学校,赶巧了,正好学校搬到了新校区。先安顿了下来,过了个轻松的周末,第一次到帝都,逛逛吧!
接下来的周一,是我噩梦的开始,学习内容对我这个零基础的人来说,除了勉强完成老师布置的作业外,我已经没有时间和精力去
- Reverse Linked List II
hcx2013
list
Reverse a linked list from position m to n. Do it in-place and in one-pass.
For example:Given 1->2->3->4->5->NULL, m = 2 and n = 4,
return
- Spring4.1新特性——页面自动化测试框架Spring MVC Test HtmlUnit简介
jinnianshilongnian
spring 4.1
目录
Spring4.1新特性——综述
Spring4.1新特性——Spring核心部分及其他
Spring4.1新特性——Spring缓存框架增强
Spring4.1新特性——异步调用和事件机制的异常处理
Spring4.1新特性——数据库集成测试脚本初始化
Spring4.1新特性——Spring MVC增强
Spring4.1新特性——页面自动化测试框架Spring MVC T
- Hadoop集群工具distcp
liyonghui160com
1. 环境描述
两个集群:rock 和 stone
rock无kerberos权限认证,stone有要求认证。
1. 从rock复制到stone,采用hdfs
Hadoop distcp -i hdfs://rock-nn:8020/user/cxz/input hdfs://stone-nn:8020/user/cxz/运行在rock端,即源端问题:报版本
- 一个备份MySQL数据库的简单Shell脚本
pda158
mysql脚本
主脚本(用于备份mysql数据库): 该Shell脚本可以自动备份
数据库。只要复制粘贴本脚本到文本编辑器中,输入数据库用户名、密码以及数据库名即可。我备份数据库使用的是mysqlump 命令。后面会对每行脚本命令进行说明。
1. 分别建立目录“backup”和“oldbackup” #mkdir /backup #mkdir /oldbackup
- 300个涵盖IT各方面的免费资源(中)——设计与编码篇
shoothao
IT资源图标库图片库色彩板字体
A. 免费的设计资源
Freebbble:来自于Dribbble的免费的高质量作品。
Dribbble:Dribbble上“免费”的搜索结果——这是巨大的宝藏。
Graphic Burger:每个像素点都做得很细的绝佳的设计资源。
Pixel Buddha:免费和优质资源的专业社区。
Premium Pixels:为那些有创意的人提供免费的素材。
- thrift总结 - 跨语言服务开发
uule
thrift
官网
官网JAVA例子
thrift入门介绍
IBM-Apache Thrift - 可伸缩的跨语言服务开发框架
Thrift入门及Java实例演示
thrift的使用介绍
RPC
POM:
<dependency>
<groupId>org.apache.thrift</groupId>