ACM - 数学小白入门:数论 / 高精度 / 组合 / 博弈论
ACM 数学
- 一、数论
-
- 1、素数
-
- 线性筛模板
- 例题1、区间筛素数(线性筛+埃氏筛) :POJ 2689 Prime Distance
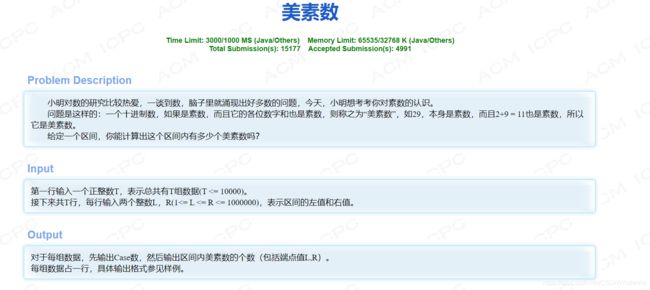
- 例题2、前缀和 + 线性筛 :HDU 4548 美素数
- 例题3、区间分解质因数 + 二分:HDU 6287 口算训练
- 2、约数
-
- 例题1、约数个数定理:E - 解方程(牛客小白月赛31)
- 3、欧拉函数 / 定理+费马小定理
-
- 定理内容
- 例题1、隔板原理+求组合数+费马降幂+快速幂:HDU 4704 Sum
- 4、快速幂、龟速乘、矩阵乘法
-
- (1)快速幂
- (2)龟速乘
- (3)矩阵乘法
- 5、逆元
-
- 例题1、逆元 + 快速幂 :HDU 1576 A / B
- 6、gcd + exgcd
-
- 解线性同余方程
- 7、卢卡斯定理
-
- 例题1、卢卡斯+组合推论:HDU 5226 Tom and matrix
- 8、高斯消元
-
- 线性方程组--模板题
- 异或线性方程组:AcWing 884
- 同余方程组:HDU 5755 Gambler Bo
- 9、斐波那契数列
-
- O(1)通项公式
- 例题1、循环节:HDU 1021 Fibonacci Again
- 例题2、构造非法三角形边长
- 10、中国剩余定理
-
- (1)定理内容
- (2)拓展中国剩余定理
- 二、高精度
-
- 1、加减乘除模板
-
- 大数加大数
- 大数减大数
- 大数乘小数
- 大数除小数
- 例题1、求阶乘 : HDU 1042 N!
- 例题2、高精度乘+除法求卡特兰数 :HDU 1134 Game of Connections
- 三、组合数学
-
- 1、求组合数
- 2、卡特兰数
-
- 基本定义和公式
- 常见题型
- 例题1、高精度乘+除求卡特兰数 HDU 1134 Game of Connections
- 例题2、快速幂+逆元求卡特兰数 HDU 5673 Robot
- 3、容斥原理
-
- 模板题 AcWing 890. 能被整除的数
- 四、博弈论
-
- 1、尼姆博弈
-
- 模板题:HDU - 2176 取(m堆)石子游戏
- 例题1、AcWing 892. 台阶-Nim游戏
- 2、SG 函数
-
- 模板题 AcWing 893. 集合-Nim游戏
- 例题1、AcWing 894. 拆分-Nim游戏
- 五、常用结论
-
- 1、求两个数不能组合成的最大整数
一、数论
1、素数
线性筛模板
int primes[N], cnt; // primes[]存储所有素数
bool st[N]; // st[x]存储x是否被筛掉
void get_primes(int n)
{
for (int i = 2; i <= n; i ++ )
{
if (!st[i]) primes[cnt ++ ] = i;
for (int j = 0; primes[j] <= n / i; j ++ )
{
st[primes[j] * i] = true;
if (i % primes[j] == 0) break;
}
}
}
例题1、区间筛素数(线性筛+埃氏筛) :POJ 2689 Prime Distance
原题链接:http://poj.org/problem?id=2689


题目大意
对于任意询问的区间【l,r】,计算出两个相邻素数之间的最短和最长距离。
如果有距离相等的,就输出素数之和最小的。
(例如 2 和 3 之间没有其他素数,则称之为两个相邻素数,且此时距离最短)
思路
因为 l 和 r 的范围很大,所以也不可能把primes和st开很大,但是无论如何最终都是要落实到用素数把合数筛掉上。
题目上 r 的上限是 21 亿多,那么当我们求出 50000 以内的所有素数的时候,假如一个在【l,r】上的数是合数,那么它的质因子一定至少有一个落在【2,50000】上。
换句话说,这道题就是筛两次。
第一次用线性筛筛出【2,50000】之间的素数,第二次用记录在primes的素数数组把【l,r】上的合数筛掉()。
第二次筛具体来说,就是从头开始遍历primes数组,找到【l,r】区间上,第一个能被primes [ i ] 整除的数,然后往下筛。
需要注意的是,如果输入的 l 是 1,那么需要把 l ++,否则会把 1 当成第一个素数;
因为在第二次筛的时候,是用 0 号索引表示【l,r】区间上的第一个数,所以要注意在找到primes [ i ] 第一个能筛的数的时候,会不会这个数是 primes [ i ] 本身。
代码
#include 例题2、前缀和 + 线性筛 :HDU 4548 美素数
原题链接:http://acm.hdu.edu.cn/showproblem.php?pid=4548

#include 例题3、区间分解质因数 + 二分:HDU 6287 口算训练
原题链接:http://acm.hdu.edu.cn/showproblem.php?pid=6287


思路
观察数据范围,发现数的大小和数的数量都在 10 ^ 5 之内,由于后面还有 m 组区间查询,所以我们需要先预处理那 n 个数,得到 n 个数的所有质因子都在哪些数出现过,出现过几次, 再通过二分区间查找 d 的每一个质因子的所在区间的上限和下限,判断 下限 - 上限 是否大于等于该质因子的数量。
代码实现方面:开一个vector数组book(动态二维数组,防止爆内存),存下 n 个数的所有质因子的信息,比如说对于题目给的样例
1
5 4
6 4 7 2 5
1 2 24
1 3 18
2 5 17
3 5 35
我们对于 6 4 7 2 5 这 5 个数处理如下:
book [ 1 ] :
book [ 2 ] :1、2、2、4
book [ 3 ] :1
book [ 4 ] :
book [ 5 ] :5
book [ 6 ] :
book [ 7 ] :3
book [ 8 ] :
对于book [ 2 ] :1、2、2、4所表达的是:这 n 个数里面,第 1、2、4个数有质因子 2,且第 2 个数有两个。
同理,book [ 7 ] :3 所表达的是 n 个数里面,第 3 个数有质因子 7 ,其他以此类推。
当获得 book 后,我们对于每一组 l r d 的查询,先分解出 d 的质因子,然后对 book [质因子] 二分查 l 的下限、r 的上限,由此判断book里面有没有足够的质因子和 d 对应。
综上。
代码
#include 2、约数
例题1、约数个数定理:E - 解方程(牛客小白月赛31)
传送门:https://blog.csdn.net/CSDNWudanna/article/details/112727606
3、欧拉函数 / 定理+费马小定理
定理内容
(1)欧拉函数 φ(n):表示从1 到 n 一共有多少个和 n 互质。
(在数论,对正整数n,欧拉函数是小于n的正整数中与n互质的数的数目)
φ(n) = n (1 - 1 / p1) (1 - 1 / p2) (1 - 1 / p3)……(1 - 1 / pk),其中 pi 为 n 的质因子。
//筛法求欧拉函数
int primes[N], cnt; // primes[]存储所有素数
int euler[N]; // 存储每个数的欧拉函数
bool st[N]; // st[x]存储x是否被筛掉
void get_eulers(int n)
{
euler[1] = 1;
for (int i = 2; i <= n; i ++ )
{
if (!st[i])
{
primes[cnt ++ ] = i;
euler[i] = i - 1;
}
for (int j = 0; primes[j] <= n / i; j ++ )
{
int t = primes[j] * i;
st[t] = true;
if (i % primes[j] == 0)
{
euler[t] = euler[i] * primes[j];
break;
}
euler[t] = euler[i] * (primes[j] - 1);
}
}
}
(2)欧拉定理:若 a 和 n 互质,那么 a ^ φ(n) ≡ 1 (mod n)
(3)费马小定理:若 n 为质数,那么φ(n) = n - 1,此时可得 a ^ (n - 1) ≡ 1 (mod n)。
例题1、隔板原理+求组合数+费马降幂+快速幂:HDU 4704 Sum
原题链接:http://acm.hdu.edu.cn/showproblem.php?pid=4704
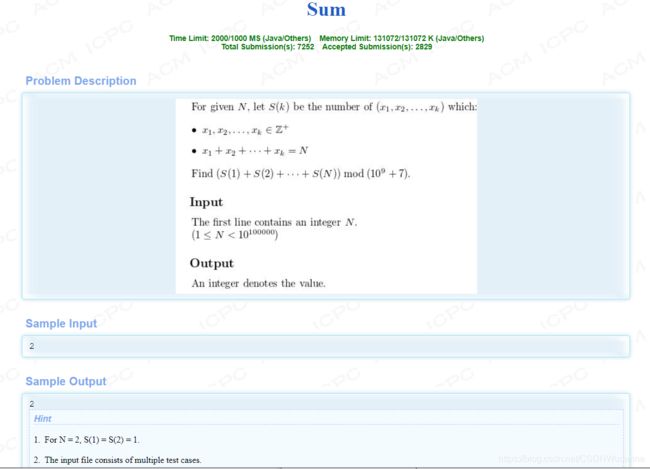
题目大意
给定一个数n ,将其分解,S(k) 表示将 n 拆成 k 个数的方案数,
求 sum( S(k) ) ,其中1 <= k <= n;
思路
① 隔板原理
n 最多可以被拆成 n 个 1, 那么在这些 1 之间,最多有 n - 1 个位置可以放隔板,假如现在在这 n - 1个位置中放 2 个隔板,那么这 n 个 1 会被分成 3 个区间,此时能得到 3 个数,而 S(3) = ( n − 1 2 ) \tbinom{n - 1}{2} (2n−1),依次类推可得出,S(1)、S(2)、S(3)……S(n) 分别为 ( n − 1 0 ) \tbinom{n - 1}{0} (0n−1)、 ( n − 1 1 ) \tbinom{n - 1}{1} (1n−1)、 ( n − 1 2 ) \tbinom{n - 1}{2} (2n−1)…… ( n − 1 n − 1 ) \tbinom{n - 1}{n - 1} (n−1n−1)。
②求组合数
故而我们可以发现 ans = ( n − 1 0 ) \tbinom{n - 1}{0} (0n−1)+ ( n − 1 1 ) \tbinom{n - 1}{1} (1n−1)+ ( n − 1 2 ) \tbinom{n - 1}{2} (2n−1)+……+ ( n − 1 n − 1 ) \tbinom{n - 1}{n - 1} (n−1n−1) = 2 ^ (n - 1)
(n - 1 个隔板的位置,每个位置都有放隔板和不放隔板两种情况,所以所有取值总和就是 2 ^ (n - 1) )
③费马小定理降幂
n 的 范围很大,所以需要对 n - 1 降幂才能求 2 ^ (n - 1) 。
我们可以发现,2 和 1e9 + 7 都是质数(满足 p 是质数且 a、p互质),根据费马小定理有 2 ^ (1e9 + 6) ≡ 1(mod 1e9 + 7),而当 n - 1 > 1e9 + 7 时,2 ^ (n - 1) = 2 ^ (1e9 + 6) * 2 ^ (n - 1 - (1e9 + 6)),换句话说,2 ^ (n - 1) = 2 ^ ((n - 1) % (1e9 + 6))。
④快速幂求解
当把 n - 1 降幂后,求 2 的幂次方最高还是可以达到 1e9 + 5,所以不能直接暴力求,需要用快速幂边乘边求模。
代码
#include 4、快速幂、龟速乘、矩阵乘法
(1)快速幂
//求 m^k mod p,时间复杂度 O(logk)。
int qmi(int m, int k, int p)
{
int res = 1 % p, t = m;
while (k)
{
if (k&1) res = res * t % p;
t = t * t % p;
k >>= 1;
}
return res;
}
(2)龟速乘
当快速幂过程中会爆 ll 时,有关于乘法的部分需要用龟速乘解决。
//龟速乘:求(a * b)% p
ll qmul(ll a, ll b, ll p) {
ll res = 0, t = a;
while (b) {
if (b & 1) res = (res + t) % p;
b >>= 1;
t = (t + t) % p;
}
return res;
}
//快速幂:求10的k次方mod c
ll qmi(ll a, ll k, ll p) {
ll res = 1, t = a;
while (k) {
if (k & 1) res = qmul(res, t, p);
k >>= 1;
t = qmul(t, t, p);
}
return res == 1;
}
(3)矩阵乘法
AcWing 1303. 斐波那契前 n 项和
原题链接:https://www.acwing.com/problem/content/description/1305/

// 构造乘法矩阵+快速幂思想
#include5、逆元
若 a / b ≡ a * x (mod p) ,则称 x 为 b 在模 p 下的逆元。
若 p 为质数,且 b、p 互质时,由费马小定理可得 x = b ^ (p - 2)。
例题1、逆元 + 快速幂 :HDU 1576 A / B
原题链接:http://acm.hdu.edu.cn/showproblem.php?pid=1576

思路
(A / B) % 9973 = (A * B-1) % 9973 = A % 9973 * B-1 % 9973 = n * B-1 % 9973。
B-1 = B ^ (9973 - 2) (B和9973互质,且9973是质数,所以用费马小定理可求逆元)
最后再用快速幂求一下结果即可。
代码
#include 6、gcd + exgcd
int gcd(int a, int b)
{
return b ? gcd(b, a % b) : a;
}
// 求x, y,使得ax + by = gcd(a, b)
int exgcd(int a, int b, int &x, int &y)
{
if (!b)
{
x = 1; y = 0;
return a;
}
int d = exgcd(b, a % b, y, x);
y -= (a/b) * x;
return d;
}
解线性同余方程
给定 a、b、m,求一个 x ,使得 ax ≡ b (mod m)。
易知,存在一个整数 y 使得 ax = my + b,则有 ax - my = b。
令 y ’ = - y,那么 ax + my ’ = b。
故当 gcd(a,m) | b 时 x 有解。
7、卢卡斯定理
若p是质数,则对于任意整数 1 <= m <= n,有:
C(n, m) = C(n % p, m % p) * C(n / p, m / p) (mod p)
例题1、卢卡斯+组合推论:HDU 5226 Tom and matrix
原题链接:http://acm.hdu.edu.cn/showproblem.php?pid=5226
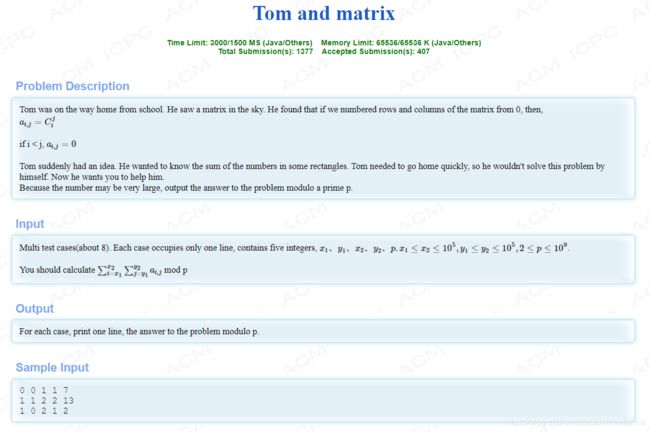
思路
首先对于组合数有
( n m ) \tbinom{n}{m} (mn)
= ( n − 1 m − 1 ) \tbinom{n - 1}{m - 1} (m−1n−1) + ( n − 1 m ) \tbinom{n - 1}{m} (mn−1)
= ( n − 1 m − 1 ) \tbinom{n - 1}{m - 1} (m−1n−1) + ( n − 2 m − 1 ) \tbinom{n - 2}{m - 1} (m−1n−2) + ( n − 2 m ) \tbinom{n - 2}{m} (mn−2)
= ( n − 1 m − 1 ) \tbinom{n - 1}{m - 1} (m−1n−1) + ( n − 2 m − 1 ) \tbinom{n - 2}{m - 1} (m−1n−2) + ( n − 3 m − 1 ) \tbinom{n - 3}{m - 1 } (m−1n−3) + ( n − 3 m ) \tbinom{n - 3}{m} (mn−3)
= ……
针对 x1 = 5,x2 = 8, y1 = 2,y2 = 4 这个样例,我们需要求:
( 5 2 ) \tbinom{5}{2} (25) ( 5 3 ) \tbinom{5}{3} (35) ( 5 4 ) \tbinom{5}{4} (45)
( 6 2 ) \tbinom{6}{2} (26) ( 6 3 ) \tbinom{6}{3} (36) ( 6 4 ) \tbinom{6}{4} (46)
( 7 2 ) \tbinom{7}{2} (27) ( 7 3 ) \tbinom{7}{3} (37) ( 7 4 ) \tbinom{7}{4} (47)
( 8 2 ) \tbinom{8}{2} (28) ( 8 3 ) \tbinom{8}{3} (38) ( 8 4 ) \tbinom{8}{4} (48)
依靠上面的推论我们可以得出: ( 9 5 ) \tbinom{9}{5} (59) = ( 8 4 ) \tbinom{8}{4} (48) + ( 7 4 ) \tbinom{7}{4} (47) + ( 6 4 ) \tbinom{6}{4} (46) + ( 5 4 ) \tbinom{5}{4} (45) + ( 5 5 ) \tbinom{5}{5} (55)
那么对于
( 8 4 ) \tbinom{8}{4} (48)
( 7 4 ) \tbinom{7}{4} (47)
( 6 4 ) \tbinom{6}{4} (46)
( 5 4 ) \tbinom{5}{4} (45)
这一列, ( 9 5 ) \tbinom{9}{5} (59) - ( 5 5 ) \tbinom{5}{5} (55) = ( 8 4 ) \tbinom{8}{4} (48) + ( 7 4 ) \tbinom{7}{4} (47) + ( 6 4 ) \tbinom{6}{4} (46) + ( 5 4 ) \tbinom{5}{4} (45)
这样就可以对问题的规模降一个维度,防止tle。
另外这道题需要对 ( n m ) \tbinom{n}{m} (mn)判断一下是否 n >= m。
(题目应该是默认输入的 x1、x2、y1、y2 合法)
代码
#include 8、高斯消元
线性方程组–模板题
基本上,消元的顺序是:
- 将方程的所有系数存成矩阵的形式(二维数组)
- 枚举每一列
- 对当前列,找出 r ~ n 行中行首绝对值最大的那一行 t ,即找出 fabs(a [ i ] [ c ])
- 交换第 t 行和第 r 行,使得每一次枚举, r 行的行首都是绝对值最大(目的是为了提高精度)
- 将交换后的第 r 行的行首化为单位1
- 将第 r + 1 行到第 n 行的第 c 列全部化为 0
- ++ r,++ c 进入下一行下一列
- 【另】:假如获得的第 t 行的行首为 0 ,即:a [ t ] [ c ] < eps,那么说明该列全为0,该列不用处理,但是该行还是需要继续消元,所以只需要 ++ c,而不用 ++ r。
获得方程组的解:
如果 r <= n,说明秩不为 n,假如出现 0 = !0 的情况,说明方程组无解,反之方程组有无穷多解;
而如果 r == n + 1,即秩为 n ,那么说明恰好有唯一解,此时还需要把每一个方程消成只剩下一项,才能得到最终解。(从倒数第二行开始消起)
#include 异或线性方程组:AcWing 884
传送门:AcWing 884 高斯消元解异或线性方程组
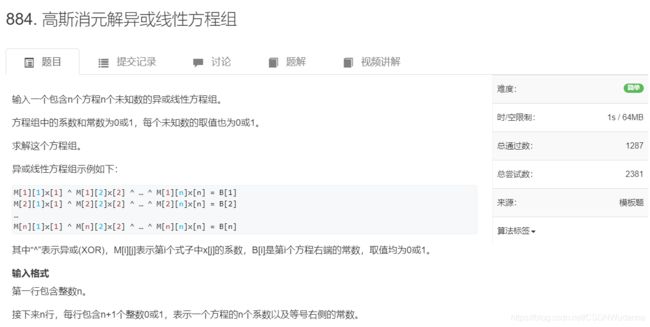

思路
假如现在有一个增广矩阵:
1 1 0 1 1
1 0 1 0 1
1 1 0 0 0
1 0 1 0 1
设对应的四个解分别为 a、b、c、d,我们先取出前两行:
1 1 0 1 1
1 0 1 0 1
我们可以发现,其实这两行表达的就是:
a ^ b ^ d = 1
a ^ c = 1
那么如果要消掉第二行的行首,我们只需要将第一行所有系数为 1 的数来异或第二行所有系数为 1 的数(当然同时最右边的常数也需要异或一下),即:
a ^ b ^ d ^ a ^ c = c ^ b ^ d = 1 ^ 1 = 0,故而这两行可以变换为:
1 1 0 1 1
0 1 1 1 0
再像解一般的线性方程组那样,逐行逐列地搞一遍,就可以得到这个矩阵的秩和最终的解。
#include 同余方程组:HDU 5755 Gambler Bo
原题链接:http://acm.hdu.edu.cn/showproblem.php?pid=5755


题目大意
对于一个 n * m 的矩阵,若在位置(x,y)处操作一次,那么(x,y)自增2,而在(x,y)的上下左右四个位置自增1,问在所有矩阵的数值 mod 3 的前提下,输出一个能在 2 * n * m 次操作内将矩阵所有数值变为 0 的操作方案。
思路
(关于本题,x 和 y 的坐标从 1 开始)
首先这是一个开关灯问题,但是因为 n 和 m 数据范围在 30 以内,如果暴力枚举第一行,显然 2 ^ 30 会tle,所以这里是将矩阵的 n * m 个位置,看成 n * m 个变量,列出 n * m 个同余方程,然后解这个方程组求的每个位置的操作次数。
比如说,对于样例
1
2 3
2 1 2
0 2 0
首先设该情况下各个位置的操作方案如下:
x y z
a b c
那么要想将(1,1)处的 2 变为 0 ,那么在mod 3 的前提下,(1,1)需要操作自增 1 才能达到想要的效果。
而(1,1)这个位置想要增加 1 + 3p,那么 1 + 3p = 2 * x + y + a。
换句话说 2x + y + 0z + a + 0b + 0c ≡ 1 (mod 3)
依次类推,就可以得到 n * m 个同余方程,进而用高斯消元解这个方程组(记得先 + 3 再 mod 3 防止出现负数(合理性:比如说 -2x + 3x,这在mod 3 的情况下是不影响结果的,详见同余的性质))
ps. 最后的求变量的值用扩展欧几里得,列出最后两行就能模拟出来。
代码
#include 9、斐波那契数列
若形如 F(1)=1,F(2)=1,F(n)=F(n-1)+F(n-2),n≥3,则称这些数构成斐波那契数列。
O(1)通项公式
int fib(int n) {
double sqrt5 = sqrt(5);
double fibN = pow((1 + sqrt5) / 2, n) - pow((1 - sqrt5) / 2, n);
return round(fibN / sqrt5);
}
例题1、循环节:HDU 1021 Fibonacci Again

思路
用程序跑出第 0 ~ 22 个该数列的数,发现第 2、6、10、14、18、22个数可以被 3 整除,所以直接利用规律就可以求。
代码
#include 例题2、构造非法三角形边长
原题链接:2021牛客寒假算法基础集训营2 J 牛牛想要成为hacker


思路
如果三条边能构成三角形,那么最小的两条边之和必定大于第三边。
换句话说,要想构造出不合法的三边,那么最小的两条边之和必定小于等于第三边,但是又要尽可能地使得有更多的数可以凑,第三边 == 最小两边之和是最划算的,而边的长度最小为1,那么我们可以构造出:
1,1,2 , 3, 5, 8, 13, 21, 34 ……(明显的斐波那契数列)
但是因为斐波那契增长很快,而 n 也不小,所以当 Fi 超过 1e9 的时候,就应该拿别的数来凑。这里我们会发现无论拿什么数都不太理想,因为 1 太小了,小到除了 2 ,没有别的数可以和 1 、1凑,那么这里我们就可以想,既然是 1 碍事,那就把两个 1 放到后面去,也就是构造 2 , 3, 5, 8, 13, 21, 34,……,433494437,701408733,1,1,1,1,1……至此完毕。
代码
#include 10、中国剩余定理
(1)定理内容

模板题:AcWing 1298. 曹冲养猪
原题链接:https://www.acwing.com/problem/content/1300/


#include(2)拓展中国剩余定理
AcWing 204. 表达整数的奇怪方式
原题链接:https://www.acwing.com/problem/content/description/206/
由 x ≡ mi(mod ai) 可得到前两条方程:
x = a1k1 + m1
x = a2k2 + m2
故而 a1k1 + m1 = a2k2 + m2(k1、k2未知)
移项,化简得:a1k1 - a2k2 = m2 - m1
用 exgcd 得到 k1 和 k2 以及 gcd 的值。
但是由于此时的 k1 是由 a1k1 - a2k2 = gcd 得到的,如果 (m2 - m1)% gcd != 0,说明方程无解,反之说明有解,但是 k1 需要乘上(m2 - m1)/ gcd 才是 a1k1 - a2k2 = m2 - m1 的其中一个解。
首先 k1 的通解 = k1 + (a2 / gcd)* k(其中k为任意整数)。
我们把通解代入 x = a1k1 + m1 可以得到:
x
= (k1 + (a2 / gcd)* k)* a1 + m1
= k1 * a1 + m1 + k * (a1 * a2) / gcd (其中k为任意整数)
很明显,前半部分的 k1 * a1 + m1 是常数,而最后面的 (a1 * a2) / gcd 也同样是常数,这和一开始的 x = a1k1 + m1 一样是 y = kx + b 这样的二元一次方程,由此可将起初的两条方程并成一条,重复 n - 1 次后,就只剩下一条方程,即可求解。
由于题目要求 x 最小,为了不爆 long long ,需要在过程中最小化 k1 的值(由前面可知,如果确定不会爆 ll 的话,其实 x 可以通过取模获得min,可以不用最小化k1)。
由 k1 的通解 = k1 + (a2 / gcd)* k,可知 k1 可以通过减少 abs(a2 / gcd)得到最小正整数解。
#include二、高精度
1、加减乘除模板
大数加大数
#include大数减大数
#include大数乘小数
#include大数除小数
#include例题1、求阶乘 : HDU 1042 N!
原题链接:http://acm.hdu.edu.cn/showproblem.php?pid=1042
#include 例题2、高精度乘+除法求卡特兰数 :HDU 1134 Game of Connections
原题链接:http://acm.hdu.edu.cn/showproblem.php?pid=1134
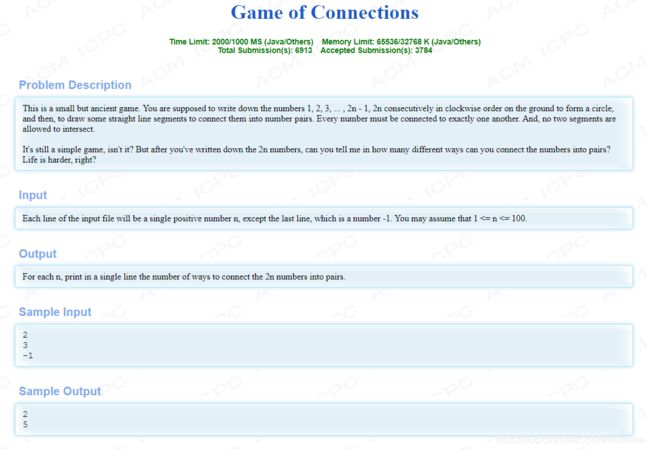
题目大意
有一个由 1、2、3、……、2n - 1、2n 顺时针围成的圆圈,现在要求每个数只能被连接一次,所有数都有且仅有一条连线,并且所有线都不会有交叉,问针对这样的 n ,连接的方案有多少种。
思路
设 f(2n)为问题的解,那么 f(2n) = f(0) * f(2n - 2) + f(2)* f(2n - 4) + f(4)* f(2n - 6) + …… + f(2n - 2)* f(0)。
其中, f(0) * f(2n - 2)可以看成是先在 1 和 2n 之间连上一条线,那么 f(0)即这条线左上角所有数的连接方案数,而 f(2n - 2)即为这条线右下角所有数的连接方案数,其他依次类推。
有这个公式可知 f (2n) = C(n),C(n)即为卡特兰数。
所以
C(n)
= ( 2 n n ) \tbinom{2n}{n} (n2n) / (n + 1)
= ((2n)!/ (n!n!))/ (n + 1)
= ((n + 2)* (n + 3)* …… * (2n))/ (1 * 2 * 3 * …… * n)。
#include 三、组合数学
1、求组合数
(1)AcWing 885. 求组合数 I
原题链接:https://www.acwing.com/problem/content/887/
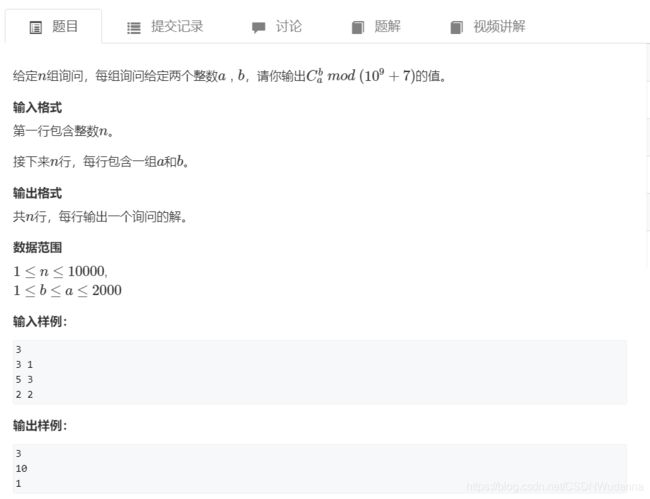
// 用杨辉三角求
#include<iostream
using namespace std;
const int mod = 1e9 + 7;
const int N = 2020;
#define ll long long
int book[N][N];
void make_table() {
for (int i = 0; i < N; ++ i) {
for (int j = 0; j <= i; ++ j) {
if (!j) book[i][j] = 1;
else book[i][j] = ((ll)book[i - 1][j] + book[i - 1][j - 1]) % mod;
}
}
}
int main() {
make_table();
int n, a, b;
scanf("%d", &n);
while (n --) {
scanf("%d%d", &a, &b);
printf("%d\n", book[a][b]);
}
}
(2)AcWing 886. 求组合数 II
原题链接:https://www.acwing.com/problem/content/888/

//用公式 C(n取m) = n ! / (m ! * (n - m) !)
#include(3)AcWing 887. 求组合数 III
原题链接:https://www.acwing.com/problem/content/description/889/
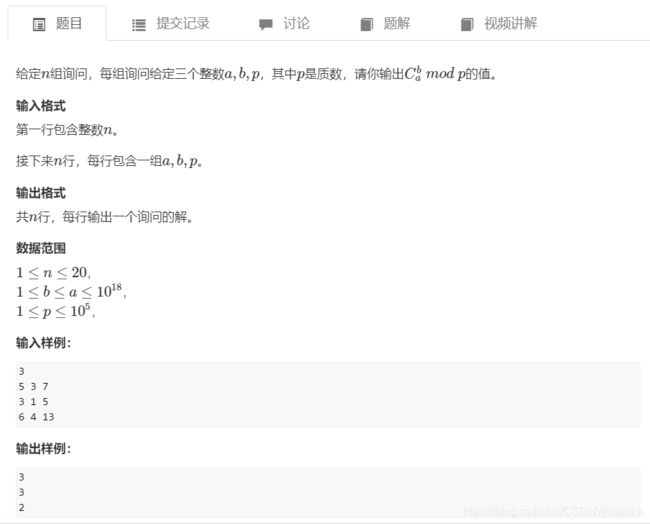
//若p是质数,则对于任意整数 1 <= m <= n,有:
// C(n, m) = C(n % p, m % p) * C(n / p, m / p) (mod p) ----- 卢卡斯定理
#include(4)AcWing 888. 求组合数 IV
原题链接:https://www.acwing.com/problem/content/890/

//统计C(n取m)是由哪些质因子相乘得到的,再用高精度计算出最终结果
#include 2、卡特兰数
基本定义和公式
给定 n 个 0 和 n 个 1,它们按照某种顺序排成一个长度为 2n 的序列,且这个序列需要满足任意前 i 个数中, 0 的个数都不少于 1 的个数。
而这样的序列的数量即满足卡特兰数:Cat(n)= ( 2 n n ) \tbinom{2n}{n} (n2n) - ( 2 n n − 1 ) \tbinom{2n}{n -1 } (n−12n) = ( 2 n n ) \tbinom{2n}{n} (n2n) / (n + 1)。
前几项为:1, 1, 2, 5, 14, 42, 132, 429, 1430, 4862, 16796, 58786, 208012, 742900, 2674440, 9694845, 35357670, 129644790, 477638700, 1767263190, 6564120420, 24466267020, 91482563640, 343059613650, 1289904147324, 4861946401452, …
为什么满足卡特兰数——传送门
由于对于组合数而言有: ( n m ) \tbinom{n}{m} (mn) = n ! / (m ! * (n - m) !) ,故而有:
C(n)
= ( 2 n n ) \tbinom{2n}{n} (n2n) / (n + 1)
= ((2n)!/ (n!n!))/ (n + 1)
= ((n + 2)* (n + 3)* …… * (2n))/ (1 * 2 * 3 * …… * n)
常见题型
题型详解:https://blog.csdn.net/wuzhekai1985/article/details/6764858
- n对括号有多少种匹配方式?—— Cat(n)
- 矩阵链乘: P=a1×a2×a3×……×an,依据乘法结合律,不改变其顺序,只用括号表示成对的乘积,试问有几种括号化的方案?—— Cat(n - 1)
- 一个栈(无穷大)的进栈序列为1,2,3,…,n,有多少个不同的合法出栈序列? —— Cat(n)
- n个节点构成的二叉树,共有多少种情形?—— Cat(n)
- 在圆上选择2n个点,将这些点成对连接起来使得所得到的n条线段不相交的方法数?—— Cat(n)
- 求一个凸多边形区域划分成三角形区域的方法数?
- 在平面直角坐标系上,每一步只能往上走或往右走,从(0,0)走到(n,n)且除了两个端点外不接触直线 y = x 的路线数量?—— 2 * Cat(n - 1)
例题1、高精度乘+除求卡特兰数 HDU 1134 Game of Connections
(在本篇博客高精度例题2里有详解)
例题2、快速幂+逆元求卡特兰数 HDU 5673 Robot
原题链接:http://acm.hdu.edu.cn/showproblem.php?pid=5673
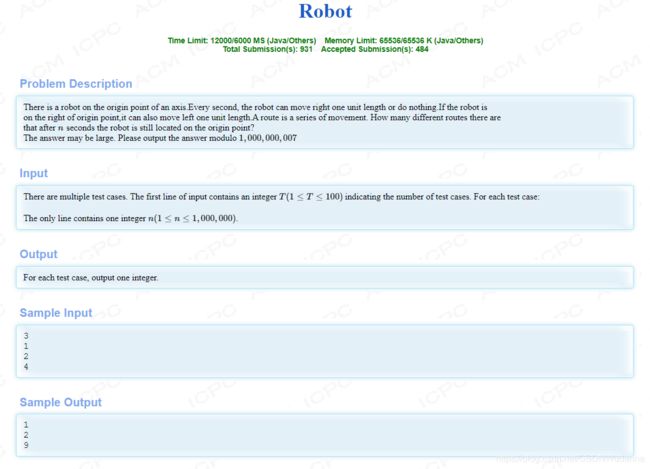
题目大意
一个机器人在坐标原点,它每秒钟可以向左或者向右移动 1 个单位,或者留在原地不动,但是任意时刻不能处于负半轴。问经历 n 秒后,机器人回到原点的方案数。
思路
首先要想回到原点,向右走的步数和向左走的步数必定是一样的,那么这就是很明显的0/1的个数问题,也就是卡特兰数。
但是因为这道题目多了一个可以停留的选择,所以需要对 0 到 n / 2 进行遍历求卡特兰数,并乘上对应的停留的方案数。
设 i 为向右走的次数,那么 ans = ( n n − 2 ∗ i ) \tbinom{n}{n - 2 * i} (n−2∗in) * ( 2 ∗ i i ) \tbinom{2 * i}{i} (i2∗i) / ( i + 1 )
i ∈ [ 0 ,n / 2 ]
( n n − 2 ∗ i ) \tbinom{n}{n - 2 * i} (n−2∗in) —— 空格方案数
( 2 ∗ i i ) \tbinom{2 * i}{i} (i2∗i) / ( i + 1 ) —— 卡特兰数
注意:题目时间限制是 6000 ms,需要对逆元预处理,不然会超时。
代码
#include 3、容斥原理
![]()
简单来说,就是在求加法的时候重叠部分被重复计算了,而在去掉重叠部分时,又去多了,所以需要再加回来二次重叠的部分循环往复。
模板题 AcWing 890. 能被整除的数
原题链接:https://www.acwing.com/problem/content/892/
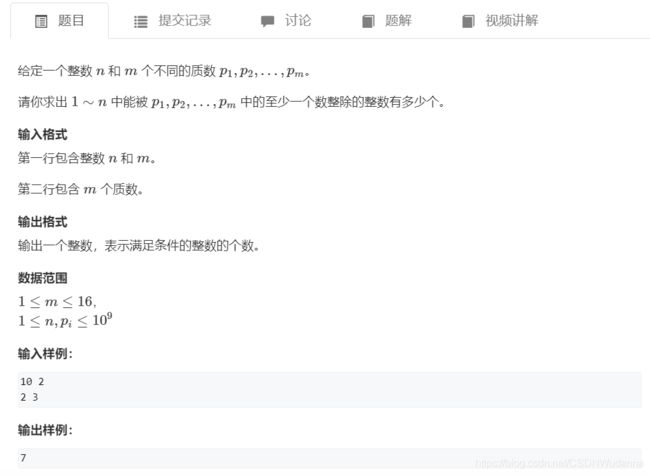
思路
对于题目样例:
10 2
2 3
我们可以列出1 ~ 10 范围内能被 2 和 3 整除的数:
S2 = {2、4、6、8、10}
S3 = {3、6、9}
而我们要计算的就是 S2 + S3 - S2 ∩ S3
显然 S2 = n / 2,S3 = n / 3,而 S2 ∩ S3 = n / (2 * 3),其余同理。
(因为题目明确说明这 m 个数一定都是素数,所以直接相乘即可)
代码
#include 四、博弈论
1、尼姆博弈
模板题:HDU - 2176 取(m堆)石子游戏
原题链接:http://acm.hdu.edu.cn/showproblem.php?pid=2176
思路
首先我们需要明确最终 n 堆石子必定会走到全部取完的状态,即 a1 ^ a2 ^ a3 …… ^ an = 0 ^ 0 ^ 0 …… ^ 0 = 0。
那么对于所有非终态,我们设 a1 ^ a2 ^ a3 …… ^ an = x :
① x == 0
无论在任意堆取任意数量,最终 a1 ^ a2 ^ a3 …… ^ an != 0
② x != 0
在最优策略下,我们完全可以在某一堆取出若干石子使得 a1 ^ a2 ^ a3 …… ^ an == 0
(例如,设 x 的二进制最高位 1 在第 k 位,则必然存在一个数 ai 的第 k 位是 1, 对 ai 堆取走 ai - (ai ^ x)颗石子,那么 ai 堆会剩下 ai ^ x 颗石子,此时 a1 ^ a2 ^ a3 ……^ ai ^ x ^ …… ^ an = x ^ x == 0)
换句话说,如果在初始状态下,a1 ^ a2 ^ a3 …… ^ an != 0,那么先手可以拿一定数目使得 xor 后变为 0,而如果此时石子还没取完,那么在后手操作后 xor 必定不等于 0,循环往复,直到某一次先手操作后 xor == 0,而且是 0 ^ 0 ^ 0 …… ^ 0 的 0,那么后手必败。
反之如果初始状态下,a1 ^ a2 ^ a3 …… ^ an == 0,那么先手必败,后手必胜。
结论:初始状态下,a1 ^ a2 ^ a3 …… ^ an != 0,先手胜,反之后手胜。
代码
#include 例题1、AcWing 892. 台阶-Nim游戏
原题链接:https://www.acwing.com/problem/content/description/894/

思路
对于序号是偶数的台阶,假如后手在第 4 阶台阶拿了 a 个放在第 3 阶,那么先手只需要从第 3 阶也拿 a 个放在第 2 阶,即先手进行镜像操作,会使得最终胜负取决于序号是奇数的台阶(因为接近地面的是奇数台阶,所以综上,后手操作偶数台阶不影响最终结果)。
对于奇数台阶,设 xor = a1 ^ a3 ^ a5 ^……
由前面尼姆游戏的模板题可知,假如 xor == 0,后手胜,反之先手胜。
这道题主要是要抽象出在第 i 阶拿若干个放在 i - 1 阶的意义。
代码
#include 2、SG 函数
设集合 S = {0、2、3}
那么 mex(S)= 1,因为mex 是求不属于集合 S 的最小自然数。
若由数 a 可以走向数 x、y、z,那么 SG(a)= mex{ SG(x) 、SG(y) 、SG(z) }。
模板题 AcWing 893. 集合-Nim游戏
原题链接:https://www.acwing.com/problem/content/895/

思路
例如对于样例第三堆中,有 7 块石头,那么有两种取法 – 拿走 2 块 or 拿走 5 块,而 SG (7) = mex{ SG(2) 、SG(5) }。
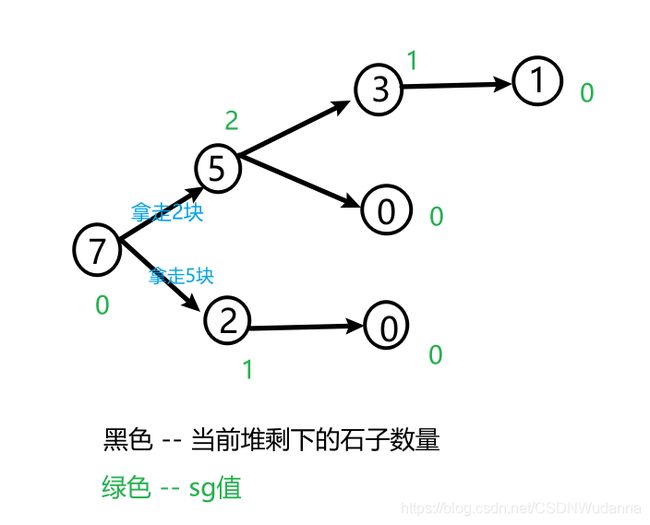
若 SG(x) != 0,说明有一种取法使得下一步的 sg == 0,而 sg == 0,说明无论怎么取再下一步的 sg 都不会为 0,即不可能是取到无路可取的状态,此时 sg 又回到一开始的 SG( x ’ ) != 0的情况,循环往复。
换而言之,若初始时 SG(x) == 0,那么先手败,反之先手胜。
但是呢,因为题目是有 n 堆石子,所以各堆的 sg 值应该看成独立的,此时就可以将 n 个 sg 值抽象成是一个 Nim游戏,对这 n 个 sg 值求 xor,若 xor == 0,先手败,反之先手胜。
这里简单类比一下:
xor == 0,没石子可取,先手败;
xor == 0,有石子可取,先手取后,xor 必定不为 0,换句话说一定有一堆石子的 sg 值不为 0,那么后手一定可以在操作后把这一堆石子的 sg 值变为 0,那么 sg == 0的局面一定是被先手遇到,换句话说先手必败;
xor != 0,类比前面可证先手必胜。
代码
#include 例题1、AcWing 894. 拆分-Nim游戏
原题链接:https://www.acwing.com/problem/content/896/
思路
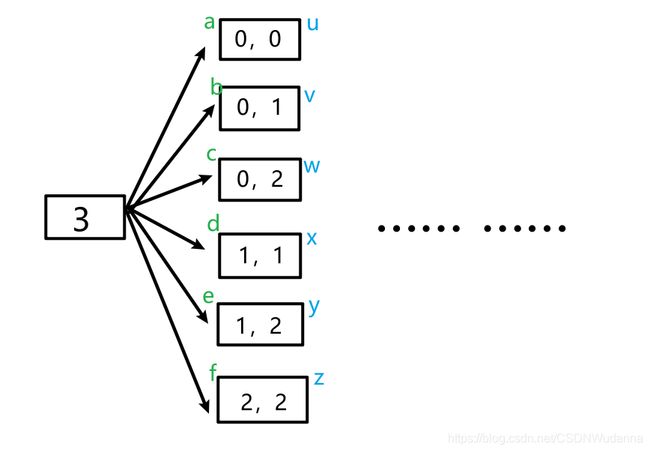
假设现在有 n 堆石头,其中有一堆石头数量只有 3 块,玩家如果选择了这一堆,需要将 3 块石头全部拿走,并且一共有 6 种可能的放回方式,其中绿色表示左边的 sg 值,蓝色表示右边的 sg 值。
那么很显然,这 6 种方式其实和上面 sg 函数的模板题一样,是 3 的可能分支,sg (3) = mex { 6 种取法的 sg 值 }。
但问题就在于,每一种取法的 sg 值怎么求?应该怎么处理一堆变两堆?
这里我们可以类比一下,在模板题里面,我们是求出每一堆的 sg 值,然后对所有堆的 sg 值求异或值判断与否。而这里的取一堆放回两堆,其实可以抽象成:现在有 2 堆石子,操作后先手是必胜还是必败。故而我们就把取完一堆放回两堆的操作看成一个子问题去求解。
综上,sg (3) = mex { sg(0) ^ sg(0),sg(0) ^ sg(1),sg(0) ^ sg(2),sg(1) ^ sg(1),sg(1) ^ sg(2),sg(2) ^ sg(2) }
代码
#include 五、常用结论
1、求两个数不能组合成的最大整数


思路
首先这是一个结论题,假如数据有解,那么不能组合成的最大数目是(n - 1)*(m - 1) - 1。
有无解的分析:
设 a、b、x、y为整数,可正可负。
① 数据无解
如果 gcd(n,m)= d > 1,那么a * n + b * m = x * d,那么由 n 和 m 能凑成的数一定只能是 d 的整数倍,非整数倍的数可以无限大,所以此时的 n 和 m 无解。
② 数据有解
假如 gcd(n,m)= d == 1,那么根据裴蜀定理( gcd(n,m)= d,则必然存在 xn + ym = d),必然可以有以下变换:
xn + ym = 1
xpn + ypm = p
(xp - am)n + (yp + bn)m = p
而 xp - am 和 yp + bn 可以使得让一个大的数减少一些,一个小的数变大一些,使得系数为正,进而可得数据有解。
结论的证明: https://www.acwing.com/solution/acwing/content/3165/
代码
import java.util.Arrays;
import java.util.Comparator;
import java.util.Scanner;
public class Main {
public static void main(String[] args){
Scanner sc = new Scanner(System.in);
int n = sc.nextInt();
int m = sc.nextInt();
System.out.println(((n - 1) * (m - 1) - 1));
}
}
——————————————————————————
2021.03.22
陆陆续续学完一些 ACM 数学入门,主要是简单数论、组合、高精度、简单博弈论,后面如果有时间会接着补充。(现文 4.7 万字)
2021.05.03
增加一些常用结论
2021.07.20
补充(拓展)中国剩余定理