- 【数据结构和算法实践-树-LeetCode110-平衡二叉树】
NeVeRMoRE_2024
数据结构与算法实践算法数据结构leetcodeb树
数据结构和算法实践-树-LeetCode110-平衡二叉树题目MyThought代码示例JAVA-8题目给定一个二叉树,判断它是否是平衡二叉树输入:root=[3,9,20,null,null,15,7]输出:trueMyThought判断平衡二叉树的条件是树的左右高度相差为1一、利用递归去遍历1、边界为节点为null,树高为0;2、树高的递增规则为,根的左节点和右节点比较值+1二、为了方便信息传
- 浅谈一下B树
AIGC Ball
b树
B树(平衡二叉树)是一种自平衡的二叉查找树,它允许搜索、顺序访问、插入和删除操作在对数时间内完成。B树的关键特性是它可以保持所有叶子节点在同一层,这使得它非常适合用于数据库和文件系统中的索引结构。B树的基本概念节点:B树的每个节点可以包含一个键值对和两个子节点的指针,除了根节点和叶子节点。根节点至少含有一个键,叶子节点包含n个键和n+1个子节点指针(n>1)。键:B树中的键是用于排序和查找的值,每
- LeetCode刷题2
Reus_try
leetcode链表算法
0612LeetCode刷题2力扣刷题1力扣刷题2力扣83题:删除排序链表中的重复元素力扣82题:删除排序链表中的重复元素II力扣第8题:字符串转换整数(atoi)力扣22题:括号生成力扣31题:下一个排列怎么用sort()对一个数组的局部进行排序?1143.最长公共子序列力扣93题:复原IP地址力扣151题:颠倒字符串中的单词力扣105题:从前序与中序遍历序列构造二叉树力扣110题:平衡二叉树力
- java将json字符串转换成对象,看这篇足矣了!
imtokenmax合约众筹
程序员面试经验分享java
20个二叉树面试高频0.几个概念1.求二叉树中的节点个数2.求二叉树的最大层数(最大深度)3.先序遍历/前序遍历4.中序遍历5.后序遍历6.分层遍历7.求二叉树第K层的节点个数8.求二叉树第K层的叶子节点个数9.判断两棵二叉树是否结构相同10.判断二叉树是不是平衡二叉树11.求二叉树的镜像12.求二叉树中两个节点的最低公共祖先节点13.求二叉树的直径14.由前序遍历序列和中序遍历序列重建二叉树15
- VAD 虚拟内存
0xwangliang
Windowswindows内核安全
Windows中的虚拟地址分配使用指令dt_EPROCESS874ed030观察EPROCESS结构体偏移为0x278的地方,这个地方就是系统拿来存放每个进程的虚拟地址空间的分配情况输入!vad874ed030+278查看该平衡二叉树Level是二叉树的层数start是该块虚拟地址空间的起始地址end为结束地址commit为请求次数写一段程序测试下#include#includeintmain()
- Java算法之判断平衡二叉树
持续输出...
#Java算法算法
判断一棵二叉树是否是平衡二叉树(即AVL树)是一个常见的问题。平衡二叉树的定义是:对于树中的每个节点,其左右子树的高度差不超过1。我们可以通过递归的方法来判断一棵二叉树是否是平衡的packagecom.huawei.od.huawei.algorithm;/***@ClassName:IsBalancedBinaryTree是否是平衡二叉树*@Desc:判断一棵二叉树是否是平衡二叉树(即AVL树)
- github源码指引:共享内存、数据结构与算法:平衡二叉树set带有互斥接口的
初级代码游戏
github源码指引共享内存数据结构与算法github哈希算法算法共享内存
初级代码游戏的专栏介绍与文章目录-CSDN博客我的github:codetoys,所有代码都将会位于ctfc库中。已经放入库中我会指出在库中的位置。这些代码大部分以Linux为目标但部分代码是纯C++的,可以在任何平台上使用。目录一、演示代码二、互斥层的实现2.1简单的互斥层实现2.2完整互斥接口的实现2.2.1互斥对象放在哪里2.2.2迭代器的互斥2.2.3方法的互斥三、互斥层的设计思想一、演示
- github源码指引:共享内存、数据结构与算法:平衡二叉树set的lower_bound
初级代码游戏
github源码指引共享内存数据结构与算法github哈希算法算法
初级代码游戏的专栏介绍与文章目录-CSDN博客我的github:codetoys,所有代码都将会位于ctfc库中。已经放入库中我会指出在库中的位置。这些代码大部分以Linux为目标但部分代码是纯C++的,可以在任何平台上使用。本篇专门讲解lower_bound的实现。目录一、STL的lower_bound和upper_bound是什么二、二叉树有没有lower_bound三、演示代码3.1定义数据
- [E二叉树] lc110. 平衡二叉树(dfs+自底向上)
Ypuyu
LeetCode深度优先算法
文章目录1.题目来源2.题目解析1.题目来源链接:110.平衡二叉树题单:链表、二叉树与一般树(前后指针/快慢指针/DFS/BFS/直径/LCA)§2.3自底向上DFS2.题目解析思路:记录每个节点的左右子树的高度,并判断高度差是否大于1即可。二叉树计算高度,可看[E二叉树]lc104.二叉树的最大深度(dfs+自顶向下)注意本题可以剪枝优化。如果有任意两个节点的高度差大于1了,那么说明整个树都不
- 线段树
Cheng Yu
线段树线段树
基础知识1、线段树是二叉树,且必定是平衡二叉树,但不一定是完全二叉树。2、对于区间[L,R],令mid=(L+R)/2,则其左子树为[L,mid],右子树为[mid+1,R],当L==R时,该区间为线段树的叶子,无需继续往下划分。3、线段树虽然不是完全二叉树,但是可以用完全二叉树的方式去构造并存储它,只是最后一层可能存在某些叶子与叶子之间出现“空叶子”,这个无需理会,同样给空叶子按顺序编号,在遍历
- 算法day14|110.平衡二叉树 (优先掌握递归)、 二叉树的所有路径(优先掌握递归)、404.左叶子之和 (优先掌握递归)、222.完全二叉树的节点个数(优先掌握递归)
桃酥403
算法数据结构c++leetcode
算法day14|110.平衡二叉树(优先掌握递归)、二叉树的所有路径(优先掌握递归)、404.左叶子之和(优先掌握递归)、222.完全二叉树的节点个数(优先掌握递归)110.平衡二叉树(优先掌握递归)257.二叉树的所有路径(优先掌握递归)404.左叶子之和(优先掌握递归)222.完全二叉树的节点个数(优先掌握递归)110.平衡二叉树(优先掌握递归)我用我原来的思路去做,发现运行不了,目前也没有找
- 浅谈数据结构之树(一)
24K不怕
数据结构树二叉树数据结构算法
浅谈数据结构之树(一)基本概念二叉树斜树满二叉树完全二叉树平衡二叉树红黑树B+树基本概念链表、栈和队列都是一对一的线性结构,树是一对多的线性结构。「一对多」就是指一个元素只能有一个前驱,但可以有多个后继。结点拥有的子树数被称为结点的度(Degree)。度为0的结点称为叶节点(Leaf)或终端结点,度不为0的结点称为分支结点。除根结点外,分支结点也被称为内部结点。结点的子树的根称为该结点的孩子(Ch
- AVL平衡二叉树
qq_187352634
C++算法数据结构平衡二叉树
AVL平衡二叉树定义平衡因子调整类型右右型左左型右左型左右型代码定义单个节点是AVL树左右子树高差差不大于1左右子树都是AVL树平衡因子左子树高度减去右子树高度如果平衡因子绝对值超过1,就必须调整。调整类型找到引起失衡的节点计算平衡因子右右型平衡因子为负则是右X型失衡结点右孩子的平衡因子为负则是右右型调整方法是整体左旋,与左左型调整类似左左型失衡结点的平衡因子为正则是左X型失衡结点左孩子的平衡因子
- 《数据结构》复试问答题总结
CarmenHu
计算机复试问答题数据结构深度优先算法
请简述深度优先遍历、广度优先遍历的基本思想?:深度遍历是在图中先选择一个顶点,随后的每次遍历中选择与顶点相邻并且还没有遍历过的结点进行遍历,类似于树的先序遍历广度遍历是先在图中选择一个顶点,并加入队列中,然后向该顶点的所有未访问过的邻接点进行扩散,加入到队列当中,类似于树的广度遍历简述二叉树,完全二叉树,二叉排序树,平衡二叉树的特性:二叉树(BinaryTree):要求其任意节点的子节点数量不超过
- 代码随想录算法训练营第17天|110.平衡二叉树 |257. 二叉树的所有路径 | 404.左叶子之和
阿豪只会阿巴
算法c++
代码随想录算法训练营第17天|110.平衡二叉树|257.二叉树的所有路径|404.左叶子之和详细布置迭代法,大家可以直接过,二刷有精力的时候再去掌握迭代法。110.平衡二叉树(优先掌握递归)再一次涉及到,什么是高度,什么是深度,可以巩固一下。题目链接/文章讲解/视频讲解:https://programmercarl.com/0110.%E5%B9%B3%E8%A1%A1%E4%BA%8C%E5%
- 代码随想录算法训练营第17天 | 110.平衡二叉树 257.二叉树的所有路径 404.左叶子之和
2301_76612880
数据结构
Leetcode-110平衡二叉树:比较高度优先考虑后序遍历,用后序遍历的递归方式解决很简单:/***Definitionforabinarytreenode.*structTreeNode{*intval;*TreeNode*left;*TreeNode*right;*TreeNode():val(0),left(nullptr),right(nullptr){}*TreeNode(intx):
- Day17|Leetcode 110. 平衡二叉树 Leetcode 257. 二叉树的所有路径 Leetcode 404. 左叶子之和
吼吼848
leetcode算法职场和发展
一刷还是以递归法为主,下面的题目都是默认递归法Leetcode110平衡二叉树题目链接110平衡二叉树本题目和二叉树的最大深度差不多,但是还是要注意几个点的:思路:如果我们的左右子树不是平衡二叉树了,那么整个二叉树就不是平衡二叉树,所以我们就不记录高度,用-1来返回给父节点,不停返回,直到根节点,除了这种判断就是题目中给的判断标准了,一个二叉树每个节点的左右两个子树的高度差的绝对值不超过1,超过一
- 代码随想录 10.09 || 二叉树 LeetCode 110.平衡二叉树、257.二叉树的所有路径、404. 左叶子之和
鱼Sun
算法leetcode数据结构
110.平衡二叉树给定一个二叉树,判断它是否是高度平衡的二叉树。本题中,一课高度平衡的二叉树定义为:一个二叉树每个节点的左右两个子树的高度差的绝对值不超过1。在开始题目之前,需要明确二叉树的深度和高度,是两种不同的概念。二叉树的深度,指根节点到当前节点的最长路径;二叉树的高度,指当前节点到叶子节点的最长路径。所以,在104.二叉树的最大深度中,我们通过求根节点的高度,进而得到二叉树的最大深度,因为
- Day17 | leetcode110. 平衡二叉树、leetcode257. 二叉树的所有路径、leetcode404. 左叶子之和
Rick_2021
算法编程题算法数据结构
Day17leetcode110.平衡二叉树leetcode257.二叉树的所有路径leetcode404.左叶子之和leetcode110.平衡二叉树再强调一波概念:二叉树节点的深度:指从根节点到该节点的最长简单路径边的条数。二叉树节点的高度:指从该节点到叶子节点的最长简单路径边的条数。求深度可以从上到下去查所以需要前序遍历(中左右)而高度只能从下到上去查,所以只能后序遍历(左右中)为什么104
- 算法训练Day17|二叉树part04(LeetCode 110.平衡二叉树、257.二叉树的所有路径、404.左叶子之和)
3分16秒
算法与数据结构算法leetcodec++数据结构
文章目录110.平衡二叉树257.二叉树的所有路径404.左叶子之和递归法迭代法110.平衡二叉树题目链接给定一个二叉树,判断它是否是高度平衡的二叉树。本题中,一棵高度平衡二叉树定义为:一个二叉树每个节点的左右两个子树的高度差的绝对值不超过1。根节点的高度就是这棵树的最大深度。求深度可以从上到下去查所以需要前序遍历(中左右),而高度只能从下到上去查,所以只能后序遍历(左右中)这里看不懂可以看二叉树
- 代码随想录训练营第17天|LeetCode 110.平衡二叉树、257.二叉树的所有路径、404.左叶子之和
忆昔z
代码随想录训练营leetcode算法数据结构
参考代码随想录题目一:LeetCode110.平衡二叉树递归法确定递归函数的参数和返回值:参数为根节点,返回值是以当前传入节点为根节点的树的高度。intgetHeight(TreeNode*root);确定终止条件:当传入的节点的为空时,返回0表示节点高度为0if(root==nullptr)return0;确定单层递归逻辑:单层逻辑里肯定是求左子树高度和右子树高度的高度差,如果高度差大于1,说明
- leetcode 110. 平衡二叉树、257. 二叉树的所有路径、257. 二叉树的所有路径
叫我卡卡西cc
leetcode算法职场和发展c++
110.平衡二叉树给定一个二叉树,判断它是否是高度平衡的二叉树。本题中,一棵高度平衡二叉树定义为:一个二叉树每个节点的左右两个子树的高度差的绝对值不超过1。示例1:输入:root=[3,9,20,null,null,15,7]输出:true示例2:输入:root=[1,2,2,3,3,null,null,4,4]输出:false示例3:输入:root=[]输出:true思路:左右子树的高度差大于1
- 第15天|LeetCode110.平衡二叉树 、LeetCode257. 二叉树的所有路径、LeetCode404.左叶子之和
小宇刷题
算法数据结构java深度优先
1.题目链接:110.平衡二叉树题目描述:给定一个二叉树,判断它是否是高度平衡的二叉树。本题中,一棵高度平衡二叉树定义为一个二叉树每个节点的左右两个子树的高度差的绝对值不超过1。解法:用递归来做,三步曲:①因为我要用-1来表示该数是否是平衡二叉树,故返回-1就不是平衡二叉树,返回不是-1就是平衡二叉树,故返回值为int型(表示的是高度);参数就root。②终止条件---if(root==null)
- Day17|leetcode 110.平衡二叉树、257.二叉树的所有路径、404.左叶子之和
只当小白脸
leetcode数据结构算法c++
leetcode110.平衡二叉树题目链接:110.平衡二叉树-力扣(LeetCode)视频链接:后序遍历求高度,高度判断是否平衡|LeetCode:110.平衡二叉树_哔哩哔哩_bilibili思路平衡二叉树定义为:一个二叉树每个节点的左右两个子树的高度差的绝对值不超过1。本题就是求左右子树的高度差,如果差值left);if(leftHeight==-1)return-1;intrightHei
- 代码随想录算法训练营第十七天| LeetCode110.平衡二叉树、LeetCode257. 二叉树的所有路径、LeetCode404.左叶子之和
wIridescent-
算法
#LeetCode110.BalancedBinaryTree#LeetCode110.视频讲解:后序遍历求高度,高度判断是否平衡|LeetCode:110.平衡二叉树_哔哩哔哩_bilibili平衡二叉树的定义是:二叉树的每个节点的左右子树的高度之差不超过1.如果计算二叉树的高度用后序遍历,二叉树的深度用前序遍历,原因如下:二叉树的高度:叶子节点所在的层为1,所以是汇总左右孩子情况后+1,返回给
- 代码随想录算法训练营(23/5/25)二叉树,LeetCode 110.平衡二叉树,LeetCode.257二叉树的所有的路径,LeetCode 4044.左子树之和
Y加油
算法leetcode职场和发展
LeetCode110.平衡二叉树这道题是判断高度平衡的二叉树,深度可以从上到下去查,所以需要前序遍历,而高度是只能从到下到上查,只能是后序遍历,要多看看题目,理解透题目的意思LeetCode257二叉树的所有的路径我不是能理解回溯,这道题半懂LeetCode404.左子树之和判断左节点是最重要的
- 算法学习|Day17-二叉树|Leetcode110.平衡二叉树,Leetcode257. 二叉树的所有路径,Leetcode404.左叶子之和
ambitious_Rgr
算法python数据结构leetcode广度优先深度优先学习
目录一、Leetcode110.平衡二叉树题目描述解题思路方法:递归总结二、Leetcode257.二叉树的所有路径题目描述解题思路方法:递归总结三、Leetcode404.左叶子之和题目描述解题思路方法一:递归方法二:层序遍历总结一、Leetcode110.平衡二叉树题目描述给定一个二叉树,判断它是否是高度平衡的二叉树。本题中,一棵高度平衡二叉树定义为:一个二叉树每个节点的左右两个子树的高度差的
- 代码随想录算法训练营第17天|110.平衡二叉树 ● 257. 二叉树的所有路径 ● 404.左叶子之和
strive340
算法数据结构
110.平衡二叉树为什么很多二叉树的题目都用后序遍历?因为左右中,可以把处理该节点放到最后,例如这题,只有得出了左右子树的高度,才能知道以该节点为根的子树是否为平衡二叉树。思路:后序遍历,计算左右子树高度差,若有一颗子树不是平衡二叉树则直接返回-1(一颗子树不平衡,则整颗子树都不平衡),否则是平衡二叉树,返回左右子树最大的一个高度加上根节点的高度。代码:intgethight(TreeNode*n
- 【LeetCode】110. 平衡二叉树(简单)——代码随想录算法训练营Day17
晴雪月乔
代码随想录算法训练营#LeetCode二叉树算法代码随想录算法训练营二叉树
题目链接:110.平衡二叉树题目描述给定一个二叉树,判断它是否是高度平衡的二叉树。本题中,一棵高度平衡二叉树定义为:一个二叉树每个节点的左右两个子树的高度差的绝对值不超过1。示例1:输入:root=[3,9,20,null,null,15,7]输出:true示例2:输入:root=[1,2,2,3,3,null,null,4,4]输出:false示例3:输入:root=[]输出:true提示:树中
- 代码随想录算法训练营day17
羊角问蕊
算法
题目:110.平衡二叉树、257.二叉树的所有路径、404.左叶子之和参考链接:代码随想录110.平衡二叉树思路:首先要搞清楚高度和深度的区别,深度是从上往下数,应该使用前序遍历的思路,而高度是从下往上数,只能后序遍历,力扣上的深度都是从1开始的。本题首先写一个计算高度的函数,然后对比左右子树的高度即可。对于求高度的函数,之前求最大高度已经写过,此处的区别就是当左右不平衡的时候直接返回-1。时间复
- mongodb3.03开启认证
21jhf
mongodb
下载了最新mongodb3.03版本,当使用--auth 参数命令行开启mongodb用户认证时遇到很多问题,现总结如下:
(百度上搜到的基本都是老版本的,看到db.addUser的就是,请忽略)
Windows下我做了一个bat文件,用来启动mongodb,命令行如下:
mongod --dbpath db\data --port 27017 --directoryperdb --logp
- 【Spark103】Task not serializable
bit1129
Serializable
Task not serializable是Spark开发过程最令人头疼的问题之一,这里记录下出现这个问题的两个实例,一个是自己遇到的,另一个是stackoverflow上看到。等有时间了再仔细探究出现Task not serialiazable的各种原因以及出现问题后如何快速定位问题的所在,至少目前阶段碰到此类问题,没有什么章法
1.
package spark.exampl
- 你所熟知的 LRU(最近最少使用)
dalan_123
java
关于LRU这个名词在很多地方或听说,或使用,接下来看下lru缓存回收的实现
1、大体的想法
a、查询出最近最晚使用的项
b、给最近的使用的项做标记
通过使用链表就可以完成这两个操作,关于最近最少使用的项只需要返回链表的尾部;标记最近使用的项,只需要将该项移除并放置到头部,那么难点就出现 你如何能够快速在链表定位对应的该项?
这时候多
- Javascript 跨域
周凡杨
JavaScriptjsonp跨域cross-domain
- linux下安装apache服务器
g21121
apache
安装apache
下载windows版本apache,下载地址:http://httpd.apache.org/download.cgi
1.windows下安装apache
Windows下安装apache比较简单,注意选择路径和端口即可,这里就不再赘述了。 2.linux下安装apache:
下载之后上传到linux的相关目录,这里指定为/home/apach
- FineReport的JS编辑框和URL地址栏语法简介
老A不折腾
finereportweb报表报表软件语法总结
JS编辑框:
1.FineReport的js。
作为一款BS产品,browser端的JavaScript是必不可少的。
FineReport中的js是已经调用了finereport.js的。
大家知道,预览报表时,报表servlet会将cpt模板转为html,在这个html的head头部中会引入FineReport的js,这个finereport.js中包含了许多内置的fun
- 根据STATUS信息对MySQL进行优化
墙头上一根草
status
mysql 查看当前正在执行的操作,即正在执行的sql语句的方法为:
show processlist 命令
mysql> show global status;可以列出MySQL服务器运行各种状态值,我个人较喜欢的用法是show status like '查询值%';一、慢查询mysql> show variab
- 我的spring学习笔记7-Spring的Bean配置文件给Bean定义别名
aijuans
Spring 3
本文介绍如何给Spring的Bean配置文件的Bean定义别名?
原始的
<bean id="business" class="onlyfun.caterpillar.device.Business">
<property name="writer">
<ref b
- 高性能mysql 之 性能剖析
annan211
性能mysqlmysql 性能剖析剖析
1 定义性能优化
mysql服务器性能,此处定义为 响应时间。
在解释性能优化之前,先来消除一个误解,很多人认为,性能优化就是降低cpu的利用率或者减少对资源的使用。
这是一个陷阱。
资源时用来消耗并用来工作的,所以有时候消耗更多的资源能够加快查询速度,保持cpu忙绿,这是必要的。很多时候发现
编译进了新版本的InnoDB之后,cpu利用率上升的很厉害,这并不
- 主外键和索引唯一性约束
百合不是茶
索引唯一性约束主外键约束联机删除
目标;第一步;创建两张表 用户表和文章表
第二步;发表文章
1,建表;
---用户表 BlogUsers
--userID唯一的
--userName
--pwd
--sex
create
- 线程的调度
bijian1013
java多线程thread线程的调度java多线程
1. Java提供一个线程调度程序来监控程序中启动后进入可运行状态的所有线程。线程调度程序按照线程的优先级决定应调度哪些线程来执行。
2. 多数线程的调度是抢占式的(即我想中断程序运行就中断,不需要和将被中断的程序协商)
a)
- 查看日志常用命令
bijian1013
linux命令unix
一.日志查找方法,可以用通配符查某台主机上的所有服务器grep "关键字" /wls/applogs/custom-*/error.log
二.查看日志常用命令1.grep '关键字' error.log:在error.log中搜索'关键字'2.grep -C10 '关键字' error.log:显示关键字前后10行记录3.grep '关键字' error.l
- 【持久化框架MyBatis3一】MyBatis版HelloWorld
bit1129
helloworld
MyBatis这个系列的文章,主要参考《Java Persistence with MyBatis 3》。
样例数据
本文以MySQL数据库为例,建立一个STUDENTS表,插入两条数据,然后进行单表的增删改查
CREATE TABLE STUDENTS
(
stud_id int(11) NOT NULL AUTO_INCREMENT,
- 【Hadoop十五】Hadoop Counter
bit1129
hadoop
1. 只有Map任务的Map Reduce Job
File System Counters
FILE: Number of bytes read=3629530
FILE: Number of bytes written=98312
FILE: Number of read operations=0
FILE: Number of lar
- 解决Tomcat数据连接池无法释放
ronin47
tomcat 连接池 优化
近段时间,公司的检测中心报表系统(SMC)的开发人员时不时找到我,说用户老是出现无法登录的情况。前些日子因为手头上 有Jboss集群的测试工作,发现用户不能登录时,都是在Tomcat中将这个项目Reload一下就好了,不过只是治标而已,因为大概几个小时之后又会 再次出现无法登录的情况。
今天上午,开发人员小毛又找到我,要我协助将这个问题根治一下,拖太久用户难保不投诉。
简单分析了一
- java-75-二叉树两结点的最低共同父结点
bylijinnan
java
import java.util.LinkedList;
import java.util.List;
import ljn.help.*;
public class BTreeLowestParentOfTwoNodes {
public static void main(String[] args) {
/*
* node data is stored in
- 行业垂直搜索引擎网页抓取项目
carlwu
LuceneNutchHeritrixSolr
公司有一个搜索引擎项目,希望各路高人有空来帮忙指导,谢谢!
这是详细需求:
(1) 通过提供的网站地址(大概100-200个网站),网页抓取程序能不断抓取网页和其它类型的文件(如Excel、PDF、Word、ppt及zip类型),并且程序能够根据事先提供的规则,过滤掉不相干的下载内容。
(2) 程序能够搜索这些抓取的内容,并能对这些抓取文件按照油田名进行分类,然后放到服务器不同的目录中。
- [通讯与服务]在总带宽资源没有大幅增加之前,不适宜大幅度降低资费
comsci
资源
降低通讯服务资费,就意味着有更多的用户进入,就意味着通讯服务提供商要接待和服务更多的用户,在总体运维成本没有由于技术升级而大幅下降的情况下,这种降低资费的行为将导致每个用户的平均带宽不断下降,而享受到的服务质量也在下降,这对用户和服务商都是不利的。。。。。。。。
&nbs
- Java时区转换及时间格式
Cwind
java
本文介绍Java API 中 Date, Calendar, TimeZone和DateFormat的使用,以及不同时区时间相互转化的方法和原理。
问题描述:
向处于不同时区的服务器发请求时需要考虑时区转换的问题。譬如,服务器位于东八区(北京时间,GMT+8:00),而身处东四区的用户想要查询当天的销售记录。则需把东四区的“今天”这个时间范围转换为服务器所在时区的时间范围。
- readonly,只读,不可用
dashuaifu
jsjspdisablereadOnlyreadOnly
readOnly 和 readonly 不同,在做js开发时一定要注意函数大小写和jsp黄线的警告!!!我就经历过这么一件事:
使用readOnly在某些浏览器或同一浏览器不同版本有的可以实现“只读”功能,有的就不行,而且函数readOnly有黄线警告!!!就这样被折磨了不短时间!!!(期间使用过disable函数,但是发现disable函数之后后台接收不到前台的的数据!!!)
- LABjs、RequireJS、SeaJS 介绍
dcj3sjt126com
jsWeb
LABjs 的核心是 LAB(Loading and Blocking):Loading 指异步并行加载,Blocking 是指同步等待执行。LABjs 通过优雅的语法(script 和 wait)实现了这两大特性,核心价值是性能优化。LABjs 是一个文件加载器。RequireJS 和 SeaJS 则是模块加载器,倡导的是一种模块化开发理念,核心价值是让 JavaScript 的模块化开发变得更
- [应用结构]入口脚本
dcj3sjt126com
PHPyii2
入口脚本
入口脚本是应用启动流程中的第一环,一个应用(不管是网页应用还是控制台应用)只有一个入口脚本。终端用户的请求通过入口脚本实例化应用并将将请求转发到应用。
Web 应用的入口脚本必须放在终端用户能够访问的目录下,通常命名为 index.php,也可以使用 Web 服务器能定位到的其他名称。
控制台应用的入口脚本一般在应用根目录下命名为 yii(后缀为.php),该文
- haoop shell命令
eksliang
hadoophadoop shell
cat
chgrp
chmod
chown
copyFromLocal
copyToLocal
cp
du
dus
expunge
get
getmerge
ls
lsr
mkdir
movefromLocal
mv
put
rm
rmr
setrep
stat
tail
test
text
- MultiStateView不同的状态下显示不同的界面
gundumw100
android
只要将指定的view放在该控件里面,可以该view在不同的状态下显示不同的界面,这对ListView很有用,比如加载界面,空白界面,错误界面。而且这些见面由你指定布局,非常灵活。
PS:ListView虽然可以设置一个EmptyView,但使用起来不方便,不灵活,有点累赘。
<com.kennyc.view.MultiStateView xmlns:android=&qu
- jQuery实现页面内锚点平滑跳转
ini
JavaScripthtmljqueryhtml5css
平时我们做导航滚动到内容都是通过锚点来做,刷的一下就直接跳到内容了,没有一丝的滚动效果,而且 url 链接最后会有“小尾巴”,就像#keleyi,今天我就介绍一款 jquery 做的滚动的特效,既可以设置滚动速度,又可以在 url 链接上没有“小尾巴”。
效果体验:http://keleyi.com/keleyi/phtml/jqtexiao/37.htmHTML文件代码:
&
- kafka offset迁移
kane_xie
kafka
在早前的kafka版本中(0.8.0),offset是被存储在zookeeper中的。
到当前版本(0.8.2)为止,kafka同时支持offset存储在zookeeper和offset manager(broker)中。
从官方的说明来看,未来offset的zookeeper存储将会被弃用。因此现有的基于kafka的项目如果今后计划保持更新的话,可以考虑在合适
- android > 搭建 cordova 环境
mft8899
android
1 , 安装 node.js
http://nodejs.org
node -v 查看版本
2, 安装 npm
可以先从 https://github.com/isaacs/npm/tags 下载 源码 解压到
- java封装的比较器,比较是否全相同,获取不同字段名字
qifeifei
非常实用的java比较器,贴上代码:
import java.util.HashSet;
import java.util.List;
import java.util.Set;
import net.sf.json.JSONArray;
import net.sf.json.JSONObject;
import net.sf.json.JsonConfig;
i
- 记录一些函数用法
.Aky.
位运算PHP数据库函数IP
高手们照旧忽略。
想弄个全天朝IP段数据库,找了个今天最新更新的国内所有运营商IP段,copy到文件,用文件函数,字符串函数把玩下。分割出startIp和endIp这样格式写入.txt文件,直接用phpmyadmin导入.csv文件的形式导入。(生命在于折腾,也许你们觉得我傻X,直接下载人家弄好的导入不就可以,做自己的菜鸟,让别人去说吧)
当然用到了ip2long()函数把字符串转为整型数
- sublime text 3 rust
wudixiaotie
Sublime Text
1.sublime text 3 => install package => Rust
2.cd ~/.config/sublime-text-3/Packages
3.mkdir rust
4.git clone https://github.com/sp0/rust-style
5.cd rust-style
6.cargo build --release
7.ctrl
 ,但是维护满叉树,难度是很大的。所以AVL树(平衡树)放宽了条件,允许左右子树的高度差在一定的范围之内,avl树平衡条件是左右子树高度相差不能为2,而不是满叉树左右子树高度相同。AVL是以提出它的两位苏联数学家的名字头字母命名的。一棵N个节点的AVL树,高度最高为1.44logx(N+1)-0.328,比满二叉树高度增加44%。
,但是维护满叉树,难度是很大的。所以AVL树(平衡树)放宽了条件,允许左右子树的高度差在一定的范围之内,avl树平衡条件是左右子树高度相差不能为2,而不是满叉树左右子树高度相同。AVL是以提出它的两位苏联数学家的名字头字母命名的。一棵N个节点的AVL树,高度最高为1.44logx(N+1)-0.328,比满二叉树高度增加44%。 现在不平横了,3节点左子树的高度为-1(空树为-1),右子树为1,相差为2,不平衡,插入在3节点的右子树的右子树,这种情况称为RR,转换为
现在不平横了,3节点左子树的高度为-1(空树为-1),右子树为1,相差为2,不平衡,插入在3节点的右子树的右子树,这种情况称为RR,转换为 ,这样就平衡了。继续插入2和1,情况如下:
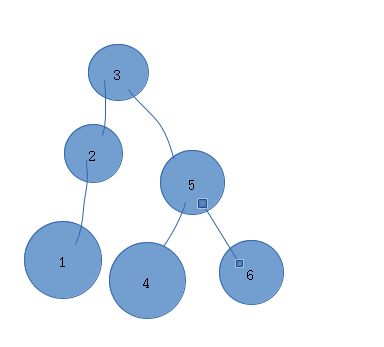
,这样就平衡了。继续插入2和1,情况如下: 节点3失去平衡,1插在3的左子树的左子树,LL情况,需要旋转,如下:
节点3失去平衡,1插在3的左子树的左子树,LL情况,需要旋转,如下: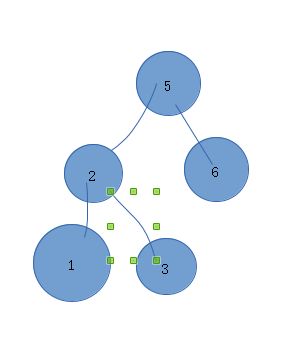 树重新平衡,现在继续插入4,如下:
树重新平衡,现在继续插入4,如下: ,节点5失去平衡,4插在它的左子树的右子树上,这种情况为LR,需要两次旋转,第一次为RR,5的左子树,然后LL5这棵树。变换后如下:
,节点5失去平衡,4插在它的左子树的右子树上,这种情况为LR,需要两次旋转,第一次为RR,5的左子树,然后LL5这棵树。变换后如下: