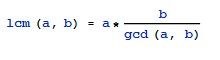- 20个新手学习c++必会的程序 输出*三角形、杨辉三角等(附代码)
X_StarX
c++学习算法大学生开发语言数据结构
示例1:HelloWorld#includeusingnamespacestd;intmain(){coutusingnamespacestd;intmain(){inta=5;intb=10;intsum=a+b;coutusingnamespacestd;intfactorial(intn){if(nusingnamespacestd;voidprintFibonacci(intn){intt
- Day_11
ROC_bird..
算法
面试题16.15.珠玑妙算-力扣(LeetCode)/***Note:Thereturnedarraymustbemalloced,assumecallercallsfree().*///下标和对应位置的值都一样,answer[0]+1,对应位置的值猜对了,但是下标不对,answer[1]+1int*masterMind(char*solution,char*guess,int*returnSiz
- LeetCode 53. Maximum Subarray
枯萎的海风
算法与OJC/C++leetcode
1.题目描述Findthecontiguoussubarraywithinanarray(containingatleastonenumber)whichhasthelargestsum.Forexample,giventhearray[−2,1,−3,4,−1,2,1,−5,4],thecontiguoussubarray[4,−1,2,1]hasthelargestsum=6.clicktos
- leetcode-124 Binary Tree Maximum Path Sum
乐观的大鹏
LeetCode
Givenanon-emptybinarytree,findthemaximumpathsum.Forthisproblem,apathisdefinedasanysequenceofnodesfromsomestartingnodetoanynodeinthetreealongtheparent-childconnections.Thepathmustcontainatleastonenodea
- 【LeetCode】53. Maximum Subarray
墨染百城
LeetCodeleetcode
问题描述问题链接:https://leetcode.com/problems/maximum-subarray/#/descriptionFindthecontiguoussubarraywithinanarray(containingatleastonenumber)whichhasthelargestsum.Forexample,giventhearray[-2,1,-3,4,-1,2,1,-
- Numpy 学习
沐辰老爹
创建指定数值的数组a=np.full((3,5),np.pi)高级通用函数的特性#1.指定输出x=np.arange(10)y=np.empty(10)np.multiply(x,10,out=y)#2.聚合x=np.arange(10)x_sum=np.add.reduce(x)#类似的可用于logical_and等np.logical_and([condition1,condition2,co
- python字符串相等怎么表示_python怎样判断字符串相等
weixin_39993989
python字符串相等怎么表示
python字符串如何判断相等1.is来判断groupName=params['groupName']##groupName的值是'url'reqBody['dim']=groupNameprint("reqBody_dim-SummaryListHandler",reqBody['dim'])##('reqBody_dim-SummaryListHandler',u'url')print("re
- 跟着黑马学mysql(5)
小杜不吃糖
mysql数据库
17.DQL-聚合函数DQL-聚合函数介绍将一列数据作为一个整体,进行纵向计算。常见聚合函数函数功能count统计数量max最大值min最小值avg平均值sum求和语法SELECT聚合函数(字段列表)FROM表名;注意:所有的null值不参与聚合函数的运算18.DQL-分组查询语法SELECT字段列表FROM表名[WHERE条件]GROUPBY分组字段名[HAVING分组后的过滤条件];where
- 蓝桥杯18小白第5题
@liu666
蓝桥杯算法职场和发展
思维,#includeusingnamespacestd;#defineintlonglongconstintn=1e6+11;inta,b[n],c[n],d,k[n];structs{intx,y,z;}q[n];boolcmp(sa1,sa2){returna1.z>a;for(inti=1;i>q[i].x;}for(inti=1;i>q[i].y;q[i].z=q[i].x+q[i].y
- 致良知之寄诸用明书
BonSun
众所周知,当今社会,父母和社会、学校对学生的期望往往是唯分数论,包括每个人对成功的理解也往往是功名利禄,忽视了最基本的学问。文中提到,花之千叶者无实,为其华美太发露耳。人只有沉下心来,韬光养晦,才能拥有真正的学问和本领。
- mysql查询统计聚合函数
三小皮
mysql数据库
业务中用户统计报表使用,查询字段使用聚合函数+条件,快速实现报表统计。SELECTMIN(s.org_name)ASorgName,s.way_nameASwayName,COUNT(s.id)ASwaybillTotal,SUM(s.take_weight)AStakeWeightTotal,SUM(s.revert_weight)ASrevertWeightTotal,SUM(s.settle
- 10/24 每周学习总结5
木木ainiks
1024程序员节
1RecordingtheMoolympicsS#include#includeusingnamespacestd;typedeflonglongint_1;intn;structnode{int_1begin_b;int_1end_e;}a[300];boolcmp(nodea,nodeb){if(a.end_e==b.end_e)returna.begin_b>b.begin_b;return
- 【数据结构和算法实践-树-LeetCode113-路径总和Ⅱ】
NeVeRMoRE_2024
数据结构与算法实践数据结构算法leetcodeb树
数据结构和算法实践-树-LeetCode113-路径总和Ⅱ题目MyThought代码示例JAVA-8题目给你二叉树的根节点root和一个整数目标和targetSum,找出所有从根节点到叶子节点路径总和等于给定目标和的路径。叶子节点是指没有子节点的节点输入:root=[5,4,8,11,null,13,4,7,2,null,null,5,1],targetSum=22输出:[[5,4,11,2],[
- C语言学习 - continue跳转语句
Hyso
continue跳转语句的使用用于for循环语句、while循环语句、dowhile循环语句中,跳过本次循环中剩余的语句而执行下一次循环。continue跳转语句的实例:#includeintmain(coid){intsum=0;inti=1;while(i<=100){sum+=i;i++;if(i==50){continue;}printf("i=%d\n",i);}printf("sum=
- Python 报错 ImportError: cannot import name xxx from partially initialized module xxx
SmallerFL
其他问题fixPython相关python深度学习pytorch人工智能
文章目录1.报错2.原因3.参考1.报错ImportError:cannotimportname'SummaryWriter'frompartiallyinitializedmodule'torch.utils.tensorboard'(mostlikelyduetoacircularimport)(/Library/Frameworks/Python.framework/Versions/3.1
- 02 Java-Lambda-Java 8 自带的函数接口
王小杰at2019
Java8自带的函数接口我们使用lambda在处理自己定义的业务时,需要自定义函数式接口,其实java8已经内置了常用的接口,这样我们在用的时候不要需要自己定义接口,根据需要选择符合自己业务逻辑的接口接口|输入参数|返回值类型|说明---|---|---|---|---Predicate|T|boolean|断言Consumer|T|/|消费一个数据|Function|T|R|输入一个T输出一个R
- 【Android 设备上的所有相关 WiFi 命令和使用方法】
开心呆哥
测试工程师My个人工具栏技术android
请注意,这些命令的可用性可能会因设备型号和Android版本的不同而有所变化。1.adbshellcmdwifi命令1.1查看WiFi状态命令:adbshellcmdwifistatus功能:查看WiFi的当前状态,如是否开启、连接的网络等。示例:adbshellcmdwifistatus1.2执行WiFi扫描命令:adbshellcmdwifiscan功能:执行WiFi扫描以查找附近的网络。示例
- 1.forEach
Night_LION
varcolors=["red","blue","green"];//ES5遍历数组方法for(vari=0;i//console.log(colors[i]);}//ES6forEachcolors.forEach(function(color){//console.log(color);});//练习:遍历数组中的值,并计算总和varnumbers=[1,2,3,4,5];varsum=0;f
- 七月宁乡诗花九朵
周柯楠
七月宁乡诗花九朵NinePoeticFlowersinJulyatNingxiang中文|白鹤清泉(BaiheQingquan)英译|周柯楠(ZhouKenan)1)萤火虫Firefly夏天的夜晚你们都提着一盏灯照亮自己无视黑暗OnsummernightsYouallcarryalampToilluminateyourselfandignorethedarkness我的眼睛跟着你们起舞东瞧瞧西逛逛
- 分布式消息队列Kafka
叶域
大数据分布式kafkascalaspark
分布式消息队列Kafka简介:Kafka是一个分布式消息队列系统,用于处理实时数据流。消息按照主题(Topic)进行分类存储,发送消息的实体称为Producer,接收消息的实体称为Consumer。Kafka集群由多个Kafka实例(Server)组成,每个实例称为Broker。主要用途:广泛应用于构建实时数据管道和流应用程序,适用于需要高吞吐量和低延迟的数据处理场景依赖:Kafka集群和消费者依
- RocketMQ 架构简析,看这篇就够了!
V搜编程进阶路
Java程序员java-rocketmqrocketmq架构
生产者组(ProducerGroup)同一类Producer的集合,这类Producer发送同一类消息且发送逻辑一致。如果发送的是事务消息且原始生产者在发送之后崩溃,则Broker服务器会联系同一生产者组的其他生产者实例以提交或回溯消费。消费者组(ConsumerGroup)同一类Consumer的集合,这类Consumer通常消费同一类消息且消费逻辑一致。消费者组使得在消息消费方面,实现负载均衡
- 网易云热评一一终会找到自己。
将倾_
1.“感情路上顺利吗?”“挺顺利的,都没有什么人。”一一网易云热评《朋友关系》2.我的梦想除了赚很多很多的钱还想和你在盛夏的晚上穿着拖鞋在街上晃荡渴了就喝瓶啤酒醉了就去山顶吹吹风困了就靠在你的肩头一一网易云热评《summerfriend》3.爱尔兰结婚不许离婚,但是可以选择年限1到100年,过期不续期就相当于自动离了。但是时间越短费用越高,1年的登记费折合人民币2万多,100年的只要6元钱。选择结
- 安装livox雷达驱动
一九零零1900
自动驾驶人工智能ubuntulinux
一、准备工作先要确保ROS和Livox-SDK安装成功!安装过程可参考:ROS安装:ubuntu20.04的ros2系统安装_一九零零1900的博客-CSDN博客二、Livox-SDK安装1.安装依赖包CMAKEsudoaptinstallcmake2.下载Livox-SDK文件gitclonehttps://github.com/Livox-SDK/Livox-SDK.git如果运行太慢,可以直
- JavaSE项目--图书管理系统代码
码来码去(未来可期)
Java学习java
结构代码展示Book类代码packagecom.xszx.beans;//实体类publicclassBook{privateintid;privateStringname;privateintbsum;publicBook(){}publicBook(intid,Stringname,intbsum){this.id=id;this.name=name;this.bsum=bsum;}publi
- LeetCode:2398. 预算内的最多机器人数目 双指针+单调队列,时间复杂度O(n)
忍界英雄
每日一题leetcode机器人算法
2398.预算内的最多机器人数目today2398.预算内的最多机器人数目题目描述你有n个机器人,给你两个下标从0开始的整数数组chargeTimes和runningCosts,两者长度都为n。第i个机器人充电时间为chargeTimes[i]单位时间,花费runningCosts[i]单位时间运行。再给你一个整数budget。运行k个机器人总开销是max(chargeTimes)+k*sum(r
- 【HDFS】【HDFS架构】【HDFS Architecture】【架构】
资源存储库
hdfs架构hadoop
目录1Introduction介绍2AssumptionsandGoals假设和目标HardwareFailure硬件故障StreamingDataAccess流式数据访问LargeDataSets大型数据集SimpleCoherencyModel简单凝聚力模型“MovingComputationisCheaperthanMovingData”“移动计算比移动数据更便宜”PortabilityAc
- python语言程序设计教程-Python语言程序设计(视频教程)
weixin_37988176
——为什么要学习计算机编程?——因为编程是件很有趣的事儿,能启迪思维,还有诗和远方...Python语言,由GuidovanRossum大牛在1990年发明,它是当今世界最受欢迎的计算机编程语言,也是一门对大多数人“学了能用、学了有用、学会能久用”的计算生态语言。本课程是一门体现大学水平的Python语言入门课程,采用“理解和运用计算生态”为教学理念,面向Python零基础学习者,不要求学习者有任
- Python【math数学函数】
Alan_Lowe
#Pythonpython
Python【math数学函数】文章目录Python【math数学函数】数论与表示函数1.ceil()和floor()2.comb()3.copysign()4.fabs()5.factorial()6.gcd()7.lcm()幂函数与对数函数1.exp()和math.e和pow()2.log()和log2()和log10()3.sqrt(x)三角函数1.asin、acos()、atan()2.s
- Python convtools 展示:流行聚合
Nikita Almakov
python后端低代码
今天,我想演示一下convtools如何帮助你避免重复实施流行的聚合。将对象序列转换为字典,其中的值按和计算fromconvtoolsimportconversionascfunc=c.aggregate(c.ReduceFuncs.DictSum(c.item("a"),c.item("b"))).gen_converter()data=[{"a":1,"b":10},{"a":2,"b":11
- python实现leetcode之40. 组合总和 II
深圳都这么冷
解题思路先将candidates排序,数组很短,排序很快然后看最小的元素candidates[0]如果最小的元素大于等于target,就可以停止递归了否则,组合包含两种情况1.有第一项first,然后才是rest的组合2.没有第一项,都是rest的组合40.组合总和II代码cache={}classSolution:defcombinationSum2(self,candidates:List[i
- java短路运算符和逻辑运算符的区别
3213213333332132
java基础
/*
* 逻辑运算符——不论是什么条件都要执行左右两边代码
* 短路运算符——我认为在底层就是利用物理电路的“并联”和“串联”实现的
* 原理很简单,并联电路代表短路或(||),串联电路代表短路与(&&)。
*
* 并联电路两个开关只要有一个开关闭合,电路就会通。
* 类似于短路或(||),只要有其中一个为true(开关闭合)是
- Java异常那些不得不说的事
白糖_
javaexception
一、在finally块中做数据回收操作
比如数据库连接都是很宝贵的,所以最好在finally中关闭连接。
JDBCAgent jdbc = new JDBCAgent();
try{
jdbc.excute("select * from ctp_log");
}catch(SQLException e){
...
}finally{
jdbc.close();
- utf-8与utf-8(无BOM)的区别
dcj3sjt126com
PHP
BOM——Byte Order Mark,就是字节序标记 在UCS 编码中有一个叫做"ZERO WIDTH NO-BREAK SPACE"的字符,它的编码是FEFF。而FFFE在UCS中是不存在的字符,所以不应该出现在实际传输中。UCS规范建议我们在传输字节流前,先传输 字符"ZERO WIDTH NO-BREAK SPACE"。这样如
- JAVA Annotation之定义篇
周凡杨
java注解annotation入门注释
Annotation: 译为注释或注解
An annotation, in the Java computer programming language, is a form of syntactic metadata that can be added to Java source code. Classes, methods, variables, pa
- tomcat的多域名、虚拟主机配置
g21121
tomcat
众所周知apache可以配置多域名和虚拟主机,而且配置起来比较简单,但是项目用到的是tomcat,配来配去总是不成功。查了些资料才总算可以,下面就跟大家分享下经验。
很多朋友搜索的内容基本是告诉我们这么配置:
在Engine标签下增面积Host标签,如下:
<Host name="www.site1.com" appBase="webapps"
- Linux SSH 错误解析(Capistrano 的cap 访问错误 Permission )
510888780
linuxcapistrano
1.ssh -v
[email protected] 出现
Permission denied (publickey,gssapi-keyex,gssapi-with-mic,password).
错误
运行状况如下:
OpenSSH_5.3p1, OpenSSL 1.0.1e-fips 11 Feb 2013
debug1: Reading configuratio
- log4j的用法
Harry642
javalog4j
一、前言: log4j 是一个开放源码项目,是广泛使用的以Java编写的日志记录包。由于log4j出色的表现, 当时在log4j完成时,log4j开发组织曾建议sun在jdk1.4中用log4j取代jdk1.4 的日志工具类,但当时jdk1.4已接近完成,所以sun拒绝使用log4j,当在java开发中
- mysql、sqlserver、oracle分页,java分页统一接口实现
aijuans
oraclejave
定义:pageStart 起始页,pageEnd 终止页,pageSize页面容量
oracle分页:
select * from ( select mytable.*,rownum num from (实际传的SQL) where rownum<=pageEnd) where num>=pageStart
sqlServer分页:
- Hessian 简单例子
antlove
javaWebservicehessian
hello.hessian.MyCar.java
package hessian.pojo;
import java.io.Serializable;
public class MyCar implements Serializable {
private static final long serialVersionUID = 473690540190845543
- 数据库对象的同义词和序列
百合不是茶
sql序列同义词ORACLE权限
回顾简单的数据库权限等命令;
解锁用户和锁定用户
alter user scott account lock/unlock;
//system下查看系统中的用户
select * dba_users;
//创建用户名和密码
create user wj identified by wj;
identified by
//授予连接权和建表权
grant connect to
- 使用Powermock和mockito测试静态方法
bijian1013
持续集成单元测试mockitoPowermock
实例:
package com.bijian.study;
import static org.junit.Assert.assertEquals;
import java.io.IOException;
import org.junit.Before;
import org.junit.Test;
import or
- 精通Oracle10编程SQL(6)访问ORACLE
bijian1013
oracle数据库plsql
/*
*访问ORACLE
*/
--检索单行数据
--使用标量变量接收数据
DECLARE
v_ename emp.ename%TYPE;
v_sal emp.sal%TYPE;
BEGIN
select ename,sal into v_ename,v_sal
from emp where empno=&no;
dbms_output.pu
- 【Nginx四】Nginx作为HTTP负载均衡服务器
bit1129
nginx
Nginx的另一个常用的功能是作为负载均衡服务器。一个典型的web应用系统,通过负载均衡服务器,可以使得应用有多台后端服务器来响应客户端的请求。一个应用配置多台后端服务器,可以带来很多好处:
负载均衡的好处
增加可用资源
增加吞吐量
加快响应速度,降低延时
出错的重试验机制
Nginx主要支持三种均衡算法:
round-robin
l
- jquery-validation备忘
白糖_
jquerycssF#Firebug
留点学习jquery validation总结的代码:
function checkForm(){
validator = $("#commentForm").validate({// #formId为需要进行验证的表单ID
errorElement :"span",// 使用"div"标签标记错误, 默认:&
- solr限制admin界面访问(端口限制和http授权限制)
ronin47
限定Ip访问
solr的管理界面可以帮助我们做很多事情,但是把solr程序放到公网之后就要限制对admin的访问了。
可以通过tomcat的http基本授权来做限制,也可以通过iptables防火墙来限制。
我们先看如何通过tomcat配置http授权限制。
第一步: 在tomcat的conf/tomcat-users.xml文件中添加管理用户,比如:
<userusername="ad
- 多线程-用JAVA写一个多线程程序,写四个线程,其中二个对一个变量加1,另外二个对一个变量减1
bylijinnan
java多线程
public class IncDecThread {
private int j=10;
/*
* 题目:用JAVA写一个多线程程序,写四个线程,其中二个对一个变量加1,另外二个对一个变量减1
* 两个问题:
* 1、线程同步--synchronized
* 2、线程之间如何共享同一个j变量--内部类
*/
public static
- 买房历程
cfyme
2015-06-21: 万科未来城,看房子
2015-06-26: 办理贷款手续,贷款73万,贷款利率5.65=5.3675
2015-06-27: 房子首付,签完合同
2015-06-28,央行宣布降息 0.25,就2天的时间差啊,没赶上。
首付,老婆找他的小姐妹接了5万,另外几个朋友借了1-
- [军事与科技]制造大型太空战舰的前奏
comsci
制造
天气热了........空调和电扇要准备好..........
最近,世界形势日趋复杂化,战争的阴影开始覆盖全世界..........
所以,我们不得不关
- dateformat
dai_lm
DateFormat
"Symbol Meaning Presentation Ex."
"------ ------- ------------ ----"
"G era designator (Text) AD"
"y year
- Hadoop如何实现关联计算
datamachine
mapreducehadoop关联计算
选择Hadoop,低成本和高扩展性是主要原因,但但它的开发效率实在无法让人满意。
以关联计算为例。
假设:HDFS上有2个文件,分别是客户信息和订单信息,customerID是它们之间的关联字段。如何进行关联计算,以便将客户名称添加到订单列表中?
&nbs
- 用户模型中修改用户信息时,密码是如何处理的
dcj3sjt126com
yii
当我添加或修改用户记录的时候对于处理确认密码我遇到了一些麻烦,所有我想分享一下我是怎么处理的。
场景是使用的基本的那些(系统自带),你需要有一个数据表(user)并且表中有一个密码字段(password),它使用 sha1、md5或其他加密方式加密用户密码。
面是它的工作流程: 当创建用户的时候密码需要加密并且保存,但当修改用户记录时如果使用同样的场景我们最终就会把用户加密过的密码再次加密,这
- 中文 iOS/Mac 开发博客列表
dcj3sjt126com
Blog
本博客列表会不断更新维护,如果有推荐的博客,请到此处提交博客信息。
本博客列表涉及的文章内容支持 定制化Google搜索,特别感谢 JeOam 提供并帮助更新。
本博客列表也提供同步更新的OPML文件(下载OPML文件),可供导入到例如feedly等第三方定阅工具中,特别感谢 lcepy 提供自动转换脚本。这里有导入教程。
- js去除空格,去除左右两端的空格
蕃薯耀
去除左右两端的空格js去掉所有空格js去除空格
js去除空格,去除左右两端的空格
>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>&g
- SpringMVC4零配置--web.xml
hanqunfeng
springmvc4
servlet3.0+规范后,允许servlet,filter,listener不必声明在web.xml中,而是以硬编码的方式存在,实现容器的零配置。
ServletContainerInitializer:启动容器时负责加载相关配置
package javax.servlet;
import java.util.Set;
public interface ServletContainer
- 《开源框架那些事儿21》:巧借力与借巧力
j2eetop
框架UI
同样做前端UI,为什么有人花了一点力气,就可以做好?而有的人费尽全力,仍然错误百出?我们可以先看看几个故事。
故事1:巧借力,乌鸦也可以吃核桃
有一个盛产核桃的村子,每年秋末冬初,成群的乌鸦总会来到这里,到果园里捡拾那些被果农们遗落的核桃。
核桃仁虽然美味,但是外壳那么坚硬,乌鸦怎么才能吃到呢?原来乌鸦先把核桃叼起,然后飞到高高的树枝上,再将核桃摔下去,核桃落到坚硬的地面上,被撞破了,于是,
- JQuery EasyUI 验证扩展
可怜的猫
jqueryeasyui验证
最近项目中用到了前端框架-- EasyUI,在做校验的时候会涉及到很多需要自定义的内容,现把常用的验证方式总结出来,留待后用。
以下内容只需要在公用js中添加即可。
使用类似于如下:
<input class="easyui-textbox" name="mobile" id="mobile&
- 架构师之httpurlconnection----------读取和发送(流读取效率通用类)
nannan408
1.前言.
如题.
2.代码.
/*
* Copyright (c) 2015, S.F. Express Inc. All rights reserved.
*/
package com.test.test.test.send;
import java.io.IOException;
import java.io.InputStream
- Jquery性能优化
r361251
JavaScriptjquery
一、注意定义jQuery变量的时候添加var关键字
这个不仅仅是jQuery,所有javascript开发过程中,都需要注意,请一定不要定义成如下:
$loading = $('#loading'); //这个是全局定义,不知道哪里位置倒霉引用了相同的变量名,就会郁闷至死的
二、请使用一个var来定义变量
如果你使用多个变量的话,请如下方式定义:
. 代码如下:
var page
- 在eclipse项目中使用maven管理依赖
tjj006
eclipsemaven
概览:
如何导入maven项目至eclipse中
建立自有Maven Java类库服务器
建立符合maven代码库标准的自定义类库
Maven在管理Java类库方面有巨大的优势,像白衣所说就是非常“环保”。
我们平时用IDE开发都是把所需要的类库一股脑的全丢到项目目录下,然后全部添加到ide的构建路径中,如果用了SVN/CVS,这样会很容易就 把
- 中国天气网省市级联页面
x125858805
级联
1、页面及级联js
<%@ page language="java" import="java.util.*" pageEncoding="UTF-8"%>
<!DOCTYPE HTML PUBLIC "-//W3C//DTD HTML 4.01 Transitional//EN">
&l