- C++数据结构————二叉树
Гений.大天才
C++语言入门以及基础算法c++数据结构开发语言
【前言】在数据结构与算法的世界里,二叉树(BinaryTree)始终占据着核心地位。它既是众多高级树形结构(B+树、红黑树、线段树、字典树……)的“基因”,又是面试、竞赛与工程实战中绕不开的考点。本文将用大约2万字的篇幅,从“零”开始,把C++二叉树的所有常见形态、常见算法、常见坑点与常见优化一次性讲透。全文配套可编译运行的C++17/20代码2000余行,所有示例均在GCC13/Clang17/
- HDU杭电OJ基础100题2010-2019(C语言版)
雁于飞
算法专栏c语言开发语言
文章目录@[TOC](文章目录)[原题出处](https://acm.hdu.edu.cn/listproblem.php?vol=11)前言p2010.水仙花数问题描述解题思路代码核心思想:p2011多项式求和问题描述代码p2003求绝对值问题描述解题思路代码扩展p2004成绩转换问题描述解题思路代码重点p2005第几天问题描述解题思路代码扩展p2006求奇数的乘积p2007平方和与立方和问题描
- P1967 [NOIP 2013 提高组] 货车运输(树链剖分+线段树)
gw_water
cocoac++算法贪心算法数据结构
文章目录题目要求一、解题思路二、解题过程1.数据结构2.求最小生成树(Kruskal算法)2.答案计算(TCD+SegementTree)AC代码题目要求A国有n座城市,编号从1到n,城市之间有m条双向道路。每一条道路对车辆都有重量限制,简称限重。现在有q辆货车在运输货物,司机们想知道每辆车在不超过车辆限重的情况下,最多能运多重的货物。一、解题思路本题求一条路径,使得其在不超过限制重量的前提下,载
- 线段树懒标记详解
xwztdas
线段树/平衡树线段树数据结构算法
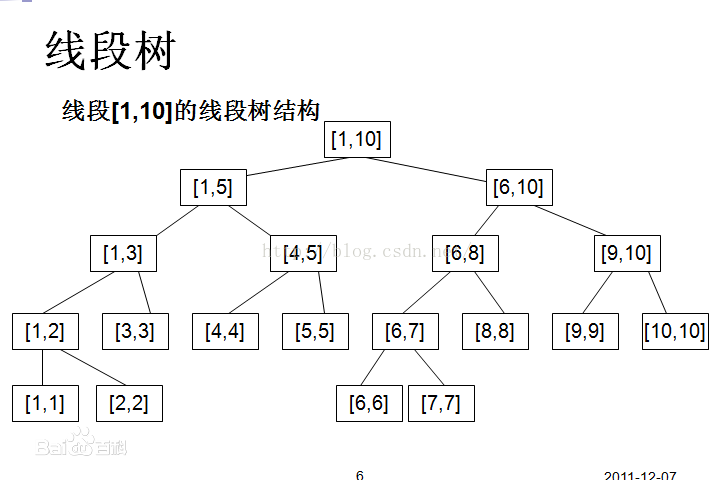
引入在上一篇题解。我们详细讲解了单点修改,区间查询的线段树。在这篇题解我们将要讲解区间修改,区间查询的线段树。懒标记背景我们发现虽然我们可以做到在O(logn)O(log_{n})O(logn)的时间内做到单点修改,但我们如果将一个区间修改,我们发现时间复杂度为O(nlogn)O(nlog_{n})O(nlogn),比暴力还慢。那我们只能想一些其他方法了。结构分析我们先从线段树的结构入手:还是这张
- 前缀和与后缀和(HDU6186)
MatrixYg
HDU水题
题目链接。题目的大意是:给一个数组,和一个数组的下标·,然后在数组中去掉这个下标对应的元素,把剩下的元素全部做&/|/^这三种位运算,输出位运算之后的结果。数据范围1e5.当然暴力是不可行的。首先需要知道的是:一个数&自己不变,|自己也是不变,^自己是0。这样我们对于每一种运算维护两个数组,一个前缀数组,一个后缀数组。这样两个结合起来可以达到去除任意一个中间元素的效果。//我们只证明一种情况,其他
- C++基础练习-二维数组
s15335
C++练习题c++开发语言
题目:https://acm.hdu.edu.cn/showproblem.php?pid=2022题解:#includeusingnamespacestd;intz[10000][10000];intmain(){intm,n;while(cin>>m>>n){intx,max=-1,l,c;//往数组里添加数据for(inti=0;i>z[i][j];}}//遍历数组并找出最大值for(int
- 数据结构-顺序表-数值统计
题目:https://acm.hdu.edu.cn/showproblem.php?pid=2008解答:#includeusingnamespacestd;#defineSLDataTypedoublestructSequlist{SLDataType*array;intsize;intcapacity;};//********************顺序表初始化***********/void
- 蓝桥杯刷题 Day5 线段树(树状数组)
雁于飞
蓝桥杯职场和发展学习笔记数据结构算法java
蓝桥杯刷题Day5线段树文章目录蓝桥杯刷题Day5线段树前言完整代码一、树状数组1.解题思路1.1问题抽象1.2核心思想1.2适用条件:1.3典型应用:2.拆解代码2.1主函数2.1.1输入以及初始化2.1.2处理查询2.2SegmentTree类2.2.1初始化数组以及最低有效位2.2.2单点更新与集区间求和二、题后收获3.1知识点前言今天写牛客网模板题中数据结构的线段树完整代码一、树状数组原题
- P3740 [HAOI2014] 贴海报 题解
lhschris
题解
P3740[HAOI2014]贴海报题解思路我们模拟一下贴海报的过程,先把x∼yx\simyx∼y的数字全部变成kkk。后面的数字可以覆盖前面的数字。如果for循环枚举的话是会超时的,我们考虑用线段树维护区间数字。那么所有操作结束后如果当前区间还有当前数字,ans++ans++ans++。那么这么判断呢?也就是pushup怎么做?求最小值最好了。因为每个区间的最小值只能是当前数字,因为当前区间已经
- 小木的算法日记-线段树
木旭林晖
算法
线段树(SegmentTree):玩转区间作的终极利器你好,未来的算法大师!想象一下,你正在处理一个巨大的数据集,比如某个电商网站一整天的用户点击流。老板突然问你:“下午2点到3点之间,我们的总点击量是多少?”几分钟后,他又问:“把10点到11点之间的数据,因为系统故障,全部乘以0.5,然后再告诉我下午的总点击量。”如果用普通的数组,每次查询都需要遍历,每次修改更是灾难。面对这种需求,我们该怎么办
- 【贪心、DP、线段树优化】Leetcode 376. 摆动序列
Wendy_robot
leetcode算法
贪心算法:选“关键转折点”初始状态:把数组第一个元素当作起点,此时前一个差值符号设为平坡(即差值为0)。遍历数组:从第二个元素开始,依次计算当前元素和前一个元素的差值。差值符号判断:差值大于0:要是之前的差值是小于等于0(平坡或者下降状态),那就说明找到了一个从下降到上升的摆动点,更新最大摆动点数,同时把前一个差值符号标记为上升(大于0)。差值小于0:若之前的差值是大于等于0(平坡或者上升状态),
- Codeforces Round 974 (Div. 3) A-F
swan416
题解图论算法c++数据结构算法竞赛Codeforces信息学竞赛
封面原图画师礼島れいあ下午的ICPC网络赛的难受一晚上全都给我打没了手速拉满再加上秒杀线段树这场简直了啊唯一可惜的是最后还是掉出了1000名一把上蓝应该没啥希望了吧A-RobinHelps题意侠盗罗宾因劫富济贫而闻名于世罗宾遇到的nnn人,从1st1_{st}1st开始,到nthn_{th}nth结束。iii第三个人有aia_iai金子。如果是ai≥ka_i\gekai≥k,罗宾会拿走所有的aia
- 【Algorithm】Segment Tree 简单介绍
CodeWithMe
C/C++c++算法python
文章目录SegmentTree1基本概念2基本思想3适用场景4代码示例(区间求和)5使用示例6使用注意事项7进阶拓展SegmentTree线段树(SegmentTree)是一种高级数据结构,主要用于在区间范围内高效地进行查询与修改操作。它是一个二叉树结构,每个节点代表一个区间的信息,通常用于解决如下问题:1基本概念线段树是对一个区间[l,r]上的数列进行划分,并在每个子区间上维护某种信息(如最值、
- 【算法笔记】树套树
PXM的算法星球
算法笔记算法笔记
一、前言在面对二维区间统计问题时,比如:查询某个一维区间中,大于某个值的数的个数对一个序列同时支持区间查询+单点修改我们常用的一维数据结构(如线段树、树状数组)往往显得力不从心。此时,我们可以考虑一种高效的数据结构组合:树套树。二、什么是树套树?“树套树”顾名思义,就是一棵树中的每个节点再套一棵树。最常见的树套树结构是:外层:线段树/树状数组,按照下标维护区间内层:平衡树(如STLmultiset
- Python·算法分类题库
欢迎关注【Python·算法分类题库】,持续更新中……知识点A字符串(AC自动机、拓展KMP、后缀数组、后缀自动机、回文自动机)图论(网络流、一般图匹配)数学(生成函数、莫比乌斯反演、快速傅里叶变换)数据结构(树链剖分、二维/动态开点线段树、平衡树、可持久化数据结构、树套树、动态树)B排序(归并、快速、桶、堆、基数)搜索(剪枝、双向BFS、记忆化搜索、迭代加深搜索、启发式搜索)DP(背包、树形、状
- 图论500题 慢慢写
daydreamer23333
题目来源https://blog.csdn.net/ffq5050139/article/details/7832991这篇博客用来记录自己刷的图论题先占个坑所有题目都来自上面的链接会慢慢更新基础一点的题会记录一下表示ac了好题会单独写一篇博客知识点题目名称,oj和题号并查集1.HowManyTablesHDU-1213(简单模板题)并查集2.小希的迷宫HDU-1272(毒瘤输入wa了一年最后发现
- HDU-2973-YAPTCHA(威尔逊定理)
Herod_
算法练习数论数论
YAPTCHAProblemDescriptionThemathdepartmenthasbeenhavingproblemslately.Duetoimmenseamountofunsolicitedautomatedprogramswhichwerecrawlingacrosstheirpages,theydecidedtoputYet-Another-Public-Turing-Test-t
- HDU-2973 YAPTCHA
STY_fish_2012
数学素数筛
题目传送门先把题目中的公式弄过来。Sn=∑k=1n⌊(3k+6)!+13k+7−⌊(3k+6)!3k+7⌋⌋S_n=\sum\limits_{k=1}^{n}\lfloor\frac{(3k+6)!+1}{3k+7}-\lfloor\frac{(3k+6)!}{3k+7}\rfloor\rfloorSn=k=1∑n⌊3k+7(3k+6)!+1−⌊3k+7(3k+6)!⌋⌋首先,得先了解威尔逊定理威
- 计算机类专业学生重要竞赛刷题网站
花开盛夏^.^
大学生竞赛大学生计算机类专业专业竞赛
团队队员常用:Codeforceshttp://codeforces.com/problemset牛客网https://www.nowcoder.com/ta/acm-training/刷题链接:http://poj.org/pojhttp://www.spoj.com/http://acm.hdu.edu.cn/hduhttps://cn.vjudge.net/vj(包含大部分网站的题库)htt
- 蓝桥杯康复训练 Day4 (前缀和)(树状数组)(线段树)
ooold_six
2022蓝桥杯java算法
昨天没状态摆了一天,今天复习一下各种区间问题前缀和常规遍历区间求和复杂度O(n)单点修改复杂度O(1)前缀和区间求和复杂度O(1)单点修改复杂度O(n)前缀和数组中每个值覆盖的是从开始到该点整个区间的和值求i~j的区间和值可以通过s[j]-s[i-1]计算可以扩展成二维三维的前缀和在单点修改时需要对所有覆盖该点的值进行修改在对区间求和复杂度要求高时使用蓝桥杯–前缀和1树状数组对比前缀和复杂度前缀和
- 线段树刷题1
code自留地
线段树
[TJOI2009]开关luogu链接:https://www.luogu.com.cn/problem/P3870ps:好好的一道省选题打绿了不说,居然是道橙题!也就和一道dfs的难度一样···你洛谷是不是人均线段树??分析题意:我们仍然是要进行区间修改的操作,那懒标记是逃不过了喂·然后我们分析至少需要维护哪些信息:亮灯总个数是需要维护的吧但是这样够不够?因为我们还要表示出“亮灯变暗,暗灯点亮”
- 线段树刷题记录
弥彦_
c++算法c++数据结构
一、区间查询无修改:(一)最值问题:1.P1816忠诚-洛谷思路:模板。注意:无。代码:#include#defineiosccios::sync_with_stdio(false),cin.tie(0),cout.tie(0)#defineendl'\n'#defineme(a,x)memset(a,x,sizeofa)#defineall(a)a.begin(),a.end()#defines
- hdu2108判断多边形是否为凸多边形
自爄創煇熿
计算几何
矢量的叉积,来判断拐向。代码如下:#include#include#includeusingnamespacestd;structcoordinate{doublex;doubley;};intcross(coordinatep1,coordinatep2,coordinatep3){if((p2.x-p1.x)*(p3.y-p1.y)-(p3.x-p1.x)*(p2.y-p1.y)>0)retu
- 数据结构---线段树
4FGR
数据结构开发语言c++算法数据结构
线段树参考:线段树-OIWiki线段树是一种二叉搜索树、平衡二叉树,对于区间的修改、维护和查询时间复杂度优化为log级别。对区间不断做平分区间,直到划分到只有一个或多个数据的区间,可表示区间的和或者最小最大值。在进行更新区间操作时,通过小区间更新大区间。对于下面的内容,我们主要针对于区间加法的线段树(即其节点表示区间之和)。局限性:问题需满足区间加法:对于[L,R]的区间,它的答案可以由[L,M]
- 洛谷所有 NOI/NOI+/CTSC 的题目
一个不会写代码的小白
c++洛谷洛谷NOI/NOI+/CTSCc++算法数据结构开发语言
洛谷——luoguOJ所有NOI/NOI+/CTSC的P/C开头题目P8861线段P5111zhtobu3232的线段树P7719「EZEC-10」多彩的线段P5210[ZJOI2017]线段树P10145[WC2024]线段树P10211[CTS2024]线段树P7445「EZEC-7」线段树P5342[TJOI2019]甲苯先生的线段树P8498[NOI2022]树上邻域数点P5659[CSP
- USST新生训练赛3KLMN
Fighter_sky
题解C++acm
题解前言题解部分KPashmakandParmida'sproblem(1800)题目大意题解参考代码LPashmakandGraph(1900)题目大意题解参考代码MLuckyChains(1600)题目大意题解参考代码NManipulatingHistory(1600)题目大意题解参考代码前言KLMN是数据结构(线段树/树状数组)+dp+数论+结论唐题题解部分KPashmakandParmid
- 权值线段树和可持久化线段树(主席树)
.Q_W.
算法算法数据结构
目录权值线段树权值线段树的基本概念权值线段树的构建权值线段树的操作添加元素查询区间[l,r]的元素个数查询整个集合中第k小(或第k大)的元素值例题代码实现可持久化线段树(主席树)例题1代码实现例题2思路代码实现权值线段树权值线段树的基本概念权值线段树是一种特殊的线段树,它的叶子节点存储的是某个元素的权值(通常是该元素的出现次数),而不是元素本身。分支节点则存储其子节点权值的某种集合值(如和、最值等
- 最长公共子串 后缀数组 算法 php,HDU 3518 Boring counting(后缀数组啊 求字符串中不重叠的重复出现最少两次的子串的个数)...
盖亚能量炮
最长公共子串后缀数组算法php
HDU3518Boringcounting(后缀数组啊求字符串中不重叠的重复出现至少两次的子串的个数)题目链接:http://acm.hdu.edu.cn/showproblem.php?pid=3518ProblemDescription035nowfacedatoughproblem,hisenglishteachergiveshimastring,whichconsistswithnlowe
- Boring counting HDU - 4358(树上出现k次的数字个数)
starlet_kiss
树状数组
InthisproblemweconsiderarootedtreewithNvertices.Theverticesarenumberedfrom1toN,andvertex1representstheroot.Thereareintegerweightsoneachvectice.Yourtaskistoansweralistofqueries,foreachquery,pleasetellu
- HDU 3518 Boring counting(后缀数组)
Nightmare丶
SASA
题意:求出不重叠切出现次数超过两次的子串个数题解:后缀数组分组后,判断每组出现的sa最大值和最小值之差是否是大于k就好了,k通过枚举即可AC代码:#includeusingnamespacestd;constintINF=0x3f3f3f3f;constintMAXN=2000+50;chars[MAXN];intrk[MAXN],h[MAXN],y[MAXN],sa[MAXN],c[MAXN];
- 分享100个最新免费的高匿HTTP代理IP
mcj8089
代理IP代理服务器匿名代理免费代理IP最新代理IP
推荐两个代理IP网站:
1. 全网代理IP:http://proxy.goubanjia.com/
2. 敲代码免费IP:http://ip.qiaodm.com/
120.198.243.130:80,中国/广东省
58.251.78.71:8088,中国/广东省
183.207.228.22:83,中国/
- mysql高级特性之数据分区
annan211
java数据结构mongodb分区mysql
mysql高级特性
1 以存储引擎的角度分析,分区表和物理表没有区别。是按照一定的规则将数据分别存储的逻辑设计。器底层是由多个物理字表组成。
2 分区的原理
分区表由多个相关的底层表实现,这些底层表也是由句柄对象表示,所以我们可以直接访问各个分区。存储引擎管理分区的各个底层
表和管理普通表一样(所有底层表都必须使用相同的存储引擎),分区表的索引只是
- JS采用正则表达式简单获取URL地址栏参数
chiangfai
js地址栏参数获取
GetUrlParam:function GetUrlParam(param){
var reg = new RegExp("(^|&)"+ param +"=([^&]*)(&|$)");
var r = window.location.search.substr(1).match(reg);
if(r!=null
- 怎样将数据表拷贝到powerdesigner (本地数据库表)
Array_06
powerDesigner
==================================================
1、打开PowerDesigner12,在菜单中按照如下方式进行操作
file->Reverse Engineer->DataBase
点击后,弹出 New Physical Data Model 的对话框
2、在General选项卡中
Model name:模板名字,自
- logbackのhelloworld
飞翔的马甲
日志logback
一、概述
1.日志是啥?
当我是个逗比的时候我是这么理解的:log.debug()代替了system.out.print();
当我项目工作时,以为是一堆得.log文件。
这两天项目发布新版本,比较轻松,决定好好地研究下日志以及logback。
传送门1:日志的作用与方法:
http://www.infoq.com/cn/articles/why-and-how-log
上面的作
- 新浪微博爬虫模拟登陆
随意而生
新浪微博
转载自:http://hi.baidu.com/erliang20088/item/251db4b040b8ce58ba0e1235
近来由于毕设需要,重新修改了新浪微博爬虫废了不少劲,希望下边的总结能够帮助后来的同学们。
现行版的模拟登陆与以前相比,最大的改动在于cookie获取时候的模拟url的请求
- synchronized
香水浓
javathread
Java语言的关键字,可用来给对象和方法或者代码块加锁,当它锁定一个方法或者一个代码块的时候,同一时刻最多只有一个线程执行这段代码。当两个并发线程访问同一个对象object中的这个加锁同步代码块时,一个时间内只能有一个线程得到执行。另一个线程必须等待当前线程执行完这个代码块以后才能执行该代码块。然而,当一个线程访问object的一个加锁代码块时,另一个线程仍然
- maven 简单实用教程
AdyZhang
maven
1. Maven介绍 1.1. 简介 java编写的用于构建系统的自动化工具。目前版本是2.0.9,注意maven2和maven1有很大区别,阅读第三方文档时需要区分版本。 1.2. Maven资源 见官方网站;The 5 minute test,官方简易入门文档;Getting Started Tutorial,官方入门文档;Build Coo
- Android 通过 intent传值获得null
aijuans
android
我在通过intent 获得传递兑现过的时候报错,空指针,我是getMap方法进行传值,代码如下 1 2 3 4 5 6 7 8 9
public
void
getMap(View view){
Intent i =
- apache 做代理 报如下错误:The proxy server received an invalid response from an upstream
baalwolf
response
网站配置是apache+tomcat,tomcat没有报错,apache报错是:
The proxy server received an invalid response from an upstream server. The proxy server could not handle the request GET /. Reason: Error reading fr
- Tomcat6 内存和线程配置
BigBird2012
tomcat6
1、修改启动时内存参数、并指定JVM时区 (在windows server 2008 下时间少了8个小时)
在Tomcat上运行j2ee项目代码时,经常会出现内存溢出的情况,解决办法是在系统参数中增加系统参数:
window下, 在catalina.bat最前面
set JAVA_OPTS=-XX:PermSize=64M -XX:MaxPermSize=128m -Xms5
- Karam与TDD
bijian1013
KaramTDD
一.TDD
测试驱动开发(Test-Driven Development,TDD)是一种敏捷(AGILE)开发方法论,它把开发流程倒转了过来,在进行代码实现之前,首先保证编写测试用例,从而用测试来驱动开发(而不是把测试作为一项验证工具来使用)。
TDD的原则很简单:
a.只有当某个
- [Zookeeper学习笔记之七]Zookeeper源代码分析之Zookeeper.States
bit1129
zookeeper
public enum States {
CONNECTING, //Zookeeper服务器不可用,客户端处于尝试链接状态
ASSOCIATING, //???
CONNECTED, //链接建立,可以与Zookeeper服务器正常通信
CONNECTEDREADONLY, //处于只读状态的链接状态,只读模式可以在
- 【Scala十四】Scala核心八:闭包
bit1129
scala
Free variable A free variable of an expression is a variable that’s used inside the expression but not defined inside the expression. For instance, in the function literal expression (x: Int) => (x
- android发送json并解析返回json
ronin47
android
package com.http.test;
import org.apache.http.HttpResponse;
import org.apache.http.HttpStatus;
import org.apache.http.client.HttpClient;
import org.apache.http.client.methods.HttpGet;
import
- 一份IT实习生的总结
brotherlamp
PHPphp资料php教程php培训php视频
今天突然发现在不知不觉中自己已经实习了 3 个月了,现在可能不算是真正意义上的实习吧,因为现在自己才大三,在这边撸代码的同时还要考虑到学校的功课跟期末考试。让我震惊的是,我完全想不到在这 3 个月里我到底学到了什么,这是一件多么悲催的事情啊。同时我对我应该 get 到什么新技能也很迷茫。所以今晚还是总结下把,让自己在接下来的实习生活有更加明确的方向。最后感谢工作室给我们几个人这个机会让我们提前出来
- 据说是2012年10月人人网校招的一道笔试题-给出一个重物重量为X,另外提供的小砝码重量分别为1,3,9。。。3^N。 将重物放到天平左侧,问在两边如何添加砝码
bylijinnan
java
public class ScalesBalance {
/**
* 题目:
* 给出一个重物重量为X,另外提供的小砝码重量分别为1,3,9。。。3^N。 (假设N无限大,但一种重量的砝码只有一个)
* 将重物放到天平左侧,问在两边如何添加砝码使两边平衡
*
* 分析:
* 三进制
* 我们约定括号表示里面的数是三进制,例如 47=(1202
- dom4j最常用最简单的方法
chiangfai
dom4j
要使用dom4j读写XML文档,需要先下载dom4j包,dom4j官方网站在 http://www.dom4j.org/目前最新dom4j包下载地址:http://nchc.dl.sourceforge.net/sourceforge/dom4j/dom4j-1.6.1.zip
解开后有两个包,仅操作XML文档的话把dom4j-1.6.1.jar加入工程就可以了,如果需要使用XPath的话还需要
- 简单HBase笔记
chenchao051
hbase
一、Client-side write buffer 客户端缓存请求 描述:可以缓存客户端的请求,以此来减少RPC的次数,但是缓存只是被存在一个ArrayList中,所以多线程访问时不安全的。 可以使用getWriteBuffer()方法来取得客户端缓存中的数据。 默认关闭。 二、Scan的Caching 描述: next( )方法请求一行就要使用一次RPC,即使
- mysqldump导出时出现when doing LOCK TABLES
daizj
mysqlmysqdump导数据
执行 mysqldump -uxxx -pxxx -hxxx -Pxxxx database tablename > tablename.sql
导出表时,会报
mysqldump: Got error: 1044: Access denied for user 'xxx'@'xxx' to database 'xxx' when doing LOCK TABLES
解决
- CSS渲染原理
dcj3sjt126com
Web
从事Web前端开发的人都与CSS打交道很多,有的人也许不知道css是怎么去工作的,写出来的css浏览器是怎么样去解析的呢?当这个成为我们提高css水平的一个瓶颈时,是否应该多了解一下呢?
一、浏览器的发展与CSS
- 《阿甘正传》台词
dcj3sjt126com
Part Ⅰ:
《阿甘正传》Forrest Gump经典中英文对白
Forrest: Hello! My names Forrest. Forrest Gump. You wanna Chocolate? I could eat about a million and a half othese. My momma always said life was like a box ochocol
- Java处理JSON
dyy_gusi
json
Json在数据传输中很好用,原因是JSON 比 XML 更小、更快,更易解析。
在Java程序中,如何使用处理JSON,现在有很多工具可以处理,比较流行常用的是google的gson和alibaba的fastjson,具体使用如下:
1、读取json然后处理
class ReadJSON
{
public static void main(String[] args)
- win7下nginx和php的配置
geeksun
nginx
1. 安装包准备
nginx : 从nginx.org下载nginx-1.8.0.zip
php: 从php.net下载php-5.6.10-Win32-VC11-x64.zip, php是免安装文件。
RunHiddenConsole: 用于隐藏命令行窗口
2. 配置
# java用8080端口做应用服务器,nginx反向代理到这个端口即可
p
- 基于2.8版本redis配置文件中文解释
hongtoushizi
redis
转载自: http://wangwei007.blog.51cto.com/68019/1548167
在Redis中直接启动redis-server服务时, 采用的是默认的配置文件。采用redis-server xxx.conf 这样的方式可以按照指定的配置文件来运行Redis服务。下面是Redis2.8.9的配置文
- 第五章 常用Lua开发库3-模板渲染
jinnianshilongnian
nginxlua
动态web网页开发是Web开发中一个常见的场景,比如像京东商品详情页,其页面逻辑是非常复杂的,需要使用模板技术来实现。而Lua中也有许多模板引擎,如目前我在使用的lua-resty-template,可以渲染很复杂的页面,借助LuaJIT其性能也是可以接受的。
如果学习过JavaEE中的servlet和JSP的话,应该知道JSP模板最终会被翻译成Servlet来执行;而lua-r
- JZSearch大数据搜索引擎
颠覆者
JavaScript
系统简介:
大数据的特点有四个层面:第一,数据体量巨大。从TB级别,跃升到PB级别;第二,数据类型繁多。网络日志、视频、图片、地理位置信息等等。第三,价值密度低。以视频为例,连续不间断监控过程中,可能有用的数据仅仅有一两秒。第四,处理速度快。最后这一点也是和传统的数据挖掘技术有着本质的不同。业界将其归纳为4个“V”——Volume,Variety,Value,Velocity。大数据搜索引
- 10招让你成为杰出的Java程序员
pda158
java编程框架
如果你是一个热衷于技术的
Java 程序员, 那么下面的 10 个要点可以让你在众多 Java 开发人员中脱颖而出。
1. 拥有扎实的基础和深刻理解 OO 原则 对于 Java 程序员,深刻理解 Object Oriented Programming(面向对象编程)这一概念是必须的。没有 OOPS 的坚实基础,就领会不了像 Java 这些面向对象编程语言
- tomcat之oracle连接池配置
小网客
oracle
tomcat版本7.0
配置oracle连接池方式:
修改tomcat的server.xml配置文件:
<GlobalNamingResources>
<Resource name="utermdatasource" auth="Container"
type="javax.sql.DataSou
- Oracle 分页算法汇总
vipbooks
oraclesql算法.net
这是我找到的一些关于Oracle分页的算法,大家那里还有没有其他好的算法没?我们大家一起分享一下!
-- Oracle 分页算法一
select * from (
select page.*,rownum rn from (select * from help) page
-- 20 = (currentPag