Codeforces Round #621 (Div. 1 + Div. 2)【A - D】

题目来源：https://codeforces.com/contest/1307
这场还好没打

---

1307A - Cow and Haybales

这题就是个简单的贪心，尽量让靠近左边的先往左移

#include
#include
#include
#include
#include
#include
#include
#include
#include
#include
#include
#define ls k<<1,l,mid
#define rs k<<1|1,mid+1,r
#define mp(x,y) make_pair(x,y)
#define r(x) read(x)
#define rrr(x,y,z) read(x);read(y);read(z)
#define FOR(i,l,r) for(int i=l;i<=r;i++)
using namespace std;
typedef long long LL;
typedef pair<int,int> pt;
const int N=1e6+5;
const int M=2e3+5;
const int INF=0x7fffffff;
const int mod=1e9+7;
const int sz=18;
const double eps=1e-10;
const double pi=acos(-1);
int n,m;
char str[N];
int f[N];
map<string,int> mm;
vector<string> v;
template<class T>
inline void read(T &x)
{
    char c; x=1;
    while((c=getchar())<'0'||c>'9') if(c=='-') x=-1;
    T res=c-'0';
    while((c=getchar())>='0'&&c<='9') res=res*10+c-'0';
    x*=res;
}
int main()
{
    int t;
    r(t);
    while(t--){
        r(n); r(m);
        LL ans=0;
        FOR(i,1,n){
            int a; r(a);
            if(m>=(i-1)*a){
                ans+=a;
                m-=(i-1)*a;
            }
            else{
                ans+=m/(i-1);
                m=m%(i-1);
            }
        }
        cout<<ans<<endl;
    }
    return 0;
}

---

1307B - Cow and Friend

这题只要线段的和大于x 那么一定是可以到达的，你让他随便弯曲总有方法到达

至于为什么不会选择多种线段组合，可以这样想 假如由两种组成a、b，且a>b
现在ka+b>=x 那(k+1)a>x也成立 所以没必要考虑

#include
#include
#include
#include
#include
#include
#include
#include
#include
#include
#include
#define ls k<<1,l,mid
#define rs k<<1|1,mid+1,r
#define mp(x,y) make_pair(x,y)
#define r(x) read(x)
#define rrr(x,y,z) read(x);read(y);read(z)
#define FOR(i,l,r) for(int i=l;i<=r;i++)
using namespace std;
typedef long long LL;
typedef pair<int,int> pt;
const int N=1e6+5;
const int M=2e3+5;
const int INF=0x7fffffff;
const int mod=1e9+7;
const int sz=18;
const double eps=1e-10;
const double pi=acos(-1);
int n,m;
char str[N];
int f[N];
template<class T>
inline void read(T &x)
{
    char c; x=1;
    while((c=getchar())<'0'||c>'9') if(c=='-') x=-1;
    T res=c-'0';
    while((c=getchar())>='0'&&c<='9') res=res*10+c-'0';
    x*=res;
}
int main()
{
    int t;
    r(t);
    while(t--){
        r(n); r(m);
        int ans=INF;
        FOR(i,1,n){
            int a;
            r(a);
            if(a>m){
                ans=min(2,ans);
            }
            else{
                int cnt=m/a;
                if(m%a!=0) cnt++;
                ans=min(ans,cnt);
            }
        }
        cout<<ans<<endl;
    }
    return 0;
}

---

1307C - Cow and Message

显而易见，最大的一定是出现在长度为1或2之中，因为如果长度为3，在这个长度里面一定会找到长度为2的。例 abc 有k次 那 ab起码有k次 所以最大只需要在长度为1和2的里面找 组合有26+26*26种 取其中的最大值即可
怎么统计呢？统计每个字母的贡献

#include
#include
#include
#include
#include
#include
#include
#include
#include
#include
#include
#define ls k<<1,l,mid
#define rs k<<1|1,mid+1,r
#define mp(x,y) make_pair(x,y)
#define r(x) read(x)
#define rrr(x,y,z) read(x);read(y);read(z)
#define FOR(i,l,r) for(int i=l;i<=r;i++)
using namespace std;
typedef long long LL;
typedef pair<int,int> pt;
const int N=1e5+5;
const int M=2e3+5;
const int INF=0x7fffffff;
const int mod=1e9+7;
const int sz=18;
const double eps=1e-10;
const double pi=acos(-1);
int n,m;
char str[N];
LL sum[N][30];
LL res[30][30];
template<class T>
inline void read(T &x)
{
    char c; x=1;
    while((c=getchar())<'0'||c>'9') if(c=='-') x=-1;
    T res=c-'0';
    while((c=getchar())>='0'&&c<='9') res=res*10+c-'0';
    x*=res;
}
int main()
{
    scanf("%s",str+1);
    n=strlen(str+1);
    FOR(i,1,n){
        int t=str[i]-'a'+1;
        FOR(j,1,26){
            if(j==t) sum[i][j]=sum[i-1][j]+1;
            else sum[i][j]=sum[i-1][j];
            //cout<
        }
        //cout<
    }
    LL ans=0;
    FOR(i,1,n){
        int t=str[i]-'a'+1;
        FOR(j,1,26){
            res[t][j]+=sum[n][j]-sum[i][j];
        }
    }
    FOR(i,1,26){
        ans=max(ans,sum[n][i]);
        FOR(j,1,26){
            ans=max(ans,res[i][j]);
        }
    }
    cout<<ans<<endl;
    return 0;
}

---

1307D - Cow and Fields

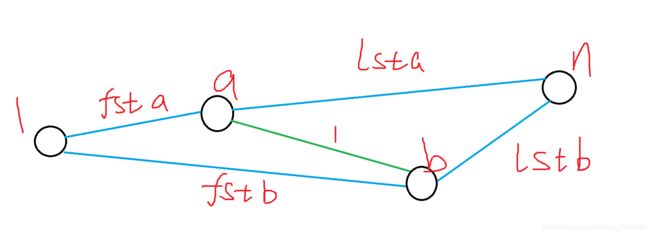
首先我们求出所有点到1 和 n的最短距离 即为fst lst
设两个特殊点a,b 假如现在连接了a b 那么会产生两个1 - n的距离 ，那个人肯定要走最近的 即 $min(fsta+lstb,fstb+lsta)+1$ 但是这个不太好求 我们转换一下$min(fsta-lsta,fstb-lstb)+lsta+lstb+1$ 我们对这些点按fsta-fstb从小到大排序，那假如我们现在选了其中的点y 和它前面的点x 那当前的答案就是$fstx-lstx+lstx+lsty+1=fstx+lsty+1$ lsty就是当前点的，而fstx当然要取前面最大的，所以排完序之后遍历的时候记录一个前缀最大值即可~

#include
#include
#include
#include
#include
#include
#include
#include
#include
#include
#include
#define ls k<<1,l,mid
#define rs k<<1|1,mid+1,r
#define mp(x,y) make_pair(x,y)
#define r(x) read(x)
#define rr(x,y) read(x);read(y)
#define rrr(x,y,z) read(x);read(y);read(z)
#define FOR(i,l,r) for(int i=l;i<=r;i++)
#define pb(x) push_back(x)
#define sss(str) scanf("%s",str+1)
#define ssf(x) scanf("%lf",&x)
#define all(x) x.begin(),x.end()
using namespace std;
typedef long long LL;
typedef double dd;
typedef pair<int,int> pt;
const int N=2e5+5;
const int M=2e3+5;
const int INF=0x7fffffff;
const int mod=1e9+7;
const int sz=18;
const double eps=1e-10;
const double pi=acos(-1);
//***********************
int fst[N],lst[N];
int f[N];
bool vis[N];
vector<int> v[N];
//***********************
template<class T>
inline void read(T &x)
{
    char c; x=1;
    while((c=getchar())<'0'||c>'9') if(c=='-') x=-1;
    T res=c-'0';
    while((c=getchar())>='0'&&c<='9') res=res*10+c-'0';
    x*=res;
}
void bfs(int x,int step)
{
    memset(vis,0,sizeof vis);
    queue<pt> q;
    q.push(mp(x,1));
    bool flag=(x==1);
    while(q.size()){
        pt now=q.front(); q.pop();
        vis[now.first]=1;
        if(flag) fst[now.first]=now.second;
        else lst[now.first]=now.second;
        for(auto y:v[now.first]){
            if(!vis[y]) vis[y]=1,q.push(mp(y,now.second+1));
        }
    }
}
int main()
{
    int n,m,k;
    rrr(n,m,k);
    FOR(i,1,k) r(f[i]);
    FOR(i,1,m){
        int a,b;
        rr(a,b);
        v[a].pb(b);
        v[b].pb(a);
    }
    bfs(1,1);
    bfs(n,1);
    vector<pt> g;
    FOR(i,1,k){
        int x=f[i];
        g.pb(mp(fst[x]-lst[x],x));
    }
    sort(all(g));
    int ans=0;
    int maxy=-INF;
    for(auto x:g){
        //cout<
        ans=max(ans,maxy+lst[x.second]);
        maxy=max(maxy,fst[x.second]);
        //cout<
    }
    cout<<min(fst[n]-1,ans-1)<<endl;
    return 0;
}

---