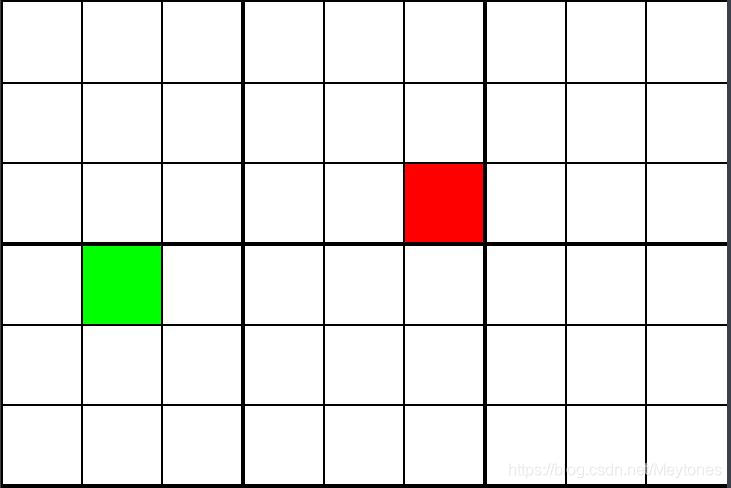
- UE求职Demo开发日志#21 背包-仓库-装备栏移动物品
学游戏开发的
UE求职Demo开发日志学习c++游戏引擎unrealengine笔记
1创建一个枚举记录来源位置UENUM(BlueprintType)enumclassEMyItemLocation:uint8{None=0,BagUMETA(DisplayName="Bag"),ArmedUMETA(DisplayName="Armed"),WareHouseUMETA(DisplayName="WareHouse"),};2创建一个BagPad和WarePad都有的UI虽然巨
- 【开发日志】数字人+LLM:从概念到实现的全程记录!
AI大模型-王哥
大模型学习大模型教程大模型人工智能LLM数字人大模型入门
数字人是各种技术的集合,所以文章尽可能完整的介绍,项目中涉及的大小模型均可在本地部署并在我本人机器上运行。系统环境:CPU:i91490016GBGPU:GTX40608GBSYS:Windows11WSL:Ubuntu22.04本文章使用到的技术内容:数字人框架:LiveTalking大模型:Llama3.1TTS:GPT-SoVits语音转视频:Wav2Lip前端展示:WebRTC项目整体架构
- 【从零开始入门unity游戏开发之——C#篇55】C#补充知识点——解构函数(Deconstruct)
向宇it
#unityc#游戏引擎编辑器开发语言
考虑到每个人基础可能不一样,且并不是所有人都有同时做2D、3D开发的需求,所以我把【零基础入门unity游戏开发】分为成了C#篇、unity通用篇、unity3D篇、unity2D篇。【C#篇】:主要讲解C#的基础语法,包括变量、数据类型、运算符、流程控制、面向对象等,适合没有编程基础的同学入门。【unity通用篇】:主要讲解unity的基础通用的知识,包括unity界面、unity脚本、unit
- python 应用开发日志工具包—— loguru
添财小哥
python应用开发pythonpip
一、简介Loguru是一个Python库,旨在让日志记录变得愉快。你是否曾因为懒得配置日志记录器而直接使用print()?…我有过,然而日志记录对于每个应用程序都是基本的,它简化了调试过程。使用Loguru,你没有理由不从一开始就使用日志记录,这就像导入fromloguruimportlogger一样简单。此外,这个库旨在通过添加一系列有用的功能来解决标准日志记录器的缺陷,从而减轻Python日志
- 【从零开始入门unity游戏开发之——C#篇46】C#补充知识点——命名参数和可选参数
向宇it
unityc#游戏引擎编辑器开发语言
考虑到每个人基础可能不一样,且并不是所有人都有同时做2D、3D开发的需求,所以我把【零基础入门unity游戏开发】分为成了C#篇、unity通用篇、unity3D篇、unity2D篇。【C#篇】:主要讲解C#的基础语法,包括变量、数据类型、运算符、流程控制、面向对象等,适合没有编程基础的同学入门。【unity通用篇】:主要讲解unity的基础通用的知识,包括unity界面、unity脚本、unit
- 【零基础入门unity游戏开发——unity通用篇36】向量(Vector3)的基本操作和运算(基于unity6开发介绍)
向宇it
unity游戏引擎3dc#开发语言
考虑到每个人基础可能不一样,且并不是所有人都有同时做2D、3D开发的需求,所以我把【零基础入门unity游戏开发】分为成了C#篇、unity通用篇、unity3D篇、unity2D篇。【C#篇】:主要讲解C#的基础语法,包括变量、数据类型、运算符、流程控制、面向对象等,适合没有编程基础的同学入门。【unity通用篇】:主要讲解unity的基础通用的知识,包括unity界面、unity脚本、unit
- 防撞仿真器开发日志-1.5.0版本新增的车道逻辑部分【2021.10.27】
海绵姥姥
防撞仿真器项目日志qtc++自动驾驶
目录1.新增了Lane车道类2.修改了接口类3.环境车部分的构想1.新增了Lane车道类车道类的数据有三部分:一是车道自身属性,包括位置和方向信息:起点、终点(弯道还有弧线圆心点)和道路交通信息:限速速度等;二是自身的标号索引,初步想了所在场景编号(用于表示所在场景之间的关系,可能今后在长路径规划时会用到)、场景内的车道编号(用于在场景内部车之间的变道和和掉头)、地图中的总车道号(在全局中可能有用
- Unity 2D Spine 外发光实现思路
Don里个冬
Unity3D技术分享unityspine游戏引擎图形渲染
Unity2DSpine外发光实现思路前言对于3D骨骼,要做外发光可以之间通过向法线方向延申来实现。但是对于2D骨骼,各顶点的法线没有向3D骨骼那样拥有垂直于面的特性,那我们如何做2D骨骼的外发光效果呢?理论基础我们要知道,要实现外发光效果,首先得先实现外描边效果。对于2D图片的描边实现有很多种方案。内描边:思路:对于任意像素,如果其四周存在透明像素,则说明是边缘。简单实现的效果如下图:这样的边缘
- 项目开发日志(登录页面):1. Header组件
博丽七七
项目开发日志(登录界面)前端vue
Header组件样式说明属性属性名含义类型是否必填默认值linkUrl图标点击后链接界面String否javascript:;(none)属性名含义类型是否必填默认值url图片路径String是无样式@mainColor->主题颜色代码exportdefault{props:{linkUrl:{type:String,require:false,default:"javascript:;"},ur
- 项目开发日志(登录界面):2. LoginTitle组件
博丽七七
项目开发日志(登录界面)css前端vue
LoginTitle组件样式说明属性属性名含义类型是否必填默认值welcomeTitle欢迎标语String是无mainTitle标题String是无样式@mainColor->主题颜色代码{{welcomeTitle}}{{mainTitle}}exportdefault{props:{welcomeTitle:{type:String,require:true,},mainTitle:{typ
- PHP开发日志 ━━ 深入理解三元操作与一般条件语句的不同
暂时先用这个名字
PHPphpandroid开发语言三元运算符三元if条件语句
概况三元运算符的功能与“if…else”流程语句一致。在一般情况下,三元操作替换if条件语句可以精简代码,并且更为直观,但是在下面的情况中使用三元操作将会返回警告。借图:案例比如原代码:classclassA{publicstaticfunction&get_instance($param=''){...if(isset(self::$_instance->$param)){returnself:
- Unity2D 基础 瓦片地图
Isaac_23ba
使用瓦片地图需要准备好你要使用的基础美术资源。1.导入也可以去Unity商店添加购买在unity中选择Window===》PackageManager===》左上角赛选项选MyAssets找到你要的使用的资源选择Download或import2.图片的处理和切割选择你要处理的图片在inspection中,选中SpriteEditor瓦片地图画板的入口Window===》2D===》TitlePal
- 我的实用小工具-日志采集模块
写给朕阅
python文章头图.png一、日志对于一个开发者来说的重要性开发日志记录对开发者来说至关重要。好的日志记录可以帮助开发者在代码开发和维护过程中发现问题、解决问题以及回顾开发思路。首先,日志可以帮助开发者调试和发现代码中的问题。通过在代码的关键部分加入日志,可以打印出变量的值、函数的入参和返回值等信息。这些信息可以帮助开发者分析问题所在,快速定位和修复bug。其次,日志还可以帮助开发者理清思路、回
- Unity2D 学习笔记 0.Unity需要记住的常用知识
Lyrig~
Unity学习c#unity
Unity2D学习笔记0.Unity需要记住的常用知识前言调整ProjectSettingTilemap相关(创建地图块)C#脚本相关程序运行函数privatevoidAwake()voidStart()voidUpdate()Collider2D碰撞检测privatevoidOnTriggerStay2D(Collider2Dplayer)privatevoidOnTriggerEnter2D(
- 彩超框架EchoSight开发日志记录
Z_Jiang
医疗超声彩超超声系统工程框架超声信号链路EchoSight
EchoSight开发记录作者:蒋志强不定期更新EchoSight开发日志记录,最近更新于2024年02月02日1.背景由于某些不可抗逆的原因,离开了以前的彩超大厂,竞业在家,难得有空闲的时间。我计划利用这段时间自己独立从零开始搭建一套彩超系统的软件工程框架。这个框架的设计水准力求达到目前商业级产品中第一梯队的水平。这套工程框架,我暂时取名为EchoSight。Echo表示声音/回声,Sight本
- 【原神游戏开发日志5】同地图多客户端玩家同步
优梦创客
Unity游戏开发c#
版权声明:●本文为“优梦创客”原创文章,您可以自由转载,但必须加入完整的版权声明●文章内容不得删减、修改、演绎●本文视频版本:见文末●相关学习资源:见文末前言●今天给大家分享选角色、进游戏打包功能展示登录注册●打开已经打包好的原神项目●项目的业务逻辑都支持热更新●等待热更新和启动资源加载完毕●这个项目是一款网络游戏●当你看到登录画面的时候●它是真的在进行网络通信●首次进入游戏需要先注册●然后点击登
- Unity2D_单向平台
Rainbow_1991
Unity2Dunity
类似空洞骑士马里奥等各种2D游戏,其中都存在单向平台。单向平台:角色可以从下穿过平台停在平台上,也可以从平台上按下或往下跳穿过平台这里用Unity实现角色能跳上平台,也能按’下’穿过平台平台需要使用Rigidbody2DCollider2DPlatformEffector2D组件Collider2D中的UsedByEffector属性需要为truePlatformEffector2D中的UseOn
- unity2D 物体移动以及多段跳跃
我天天哇
unity
针对实现在Unity中对2D物体的移动以及多端跳跃的实现在移动方面采用Translate()方法直接移动物体位在跳跃方面使用两个bool变量jumpTime和jumpTimes控制跳跃时间和跳跃次数代码如下://定义物体是否在地面状态privateboolisGrounded;//定义物体脚下位置publicTransformfeetPos;//定义碰撞检测范围publicfloatcheckRa
- unity2D:角色左右移动、跳跃
Karon_NeverAlone
unity2Dunity
移动1.获取左右移动信息Input.GetAxis("Horizontal");//返回值为-1~1Input.GetAxisRaw("Horizontal");//返回值为-1、0、12.修改刚体的velocity,如果是水平移动就修改x轴,相对应的,如果是前后移动就修改y轴_myrb.velocity=newVector2(_myhorizontalmove*Speed*Time.deltaT
- Unity2D_角色移动&跳跃
Rainbow_1991
Unity2Dunity
水平移动Rigidbody2D使用Unity自带的刚体组件,通过修改刚体物品中Rigidbody2D的属性velocity速度来实现物体移动声明Rigidbody2D属性,以及角色移动速度publicfloatplayerSpeed=5f;privateRigidbody2Drigidbody2D;在Start方法中将rigidbody2D绑定上物体的对应组件voidStart(){rigidbo
- (M)unity2D敌人的创建、人物属性设置,遇敌掉血
MIMO. mimo
unity
敌人的创建1.敌人添加与组件设置1)添加敌人后,刚体添加,碰撞体添加(一个碰撞体使猪在地上走,不接触人,另一个碰撞体组件使人和猪碰在一起产生伤害)①刚体②碰撞体一设置的只在脚下,只需要敌人可以站在场景中就可以但存在添加碰撞体积后,会和其他移动的人物或敌人产生碰撞使得无法擦肩而过的效果,通过组件中的layeroverrides解决敌人与player碰撞问题,通过触发器trigger解决人物碰到猪以后
- 【Unity2D】实现人物死亡动画+代码
dangoxiba
动画c#visualstudiounityvisualstudio
学习目标:实现人物死亡动画+代码参考视频:秦无邪OvO的个人空间_哔哩哔哩_Bilibili秦无邪OvO,独立游戏开发者/美术/编曲;秦无邪OvO的主页、动态、视频、专栏、频道、收藏、订阅等。哔哩哔哩Bilibili,你感兴趣的视频都在B站。https://space.bilibili.com/335835274?from=search&seid=4530647365121956206&spm_i
- 【原神游戏开发日志2】用户注册
优梦创客
游戏开发MMORPGUnity原神网络游戏框架知识
【原神游戏开发日志2】用户注册版权声明本文为“优梦创客”原创文章,您可以自由转载,但必须加入完整的版权声明文章内容不得删减、修改、演绎相关学习资源见文末大家好,今天继续更新原神游戏开发本次学习的目标:完成原神游戏中用户账户注册的功能以下是任务目标和步骤说明:数据加载完毕才显示LoginPanel实现“注册”功能客户端引入“MMO训练营”写好的客户端部分的Program类的代码(网络框架代码)在Lo
- 【原神游戏开发日志3】登录和注册有何区别?
优梦创客
Unity使用Unity教程游戏开发游戏开发MMORPGunity原神网络游戏框架ARPG
版权声明:●本文为“优梦创客”原创文章,您可以自由转载,但必须加入完整的版权声明●文章内容不得删减、修改、演绎●本文视频版本:见文末●相关学习资源:见文末前言●这是我们原神游戏开发日记的第三期●在前一期的开发日记当中●分享了开发原神用户注册模块的过程●在本期将接着给大家分享开发登陆模块的过程●可能很多人会疑惑登录和注册功能逻辑不是差不多吗○从开发者的角度来说○注册是判读用户是否不存在○登录是判读用
- Unity2D新手教程[安装和创建项目]
IoOozZzzz
Unity2D新手教程unity
1.教程简介本教程是YouTube大佬教程视频的随笔,目前正在更新中...教程地址[YouTube](https://www.youtube.com/watch?v=XtQMytORBmM)2.安装本教程使用的Unity版本2021.3.34f1,安装好该LTS(长期支持)版本之后,在左侧Install选项卡Tab中选择对应版本右边的齿轮,单机后在菜单栏中选择Addmodules,然后会弹出一个窗
- Unity2D中逐帧动画的制作
翼風
unity游戏
Unity2D中图片序列帧动画的制作0.前言由于我们正在开发一个2D游戏,动画是非常容易的。我们制作动画的步骤分为两步:导入图片与创建动画。1.导入图片这里以植物大战僵尸里寒冰射手的图片为例。注意:右击图像,选择另存为。并将其保存在项目的Assets/Sprites文件夹。导入设置点击SpriteEditor,然后使用以下设置对其进行切片:将其中的type改为size,并将下面的size改为32,
- 简单的unity练习----打地鼠 学习笔记
afrecon
打地鼠是一款非常简单的小游戏,但是它能让我们熟悉unity的大部分操作。创建并导入素材首先创建一个unity2D工程,并导入所有素材,将素材拖入scene窗口,依据maincarema的范围调整素材大小出现地鼠创建一个空物体gameobject,用于加载create地鼠代码,利用random.range来产生随机数,使地鼠在九个位置随机产生usingUnityEngine;publicclassc
- 抖音弹幕直播玩法汉字找不同文字找不同无人值执守自动玩游戏自带语音播报的开发日志
小和尚敲代码
玩游戏自动玩游戏animejspreloadjs
#找不同#要解决如下几个问题:1.声音sprite的录制和调用,解决方案以及解决库如下:howler.min.js://一款不错的音频播放js库。2.鼠标自动飘浮,使用的库anime.min.js3.资源预加载preload.min.js4.其它使用到的库jquery,vue
- 使用 ngrok 进行内网穿透
HOLD ON!
使用ngrok进行内网穿透2018年09月04日19:51:59一叶之秋Tel阅读数2409更多个人分类:Linux环境搭建开发日志版权声明:本文为博主原创文章,未经博主允许不得转载。https://blog.csdn.net/guoqian1408/article/details/82388642革命的果实最香甜~~劳动的人民最光荣~~经过一下午的鼓捣,终于实现了ngrok内网穿透部署软件需求G
- Unity文字游戏开发日志(2)——存档与读档
鹤上听雷
unity游戏引擎
存档与读档较为简单的实现今天学习了如何存读档。采用了Unity自带的方式PlayerPrefs写了一个示例代码功能是:建立一个名字的新档,每次打开游戏名字都会变。PlayerPrefs.SetString("save","kkk");//创建名为save数据,存一个字符串kkkPlayerPrefs.GetString("save");;//访问PlayerPrefs.HasKey("save")
- [黑洞与暗粒子]没有光的世界
comsci
无论是相对论还是其它现代物理学,都显然有个缺陷,那就是必须有光才能够计算
但是,我相信,在我们的世界和宇宙平面中,肯定存在没有光的世界....
那么,在没有光的世界,光子和其它粒子的规律无法被应用和考察,那么以光速为核心的
&nbs
- jQuery Lazy Load 图片延迟加载
aijuans
jquery
基于 jQuery 的图片延迟加载插件,在用户滚动页面到图片之后才进行加载。
对于有较多的图片的网页,使用图片延迟加载,能有效的提高页面加载速度。
版本:
jQuery v1.4.4+
jQuery Lazy Load v1.7.2
注意事项:
需要真正实现图片延迟加载,必须将真实图片地址写在 data-original 属性中。若 src
- 使用Jodd的优点
Kai_Ge
jodd
1. 简化和统一 controller ,抛弃 extends SimpleFormController ,统一使用 implements Controller 的方式。
2. 简化 JSP 页面的 bind, 不需要一个字段一个字段的绑定。
3. 对 bean 没有任何要求,可以使用任意的 bean 做为 formBean。
使用方法简介
- jpa Query转hibernate Query
120153216
Hibernate
public List<Map> getMapList(String hql,
Map map) {
org.hibernate.Query jpaQuery = entityManager.createQuery(hql);
if (null != map) {
for (String parameter : map.keySet()) {
jp
- Django_Python3添加MySQL/MariaDB支持
2002wmj
mariaDB
现状
首先,
[email protected] 中默认的引擎为 django.db.backends.mysql 。但是在Python3中如果这样写的话,会发现 django.db.backends.mysql 依赖 MySQLdb[5] ,而 MySQLdb 又不兼容 Python3 于是要找一种新的方式来继续使用MySQL。 MySQL官方的方案
首先据MySQL文档[3]说,自从MySQL
- 在SQLSERVER中查找消耗IO最多的SQL
357029540
SQL Server
返回做IO数目最多的50条语句以及它们的执行计划。
select top 50
(total_logical_reads/execution_count) as avg_logical_reads,
(total_logical_writes/execution_count) as avg_logical_writes,
(tot
- spring UnChecked 异常 官方定义!
7454103
spring
如果你接触过spring的 事物管理!那么你必须明白 spring的 非捕获异常! 即 unchecked 异常! 因为 spring 默认这类异常事物自动回滚!!
public static boolean isCheckedException(Throwable ex)
{
return !(ex instanceof RuntimeExcep
- mongoDB 入门指南、示例
adminjun
javamongodb操作
一、准备工作
1、 下载mongoDB
下载地址:http://www.mongodb.org/downloads
选择合适你的版本
相关文档:http://www.mongodb.org/display/DOCS/Tutorial
2、 安装mongoDB
A、 不解压模式:
将下载下来的mongoDB-xxx.zip打开,找到bin目录,运行mongod.exe就可以启动服务,默
- CUDA 5 Release Candidate Now Available
aijuans
CUDA
The CUDA 5 Release Candidate is now available at http://developer.nvidia.com/<wbr></wbr>cuda/cuda-pre-production. Now applicable to a broader set of algorithms, CUDA 5 has advanced fe
- Essential Studio for WinRT网格控件测评
Axiba
JavaScripthtml5
Essential Studio for WinRT界面控件包含了商业平板应用程序开发中所需的所有控件,如市场上运行速度最快的grid 和chart、地图、RDL报表查看器、丰富的文本查看器及图表等等。同时,该控件还包含了一组独特的库,用于从WinRT应用程序中生成Excel、Word以及PDF格式的文件。此文将对其另外一个强大的控件——网格控件进行专门的测评详述。
网格控件功能
1、
- java 获取windows系统安装的证书或证书链
bewithme
windows
有时需要获取windows系统安装的证书或证书链,比如说你要通过证书来创建java的密钥库 。
有关证书链的解释可以查看此处 。
public static void main(String[] args) {
SunMSCAPI providerMSCAPI = new SunMSCAPI();
S
- NoSQL数据库之Redis数据库管理(set类型和zset类型)
bijian1013
redis数据库NoSQL
4.sets类型
Set是集合,它是string类型的无序集合。set是通过hash table实现的,添加、删除和查找的复杂度都是O(1)。对集合我们可以取并集、交集、差集。通过这些操作我们可以实现sns中的好友推荐和blog的tag功能。
sadd:向名称为key的set中添加元
- 异常捕获何时用Exception,何时用Throwable
bingyingao
用Exception的情况
try {
//可能发生空指针、数组溢出等异常
} catch (Exception e) {
- 【Kafka四】Kakfa伪分布式安装
bit1129
kafka
在http://bit1129.iteye.com/blog/2174791一文中,实现了单Kafka服务器的安装,在Kafka中,每个Kafka服务器称为一个broker。本文简单介绍下,在单机环境下Kafka的伪分布式安装和测试验证 1. 安装步骤
Kafka伪分布式安装的思路跟Zookeeper的伪分布式安装思路完全一样,不过比Zookeeper稍微简单些(不
- Project Euler
bookjovi
haskell
Project Euler是个数学问题求解网站,网站设计的很有意思,有很多problem,在未提交正确答案前不能查看problem的overview,也不能查看关于problem的discussion thread,只能看到现在problem已经被多少人解决了,人数越多往往代表问题越容易。
看看problem 1吧:
Add all the natural num
- Java-Collections Framework学习与总结-ArrayDeque
BrokenDreams
Collections
表、栈和队列是三种基本的数据结构,前面总结的ArrayList和LinkedList可以作为任意一种数据结构来使用,当然由于实现方式的不同,操作的效率也会不同。
这篇要看一下java.util.ArrayDeque。从命名上看
- 读《研磨设计模式》-代码笔记-装饰模式-Decorator
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
import java.io.BufferedOutputStream;
import java.io.DataOutputStream;
import java.io.FileOutputStream;
import java.io.Fi
- Maven学习(一)
chenyu19891124
Maven私服
学习一门技术和工具总得花费一段时间,5月底6月初自己学习了一些工具,maven+Hudson+nexus的搭建,对于maven以前只是听说,顺便再自己的电脑上搭建了一个maven环境,但是完全不了解maven这一强大的构建工具,还有ant也是一个构建工具,但ant就没有maven那么的简单方便,其实简单点说maven是一个运用命令行就能完成构建,测试,打包,发布一系列功
- [原创]JWFD工作流引擎设计----节点匹配搜索算法(用于初步解决条件异步汇聚问题) 补充
comsci
算法工作PHP搜索引擎嵌入式
本文主要介绍在JWFD工作流引擎设计中遇到的一个实际问题的解决方案,请参考我的博文"带条件选择的并行汇聚路由问题"中图例A2描述的情况(http://comsci.iteye.com/blog/339756),我现在把我对图例A2的一个解决方案公布出来,请大家多指点
节点匹配搜索算法(用于解决标准对称流程图条件汇聚点运行控制参数的算法)
需要解决的问题:已知分支
- Linux中用shell获取昨天、明天或多天前的日期
daizj
linuxshell上几年昨天获取上几个月
在Linux中可以通过date命令获取昨天、明天、上个月、下个月、上一年和下一年
# 获取昨天
date -d 'yesterday' # 或 date -d 'last day'
# 获取明天
date -d 'tomorrow' # 或 date -d 'next day'
# 获取上个月
date -d 'last month'
#
- 我所理解的云计算
dongwei_6688
云计算
在刚开始接触到一个概念时,人们往往都会去探寻这个概念的含义,以达到对其有一个感性的认知,在Wikipedia上关于“云计算”是这么定义的,它说:
Cloud computing is a phrase used to describe a variety of computing co
- YII CMenu配置
dcj3sjt126com
yii
Adding id and class names to CMenu
We use the id and htmlOptions to accomplish this. Watch.
//in your view
$this->widget('zii.widgets.CMenu', array(
'id'=>'myMenu',
'items'=>$this-&g
- 设计模式之静态代理与动态代理
come_for_dream
设计模式
静态代理与动态代理
代理模式是java开发中用到的相对比较多的设计模式,其中的思想就是主业务和相关业务分离。所谓的代理设计就是指由一个代理主题来操作真实主题,真实主题执行具体的业务操作,而代理主题负责其他相关业务的处理。比如我们在进行删除操作的时候需要检验一下用户是否登陆,我们可以删除看成主业务,而把检验用户是否登陆看成其相关业务
- 【转】理解Javascript 系列
gcc2ge
JavaScript
理解Javascript_13_执行模型详解
摘要: 在《理解Javascript_12_执行模型浅析》一文中,我们初步的了解了执行上下文与作用域的概念,那么这一篇将深入分析执行上下文的构建过程,了解执行上下文、函数对象、作用域三者之间的关系。函数执行环境简单的代码:当调用say方法时,第一步是创建其执行环境,在创建执行环境的过程中,会按照定义的先后顺序完成一系列操作:1.首先会创建一个
- Subsets II
hcx2013
set
Given a collection of integers that might contain duplicates, nums, return all possible subsets.
Note:
Elements in a subset must be in non-descending order.
The solution set must not conta
- Spring4.1新特性——Spring缓存框架增强
jinnianshilongnian
spring4
目录
Spring4.1新特性——综述
Spring4.1新特性——Spring核心部分及其他
Spring4.1新特性——Spring缓存框架增强
Spring4.1新特性——异步调用和事件机制的异常处理
Spring4.1新特性——数据库集成测试脚本初始化
Spring4.1新特性——Spring MVC增强
Spring4.1新特性——页面自动化测试框架Spring MVC T
- shell嵌套expect执行命令
liyonghui160com
一直都想把expect的操作写到bash脚本里,这样就不用我再写两个脚本来执行了,搞了一下午终于有点小成就,给大家看看吧.
系统:centos 5.x
1.先安装expect
yum -y install expect
2.脚本内容:
cat auto_svn.sh
#!/bin/bash
- Linux实用命令整理
pda158
linux
0. 基本命令 linux 基本命令整理
1. 压缩 解压 tar -zcvf a.tar.gz a #把a压缩成a.tar.gz tar -zxvf a.tar.gz #把a.tar.gz解压成a
2. vim小结 2.1 vim替换 :m,ns/word_1/word_2/gc
- 独立开发人员通向成功的29个小贴士
shoothao
独立开发
概述:本文收集了关于独立开发人员通向成功需要注意的一些东西,对于具体的每个贴士的注解有兴趣的朋友可以查看下面标注的原文地址。
明白你从事独立开发的原因和目的。
保持坚持制定计划的好习惯。
万事开头难,第一份订单是关键。
培养多元化业务技能。
提供卓越的服务和品质。
谨小慎微。
营销是必备技能。
学会组织,有条理的工作才是最有效率的。
“独立
- JAVA中堆栈和内存分配原理
uule
java
1、栈、堆
1.寄存器:最快的存储区, 由编译器根据需求进行分配,我们在程序中无法控制.2. 栈:存放基本类型的变量数据和对象的引用,但对象本身不存放在栈中,而是存放在堆(new 出来的对象)或者常量池中(字符串常量对象存放在常量池中。)3. 堆:存放所有new出来的对象。4. 静态域:存放静态成员(static定义的)5. 常量池:存放字符串常量和基本类型常量(public static f