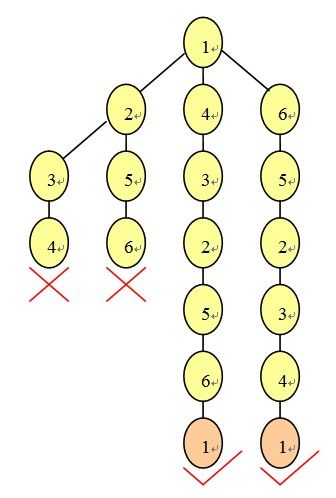
- 算法设计与分析期末复习题汇总
wisdom_zhe
Java题库算法
文章目录1、选择题1.1选择题11.2选择题22、判断题2.1判断题12.2判断题23、填空题3.1算法填空3.2填空题24、简答题1、选择题1.1选择题11、下列不是动态规划算法基本步骤的是(A)。A、找出最优解的解空间B、构造最优解C、算出最优解D、定义最优解2、最大效益优先是(A)的一搜索方式。A、分支界限法B、动态规划法C、贪心法D、回溯法3、最长公共子序列算法利用的算法是(B)。A、分支
- 将长度为n的数组分割成m个子数组的所有情况(JAVA实现)
一夏之风
将长度为n的数组分隔成m个子数组,可以看作是将m-1个分隔符插入原来数组的n-1个位置中,所以我们只需要求出这m-1个分隔符在原来数组中的下标索引,即可得到子数组的所有情形。所以问题就转换成在n-1个位置中寻找m-1个分隔符,一共有C_(n-1)(m-1)种情况,我们采用回溯法来生成所有情形:importjava.util.ArrayList;importjava.util.List;/*把一个长
- 回溯——1.组合
plutomty
算法
给定两个整数n和k,返回1...n中所有可能的k个数的组合。示例:输入:n=4,k=2输出:[[2,4],[3,4],[2,3],[1,2],[1,3],[1,4],]解题思路总结回溯法是一种非常有效的组合生成策略。它通过在路径中添加元素,并不断递归深入,再回溯撤销操作,确保能够找到所有可能的组合。优化点在于通过控制循环的范围,避免了不必要的计算,从而提升了算法的效率。通过提前计算可以填入组合的数
- 【动态规划】【打卡121天】:背包理论基础
晓风残月一望关河萧索
【算法】
1、背包理论基础有N件物品和一个最多能背重量为W的背包。第i件物品的重量是weight[i],得到的价值是value[i]。每件物品只能用一次,求解将哪些物品装入背包里物品价值最大。其实这是标准的背包问题。每一件物品有2种状态,取物品放入背包中,不取该物品放入背包中。所以可以使用回溯法搜索出所有的情况,那么时间复杂度就是O(2^n),这里的n表示物品数量。2、算法分析①确定dp数组以及下标的含义对
- Java 算法-背包问题 VI(动态规划)
琼珶和予
今天做了一道背包问题的变种问题,这个问题还是用动态规划来做,但是做法上跟原来的背包问题有很大的区别。题意给出一个都是正整数的数组nums,其中没有重复的数。从中找出所有的和为target的组合个数。样例给出nums=[1,2,4],target=4可能的所有组合有:[1,1,1,1][1,1,2][1,2,1][2,1,1][2,2][4]返回61.最简单的方法--回溯法(超时) 看到这种问
- LeetCode216 组合总和 III
红毛乌龟
算法刷题数据结构算法leetcodec++
前言题目:216.组合总和III文档:代码随想录——组合总和III编程语言:C++解题状态:成功解答思路组合题的变种,其实是变得更简单了,还是可以使用回溯法来解决。代码回溯法classSolution{private:vector>res;vectorpath;voidbacktracking(intk,intn,intstartIndex){if(path.size()==k){intsum=0
- 代码随想录算法训练营第二十二天 | 回溯理论基础、77. 组合、216.组合总和III、17.电话号码的字母组合
Cedric7
代码随想录算法训练营算法
一、回溯理论基础文章讲解:代码随想录(programmercarl.com)——回溯理论基础视频讲解:带你学透回溯算法(理论篇)|回溯法精讲!_哔哩哔哩_bilibili1.解决问题(1)组合问题:N个数里面按一定规则找出k个数的集合;(2)切割问题:给一个字符串,问右几种切割方式;(3)子集问题:N个数有多少个符合条件的子集;(4)排列问题:强调元素顺序;(5)棋盘问题:N皇后,解数独等。2.如
- LeetCode77 组合
红毛乌龟
算法刷题算法数据结构leetcodec++
前言题目:77.组合文档:代码随想录——组合编程语言:C++解题状态:没尝试出来思路经典的组合问题,可以考虑使用回溯法。使用回溯法时可以根据回溯法的模板来考虑如何解决。代码回溯法classSolution{private:vector>res;vectorpath;voidbacktracking(intn,intk,intstartIndex){if(path.size()==k){res.pu
- Java回溯知识点(含面试大厂题和源码)
一成码农
java面试开发语言
回溯算法是一种通过遍历所有可能的候选解来寻找所有解的算法,如果候选解被确认不是一个解(或至少不是最后一个解),回溯算法会通过在上一步进行一些变化来丢弃这个解,即“回溯”并尝试另一个候选解。回溯法通常用递归方法来实现,在解决排列、组合、选择问题时非常有效。回溯算法的核心要点:路径:也就是已经做出的选择。选择列表:也就是你当前可以做的选择。结束条件:也就是到达决策树底层,无法再做出选择的条件。回溯算法
- 重拾C++之菜鸟刷算法第11篇---回溯算法(上)
阿卡西番茄酱
C++算法算法c++开发语言
今天是个好日子,二月二龙抬头,龙年龙日龙抬头,顺风顺水好兆头,万事万物开好头,金银珠宝往家里走!offer往家走!回溯算法回溯法可以解决的问题组合问题:N个数里面按照一定规则找出k个数的集合切割问题:一个字符串按一定规则有几种切割方式子集问题:一个N个数的集合里有多少符合条件的子集排列问题:N个数按一定规则全排列,有几种排列方式棋盘问题:N皇后,解数独回溯三部曲回溯函数模板返回值以及参数回溯函数终
- 代码随想录算法训练营三刷day24 | 回溯算法 之 理论基础 77. 组合
頔枫
算法训练营算法数据结构c++leetcode
三刷day24理论基础77.组合递归函数的返回值以及参数回溯函数终止条件单层搜索的过程理论基础回溯法解决的问题都可以抽象为树形结构。因为回溯法解决的都是在集合中递归查找子集,集合的大小就构成了树的宽度,递归的深度,都构成的树的深度。递归就要有终止条件,所以必然是一棵高度有限的树(N叉树)。回溯三部曲回溯函数模板返回值以及参数在回溯算法中,我的习惯是函数起名字为backtracking,这个起名大家
- 回溯法与分支限界法(以0-1背包问题为例)
Loading_Reparo.
算法数据结构算法
回溯法与分支限界法(以0-1背包问题为例)回溯法总体上概述来讲:回溯法:原则上依据深度优先遍历的寻找方式,每次都是一直深入寻找的过程,只不过在这个深入的过程中,我们限定一定的上界条件,可以实现事先判断该条深入路线是否可以得到目前情况下的一个比我们手上有的最优值要优的或者是压根这条路得到的值明显离我们的期望值差的很远的,可以直接舍弃。舍弃后便会发生回溯,重新选择一条路继续探索直到到达叶子节点。(这里
- leetcode51 N皇后问题
浦东新村轱天乐
leetcode算法数据结构
https://programmercarl.com/0051.N%E7%9A%87%E5%90%8E.html代码随想录讲的很清楚。回溯法从上到下按行搜索,因此back_tracking(chessboard,row+1)其参数为row+1判断该位置是否符合终止条件是i==nclassSolution{public://vectorpath_;vector>res_;boolvalid(vect
- 【leetcode热题】分割回文串
kiugvui
leetcode热题leetcode算法职场和发展
难度:中等通过率:38.7%题目链接:.-力扣(LeetCode)题目描述给定一个字符串s,将s分割成一些子串,使每个子串都是回文串。返回s所有可能的分割方案。示例:输入: "aab"输出:[["aa","b"],["a","a","b"]]解法:直接采用回溯法暴力搜索即可。classSolution:defpartition(self,s:str)->List[List[str]]:result
- 回溯法详解以及剪枝优化 以leetcode 77.组合问题为例
学不完了ccccc
leetcodec++算法数据结构
题目描述回溯法的简单介绍回溯法是为了一类特定的问题概括起来就是集合里面根据要求搜索集合(不止一个),拿leetcode77.来举例,可以用多层for循环进行构思,但是要多少层呢,同时层数太多会导致时间复杂度急剧增高。因此回溯法出现可以使得这个难度降低可以解决以下几类问题:个人感觉回溯法的过程是下面这样的例在1,2,3,4这个集合中找到元素个数为2的集合这个在数学上就是一个组合问题我们在手动模拟计算
- leetcode77组合 剪枝条件详细解释
猫鱼Ω
leetcode刷题笔记剪枝算法回溯代码随想录
题目:77.组合-力扣(LeetCode)题解:力扣(LeetCode)官网-全球极客挚爱的技术成长平台思路来自代码随想录:带你学透回溯算法-组合问题(对应力扣题目:77.组合)|回溯法精讲!_哔哩哔哩_bilibili带你学透回溯算法-组合问题的剪枝操作(对应力扣题目:77.组合)|回溯法精讲!_哔哩哔哩_bilibili对其中的剪枝条件做详细解释剪枝部分代码为for(inti=index;i=
- 代码随想录算法训练营day17|Leetcode110/257/404
yrrej0
算法leetcode职场和发展python数据结构
Leetcode257二叉树的所有路径链接:257.二叉树的所有路径-力扣(LeetCode)给你一个二叉树的根节点root,按任意顺序,返回所有从根节点到叶子节点的路径。叶子节点是指没有子节点的节点。思路:递归+回溯法这里有两种方法。第一:两层for循环,分别遍历左子树和右子树。特例:如果只有1个root,那么可以直接添加到结果集种[str(root)],注意在添加的过程中要对应上lettcod
- leetcode-140. 单词拆分 II (字典树/dp + 回溯法) + 字节测开字典树算法题
Anpedestrian
字典树leetcode之路
给定一个非空字符串s和一个包含非空单词列表的字典wordDict,在字符串中增加空格来构建一个句子,使得句子中所有的单词都在词典中。返回所有这些可能的句子。说明:分隔时可以重复使用字典中的单词。你可以假设字典中没有重复的单词。示例1:输入:s="catsanddog"wordDict=["cat","cats","and","sand","dog"]输出:["catsanddog","catsan
- 77. 组合
ToxicantC
算法leetcode
给定两个整数n和k,返回范围[1,n]中所有可能的k个数的组合。你可以按任何顺序返回答案。示例1:输入:n=4,k=2输出:[[2,4],[3,4],[2,3],[1,2],[1,3],[1,4],]示例2:输入:n=1,k=1输出:[[1]]将上述组合问题画成一个多叉树,回溯法搜索就是树型结构的搜索过程,for循环控制横向遍历,用递归来控制纵向遍历,path的大小等于k的时候跳出递归classS
- 【LeetCode-494】目标和(回溯&动归)
叮咚Zz
leetcode算法职场和发展回归动态规划java
目录LeetCode494.目标和题目描述解法1:回溯法代码实现解法2:动态规划代码实现LeetCode494.目标和题目链接题目描述给定一个非负整数数组,a1,a2,...,an,和一个目标数,S。现在你有两个符号+和-。对于数组中的任意一个整数,你都可以从+或-中选择一个符号添加在前面。返回可以使最终数组和为目标数S的所有添加符号的方法数。示例:输入:nums:[1,1,1,1,1],S:3输
- 代码随想录 Day-30 回溯总结篇
1234567今天你有没有摸鱼
pythonleetcode
回溯法理论基础回溯是递归的副产品,有递归就会有回溯回溯法就是暴力搜索回溯法能解决(组合问题/排列问题/切割问题/子集问题/棋盘问题)回溯三部曲:1)递归函数的返回值和参数2)确认终止条件3)单层搜索过程(可剪枝优化)组合问题:1)一个集合来求组合,需要startIndex2)多个集合取组合,各个集合之间相互不影响,不用startIndex3)当集合元素会有重复,但要求解集不能包含重复的组合时,使用
- 代码随想录day21--回溯基础
诗以言志659
数据结构算法
理论基础回溯法也可以叫回溯搜索法,它是一种搜索的方式。我们在二叉树中也多次提到了回溯。回溯是递归的副产品,只要使用了递归就会有回溯,我们我们就可以笼统的将回溯函数称为递归函数回溯法解决的问题1.组合问题:N个数里面按一定规则找出k个数的集合2.切割问题:一个字符串按一定规则有几种切割方式3.子集问题:一个N个数的集合里有多少符合条件的子集4.排列问题:N个数按一定规则全排列,有几种排列方式5.棋盘
- 代码随想录Day18-LeetCode 513 & 112 & 106
阿林-.-
leetcode算法职场和发展
513-找树左下角的值题目链接:LeetCode-513思考:最后层左下角值,左下角不一定是左下角,得先满足最后一行才行,达到深度最大,左下角的值不一定是左孩子,也可以是右孩子,优先遍历左侧就行方法一:回溯法classSolution{public:intmaxLen=INT_MIN;intmaxleftValue;voidtraversal(TreeNode*root,intleftLen){i
- 代码随想录Day 24 - 回溯
sweet_cream
代码随想录算法c++leetcode
代码随想录Day24-回溯理论基础77.组合剪枝216.组合总和III剪枝理论基础回溯法解决的问题都可以抽象为树形结构。回溯法解决的都是在集合中递归查找子集,集合的大小就构成了树的宽度,递归的深度,都构成的树的深度。77.组合给定两个整数n和k,返回范围[1,n]中所有可能的k个数的组合。你可以按任何顺序返回答案。n相当于树的宽度,k相当于树的深度.classSolution{List>resul
- 回溯法解决P2089 烤鸡问题太香啦,组合问题就用回溯法就对了!
remember_me.
代码随想录回溯算法java
学回溯的第二天,发现之前做过的一道洛谷的枚举题也可以用回溯法去解决,还是相当滴nice的。先来看看leetcode上的这两道题216.组合总和III题目链接:216.组合总和III思路就是比组合问题多了一个和为n的限制,大体还是可以按模板来的,代码如下:代码:classSolution{Listtemp=newArrayList>result=newArrayList>combinationSum
- 代码随想录day21 Java版
洒水水儿
代码随想录打卡算法
过完年开始刷回溯算法,寒假在家时间多点,争取每天多刷点题回溯的本质是穷举,穷举所有可能,然后选出我们想要的答案。通常是解决复杂的题。回溯法解决的问题都可以抽象为树形结构,因为回溯法解决的都是在集合中递归查找子集,集合的大小就构成了树的宽度,递归的深度,都构成的树的深度。模板voidbacktracking(参数){if(终止条件){存放结果;return;}for(选择:本层集合中元素(树中节点孩
- 代码随想录刷题笔记 DAY 24 | 回溯算法理论基础 | 组合问题 No. 77
*Soo_Young*
代码随想录笔记算法
文章目录Day2401.回溯算法理论基础1.1什么是回溯法?1.2为什么要使用回溯法?1.3如何理解回溯法?02.组合问题(No.77)2.1题目2.2笔记2.3代码Day2401.回溯算法理论基础1.1什么是回溯法?递归函数的下面就是回溯的逻辑(有递归就有回溯)递归函数的后面位置其实就是平时提到的后序位置也就是当前这个节点做完所有的操作返回上一个节点的时候,这时候对这个节点可以通过某些逻辑做回溯
- 【leetcode热题100】子集 II
kiugvui
leetcode热题100leetcode算法职场和发展
给你一个整数数组nums,其中可能包含重复元素,请你返回该数组所有可能的子集(幂集)。解集不能包含重复的子集。返回的解集中,子集可以按任意顺序排列。示例1:输入:nums=[1,2,2]输出:[[],[1],[1,2],[1,2,2],[2],[2,2]]示例2:输入:nums=[0]输出:[[],[0]]解法一回溯法这个比较好改,我们只需要判断当前数字和上一个数字是否相同,相同的话跳过即可。当然
- LeetCode Hot100 15.三数之和
Daydreaming Kid
Leetcode刷题之路leetcode算法深度优先
文章目录题目描述回溯法(解不了,超时了,参考下剪枝)双指针循环题目描述给你一个整数数组nums,判断是否存在三元组[nums[i],nums[j],nums[k]]满足i!=j、i!=k且j!=k,同时还满足nums[i]+nums[j]+nums[k]==0。请你返回所有和为0且不重复的三元组。注意答案中不可以包含重复的三元组。示例1:输入:nums=[-1,0,1,2,-1,-4]输出:[[-
- 动态规划-背包问题
robes knight
动态规划算法
背包问题总结后期写背包问题介绍1.01背包有n件物品和一个最多能背重量为w的背包。第i件物品的重量是weight[i],得到的价值是value[i]。每件物品只能用一次,求解将哪些物品装入背包里物品价值总和最大。每一件物品其实只有两个状态,取或者不取,所以可以使用回溯法搜索出所有的情况,那么时间复杂度就是o(2^n),这里的n表示物品数量。所以暴力的解法是指数级别的时间复杂度。进而才需要动态规划的
- Java 并发包之线程池和原子计数
lijingyao8206
Java计数ThreadPool并发包java线程池
对于大数据量关联的业务处理逻辑,比较直接的想法就是用JDK提供的并发包去解决多线程情况下的业务数据处理。线程池可以提供很好的管理线程的方式,并且可以提高线程利用率,并发包中的原子计数在多线程的情况下可以让我们避免去写一些同步代码。
这里就先把jdk并发包中的线程池处理器ThreadPoolExecutor 以原子计数类AomicInteger 和倒数计时锁C
- java编程思想 抽象类和接口
百合不是茶
java抽象类接口
接口c++对接口和内部类只有简介的支持,但在java中有队这些类的直接支持
1 ,抽象类 : 如果一个类包含一个或多个抽象方法,该类必须限定为抽象类(否者编译器报错)
抽象方法 : 在方法中仅有声明而没有方法体
package com.wj.Interface;
- [房地产与大数据]房地产数据挖掘系统
comsci
数据挖掘
随着一个关键核心技术的突破,我们已经是独立自主的开发某些先进模块,但是要完全实现,还需要一定的时间...
所以,除了代码工作以外,我们还需要关心一下非技术领域的事件..比如说房地产
&nb
- 数组队列总结
沐刃青蛟
数组队列
数组队列是一种大小可以改变,类型没有定死的类似数组的工具。不过与数组相比,它更具有灵活性。因为它不但不用担心越界问题,而且因为泛型(类似c++中模板的东西)的存在而支持各种类型。
以下是数组队列的功能实现代码:
import List.Student;
public class
- Oracle存储过程无法编译的解决方法
IT独行者
oracle存储过程
今天同事修改Oracle存储过程又导致2个过程无法被编译,流程规范上的东西,Dave 这里不多说,看看怎么解决问题。
1. 查看无效对象
XEZF@xezf(qs-xezf-db1)> select object_name,object_type,status from all_objects where status='IN
- 重装系统之后oracle恢复
文强chu
oracle
前几天正在使用电脑,没有暂停oracle的各种服务。
突然win8.1系统奔溃,无法修复,开机时系统 提示正在搜集错误信息,然后再开机,再提示的无限循环中。
无耐我拿出系统u盘 准备重装系统,没想到竟然无法从u盘引导成功。
晚上到外面早了一家修电脑店,让人家给装了个系统,并且那哥们在我没反应过来的时候,
直接把我的c盘给格式化了 并且清理了注册表,再装系统。
然后的结果就是我的oracl
- python学习二( 一些基础语法)
小桔子
pthon基础语法
紧接着把!昨天没看继续看django 官方教程,学了下python的基本语法 与c类语言还是有些小差别:
1.ptyhon的源文件以UTF-8编码格式
2.
/ 除 结果浮点型
// 除 结果整形
% 除 取余数
* 乘
** 乘方 eg 5**2 结果是5的2次方25
_&
- svn 常用命令
aichenglong
SVN版本回退
1 svn回退版本
1)在window中选择log,根据想要回退的内容,选择revert this version或revert chanages from this version
两者的区别:
revert this version:表示回退到当前版本(该版本后的版本全部作废)
revert chanages from this versio
- 某小公司面试归来
alafqq
面试
先填单子,还要写笔试题,我以时间为急,拒绝了它。。时间宝贵。
老拿这些对付毕业生的东东来吓唬我。。
面试官很刁难,问了几个问题,记录下;
1,包的范围。。。public,private,protect. --悲剧了
2,hashcode方法和equals方法的区别。谁覆盖谁.结果,他说我说反了。
3,最恶心的一道题,抽象类继承抽象类吗?(察,一般它都是被继承的啊)
4,stru
- 动态数组的存储速度比较 集合框架
百合不是茶
集合框架
集合框架:
自定义数据结构(增删改查等)
package 数组;
/**
* 创建动态数组
* @author 百合
*
*/
public class ArrayDemo{
//定义一个数组来存放数据
String[] src = new String[0];
/**
* 增加元素加入容器
* @param s要加入容器
- 用JS实现一个JS对象,对象里有两个属性一个方法
bijian1013
js对象
<html>
<head>
</head>
<body>
用js代码实现一个js对象,对象里有两个属性,一个方法
</body>
<script>
var obj={a:'1234567',b:'bbbbbbbbbb',c:function(x){
- 探索JUnit4扩展:使用Rule
bijian1013
java单元测试JUnitRule
在上一篇文章中,讨论了使用Runner扩展JUnit4的方式,即直接修改Test Runner的实现(BlockJUnit4ClassRunner)。但这种方法显然不便于灵活地添加或删除扩展功能。下面将使用JUnit4.7才开始引入的扩展方式——Rule来实现相同的扩展功能。
1. Rule
&n
- [Gson一]非泛型POJO对象的反序列化
bit1129
POJO
当要将JSON数据串反序列化自身为非泛型的POJO时,使用Gson.fromJson(String, Class)方法。自身为非泛型的POJO的包括两种:
1. POJO对象不包含任何泛型的字段
2. POJO对象包含泛型字段,例如泛型集合或者泛型类
Data类 a.不是泛型类, b.Data中的集合List和Map都是泛型的 c.Data中不包含其它的POJO
- 【Kakfa五】Kafka Producer和Consumer基本使用
bit1129
kafka
0.Kafka服务器的配置
一个Broker,
一个Topic
Topic中只有一个Partition() 1. Producer:
package kafka.examples.producers;
import kafka.producer.KeyedMessage;
import kafka.javaapi.producer.Producer;
impor
- lsyncd实时同步搭建指南——取代rsync+inotify
ronin47
1. 几大实时同步工具比较 1.1 inotify + rsync
最近一直在寻求生产服务服务器上的同步替代方案,原先使用的是 inotify + rsync,但随着文件数量的增大到100W+,目录下的文件列表就达20M,在网络状况不佳或者限速的情况下,变更的文件可能10来个才几M,却因此要发送的文件列表就达20M,严重减低的带宽的使用效率以及同步效率;更为要紧的是,加入inotify
- java-9. 判断整数序列是不是二元查找树的后序遍历结果
bylijinnan
java
public class IsBinTreePostTraverse{
static boolean isBSTPostOrder(int[] a){
if(a==null){
return false;
}
/*1.只有一个结点时,肯定是查找树
*2.只有两个结点时,肯定是查找树。例如{5,6}对应的BST是 6 {6,5}对应的BST是
- MySQL的sum函数返回的类型
bylijinnan
javaspringsqlmysqljdbc
今天项目切换数据库时,出错
访问数据库的代码大概是这样:
String sql = "select sum(number) as sumNumberOfOneDay from tableName";
List<Map> rows = getJdbcTemplate().queryForList(sql);
for (Map row : rows
- java设计模式之单例模式
chicony
java设计模式
在阎宏博士的《JAVA与模式》一书中开头是这样描述单例模式的:
作为对象的创建模式,单例模式确保某一个类只有一个实例,而且自行实例化并向整个系统提供这个实例。这个类称为单例类。 单例模式的结构
单例模式的特点:
单例类只能有一个实例。
单例类必须自己创建自己的唯一实例。
单例类必须给所有其他对象提供这一实例。
饿汉式单例类
publ
- javascript取当月最后一天
ctrain
JavaScript
<!--javascript取当月最后一天-->
<script language=javascript>
var current = new Date();
var year = current.getYear();
var month = current.getMonth();
showMonthLastDay(year, mont
- linux tune2fs命令详解
daizj
linuxtune2fs查看系统文件块信息
一.简介:
tune2fs是调整和查看ext2/ext3文件系统的文件系统参数,Windows下面如果出现意外断电死机情况,下次开机一般都会出现系统自检。Linux系统下面也有文件系统自检,而且是可以通过tune2fs命令,自行定义自检周期及方式。
二.用法:
Usage: tune2fs [-c max_mounts_count] [-e errors_behavior] [-g grou
- 做有中国特色的程序员
dcj3sjt126com
程序员
从出版业说起 网络作品排到靠前的,都不会太难看,一般人不爱看某部作品也是因为不喜欢这个类型,而此人也不会全不喜欢这些网络作品。究其原因,是因为网络作品都是让人先白看的,看的好了才出了头。而纸质作品就不一定了,排行榜靠前的,有好作品,也有垃圾。 许多大牛都是写了博客,后来出了书。这些书也都不次,可能有人让为不好,是因为技术书不像小说,小说在读故事,技术书是在学知识或温习知识,有
- Android:TextView属性大全
dcj3sjt126com
textview
android:autoLink 设置是否当文本为URL链接/email/电话号码/map时,文本显示为可点击的链接。可选值(none/web/email/phone/map/all) android:autoText 如果设置,将自动执行输入值的拼写纠正。此处无效果,在显示输入法并输
- tomcat虚拟目录安装及其配置
eksliang
tomcat配置说明tomca部署web应用tomcat虚拟目录安装
转载请出自出处:http://eksliang.iteye.com/blog/2097184
1.-------------------------------------------tomcat 目录结构
config:存放tomcat的配置文件
temp :存放tomcat跑起来后存放临时文件用的
work : 当第一次访问应用中的jsp
- 浅谈:APP有哪些常被黑客利用的安全漏洞
gg163
APP
首先,说到APP的安全漏洞,身为程序猿的大家应该不陌生;如果抛开安卓自身开源的问题的话,其主要产生的原因就是开发过程中疏忽或者代码不严谨引起的。但这些责任也不能怪在程序猿头上,有时会因为BOSS时间催得紧等很多可观原因。由国内移动应用安全检测团队爱内测(ineice.com)的CTO给我们浅谈关于Android 系统的开源设计以及生态环境。
1. 应用反编译漏洞:APK 包非常容易被反编译成可读
- C#根据网址生成静态页面
hvt
Web.netC#asp.nethovertree
HoverTree开源项目中HoverTreeWeb.HVTPanel的Index.aspx文件是后台管理的首页。包含生成留言板首页,以及显示用户名,退出等功能。根据网址生成页面的方法:
bool CreateHtmlFile(string url, string path)
{
//http://keleyi.com/a/bjae/3d10wfax.htm
stri
- SVG 教程 (一)
天梯梦
svg
SVG 简介
SVG 是使用 XML 来描述二维图形和绘图程序的语言。 学习之前应具备的基础知识:
继续学习之前,你应该对以下内容有基本的了解:
HTML
XML 基础
如果希望首先学习这些内容,请在本站的首页选择相应的教程。 什么是SVG?
SVG 指可伸缩矢量图形 (Scalable Vector Graphics)
SVG 用来定义用于网络的基于矢量
- 一个简单的java栈
luyulong
java数据结构栈
public class MyStack {
private long[] arr;
private int top;
public MyStack() {
arr = new long[10];
top = -1;
}
public MyStack(int maxsize) {
arr = new long[maxsize];
top
- 基础数据结构和算法八:Binary search
sunwinner
AlgorithmBinary search
Binary search needs an ordered array so that it can use array indexing to dramatically reduce the number of compares required for each search, using the classic and venerable binary search algori
- 12个C语言面试题,涉及指针、进程、运算、结构体、函数、内存,看看你能做出几个!
刘星宇
c面试
12个C语言面试题,涉及指针、进程、运算、结构体、函数、内存,看看你能做出几个!
1.gets()函数
问:请找出下面代码里的问题:
#include<stdio.h>
int main(void)
{
char buff[10];
memset(buff,0,sizeof(buff));
- ITeye 7月技术图书有奖试读获奖名单公布
ITeye管理员
活动ITeye试读
ITeye携手人民邮电出版社图灵教育共同举办的7月技术图书有奖试读活动已圆满结束,非常感谢广大用户对本次活动的关注与参与。
7月试读活动回顾:
http://webmaster.iteye.com/blog/2092746
本次技术图书试读活动的优秀奖获奖名单及相应作品如下(优秀文章有很多,但名额有限,没获奖并不代表不优秀):
《Java性能优化权威指南》
![]()