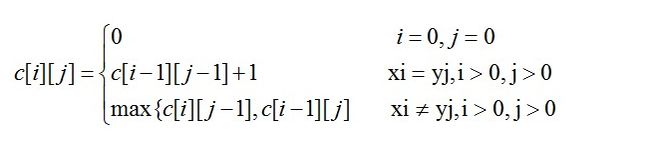最长公共子序列问题

问题描述：字符序列的子序列是指从给定字符序列中随意地（不一定连续）去掉若干个字符（可能一个也不去掉）后所形成的字符序列。令给定的字符序列X=“x0，x1，…，xm-1”，序列Y=“y0，y1，…，yk-1”是X的子序列，存在X的一个严格递增下标序列<i0，i1，…，ik-1>，使得对所有的j=0，1，…，k-1，有xij=yj。例如，X=“ABCBDAB”，Y=“BCDB”是X的一个子序列。

解决方法：

1、穷举法：针对序列x中所有的子序列(共2^m个)，在Y序列中寻列是否存在相同序列，并找出其中最大的序列。这种方法的时间复杂度为O(2^m*2^n)。

2、动态规划法：引进一个二维数组c[][]，用c[i][j]记录X[i]与Y[j] 的LCS 的长度，b[i][j]记录c[i][j]是通过哪一个子问题的值求得的，以决定搜索的方向。可以通过以下公式来计算从c[i][j]的值。

复杂度分析：

时间复杂度：O(m*n);

空间复杂度：O(m*n);

代码：

#include "stdafx.h"
#include <iostream>
#include <string>
#define MAXLEN 100
using namespace std;

void GetLCSLen(string str1, string str2,int m,int n,int c[][MAXLEN],int b[][MAXLEN])
{
    int i, j;
    for (i = 0; i <= m; i++)
        c[i][0] = 0;
    for (j = 1; j <= n; j++)
        c[0][j] = 0;
    
    for (i = 1; i <= m; i++)
    {
        for (j = 1; j <= n; j++)
        {
            if (str1[i - 1] == str2[j - 1])
            {
                c[i][j] = c[i - 1][j - 1] + 1;
                b[i][j] = 0;
            }
            else
            {
                if (c[i - 1][j] >= c[i][j - 1])
                {
                    c[i][j] = c[i - 1][j];
                    b[i][j] = 1;
                }
                else
                {
                    c[i][j] = c[i][j - 1];
                    b[i][j] = 2;
                }
            }
        }
    }
}

void PrintLCS(int b[][MAXLEN], string x, int i, int j)  //递归回溯最长子序列
{
    if (i == 0 || j == 0)
        return;
    if (b[i][j] == 0)
    {
        PrintLCS(b, x, i - 1, j - 1);
        cout<<x[i-1];
    }
    else if (b[i][j] == 1)
        PrintLCS(b, x, i - 1, j);
    else
        PrintLCS(b, x, i, j - 1);
}

int _tmain(int argc, _TCHAR* argv[])
{
    string s1 = "ABCBDAB";
    string s2 = "BDCABA";
    int strlen1 = s1.size();
    int strlen2 = s2.size();
    int b[MAXLEN][MAXLEN];
    int c[MAXLEN][MAXLEN]; 
    GetLCSLen(s1,s2,strlen1,strlen2,c,b);
    cout << c[strlen1][strlen2] << endl;  //输出最长子序列长度
    PrintLCS(b,s1,strlen1,strlen2);
    return 0;
}