- Codeforces Round 972 (Div. 2) A-C 题解
AKDreamer_HeXY
Codeforces比赛题解c++算法动态规划数据结构贪心算法
本来以为B2难度会1900什么的,结果感觉1200还没有,先做的B1,后悔了QwQ关于我现场没切出C这件事……现场排名:A.SimplePalindrome题意构造一个长度为nnn的字符串,只包含aeiou五种字母,需要使得构造出来的字符串所包含的回文子序列数量最小思路当n≤5n\le5n≤5时,只要555个字母不重复出现都是最优情况当n>5n>5n>5时,可以证明:把相同字母放在一起是最优情况:
- Codeforces Round 969 (Div. 2) C. Dora and C++ (裴蜀定理)
致碑前繁花
刷题记录c语言c++开发语言
什么?竟然是裴蜀定理。。。由于这里给出了a和b两个数,我们或许可以想到使用同样是需要给出两个定值的裴蜀定理,即:如果给定xxx和yyy,那么一定有ax+by=gcd(x,y)ax+by=gcd(x,y)ax+by=gcd(x,y)。所以在这时候我们就可以让输入的所有数都去对gcd(a,b)gcd(a,b)gcd(a,b)取模,这样就能够得到所有数的最简形式(可以当成是让所有数尽可能消去aaa和bb
- Codeforces Round #956 (Div. 2) C. Have Your Cake and Eat It Too
abTao_lx
c语言算法开发语言
CodeforcesRound#956(Div.2)C.HaveYourCakeandEatItToo题目大意:有长度为nnn的数组a,b,ca,b,ca,b,c,三个数组的和相同,把nnn分为三段非空连续段[la,ra],[lb,rb],[lc,rc][l_a,r_a],[l_b,r_b],[l_c,r_c][la,ra],[lb,rb],[lc,rc],互不重合。保证∑i=laraai,∑i=
- Codeforces Round #787 (Div. 3)个人题解
旋转卡题
算法贪心算法图论动态规划
CodeforcesRound#787(Div.3)个人题解文章目录CodeforcesRound#787(Div.3)个人题解A.FoodforAnimals题目大意思路参考代码B.MakeItIncreasing题目大意思路参考代码C.DetectiveTask题目大意思路参考代码D.VerticalPaths题目大意思路参考代码E.ReplaceWiththePrevious,Minimiz
- Codeforces Round 966 (Div. 3) ABCDEF
菜比乌斯反演
Codeforces算法c++数据结构
A题:PrimaryTask题意Dmitry写了t个整数,但是在写幂的时候写错了,将^符号给省略掉了。问哪几个是写幂时写错的思路写错的数符合10X,其中X(X>=2)是不含前导零的正整数,我们依此进行判断即可。代码inlinevoidsolve(){strings;cin>>s;intn=s.size();if(n='2'||n>3&&s[2]!='0')cout>n;vectorvis(n+2)
- 程序员进阶之算法练习(四十)Codeforces
落影loyinglin
正文题目1题目链接题目大意:在一维坐标轴上有三个点,坐标是a、b、c;现在需要移动这三个点的位置,使得三个点之间两两间隔大于d;每次只能移动一个点,每秒只能移动距离1;问最少需要多少秒,才能满足要求。输入:一行,四个数字,,,(1≤,,,≤10^9)输出:最少的秒数。Examplesinput5263output2题目解析:a、b、c之间没有关系,可以先排序,使得a=d的时候,ans=max(0,
- Codeforces Round 938 (Div. 3)
沫刃起
codeforces算法c++数据结构
A.YogurtSale#include#defineendl'\n'#defineintlonglongusingnamespacestd;intn,a,b;voidsolve(){cin>>n>>a>>b;if(2*a>t;while(t--){solve();}return0;}B.ProgressiveSquare#include#defineendl'\n'#defineintlongl
- 2023ICPC济南站训练补题
farawaytravelerchy
ACM-ICPC训练补题算法python
title:2023ICPC济南站VP补题记录(第48届)date:2024-01-1812:16:23mathjax:truetags:XCPCcategories:Algorithm文章目录2023ICPC济南站训练补题注:暂时更新vp时ac的4道题,其余题目之后持续更新[Problem-D-LargestDigit](https://codeforces.com/gym/104901/pro
- cf973Div3E
Colinnian
codeforces
https://codeforces.com/contest/2008/problem/Eusingll=longlong;voidsolve(){intn;std::cin>>n;std::strings;std::cin>>s;intans=n;//_____________________________//_____________________________//首先将奇数位置和偶数位
- Codeforces Round 969 (Div. 2 ABCDE题) 视频讲解
阿史大杯茶
Codeforces算法c++数据结构
A.Dora’sSetProblemStatementDorahasasetssscontainingintegers.Inthebeginning,shewillputallintegersin[l,r][l,r][l,r]intothesetsss.Thatis,anintegerxxxisinitiallycontainedinthesetifandonlyifl≤x≤rl\leqx\leq
- CF C. Candy Store
Jiu-yuan
算法
原题链接:Problem-C-Codeforces题意:多测,先给出n代表n种糖果,每种糖果分别给出数量和单价,可以将糖果平均分成若干袋,每一袋的的价格是一袋糖果数量×单价,对于每一种糖果都求出一袋的价格,对于每种糖果都需要用标签贴出一袋的价格,但是如果相邻的糖果价格相同,那么就可以用一个标签来代表价格,问最少使用几个标签。思路:如果一段糖果价格是一样的,那么设置价格为cost。因为每一袋糖果的价
- Codeforces Round 969 (Div. 2):A - Dora‘s Set题解C++,给自己陷入死胡同了,果然真理总是简洁朴素优美
醒了不起的盖茨比Z
c++算法leetcode动态规划c语言图论笔记
先说答案,有需要的大家看看:#includeusingnamespacestd;#defineendl'\n'intmain(){ios::sync_with_stdio(0),cin.tie(0),cout.tie(0);intt,l,r;cin>>t;while(t--){cin>>l>>r;intab=r-l+1;//这一行数字的长度if((ab%4==3)&&r%2!=0)//奇数结尾会有
- 关于求数组中两部分是否相等问题可以考虑前缀和的思路
shadowcase
算法c++
当之后你不记得标题由何而来时,请回顾codeforcesround918(div4)的E题/*这道题的核心就是题干中等式的转化,然后利用前缀和。还要注意flag[0]=1不能漏,不然就都是yes了*/#includeusingnamespacestd;#definelllonglongconstintmaxn=2e5;constintmod=1e6+7;intmain(){ios::sync_wi
- codeforces round 748 题解 A~D2
刘心奶黄包qaq
算法算法c++
文章目录A.Elections题目大意思路AC代码B.MakeitDivisibleby25题目大意思路AC代码C.SaveMoreMice题目大意思路AC代码D1.AllareSame题目大意思路AC代码D2.HalfofSame题目大意思路AC代码A.ElectionsA.Elections题目大意有三个候选人,每个候选人都有一个初始票数,问每个候选人至少增加多少票才能绝对被选上。思路判断其中
- Codeforces Round 963 (Div. 2)
lskkkkkkkkkkkk
题解dp动态规划思维模拟数论
CodeforcesRound963(Div.2)A.QuestionMarks题意有一场考试一共4∗n4*n4∗n道题目,其中答案为A,B,C,D的题目各nnn道,现在你有一份考试的结果,由字母A,B,C,D组成,请问最多得到多少分。思路每种选项最多答对n道题目,我们统计每个选项的出现次数,和n取较小值,将结果加起来即可。代码#includeusingnamespacestd;#defineIO
- Codeforces Round 935 (Div. 3)
lskkkkkkkkkkkk
算法数据结构c++
题目链接A.SettingupCamp题意有一些屋子,每个屋子最多容纳三个人,有三种人,内向人必须一个人一个屋,外向人必须三个人同时一个屋子,综合人随意(一个两个三个都可)现在有aaa个内向人,bbb个外向人,ccc个综合人。问最少需要多少个屋子才能满足所有人的要求,如果无论如何都不能满足那么就输出-1思路我们很容易发现,对于内向人,不会导致输出-1.因为给他们一人一个屋子即可。对于综合人同样不会
- Codeforces Round 936 (Div. 2)
lskkkkkkkkkkkk
c++算法数据结构
CodeforcesRound936(Div.2)题目链接A.MedianofanArray题意给出一个数组aaa,每次操作可以让其中一个数增加一。问最少需要几次操作能改变中位数的值。长度为n的数组a的中位数表示为,令b是a排完序后的数组,那么b⌈n2⌉b_{\lceil\frac{n}{2}\rceil}b⌈2n⌉即为a的中位数。思路假设中位数为mmm,那么我们就需要让最终的中位数变为m+1m+
- CF 967 D. Longest Max Min Subsequence
Jiu-yuan
算法数据结构
原题链接:Problem-D-Codeforces题意:多测,每次给出长度为n的数组,要求找出没有重复元素的,最长的子序列,如果不止一个最长子序列,那么就选择字典序最小的,比较字典序的时候,如果这个元素的下标是奇数,那么就变成负数比较。思路:线段树+贪心,观察题意可知,最终的子序列肯定是正负相间的,那么对于奇数位置,这个数越大越好,对于偶数位置,这个数越小越好。那么就可以贪心的考虑这个问题,设置二
- [Codeforces 115E]Linear Kingdom Races
ddpx3313
c/c++
题目大意:有n块地,初始是荒地。你可以把某些荒地开垦(需要花费相应的价值\(a_i\)(正整数)),然后这些荒地就可以种田。现在有m年,每年要在l到r区间内种田,获得p(正整数)的价值(必须保证l~r都已经开荒,否则不能种田)。问最大收益。解题思路:DP。设F[i][j]表示前i块地,最后有连续的j块地已开荒的最大收益。则\(F[i+1][0]=max\{F[i][j]\}\)。不开荒,则中间断了
- Codeforces Round 917 (Div. 2)
Lanthanmum
算法
A.考虑有没有负数,偶数(把没有负数考虑进去了)就要改变一个数为0,为min,如果是奇数就不用考虑ー、_ィ│/////へ/ノ<|\\//ヽ_ノ(_/│////7|///>―r ̄ ̄`ー―_#include#include#include#include#include#include#include#include#include#include#include#include#include#de
- 【codeforces 115E】Linear Kingdom Races 题意&题解&代码(c++)
deritt
oi之路DERIT的博客专栏线段树-dp
**E.LinearKingdomRaces**timelimitpertest5secondsmemorylimitpertest256megabytesYouareacarraceorganizerandwouldliketoarrangesomeracesinLinearKingdom.LinearKingdomhasnconsecutiveroadsspanningfromlefttori
- E. Linear Kingdom Races
Lanthanmum
算法数据结构动态规划
https://codeforces.com/problemset/problem/115/E线段树优化dpO(n2)->O(nlogn)分析题意发现可以有暴力dpdp(i)是前i条路最大利润dp(i)=dp(i-1)不选第i条路dp(i)=max(dp(j)+val(j)-cost(j))选这i条路dp(i)=max(dp(i-1),max(dp(j)+val(j)-cost(j))显然右边值可
- 2024ccpc中国郑州
Pown_ShanYu
算法
题目链接:Dashboard-2024NationalInvitationalofCCPC(Zhengzhou),2024CCPCHenanProvincialCollegiateProgrammingContest-Codeforces文章目录F.优秀字符串J.排列与合数B.扫雷1A.OnceInMyLifeM.有效算法H.随机栈//Solve函数结合末尾模板F.优秀字符串题意:优秀字符串:长度
- Codeforces Round 967 (Div. 2) C题Guess The Tree
青衫酒145
算法
题目链接令1是根,我们可以一层一层的递推出去。容易知道询问a,b如果结果是c,那么c就是a,b路径上的中点。我们可以先让根1和其他n-1个点都询问一遍,如果返回值是1,那么这些点就是第二层,深度为2的点。我们发现一个c点会对应两层的深节点,比如一条链1234,如果询问13和14返回都会是2,那么我们就让2和34分别连一条边,表示3和4是可能和2直接连边的。然后因为第二层我们已经推出来有哪些点了,再
- Codeforces Round 938 (Div. 3)A-C,E-H
青衫酒145
c语言算法c++
题目链接A.YogurtSale贪心的选两个物品价格少的,若总个数为奇数再加是单个价格代码:#include#definelllonglongusingnamespacestd;voidsolve(){intn,a,b;cin>>n>>a>>b;intr=0;if(n&1){if(b>t;while(t--){solve();}return0;}B.ProgressiveSquare因为c,d大于
- Codeforces gym102423 - J One of Each(贪心 + 栈)
Happig丶
#
传送门题目大意给出nnn个数,仅包含[1,k][1,k][1,k]中的数,且至少含有kkk个不同的数。找到一个长度为kkk的子序列是kkk的一个排列且字典序最小。解题思路这个题算是比较难想的贪心了,而且要用栈维护。一开始用尺取发现维护不了,贪心的话自己又陷入了如下几个误区:如果从后向前贪心,显然是可以的,但是对于已经选过的数,无法得知前缀中待选的数是否能全部出现,即使bitsetbitsetbit
- CF 966 Div3 F. Color Rows and Columns
Jiu-yuan
算法
原题链接:Problem-F-Codeforces题意:多测,每组测试数据给出n和k,n代表有n个长方形,k代表需要的到k分,每个长方形都有宽和高,每次可以填涂一个格子,如果填满一列或者一行就可以获得一分,问达到k分最少需要填涂多少格子。赛时思路:背包dp+随机化,按照背包dp的思路来想,就是选择了某个长方形,如果填满这个长方形分数也不能到达k,那么就直接填满,如果大于等于k那么就用最小代价来填满
- Codeforces Round 966 (Div. 3) 前四题python题解
CodeNerd影
python开发语言
A.PrimaryTasktimelimitpertest1secondmemorylimitpertest256megabytesDmitrywrotedowntintegersontheboard,andthatisgood.Heissurethathelostanimportantintegernamongthem,andthatisbad.Theintegernnhadtheform10^
- Codeforces Round 933 (Div. 3) (A~E)
叶域
算法竞赛算法codeforcesc++
CodeforcesRound933(Div.3)(A~E)目录:ABCDEA题:RudolfandtheTicket标签:暴力枚举(bruteforce)数学(math)排序算法(sortings)双指针算法(twopointers)题目大意给一个长度为n的数组b,和一个长度为m的数组c,还有一个整数k。从b,c中各选一个数,问有多少种选法使这两个数小于等于k思路前缀和快速得出在b数组中小于等于
- Codeforces Round 927 (Div. 3)(A~E)
叶域
算法竞赛c++codeforces算法
CodeforcesRound927(Div.3)(A~E)目录:ABCDEA题:ThornsandCoins标签:动态规划(dp)贪心(greedy)实现问题,编程技巧,模拟(implementation)题目大意由n个连续单元组成的路径,每个单元可以是空的,含有荆棘,或者含有一枚硬币,在一次移动中,你可以沿着路径移动一个或两个单元,前提是目标单元不含有荆棘(并且属于路径)如果你移动到含有硬币的
- Spring的注解积累
yijiesuifeng
spring注解
用注解来向Spring容器注册Bean。
需要在applicationContext.xml中注册:
<context:component-scan base-package=”pagkage1[,pagkage2,…,pagkageN]”/>。
如:在base-package指明一个包
<context:component-sc
- 传感器
百合不是茶
android传感器
android传感器的作用主要就是来获取数据,根据得到的数据来触发某种事件
下面就以重力传感器为例;
1,在onCreate中获得传感器服务
private SensorManager sm;// 获得系统的服务
private Sensor sensor;// 创建传感器实例
@Override
protected void
- [光磁与探测]金吕玉衣的意义
comsci
这是一个古代人的秘密:现在告诉大家
信不信由你们:
穿上金律玉衣的人,如果处于灵魂出窍的状态,可以飞到宇宙中去看星星
这就是为什么古代
- 精简的反序打印某个数
沐刃青蛟
打印
以前看到一些让求反序打印某个数的程序。
比如:输入123,输出321。
记得以前是告诉你是几位数的,当时就抓耳挠腮,完全没有思路。
似乎最后是用到%和/方法解决的。
而今突然想到一个简短的方法,就可以实现任意位数的反序打印(但是如果是首位数或者尾位数为0时就没有打印出来了)
代码如下:
long num, num1=0;
- PHP:6种方法获取文件的扩展名
IT独行者
PHP扩展名
PHP:6种方法获取文件的扩展名
1、字符串查找和截取的方法
1
$extension
=
substr
(
strrchr
(
$file
,
'.'
), 1);
2、字符串查找和截取的方法二
1
$extension
=
substr
- 面试111
文强chu
面试
1事务隔离级别有那些 ,事务特性是什么(问到一次)
2 spring aop 如何管理事务的,如何实现的。动态代理如何实现,jdk怎么实现动态代理的,ioc是怎么实现的,spring是单例还是多例,有那些初始化bean的方式,各有什么区别(经常问)
3 struts默认提供了那些拦截器 (一次)
4 过滤器和拦截器的区别 (频率也挺高)
5 final,finally final
- XML的四种解析方式
小桔子
domjdomdom4jsax
在平时工作中,难免会遇到把 XML 作为数据存储格式。面对目前种类繁多的解决方案,哪个最适合我们呢?在这篇文章中,我对这四种主流方案做一个不完全评测,仅仅针对遍历 XML 这块来测试,因为遍历 XML 是工作中使用最多的(至少我认为)。 预 备 测试环境: AMD 毒龙1.4G OC 1.5G、256M DDR333、Windows2000 Server
- wordpress中常见的操作
aichenglong
中文注册wordpress移除菜单
1 wordpress中使用中文名注册解决办法
1)使用插件
2)修改wp源代码
进入到wp-include/formatting.php文件中找到
function sanitize_user( $username, $strict = false
- 小飞飞学管理-1
alafqq
管理
项目管理的下午题,其实就在提出问题(挑刺),分析问题,解决问题。
今天我随意看下10年上半年的第一题。主要就是项目经理的提拨和培养。
结合我自己经历写下心得
对于公司选拔和培养项目经理的制度有什么毛病呢?
1,公司考察,选拔项目经理,只关注技术能力,而很少或没有关注管理方面的经验,能力。
2,公司对项目经理缺乏必要的项目管理知识和技能方面的培训。
3,公司对项目经理的工作缺乏进行指
- IO输入输出部分探讨
百合不是茶
IO
//文件处理 在处理文件输入输出时要引入java.IO这个包;
/*
1,运用File类对文件目录和属性进行操作
2,理解流,理解输入输出流的概念
3,使用字节/符流对文件进行读/写操作
4,了解标准的I/O
5,了解对象序列化
*/
//1,运用File类对文件目录和属性进行操作
//在工程中线创建一个text.txt
- getElementById的用法
bijian1013
element
getElementById是通过Id来设置/返回HTML标签的属性及调用其事件与方法。用这个方法基本上可以控制页面所有标签,条件很简单,就是给每个标签分配一个ID号。
返回具有指定ID属性值的第一个对象的一个引用。
语法:
&n
- 励志经典语录
bijian1013
励志人生
经典语录1:
哈佛有一个著名的理论:人的差别在于业余时间,而一个人的命运决定于晚上8点到10点之间。每晚抽出2个小时的时间用来阅读、进修、思考或参加有意的演讲、讨论,你会发现,你的人生正在发生改变,坚持数年之后,成功会向你招手。不要每天抱着QQ/MSN/游戏/电影/肥皂剧……奋斗到12点都舍不得休息,看就看一些励志的影视或者文章,不要当作消遣;学会思考人生,学会感悟人生
- [MongoDB学习笔记三]MongoDB分片
bit1129
mongodb
MongoDB的副本集(Replica Set)一方面解决了数据的备份和数据的可靠性问题,另一方面也提升了数据的读写性能。MongoDB分片(Sharding)则解决了数据的扩容问题,MongoDB作为云计算时代的分布式数据库,大容量数据存储,高效并发的数据存取,自动容错等是MongoDB的关键指标。
本篇介绍MongoDB的切片(Sharding)
1.何时需要分片
&nbs
- 【Spark八十三】BlockManager在Spark中的使用场景
bit1129
manager
1. Broadcast变量的存储,在HttpBroadcast类中可以知道
2. RDD通过CacheManager存储RDD中的数据,CacheManager也是通过BlockManager进行存储的
3. ShuffleMapTask得到的结果数据,是通过FileShuffleBlockManager进行管理的,而FileShuffleBlockManager最终也是使用BlockMan
- yum方式部署zabbix
ronin47
yum方式部署zabbix
安装网络yum库#rpm -ivh http://repo.zabbix.com/zabbix/2.4/rhel/6/x86_64/zabbix-release-2.4-1.el6.noarch.rpm 通过yum装mysql和zabbix调用的插件还有agent代理#yum install zabbix-server-mysql zabbix-web-mysql mysql-
- Hibernate4和MySQL5.5自动创建表失败问题解决方法
byalias
J2EEHibernate4
今天初学Hibernate4,了解了使用Hibernate的过程。大体分为4个步骤:
①创建hibernate.cfg.xml文件
②创建持久化对象
③创建*.hbm.xml映射文件
④编写hibernate相应代码
在第四步中,进行了单元测试,测试预期结果是hibernate自动帮助在数据库中创建数据表,结果JUnit单元测试没有问题,在控制台打印了创建数据表的SQL语句,但在数据库中
- Netty源码学习-FrameDecoder
bylijinnan
javanetty
Netty 3.x的user guide里FrameDecoder的例子,有几个疑问:
1.文档说:FrameDecoder calls decode method with an internally maintained cumulative buffer whenever new data is received.
为什么每次有新数据到达时,都会调用decode方法?
2.Dec
- SQL行列转换方法
chicony
行列转换
create table tb(终端名称 varchar(10) , CEI分值 varchar(10) , 终端数量 int)
insert into tb values('三星' , '0-5' , 74)
insert into tb values('三星' , '10-15' , 83)
insert into tb values('苹果' , '0-5' , 93)
- 中文编码测试
ctrain
编码
循环打印转换编码
String[] codes = {
"iso-8859-1",
"utf-8",
"gbk",
"unicode"
};
for (int i = 0; i < codes.length; i++) {
for (int j
- hive 客户端查询报堆内存溢出解决方法
daizj
hive堆内存溢出
hive> select * from t_test where ds=20150323 limit 2;
OK
Exception in thread "main" java.lang.OutOfMemoryError: Java heap space
问题原因: hive堆内存默认为256M
这个问题的解决方法为:
修改/us
- 人有多大懒,才有多大闲 (评论『卓有成效的程序员』)
dcj3sjt126com
程序员
卓有成效的程序员给我的震撼很大,程序员作为特殊的群体,有的人可以这么懒, 懒到事情都交给机器去做 ,而有的人又可以那么勤奋,每天都孜孜不倦得做着重复单调的工作。
在看这本书之前,我属于勤奋的人,而看完这本书以后,我要努力变成懒惰的人。
不要在去庞大的开始菜单里面一项一项搜索自己的应用程序,也不要在自己的桌面上放置眼花缭乱的快捷图标
- Eclipse简单有用的配置
dcj3sjt126com
eclipse
1、显示行号 Window -- Prefences -- General -- Editors -- Text Editors -- show line numbers
2、代码提示字符 Window ->Perferences,并依次展开 Java -> Editor -> Content Assist,最下面一栏 auto-Activation
- 在tomcat上面安装solr4.8.0全过程
eksliang
Solrsolr4.0后的版本安装solr4.8.0安装
转载请出自出处:
http://eksliang.iteye.com/blog/2096478
首先solr是一个基于java的web的应用,所以安装solr之前必须先安装JDK和tomcat,我这里就先省略安装tomcat和jdk了
第一步:当然是下载去官网上下载最新的solr版本,下载地址
- Android APP通用型拒绝服务、漏洞分析报告
gg163
漏洞androidAPP分析
点评:记得曾经有段时间很多SRC平台被刷了大量APP本地拒绝服务漏洞,移动安全团队爱内测(ineice.com)发现了一个安卓客户端的通用型拒绝服务漏洞,来看看他们的详细分析吧。
0xr0ot和Xbalien交流所有可能导致应用拒绝服务的异常类型时,发现了一处通用的本地拒绝服务漏洞。该通用型本地拒绝服务可以造成大面积的app拒绝服务。
针对序列化对象而出现的拒绝服务主要
- HoverTree项目已经实现分层
hvt
编程.netWebC#ASP.ENT
HoverTree项目已经初步实现分层,源代码已经上传到 http://hovertree.codeplex.com请到SOURCE CODE查看。在本地用SQL Server 2008 数据库测试成功。数据库和表请参考:http://keleyi.com/a/bjae/ue6stb42.htmHoverTree是一个ASP.NET 开源项目,希望对你学习ASP.NET或者C#语言有帮助,如果你对
- Google Maps API v3: Remove Markers 移除标记
天梯梦
google maps api
Simply do the following:
I. Declare a global variable:
var markersArray = [];
II. Define a function:
function clearOverlays() {
for (var i = 0; i < markersArray.length; i++ )
- jQuery选择器总结
lq38366
jquery选择器
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40
- 基础数据结构和算法六:Quick sort
sunwinner
AlgorithmQuicksort
Quick sort is probably used more widely than any other. It is popular because it is not difficult to implement, works well for a variety of different kinds of input data, and is substantially faster t
- 如何让Flash不遮挡HTML div元素的技巧_HTML/Xhtml_网页制作
刘星宇
htmlWeb
今天在写一个flash广告代码的时候,因为flash自带的链接,容易被当成弹出广告,所以做了一个div层放到flash上面,这样链接都是a触发的不会被拦截,但发现flash一直处于div层上面,原来flash需要加个参数才可以。
让flash置于DIV层之下的方法,让flash不挡住飘浮层或下拉菜单,让Flash不档住浮动对象或层的关键参数:wmode=opaque。
方法如下:
- Mybatis实用Mapper SQL汇总示例
wdmcygah
sqlmysqlmybatis实用
Mybatis作为一个非常好用的持久层框架,相关资料真的是少得可怜,所幸的是官方文档还算详细。本博文主要列举一些个人感觉比较常用的场景及相应的Mapper SQL写法,希望能够对大家有所帮助。
不少持久层框架对动态SQL的支持不足,在SQL需要动态拼接时非常苦恼,而Mybatis很好地解决了这个问题,算是框架的一大亮点。对于常见的场景,例如:批量插入/更新/删除,模糊查询,多条件查询,联表查询,
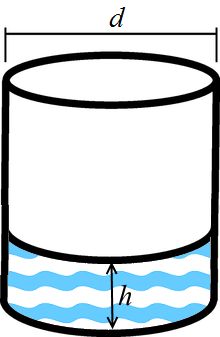
![]() , thus we can conclude that you decrease the level of water by
, thus we can conclude that you decrease the level of water by ![]() centimeters per second. At the same time water level increases by 1 centimeter per second due to rain. Thus, cup will be empty in
centimeters per second. At the same time water level increases by 1 centimeter per second due to rain. Thus, cup will be empty in ![]() seconds.
seconds.
![]() .
.