hdu 3480 Division 斜率优化
链接:http://acm.hdu.edu.cn/showproblem.php?pid=3480
Division
Time Limit: 10000/5000 MS (Java/Others) Memory Limit: 999999/400000 K (Java/Others)Total Submission(s): 3864 Accepted Submission(s): 1479
Problem Description
Little D is really interested in the theorem of sets recently. There’s a problem that confused him a long time.
Let T be a set of integers. Let the MIN be the minimum integer in T and MAX be the maximum, then the cost of set T if defined as (MAX – MIN)^2. Now given an integer set S, we want to find out M subsets S1, S2, …, SM of S, such that
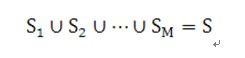
and the total cost of each subset is minimal.
Let T be a set of integers. Let the MIN be the minimum integer in T and MAX be the maximum, then the cost of set T if defined as (MAX – MIN)^2. Now given an integer set S, we want to find out M subsets S1, S2, …, SM of S, such that
and the total cost of each subset is minimal.
Input
The input contains multiple test cases.
In the first line of the input there’s an integer T which is the number of test cases. Then the description of T test cases will be given.
For any test case, the first line contains two integers N (≤ 10,000) and M (≤ 5,000). N is the number of elements in S (may be duplicated). M is the number of subsets that we want to get. In the next line, there will be N integers giving set S.
In the first line of the input there’s an integer T which is the number of test cases. Then the description of T test cases will be given.
For any test case, the first line contains two integers N (≤ 10,000) and M (≤ 5,000). N is the number of elements in S (may be duplicated). M is the number of subsets that we want to get. In the next line, there will be N integers giving set S.
Output
For each test case, output one line containing exactly one integer, the minimal total cost. Take a look at the sample output for format.
Sample Input
2 3 2 1 2 4 4 2 4 7 10 1
Sample Output
Case 1: 1 Case 2: 18
Hint
The answer will fit into a 32-bit signed integer.
题意:把n个数字 分成m个集合。 每个集合的价值是 这个集合中 (max-min)^2。 输出最少的价值
做法:如果想价值最小,不妨先排个序。这样 最后的答案肯定在排序后的数组中 是连续的。
可以得到数组 dp[i][c]=min{dp[j][c-1]+(num[i]-num[j+1])^2
直接dp 复杂度 N*N*M 瞬间爆炸。
可以斜率优化来写;
推导出
y(j)=dp[j][c-1]+num[j+1]*num[j+1]
x(j)=num[j+1]
当 k [y(j)-y(k)]/[x[k]-x[j]]<=2*num[i] 当不等式成立是,j的取值更优。#include