233 Matrix（矩阵快速幂）

233 Matrix

Time Limit: 10000/5000 MS (Java/Others)    Memory Limit: 65536/65536 K (Java/Others)
Total Submission(s): 1471    Accepted Submission(s): 863

Problem Description

In our daily life we often use 233 to express our feelings. Actually, we may say 2333, 23333, or 233333 ... in the same meaning. And here is the question: Suppose we have a matrix called 233 matrix. In the first line, it would be 233, 2333, 23333... (it means a _0,1 = 233,a _0,2 = 2333,a _0,3 = 23333...) Besides, in 233 matrix, we got a _i,j = a _i-1,j +a _i,j-1( i,j ≠ 0). Now you have known a _1,0,a _2,0,...,a _n,0, could you tell me a _n,m in the 233 matrix?

 

Input

There are multiple test cases. Please process till EOF.

For each case, the first line contains two postive integers n,m(n ≤ 10,m ≤ 10 ⁹). The second line contains n integers, a _1,0,a _2,0,...,a _n,0(0 ≤ a _i,0 < 2 ³¹).

 

Output

For each case, output a _n,m mod 10000007.

 

Sample Input

1 1 1 2 2 0 0 3 7 23 47 16

 

Sample Output

234 2799 72937

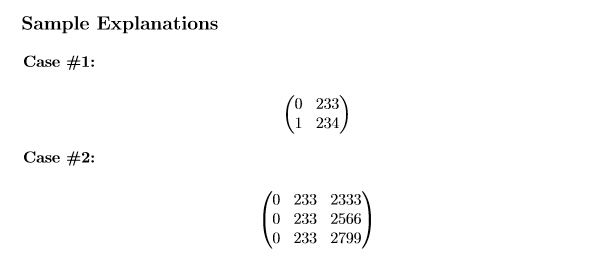
Hint

 

Source

2014 ACM/ICPC Asia Regional Xi'an Online

 

题意：

给你一个矩阵的第一行和第一列，让你求第n行第m列的值。其中矩阵中元素有递推式a[i][j] = a[i-1][j]+a[i][j-1]。其中第一行为0,233,2333,23333...，第一列为0,x1,x2,x3...，xi在输入中给出。n<=10，m<=10^9

分析：

一开始我还想通过递推关系找规律呢==，后来发现不是很好找，即使找到相应的东西也需要求10^9个元素的和。。。想想可以用矩阵快速幂解决，对于这种有着递推关系且数量较大的递推式来说，但始终想不到如何构造矩阵。。。后来看题解才知道，原来可以一列一列往右推构成递推关系。如果去掉第一行，我们很容易构造矩阵来将第一列推到第二列，而对于233到2333这些我们可以*10+3递推到，然后如果要合一起的话这里需要再增加一行才能实现233推到2333。首先第一行第一个数可以改为23这样更好满足*10+3的关系且并不会影响最终结果，然后我们再增加n+1这行，全是3，这样就很容易利用矩阵从一列推到另一列了。例如n=3,m=3的情况，可以构造矩阵：

233                         10 0 0 0 1       23
x1+233                   10 1 0 0 1       x1
x1+x2+233      =     10 1 1 0 1   *   x2
x1+x2+x3+233       10 1 1 1 1       x3
3                             0  0 0 0 0       3

code:

#include
#include
#include
#include
#include
#include
using namespace std;
const int mod = 1e7+7;
const int N = 1e5+10;
typedef long long ll;
#define INF 1<<30
int n, m;
struct Mat
{
    ll mat[15][15];
};

Mat operator * (Mat a, Mat b)
{
    Mat c;
    memset(c.mat, 0, sizeof(c.mat));
    for(int k=0; k= j && i != n+1) || j == n+1) a.mat[i][j] = 1;
                else a.mat[i][j] = 0;
            }
        }
        for(; m>0; m>>=1)
        {
            if(m & 1) res = res*a;
            a = a*a;
        }
        ll x, ans = 23*res.mat[n][0];
        for(int i=1; i<=n; i++)
        {
            scanf("%lld", &x);
            ans = (ans+x*res.mat[n][i]%mod)%mod;
        }
        ans = (ans+3*res.mat[n][n+1]%mod)%mod;
        printf("%lld\n", ans);
    }
    return 0;
}