- 学霸父母学渣娃,这孩子真是亲生的?太扎心了!
东北SK皇家成长中心
现在的社会,每个家庭基本都把孩子的教育放在第一位,哪怕父母平时上班再苦再累也不敢在孩子的教育上有丝毫的马虎,平时对孩子的照顾真的是无微不至,每天早起送孩子上学,晚上回家辅导孩子写作业,有的父母的文化程度非常高,但是每每到了辅导孩子写作业这个时候,父母们内心都有这样一种想法,这个孩子真的是我亲生的吗?真想一巴掌拍死他,我上辈子是做了什么孽生出这么一个智障的孩子,家里每每就要上演全武行,看看这些孩子到
- 数据结构之哈希表
X同学的开始
数据结构数据结构散列表
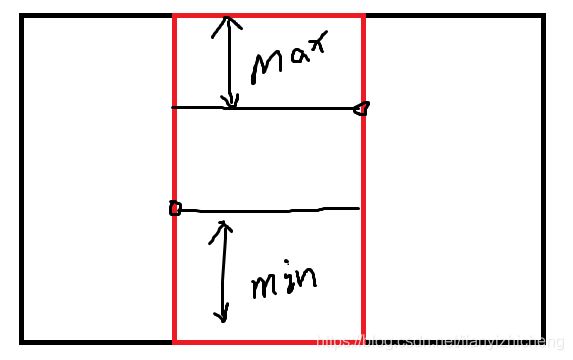
哈希表(散列表)出现的原因在顺序表中查找时,需要从表头开始,依次遍历比较a[i]与key的值是否相等,直到相等才返回索引i;在有序表中查找时,我们经常使用的是二分查找,通过比较key与a[i]的大小来折半查找,直到相等时才返回索引i。最终通过索引找到我们要找的元素。但是,这两种方法的效率都依赖于查找中比较的次数。我们有一种想法,能不能不经过比较,而是直接通过关键字key一次得到所要的结果呢?这时,
- 戴容容 中原焦点团队.网络初级第33期,坚持分享第19天 2022年3月9日
TessDai
《每个人眼中的世界都是不同的》“一千个人眼里有一千个哈姆雷特”世界是多元的,每个人都有自己的道理,人人按照自己的理解去看待这个世界的人和物.我们如此,其他人也是如此.因此,任何事情,我们要放下自己以为的真理,去理解他人认为的真理,只有同频方能共振.孩子在慢慢长大的过程中慢慢学会独立,甚至对抗.尤其当孩子处于青春期的时候,他们开始有很多自己独立的想法,和一些特立独行的做法,家长常常会觉得不可思议,觉
- 2018-12-22 《金刚经修心课:不焦虑的活法》摘录
Cintia1004
不为外界干扰的神奇力量如果你即将开始阅读金刚经,请试着把你的心空下来,把你各种习惯性的想法放在一边,以一种敞开的心态去阅读它。在敞开的阅读里,你会慢慢领悟到,金刚经没有任何结论,只是一种启迪,一种指引,指引你彻底地自我解放,从一切的成见里解放出来。你会惊奇地发现,金刚经……你都能够获得一种不为外界干扰的平静的力量。当这种力量充满你的日常生活,你会不害怕失败,……没有得到的时候,想要得到;已经得到的
- 2022-10-10
幸福芳芳
10.10日觉察日记1.事件:开晨会员工来不齐,路远的请假,离得近的也请假,一律不批!2.感受:生气,气愤(情绪如何转化或使用)3.想法:1.今年已经很少开晨会了,非必要不会通知开会的,临近点了再打电话请假,又不是特别忙的季节,借口都会找~~2.不来的按公司标准执行负激励,待岗处理!我为你们负责,你们安全重要会议都不参加,自己都不为自己负责!以后有事也别找我!尤其是经销商老板,自己都不清楚自己用工
- 摄影小白,怎么才能拍出高大上产品图片?
是波妞唉
很多人以为文案只要会码字,会排版就OK了!说实话,没接触到这一行的时候,我的想法更简单,以为只要会写字就行!可是真做了文案才发现,码字只是入门级的基本功。一篇文章离不开排版、配图,说起来很简单!从头做到尾你就会发现,写文章用两个小时,找合适的配图居然要花掉半天的时间,甚至更久!图片能找到合适的就不怕,还有找不到的,比如产品图,只能亲自拍。拿着摆弄了半天,就是拍不出想要的效果,光线不好、搭出来丑破天
- 随笔(探悟)
杰语唱响
亲兄弟姐妹之间不来往,其实不就是吃亏的人不想吃亏了,或者是占便宜的人占不到便宜了,从此就断绝了来往,互不搭理了。那些极度自私的人,是最不讲道理的,无论他们遇到什么事,都要让他占到便宜。顺着他的心情才行。否则那就是别人的不对。有这种想法的人,一看就是个穷命,那些个处处想着占便宜的人,老是想多要点,原因就是我没有吗,什么都没有,德行也没有,财富也没有,你说他的命怎么可能会好。家庭不和,兄弟姐妹断绝来往
- Python编程 - 函数进阶
易辰君
Python核心编程python开发语言
目录前言一、函数参数的高级用法(一)缺省参数(二)命名参数(三)不定长参数二、拆包(一)函数返回值拆包(二)通过星号拆包(三)总结三、匿名函数(一)函数定义(二)使用匿名函数四、递归函数(一)简介(二)基本结构(三)简单示例(四)优缺点总结前言上篇文章主要了解了函数基础,如何定义函数,函数种类以及局部变量和全局变量的差异等,接下来就讲解python函数较为进阶的知识点,若有任何想法欢迎一起沟通讨论
- #跟宇彤老师成为声音万人迷#第十季打卡营第二十一天#
穆希乐
今天是这季打卡营的最后一天,今天的打卡是用心诵读“初心”!很有意义的俩个字,但函给了每一位来练声的伙伴们最初的想法,让我也想起了我来练声的初心:就是想让自己的声音听起来有亲和力。从每日的基本功练习、到喜怒哀乐的绕口令在到各种的配音秀,最后俩天是用心的朗读等等,也是各种的第一次,想来有的伙伴们也和我一样是各种的第一次,这些第一次带着新鲜、刺激、更多的是激动与感恩!激动的是伙伴们在愉快的环境中每天开心
- 2022-10-20
择凡
疫情又一次给包头按下了暂停键,我在家中闲来无事,又动了写作的念头。从有这个念头到现在也已有几天的时间却迟迟没有动笔,因为我深感在时代的大浪潮下,我不知道是应该把我的想法写下来还是用其他方式来表达。我下定决心开始动笔,并不是因为我觉得文字是最好的表达方式,但却是我最想用的方式。我读了一下自己以前写的文字,非常感谢这个平台替我保留了下来,还记得在我上学的时候也写过一些文字,但是都遗失了,未免遗憾啊。以
- 国庆节的一天
安心雨
昨晚朋友间就转发国庆阅兵时间安排细节。今早,六点起床,到公园散步,一路上国旗招展,浓浓喜庆味。图片发自App准时坐到电脑前,拉上窗帘,关了房门,一个人静静感受,视觉和心灵的震撼。怕大脑内存不足,想要永远留存住那些属于这个时代,属于这个国家的骄傲。于是,拿出手机,对着屏幕拍了一张一张又一张。下午,朋友圈各种关于国庆的想法、评论、图片刷屏,翻了一遍一遍又一遍,每一遍都是骄傲和自豪。为生在这个伟大的时代
- 等
缓光
心数实验显示,量子场不能单纯回应我们的愿望(情感要求),也不能单纯回应我们的目的(想法);只有当这两者同调时,或者说,当他们一致传播出相同的信号时,量子场才会有所回应。当我们结合高昂的情绪、开放的心,有意义的意念及明确的思维,我们将能发送出信息,使量子场以令人惊喜的方式回应我们。然而,量子场并非回应我们想要的,而是反应了我们是怎样的存在!摘自《未来预演》让心❤与脑一起演化出我们真正的存在吧!
- 高仿一个echarts饼图
街角小林2
开头饼图,很常见的一种图表,使用任何一个图表库都能轻松的渲染出来,但是,我司的交互想法千奇百怪,布局捉摸不透,本身饼图是没啥可变的,但是配套的图例千变万化,翻遍ECharts配置文档都还原不出来,那么有两条路可以选,一是跟交互说实现不了,说服交互按图表库的布局来,但是一般交互可能会对你灵魂拷问,为什么别人都能做出来,你做不出来?所以我选第二种,自己做一个得了。用canvas实现一个饼图很简单,所以
- 9月25日,十二星座,星座运势
葫芦姐姐聊星座
白羊座:想法欠稳妥,你还是会被某些事情牵着鼻子走,让自己显得十分被动,建议得尽快放下心中的杂念,找到恰当的方式去解决问题;感情上患得患失,在相处过程中,若你提出的要求,对方都有默默关注并改进,代表对方是很在意你的。金牛座:气定神闲,你能统筹安排好手头的事情,合理的走完日常行程,并且将积压已久的事情彻底解决,只是在处理文字协议的时候,要多留点心;感情上单身族或显太过矜持,建议要主动表达自己的想法,才
- 2023-05-11 关于科研
姐弟的老师妈妈
越来越觉得,科研并没有想象中那么难。为何呢?科研的过程不难。随着对科研的进一步深入了解发现:科研其实就是将自己在工作中遇到的问题——解决问题的方法、过程——问题解决后的收获做一个完整的记录。这其实是我们在工作中一直都在做的事情。科研过程的记录难。用最少的字表达清楚自己的想法,应该是科研成果能够称得上是成果,并可能被推广的精髓所在。从提出问题开始:科研题目就是明确的方向——让自己和旁人都能通过看见题
- 2023-02-06
暖暖de严严
中原焦点团队第33期中级班学员坚持分享第353天总约练124次来访者83观察员37咨询师4过了正月十五,这个年也就算过去了。早上起床,竟然也有种不想上班的感觉。当然,这只是一瞬间的想法,责任还是促使自己立马行动起来。审视自己的生活:我对自己的要求都达到了吗?那些列在计划表上的内容都在慢慢实施了吗?那些简单易行的生活好习惯,都在坚持吗?终生学习的任务,有在完成吗?最近工作开始忙碌,手上的工作都在按步
- 做有良心的教育——读《怎样教语文》今日所感
瑾言_lw999
“精彩的课堂教学应该是展示一个过程,而不是展示一个结果。”初读之时,没有太多感触;反复嚼读,心中波澜起伏。脑中突然冒出“”做有良心的教育”这一想法来,我又是否做到了有良心的教育呢?课堂之上,某个同学回答不出问题,我耐心地引导他了吗?教学效果不好之时,我深刻地反省自己了吗?学生的冲突与矛盾,我又是否打开了他们的心结?让他们不再有怨恨呢?我是教学生有了好的成绩还是培养了他的能力呢?诸多问题,一时涌现在
- 钟汉良日记:自媒体写作要向古代拜师学艺的人取经
钟汉良日记
2022年9月8日周四晴朗的天前几天晚上,和实操班的学员谈了很多为什么要写日记的原因。前后两个多小时,推荐了好几本书,隔了一天再回忆竟然不能把所有的书籍都列出来。是自己年龄大了,记忆力衰退了?我觉得不是。而是谈话这种氛围,比起文字写作更容易激发我们的潜能。一些灵光乍现的想法,会在那种特别融洽的时候突然涌现出来。有的想法和总结,你平时就是绞尽脑汁也想不出来,但就是在那种你侃侃而谈的时候,思想会像流水
- #启发日记#063 坏情绪并非坏事,里面也有宝藏
磁石在提问
今日启发问题:“当坏情绪来临的时候,你还会做些什么事?”月有阴晴圆缺,人有悲欢离合。每个人总会情绪不好的时候,我们会讨厌坏情绪。不过从另一个角度看,其实坏情绪也有一定价值。就是当坏情绪出现的时候,依然努力去做的事情,这件事情可能真的自己心里最看重的。当你情绪不好的时候,有理性的意识处在较弱的状态,看起来颓废,有些事情不做,有些事情做了又没做好,而此时潜意识的作用就出来了,用内心深处最自然的想法帮你
- 丁俊贵之《“女人和男人”那些事》
兴时态_198812
【“女人和男人”那些事】生活中,我们经常用性别来给很多现象和问题贴标签。比如:女性发脾气是常见的事情,所以不要跟她们讲道理,要让着她们;女性考虑问题总是比较感性,不如男性那么理性、严谨、全面;女生的数学成绩普遍比较差,因此选文科的女生更多;……许许多多像这样的认知,已经成为我们根深蒂固的信念。我们在生活中哪怕不会直接这样讲,但多多少少都会有类似的想法和感受,并且用这些信念去理解和认知他人。一、人世
- 【8月星座运势】巨蟹座金钱至上、天蝎贵人相助、双鱼座平衡万物
筝筝陪你看星星
本月重大天象8月2日星期二满月@水瓶座「集思广益、团体活动」水瓶座满月可能带来更强烈的情感和思维的挑战,存在更多的预料不及的突发事件,但同时也增加了对真理和公正的透视力。我们可能会感到更加亲近科技和革新的想法。对于自我实现,人道主义,团体活动和创新有着重要的影响。同时,满月会让我们进行反思,修改策略并将精力投入到这些领域。8月16日星期三新月@狮子座「展翅翱翔、积极自信」新月发生在狮子座是创造性,
- 最近在读《最好的决定》
碎沫
我想起上次我妈本来和我聊天,不知怎么说起那些不要孩子的人,然后她说别人自私自利,女生就是必须的生孩子,blabla……然后我就情绪激动,和她辩论,最后我俩吵起来了,我甚至想连夜逃跑。后来一想,我妈似乎心里明白我的想法吧,尽管我从来没和她讨论过这个话题。似乎这是她的试探……我在想,我自己要不要和父母说这个想法呢,母亲如此激烈的反应,我本来觉得这件事是我自己的事,可以不用说的。偶然看到这本书,所以我来
- 《京瓷哲学》关于开展日常工作读后感
平粤奇妙广东海岛and长隆
至此,又到了《京瓷哲学》这本书的尾声。读书历程,就像在感受一位父母,或者老师,或者是长辈的唠叨和教诲,长篇大论,孜是觉得这些人生的道理谁都会说道两句,当然这种想法很快被印证是错的,没有一颗博爱的心,无私的心,稻盛先生的理念甚至盛和塾可能将不存在。依旧是选择2点分享,第一点制造完美无瑕的产品。随着社会不断的前进发展,人们的标准不再停留于单纯拥有这个层面,而是想拥有更好的。小到日常用餐的摆盘,大到将房
- 我在他乡挺好的
栀伊婉湫
最近芒果上映的一部新剧,戳中了异乡人的泪点。乔夕辰经历自己半年房租被骗,好朋友自杀去世,自己马上被公司辞退,连夜加班,却依然和父母打电话时,说自己没事,一个人挺好的。每个异乡人,在他乡,同父母打电话,只报喜,却不报忧。同样,父母也是如此,他们只是都不想让彼此担心。异乡人把自己的梦想交付给另一个城市,他也是对自己下了赌注,要在城市,营造属于自己的小天地。可想法是好的,梦想是美的,但现实是骨感的,残酷
- 远离导致贫穷的习惯
小强聊成长
书籍:富有的习惯01.投机追求不劳而获的人,及时获得了一时的运气,长期来看,还是会陷入贫穷状态。那些长期买彩票的都是没钱的人。02.过度饮酒醉酒会影响脑细胞中神经传导接收器的工作,长期过度饮酒,会导致反应迟钝。影响人的记忆力和思考能力。03.过度沉迷于电视节目穷人靠电视剧打发时间,现在互联网时代,刷手机视频,就像是毒品,吸引着人们不断的刷。大量的时间都花在上面。04.消极心态想法消极的人,很难正视
- 《从怕学习到爱学习》多元思考力之科学思维
瑞蔓
一、收获新知1.科学思维是一种独立思考和开放的思考方式。2.提高科学思维的方法:家长给孩子独立空间,弱化孩子依赖性。孩子的新想法和疑问,不反对,不嘲笑。鼓励孩子发表意见和想法并一起讨论。引导孩子了解世界复杂性和多变性,万事都不绝对。二、改变就知1.总担心孩子小,好多事儿考虑不到,遇到事儿家长大包大揽,没有给孩子独处学会思考的能力。2.孩子有些疑问和想法,家长总感觉是孩子不成熟的想法,没有认真对待。
- 新媒体人#自媒体魂!新手到入门|一篇足矣
ph萝卜
最近已学习《新媒体写作平台策划与运营》课程,先梳理梳理学习感悟,后上满满的干货!希望阅读文章的你可以带来一丝想法,目的就达到了!想干成一件事,最靠谱的就是去认识一个已经做成了这件事情的人,或是认识与这件事情相关的人。做到不耻下问,足或有所长,你找他们聊天一小时,足以比你看多少书来的实际,就打个比方,我想利用我的空余时间做微信公众平台,我想到的是学习相关知识,包括编辑,美化,排版,运营,与其同时,我
- 连载《你在桥上看风景》五十八 海涛满地鸡毛的生活
塞上艾青
无戒学堂脑海里一次又一次闪过这样的画面,一脚将她踢翻在地或是一个巴掌向她扇过去。可是,过了一秒钟,这样的想法马上又被自己否定了。妻子被打后,马上就像猴子一样,张牙舞爪的向自己挠过来了,或是自己下手太狠了,她会号啕大哭起来,一边还会向将电话打给自己的母亲,这样的话,那位一向慈善的老母亲又会迈着蹒跚的步伐来收拾残局,或是充当一个调停者,或是直接朝自己的脸上回扇过来。那本不是母亲的本意,可是面对这样破碎
- 梁小妹:比芭布婴装创业连载69
服装厂梁姐
图片发自App今天要开始下一个季节的新款产品出货了,明明有好多想法的,明明有好多思路的,到了真的需要的时候,灵感不知道跑到哪里去了。今晚集中精力研究了一下硫酸纸包装袋,问了一个厂家,单价算下来价格太高了。虽然我一再强调不考虑成本,要注重颜值。可是一个袋子下来要贵了4毛钱,每条小毛巾的利润也就那么2--3毛钱,所以这个结果还是吓到了我。价格太高,就会没有销量。优质的产品,肯定是全方位的高成本的,我不
- 关于灵感的一些想法
高温若寒的坚持
灵感是个稍纵即逝的东西,如果不好好抓住,只能与它挥手告别。相信我们都有过这样的时刻,走着走着路或者吃着吃着饭时一个想法的出现,那就是灵感的再现。灵感虽然是个可遇不可求的东西,但我们可以通过一些途径增加灵感出现的概率。1.多和别人聊天。相信大家都知道《聊斋志异》这部作品,而蒲松龄创作这部小说的灵感多来自于与周围人的聊天之中。我们如果想增加灵感出现的概率,就要多和身边的人聊聊天,特别是和优秀的榜样人物
- ztree设置禁用节点
3213213333332132
JavaScriptztreejsonsetDisabledNodeAjax
ztree设置禁用节点的时候注意,当使用ajax后台请求数据,必须要设置为同步获取数据,否者会获取不到节点对象,导致设置禁用没有效果。
$(function(){
showTree();
setDisabledNode();
});
- JVM patch by Taobao
bookjovi
javaHotSpot
在网上无意中看到淘宝提交的hotspot patch,共四个,有意思,记录一下。
7050685:jsdbproc64.sh has a typo in the package name
7058036:FieldsAllocationStyle=2 does not work in 32-bit VM
7060619:C1 should respect inline and
- 将session存储到数据库中
dcj3sjt126com
sqlPHPsession
CREATE TABLE sessions (
id CHAR(32) NOT NULL,
data TEXT,
last_accessed TIMESTAMP NOT NULL,
PRIMARY KEY (id)
);
<?php
/**
* Created by PhpStorm.
* User: michaeldu
* Date
- Vector
171815164
vector
public Vector<CartProduct> delCart(Vector<CartProduct> cart, String id) {
for (int i = 0; i < cart.size(); i++) {
if (cart.get(i).getId().equals(id)) {
cart.remove(i);
- 各连接池配置参数比较
g21121
连接池
排版真心费劲,大家凑合看下吧,见谅~
Druid
DBCP
C3P0
Proxool
数据库用户名称 Username Username User
数据库密码 Password Password Password
驱动名
- [简单]mybatis insert语句添加动态字段
53873039oycg
mybatis
mysql数据库,id自增,配置如下:
<insert id="saveTestTb" useGeneratedKeys="true" keyProperty="id"
parameterType=&
- struts2拦截器配置
云端月影
struts2拦截器
struts2拦截器interceptor的三种配置方法
方法1. 普通配置法
<struts>
<package name="struts2" extends="struts-default">
&
- IE中页面不居中,火狐谷歌等正常
aijuans
IE中页面不居中
问题是首页在火狐、谷歌、所有IE中正常显示,列表页的页面在火狐谷歌中正常,在IE6、7、8中都不中,觉得可能那个地方设置的让IE系列都不认识,仔细查看后发现,列表页中没写HTML模板部分没有添加DTD定义,就是<!DOCTYPE html PUBLIC "-//W3C//DTD XHTML 1.0 Transitional//EN" "http://www.w3
- String,int,Integer,char 几个类型常见转换
antonyup_2006
htmlsql.net
如何将字串 String 转换成整数 int?
int i = Integer.valueOf(my_str).intValue();
int i=Integer.parseInt(str);
如何将字串 String 转换成Integer ?
Integer integer=Integer.valueOf(str);
如何将整数 int 转换成字串 String ?
1.
- PL/SQL的游标类型
百合不是茶
显示游标(静态游标)隐式游标游标的更新和删除%rowtyperef游标(动态游标)
游标是oracle中的一个结果集,用于存放查询的结果;
PL/SQL中游标的声明;
1,声明游标
2,打开游标(默认是关闭的);
3,提取数据
4,关闭游标
注意的要点:游标必须声明在declare中,使用open打开游标,fetch取游标中的数据,close关闭游标
隐式游标:主要是对DML数据的操作隐
- JUnit4中@AfterClass @BeforeClass @after @before的区别对比
bijian1013
JUnit4单元测试
一.基础知识
JUnit4使用Java5中的注解(annotation),以下是JUnit4常用的几个annotation: @Before:初始化方法 对于每一个测试方法都要执行一次(注意与BeforeClass区别,后者是对于所有方法执行一次)@After:释放资源 对于每一个测试方法都要执行一次(注意与AfterClass区别,后者是对于所有方法执行一次
- 精通Oracle10编程SQL(12)开发包
bijian1013
oracle数据库plsql
/*
*开发包
*包用于逻辑组合相关的PL/SQL类型(例如TABLE类型和RECORD类型)、PL/SQL项(例如游标和游标变量)和PL/SQL子程序(例如过程和函数)
*/
--包用于逻辑组合相关的PL/SQL类型、项和子程序,它由包规范和包体两部分组成
--建立包规范:包规范实际是包与应用程序之间的接口,它用于定义包的公用组件,包括常量、变量、游标、过程和函数等
--在包规
- 【EhCache二】ehcache.xml配置详解
bit1129
ehcache.xml
在ehcache官网上找了多次,终于找到ehcache.xml配置元素和属性的含义说明文档了,这个文档包含在ehcache.xml的注释中!
ehcache.xml : http://ehcache.org/ehcache.xml
ehcache.xsd : http://ehcache.org/ehcache.xsd
ehcache配置文件的根元素是ehcahe
ehcac
- java.lang.ClassNotFoundException: org.springframework.web.context.ContextLoaderL
白糖_
javaeclipsespringtomcatWeb
今天学习spring+cxf的时候遇到一个问题:在web.xml中配置了spring的上下文监听器:
<listener>
<listener-class>org.springframework.web.context.ContextLoaderListener</listener-class>
</listener>
随后启动
- angular.element
boyitech
AngularJSAngularJS APIangular.element
angular.element
描述: 包裹着一部分DOM element或者是HTML字符串,把它作为一个jQuery元素来处理。(类似于jQuery的选择器啦) 如果jQuery被引入了,则angular.element就可以看作是jQuery选择器,选择的对象可以使用jQuery的函数;如果jQuery不可用,angular.e
- java-给定两个已排序序列,找出共同的元素。
bylijinnan
java
import java.util.ArrayList;
import java.util.Arrays;
import java.util.List;
public class CommonItemInTwoSortedArray {
/**
* 题目:给定两个已排序序列,找出共同的元素。
* 1.定义两个指针分别指向序列的开始。
* 如果指向的两个元素
- sftp 异常,有遇到的吗?求解
Chen.H
javajcraftauthjschjschexception
com.jcraft.jsch.JSchException: Auth cancel
at com.jcraft.jsch.Session.connect(Session.java:460)
at com.jcraft.jsch.Session.connect(Session.java:154)
at cn.vivame.util.ftp.SftpServerAccess.connec
- [生物智能与人工智能]神经元中的电化学结构代表什么?
comsci
人工智能
我这里做一个大胆的猜想,生物神经网络中的神经元中包含着一些化学和类似电路的结构,这些结构通常用来扮演类似我们在拓扑分析系统中的节点嵌入方程一样,使得我们的神经网络产生智能判断的能力,而这些嵌入到节点中的方程同时也扮演着"经验"的角色....
我们可以尝试一下...在某些神经
- 通过LAC和CID获取经纬度信息
dai_lm
laccid
方法1:
用浏览器打开http://www.minigps.net/cellsearch.html,然后输入lac和cid信息(mcc和mnc可以填0),如果数据正确就可以获得相应的经纬度
方法2:
发送HTTP请求到http://www.open-electronics.org/celltrack/cell.php?hex=0&lac=<lac>&cid=&
- JAVA的困难分析
datamachine
java
前段时间转了一篇SQL的文章(http://datamachine.iteye.com/blog/1971896),文章不复杂,但思想深刻,就顺便思考了一下java的不足,当砖头丢出来,希望引点和田玉。
-----------------------------------------------------------------------------------------
- 小学5年级英语单词背诵第二课
dcj3sjt126com
englishword
money 钱
paper 纸
speak 讲,说
tell 告诉
remember 记得,想起
knock 敲,击,打
question 问题
number 数字,号码
learn 学会,学习
street 街道
carry 搬运,携带
send 发送,邮寄,发射
must 必须
light 灯,光线,轻的
front
- linux下面没有tree命令
dcj3sjt126com
linux
centos p安装
yum -y install tree
mac os安装
brew install tree
首先来看tree的用法
tree 中文解释:tree
功能说明:以树状图列出目录的内容。
语 法:tree [-aACdDfFgilnNpqstux][-I <范本样式>][-P <范本样式
- Map迭代方式,Map迭代,Map循环
蕃薯耀
Map循环Map迭代Map迭代方式
Map迭代方式,Map迭代,Map循环
>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>
蕃薯耀 2015年
- Spring Cache注解+Redis
hanqunfeng
spring
Spring3.1 Cache注解
依赖jar包:
<!-- redis -->
<dependency>
<groupId>org.springframework.data</groupId>
<artifactId>spring-data-redis</artifactId>
- Guava中针对集合的 filter和过滤功能
jackyrong
filter
在guava库中,自带了过滤器(filter)的功能,可以用来对collection 进行过滤,先看例子:
@Test
public void whenFilterWithIterables_thenFiltered() {
List<String> names = Lists.newArrayList("John"
- 学习编程那点事
lampcy
编程androidPHPhtml5
一年前的夏天,我还在纠结要不要改行,要不要去学php?能学到真本事吗?改行能成功吗?太多的问题,我终于不顾一切,下定决心,辞去了工作,来到传说中的帝都。老师给的乘车方式还算有效,很顺利的就到了学校,赶巧了,正好学校搬到了新校区。先安顿了下来,过了个轻松的周末,第一次到帝都,逛逛吧!
接下来的周一,是我噩梦的开始,学习内容对我这个零基础的人来说,除了勉强完成老师布置的作业外,我已经没有时间和精力去
- 架构师之流处理---------bytebuffer的mark,limit和flip
nannan408
ByteBuffer
1.前言。
如题,limit其实就是可以读取的字节长度的意思,flip是清空的意思,mark是标记的意思 。
2.例子.
例子代码:
String str = "helloWorld";
ByteBuffer buff = ByteBuffer.wrap(str.getBytes());
Sy
- org.apache.el.parser.ParseException: Encountered " ":" ": "" at line 1, column 1
Everyday都不同
$转义el表达式
最近在做Highcharts的过程中,在写js时,出现了以下异常:
严重: Servlet.service() for servlet jsp threw exception
org.apache.el.parser.ParseException: Encountered " ":" ": "" at line 1,
- 用Java实现发送邮件到163
tntxia
java实现
/*
在java版经常看到有人问如何用javamail发送邮件?如何接收邮件?如何访问多个文件夹等。问题零散,而历史的回复早已经淹没在问题的海洋之中。
本人之前所做过一个java项目,其中包含有WebMail功能,当初为用java实现而对javamail摸索了一段时间,总算有点收获。看到论坛中的经常有此方面的问题,因此把我的一些经验帖出来,希望对大家有些帮助。
此篇仅介绍用
- 探索实体类存在的真正意义
java小叶檀
POJO
一. 实体类简述
实体类其实就是俗称的POJO,这种类一般不实现特殊框架下的接口,在程序中仅作为数据容器用来持久化存储数据用的
POJO(Plain Old Java Objects)简单的Java对象
它的一般格式就是
public class A{
private String id;
public Str