第十四周项目6 判断是否为二叉排序树

/*
* Copyright (c)2015,烟台大学计算机与控制工程学院
* All rights reserved.
* 文件名称：项目4.cbp
* 作    者：朱希康
* 完成日期：2015年12月14日
* 版 本 号：v1.0
* 问题描述：构造二叉排序树，替换一个元素后判断是否为二叉排序树
* 输入描述：无
* 程序输出：判断是否为二叉排序树
*/

#include <stdio.h>
#include <malloc.h>
#define MaxSize 100
typedef int KeyType;                    //定义关键字类型
typedef char InfoType;
typedef struct node                     //记录类型
{
    KeyType key;                        //关键字项
    InfoType data;                      //其他数据域
    struct node *lchild,*rchild;        //左右孩子指针
} BSTNode;
int path[MaxSize];                      //全局变量,用于存放路径
void DispBST(BSTNode *b);               //函数说明
int InsertBST(BSTNode *&p,KeyType k)    //在以*p为根节点的BST中插入一个关键字为k的节点
{
    if (p==NULL)                        //原树为空, 新插入的记录为根节点
    {
        p=(BSTNode *)malloc(sizeof(BSTNode));
        p->key=k;
        p->lchild=p->rchild=NULL;
        return 1;
    }
    else if (k==p->key)
        return 0;
    else if (k<p->key)
        return InsertBST(p->lchild,k);  //插入到*p的左子树中
    else
        return InsertBST(p->rchild,k);  //插入到*p的右子树中
}
BSTNode *CreatBST(KeyType A[],int n)
//由数组A中的关键字建立一棵二叉排序树
{
    BSTNode *bt=NULL;                   //初始时bt为空树
    int i=0;
    while (i<n)
        InsertBST(bt,A[i++]);       //将A[i]插入二叉排序树T中
    return bt;                          //返回建立的二叉排序树的根指针
}

void DispBST(BSTNode *bt)
//以括号表示法输出二叉排序树bt
{
    if (bt!=NULL)
    {
        printf("%d",bt->key);
        if (bt->lchild!=NULL || bt->rchild!=NULL)
        {
            printf("(");
            DispBST(bt->lchild);
            if (bt->rchild!=NULL) printf(",");
            DispBST(bt->rchild);
            printf(")");
        }
    }
}

/*
int JudgeBST(BSTNode *bt)为判断一个树是否为排序二叉树设计的算法的实现
*/
KeyType predt=-32767; //predt为全局变量,保存当前节点中序前趋的值,初值为-∞
int JudgeBST(BSTNode *bt)   //判断bt是否为BST
{
    int b1,b2;
    if (bt==NULL)
        return 1;    //空二叉树是排序二叉树
    else
    {
        b1=JudgeBST(bt->lchild);   //返回对左子树的判断，非排序二叉树返回0，否则返回1
        if (b1==0 || predt>=bt->key)  //当左子树非排序二叉树，或中序前趋（全局变量）大于当前根结点时
            return 0;    //返回“不是排序二叉树”
        predt=bt->key;   //记录当前根为右子树的中序前趋
        b2=JudgeBST(bt->rchild);   //对右子树进行判断
        return b2;
    }
}

int main()
{
    BSTNode *bt;
    int a[]= {43,91,10,18,82,65,33,59,27,73},n=10;
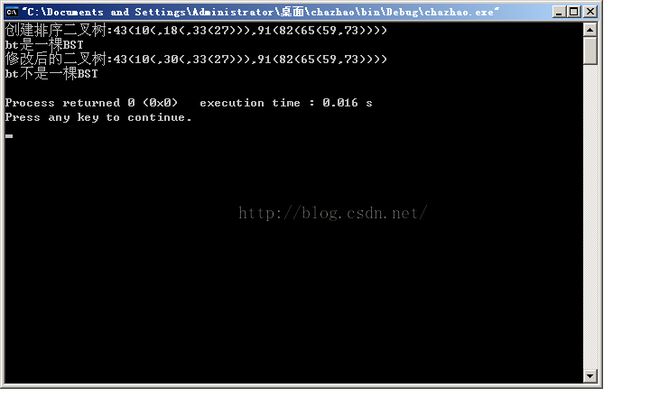
    printf("创建排序二叉树:");
    bt=CreatBST(a,n);
    DispBST(bt);
    printf("\n");
    printf("bt%s\n",(JudgeBST(bt)?"是一棵BST":"不是一棵BST"));
    bt->lchild->rchild->key = 30;  //搞个破坏！
    printf("修改后的二叉树:");
    DispBST(bt);
    printf("\n");
    printf("bt%s\n",(JudgeBST(bt)?"是一棵BST":"不是一棵BST"));
    return 0;
}

运行结果：

知识点总结：

判断是否为二叉树即判断该节点为左孩子还是右孩子，再和其根节点进行比较。