- 致良知之寄诸用明书
BonSun
众所周知,当今社会,父母和社会、学校对学生的期望往往是唯分数论,包括每个人对成功的理解也往往是功名利禄,忽视了最基本的学问。文中提到,花之千叶者无实,为其华美太发露耳。人只有沉下心来,韬光养晦,才能拥有真正的学问和本领。
- Python【math数学函数】
Alan_Lowe
#Pythonpython
Python【math数学函数】文章目录Python【math数学函数】数论与表示函数1.ceil()和floor()2.comb()3.copysign()4.fabs()5.factorial()6.gcd()7.lcm()幂函数与对数函数1.exp()和math.e和pow()2.log()和log2()和log10()3.sqrt(x)三角函数1.asin、acos()、atan()2.s
- python 实现eulers totient欧拉方程算法
luthane
算法python开发语言
eulerstotient欧拉方程算法介绍欧拉函数(Euler’sTotientFunction),通常表示为(),是一个与正整数相关的函数,它表示小于或等于的正整数中与互质的数的数目。欧拉函数在数论和密码学中有广泛的应用。欧拉函数的性质1.**对于质数,有φ(p)=p−1∗∗φ(p)=p−1^{**}φ(p)=p−1∗∗。2.**如果是质数的次幂,即n=pkn=p^kn=pk,则φ(n)=pk−
- 11.4 看不懂就慢慢看啊
反复练习的阿离很笨吧
记得组合数学正交拉丁方从0开始!突然觉得老师说得很有道理,演化计算里活得最好的,不是最优秀的但也不是最差的,是最能适应环境的,别人怎么做,他就怎么做。动态规划,运筹学贝叶斯是生成学习算法,生成一个概率模型判别学习算法高斯判别分析/**NB.java*Copyright2005LiangxiaoJiang*/packageweka.classifiers.gla;importweka.core.*;
- 算法设计与分析学习(6)——数论
罗塞菈桔梨萝柚
算法学习算法线性代数
数论整除基本概念若aaa和bbb为整数,且a≠0a≠0a=0若存在整数qqq使得b=aqb=aqb=aq,那么就说aaa可以整除bbb或是bbb被aaa整除,记作a∣ba|ba∣b。aaa也被称为bbb的约数,bbb也被称为a的倍数。若bbb不能被aaa整除,则记作a∤ba\not{|}ba∣b。整数p≠0,±1p≠0,±1p=0,±1,且除了±1,±p±1,±p±1,±p外没有其他的约数
- 数论——欧几里得算法
NarutoTime
数论算法c++数据结构c语言
1.欧几里得简介 欧几里得(希腊文:Ευκλειδης,约公元前330年—公元前275年),古希腊数学家,被称为“几何之父”。他最著名的著作《几何原本》是欧洲数学的基础,在书中他提出五大公设。欧几里得的《几何原本》被广泛的认为是历史上最成功的教科书。欧几里得也写了一些关于透视、圆锥曲线、球面几何学及数论的作品。2.欧几里得算法欧几里得算法用于:求解a和b的最大公约数。最大公约数英文为:Gre
- 数论——扩展欧几里得算法
NOI_yzk
欧几里得&拓展欧几里得(Euclid&Extend-Euclid)欧几里得算法(Euclid)背景:欧几里德算法又称辗转相除法,用于计算两个正整数a,b的最大公约数。——百度百科代码:递推的代码是相当的简洁:intgcd(inta,intb){returnb==0?a:gcd(b,a%b);}分析:方法说了是辗转相除法,自然没有什么好介绍的了。。Fresh肯定会觉得这样递归下去会不会爆栈?实际上在
- 数论学习1(欧几里德算法+唯一分解定理+埃氏筛+拓展欧几里德+同余与模算术)
new出新对象!
数学数算法学习
目录1.唯一分解定理2.欧几里德算法(求最大公约数)3.求最小公倍数4.埃氏筛5.拓展欧几里德算法(1)证明一下线性方程组的正数的最小值是多少,(2)如何通过裴蜀定理退出拓展欧几里得算法(贝祖定理)6.同余与模算术(1)取模运算操作加法取模运算减法取模运算乘法取模运算(2)特殊的取模操作大整数取模幂取模(3)同余式,乘法逆元,费马小定理今天也是小小的开始学习数论方面的知识了,首先数论的入门章节必然
- Collatz 猜想和 Python
不连续小姐
PythonDay4:CollatzConjecture原来总有学生问我,微积分有什么用啊,我说如果微积分学好了,也许抽象代数和数论就能学好,那最后就能像AndrewWiles一样上人物年度杂志的封面了.(AndrewWiles证明了Fermat'sLastTheorem,费玛大定理).[captionid="attachment_1466"align="alignnone"width="300"
- 初等数论--整除--带余除法
WeidanJi
初等数论数学密码学信息安全
初等数论--整除--带余除法概念基本性质带余除法博主本人是初学初等数论(整除+同余+原根),本意是想整理一些较难理解的定理、算法,加深记忆也方便日后查找;如果有错,欢迎指正。我整理成一个系列:初等数论,方便检索。概念初等数论研究对象是整数集合和自然数集合。初等数论研究对象是整数集合和自然数集合。初等数论研究对象是整数集合和自然数集合。b∣a:若a,b∈Z,b≠0,∃c∈Z,使a=bc,则称b整除a
- 信息学奥赛初赛天天练-26-CSP-J2023基础题攻略,组合数学、高精度算法、计算机存储奥秘与操作系统实践
ya888g
信息学奥赛初赛算法组合数学高精度算法信息学奥赛
PDF文档公众号回复关键字:20240611单项选择题(共15题,每题2分,共计30分:每题有且仅有一个正确选项)6小明在某一天中依次有七个空闲时间段,他想要选出至少一个空闲时间段来练习唱歌,但他希望任意两个练习的时间段之间都有至少两个空闲的时间段让他休息,则小明一共有()种选择时间段的方案。A31B18C21D337以下关于高精度运算的说法错误的是()。A高精度计算主要是用来处理大整数或需要保留
- 河南萌新2024第四场
Pown_ShanYu
算法数据结构
C岗位分配题目大意:有n个岗位,m位志愿者,每个岗位至少需要a个志愿者,并且可以有志愿者可以空闲下来作预备,给出可能的分配情况总数思路:首先将每个岗位分配好至少需要的志愿者,再将剩下的人进行分配,那就满足球同盒不同模型(允许空盒),可用隔板法进行分配,需要额外开设一个空闲岗位用来预备,那么按照4个人去4个岗位,那么为c73,具体操作可看数论模板中发布的隔板法问题,递归求组合数Solved:intn
- 【读书笔记】吴非《致青年教师》(4)
冬儿菇凉
一、精要摘录(48——106页)1.教育教学不能“唯分数论“,比分数重要的是学生思维品质和解决实际问题的能力。2.一名教师心中有使命感,心中有学生才会很在意学生对他的态度,在意学生的接受度。3.作为教师,你要善于向学生问出有意思的问题。4.教育就是要培养好习惯,教是为了达到不需要教学生,不需要老师教了是教学的成功,也是教师的职业追求。5.教师是学习者,在学习上教师首先要郑重其事,学生才有可能养成敬
- 【洛谷 P8649】[蓝桥杯 2017 省 B] k 倍区间 题解(前缀和+同余定理+组合数学)
HEX9CF
AlgorithmProblems蓝桥杯职场和发展
[蓝桥杯2017省B]k倍区间题目描述给定一个长度为NNN的数列,A1,A2,⋯ANA_1,A_2,\cdotsA_NA1,A2,⋯AN,如果其中一段连续的子序列Ai,Ai+1,⋯Aj(i≤j)A_i,A_{i+1},\cdotsA_j(i\lej)Ai,Ai+1,⋯Aj(i≤j)之和是KKK的倍数,我们就称这个区间[i,j][i,j][i,j]是KKK倍区间。你能求出数列中总共有多少个KKK倍区
- 【代码随想录算法训练营Day39】62.不同路径;63. 不同路径 II
想做一只潜水的猪
算法
文章目录❇️Day39第九章动态规划part02✴️今日任务❇️62.不同路径自己的思路自己的代码随想录思路随想录代码❇️63.不同路径II自己的思路自己的代码随想录代码❇️Day39第九章动态规划part02✴️今日任务今天开始逐渐有dp的感觉了,题目不多,就两个不同路径,可以好好研究一下62.不同路径63.不同路径II❇️62.不同路径本题大家掌握动态规划的方法就可以。数论方法有点非主流,很难
- 算法D39 | 动态规划2 | 62.不同路径 63. 不同路径 II
memolaner
算法训练营算法动态规划数据结构c++python
今天开始逐渐有dp的感觉了,题目不多,就两个不同路径,可以好好研究一下62.不同路径本题大家掌握动态规划的方法就可以。数论方法有点非主流,很难想到。代码随想录视频讲解:动态规划中如何初始化很重要!|LeetCode:62.不同路径_哔哩哔哩_bilibili这个题看到路径的表示,第一直觉就是一个组合数的问题,学了一下C++计算组合数防止溢出的小技巧。第二个方法再动态规划完成,重点是把二维的动态规划
- 牛客周赛 Round 35(A,B,C,D,E,F,G)
邪神与厨二病
牛客算法暴力c++数论滑动窗口单调队列贪心构造
这场简单,甚至赛时90分钟不到就AK了。比赛链接,队友题解友链刚入住学校监狱,很不适应,最近难受的要死,加上最近几场CF打的都不顺利,san值要爆掉了,只能慢慢补题了。这场C是个滑动窗口,D是贪心,E是有点麻烦的构造,FG是数论。A小红的字符串切割思路:记录一下字符串长度,然后从中间拆开。code:#include#include#includeusingnamespacestd;strings;
- 算法——数论——同余
戏拈秃笔
数据结构与算法(java版)算法
目录同余一、试题算法训练同余方程同余同余使人们能够用等式的形式简洁地描述整除关系同余:若m(正整数),a和b是整数,a%m==b%m,或(a-b)%m==0,记为ab(modm)求解一元线性同余方程等价于求解二元线性丢番图方程一元线性同余方程,解法看下面第一题二元线性丢番图方程逆:的一个解为a模m的逆一、试题算法训练同余方程问题描述求关于x的同余方程ax≡1(modb)的最小正整数解。输入格式输入
- 算法——组合数学——二项式定理
戏拈秃笔
数据结构与算法(java版)算法
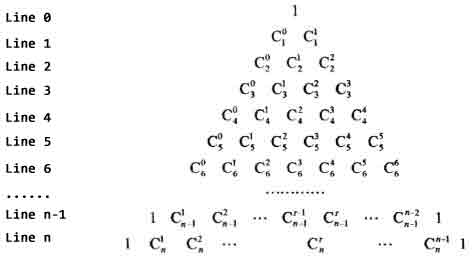
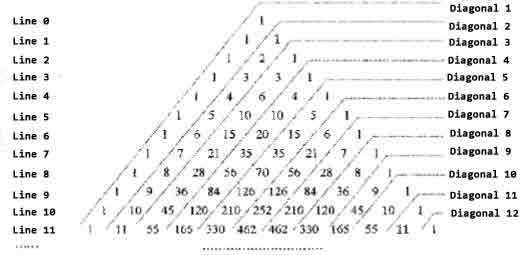
杨辉三角是二项式系数的典型应用当n较大,且需要取模时,二项式系数有两种计算方法:一:递推公式,二:逆方法一:用递推公式计算二项式系数publicclassBinomialCoefficient{publicstaticintcalculate(intn,intk){if(k>n){return0;//如果k大于n,则二项式系数为0}int[][]dp=newint[n+1][k+1];for(in
- pku acm 题目分类
moxiaomomo
算法数据结构numbers优化calendarcombinations
1.搜索//回溯2.DP(动态规划)3.贪心北大ACM题分类2009-01-2714.图论//Dijkstra、最小生成树、网络流5.数论//解模线性方程6.计算几何//凸壳、同等安置矩形的并的面积与周长sp;7.组合数学//Polya定理8.模拟9.数据结构//并查集、堆sp;10.博弈论1、排序sp;1423,1694,1723,1727,1763,1788,1828,1838,1840,22
- C++STL之Queue容器
芯片烧毁大师
数据结构C++c++开发语言
C++STL之Queue容器1.再谈队列回顾一下之前所学的队列,队列和栈不同,队列是一种先进先出的数据结构,STL的队列内容极其重要,虽然内容较少但是请务必掌握,STL的队列是快速构建搜索算法以及相关的数论图论的状态存储的基础。2.相关头文件头文件:#include3.初始化格式为:**explicit**queue(**const**container_type&ctnr=container_t
- 世界顶级名校计算机专业,都在用哪些书当教材?(文末送书)
小尘要自信
java开发语言数据库算法赠书计算机组成
目录01《深入理解计算机系统》02《算法导论》03《计算机程序的构造和解释》04《数据库系统概念》05《计算机组成与设计:硬件/软件接口》06《离散数学及其应用》07《组合数学》08《斯坦福算法博弈论二十讲》参与规则清华、北大、MIT、CMU、斯坦福的学霸们在新学期里要学什么?今天我们来盘点一下那些世界名校计算机专业采用的教材。01《深入理解计算机系统》原书第3版)作者:兰德尔E.布莱恩特大卫R.
- 数字签名算法MD5withRSA
Just_Paranoid
技术流Clipmd5rsasignatrue
数字签名MD5withRSA,:将正文通过MD5数字摘要后,将密文再次通过生成的RSA密钥加密,生成数字签名,将明文与密文以及公钥发送给对方,对方拿到私钥/公钥对数字签名进行解密,然后解密后的,与明文经过MD5加密进行比较,如果一致则通过使用Signature的API来实现MD5withRSARSA原理:RSA算法基于一个十分简单的数论事实,将两个大素数相乘十分容易,但反过来想要对其乘积进行因式分
- 2301: 不定方程解的个数
jht0105
算法
题目描述输出不定方程解的个数。在数学中,不定方程是数论中的一个重要课题,在各种比赛中也常常出现.对于不定方程,有时我们往往只求非负整数解,现有方程ax+by+c=0,其中x、y为未知量且不超过10000,当给定a、b、c的值以后,可求出n组x、y的非负整数解,n>=0,,其中a,b,c均为[-10000,10000].输入描述一行,三个空格隔开的整数,为a、b、c的值。输出描述一个整数,为合法的解
- python伯努利多项式
微小冷
#sympypython开发语言sympy伯努利数排列组合符号计算
文章目录伯努利数和多项式sympy实现伯努利数是一种在数学、物理和工程中广泛应用的特殊数列,以瑞士数学家雅各布·伯努利(JacobBernoulli)的名字命名,并在许多领域中发挥重要作用。在数学中,它们与斐波那契数列、卡塔兰数、贝尔数等数列有密切联系,可以用于解决循环问题、组合问题和递推关系等数学问题。伯努利数和多项式伯努利(Bernoulli)数是一组在数论和复分析中出现的数,与伯努利多项式有
- 二次剩余问题x的求解及代码实现(python)
JustGo12
数论安全1024程序员节
一、问题引入二次剩余是数论基本概念之一。它是初等数论中非常重要的结果,不仅可用来判断二次同余式是否有解,还有很多用途。C.F.高斯称它为算术中的宝石,他一人先后给出多个证明。[1]研究二次剩余的理论称为二次剩余理论。二次剩余理论在实际上有广泛的应用,包括从噪音工程学到密码学以及大数分解。即关于方x^2≡a(modp)对于这个方程,求出满足条件的x。二、x的求解在上述问题下,根据p值的不同性质,可以
- 【数论】exgcd 扩展欧几里得算法
Texcavator
数论算法
参考:exgcd详解-zzt1208-博客园(cnblogs.com)exgcd(扩展欧几里得算法),用来求形如ax+by=gcd(a,b)ax+by=gcd(a,b)ax+by=gcd(a,b)(a,ba,ba,b为常数)的方程的一组整数解。(如果不确定等号右边是不是gcd,可以先当做gcd,求出来之后验证,是的话就是解,不是的话就不是解)推导见上面的链接,这篇只放个板子codeintexgcd
- 混乱的数组 蓝桥杯2024省赛 题解
鱼香猫猫头
蓝桥杯算法数据结构pythonjavac++c语言
混乱的数组题意思路如下:①优先考虑特殊情况,数组是一个严格递减的数组(每个数字之间相差1,最后一位必须为1),例子如下:长度为7,对应的数组为[7,6,5,4,3,2,1],根据组合数学C(7,2)=21,共有21对逆序对长度为8,对应的数组为[8,7,6,5,4,3,2,1],根据组合数学C(8,2)=28,共有28对逆序对假设逆序对个数为x时,当x∈(21,28],其数组长度为8;当x=21时
- 备战蓝桥杯---组合数学2
cocoack
蓝桥杯算法数学c++
本专题主要介绍容斥原理。大家高中的时候肯定接触过韦恩图,容斥原理比较通俗的理解就是减去所有可能并加上重叠的部分。我们直接看公式:知道后,我们先看道模板题:下面是AC代码:#includeusingnamespacestd;#defineintlonglonginta[6],n;signedmain(){a[0]=2;a[1]=5;a[2]=11;a[3]=13;while(cin>>n){ints
- LeetCode62不同路径解题记录
shuangge666666
java数据结构动态规划leetcode算法
LeetCode62.不同路径解题感想一.题目介绍二.解题思路及代码实现方法一:深度优先搜索(dfs)方法二:记忆化搜索方法三:动态规划方法四:组合数学法总结一.题目介绍题目链接:LeetCode62.不同路径;二.解题思路及代码实现方法一:深度优先搜索(dfs)由于是求从一个点到另一个点的路径有多少条,显而易见,可以采用深度优先搜索的方式,遍历所有路径,如果能够到达目标坐标的路径并统计路径数目然
- java观察者模式
3213213333332132
java设计模式游戏观察者模式
观察者模式——顾名思义,就是一个对象观察另一个对象,当被观察的对象发生变化时,观察者也会跟着变化。
在日常中,我们配java环境变量时,设置一个JAVAHOME变量,这就是被观察者,使用了JAVAHOME变量的对象都是观察者,一旦JAVAHOME的路径改动,其他的也会跟着改动。
这样的例子很多,我想用小时候玩的老鹰捉小鸡游戏来简单的描绘观察者模式。
老鹰会变成观察者,母鸡和小鸡是
- TFS RESTful API 模拟上传测试
ronin47
TFS RESTful API 模拟上传测试。
细节参看这里:https://github.com/alibaba/nginx-tfs/blob/master/TFS_RESTful_API.markdown
模拟POST上传一个图片:
curl --data-binary @/opt/tfs.png http
- PHP常用设计模式单例, 工厂, 观察者, 责任链, 装饰, 策略,适配,桥接模式
dcj3sjt126com
设计模式PHP
// 多态, 在JAVA中是这样用的, 其实在PHP当中可以自然消除, 因为参数是动态的, 你传什么过来都可以, 不限制类型, 直接调用类的方法
abstract class Tiger {
public abstract function climb();
}
class XTiger extends Tiger {
public function climb()
- hibernate
171815164
Hibernate
main,save
Configuration conf =new Configuration().configure();
SessionFactory sf=conf.buildSessionFactory();
Session sess=sf.openSession();
Transaction tx=sess.beginTransaction();
News a=new
- Ant实例分析
g21121
ant
下面是一个Ant构建文件的实例,通过这个实例我们可以很清楚的理顺构建一个项目的顺序及依赖关系,从而编写出更加合理的构建文件。
下面是build.xml的代码:
<?xml version="1
- [简单]工作记录_接口返回405原因
53873039oycg
工作
最近调接口时候一直报错,错误信息是:
responseCode:405
responseMsg:Method Not Allowed
接口请求方式Post.
- 关于java.lang.ClassNotFoundException 和 java.lang.NoClassDefFoundError 的区别
程序员是怎么炼成的
真正完成类的加载工作是通过调用 defineClass来实现的;
而启动类的加载过程是通过调用 loadClass来实现的;
就是类加载器分为加载和定义
protected Class<?> findClass(String name) throws ClassNotFoundExcept
- JDBC学习笔记-JDBC详细的操作流程
aijuans
jdbc
所有的JDBC应用程序都具有下面的基本流程: 1、加载数据库驱动并建立到数据库的连接。 2、执行SQL语句。 3、处理结果。 4、从数据库断开连接释放资源。
下面我们就来仔细看一看每一个步骤:
其实按照上面所说每个阶段都可得单独拿出来写成一个独立的类方法文件。共别的应用来调用。
1、加载数据库驱动并建立到数据库的连接:
Html代码
St
- rome创建rss
antonyup_2006
tomcatcmsxmlstrutsOpera
引用
1.RSS标准
RSS标准比较混乱,主要有以下3个系列
RSS 0.9x / 2.0 : RSS技术诞生于1999年的网景公司(Netscape),其发布了一个0.9版本的规范。2001年,RSS技术标准的发展工作被Userland Software公司的戴夫 温那(Dave Winer)所接手。陆续发布了0.9x的系列版本。当W3C小组发布RSS 1.0后,Dave W
- html表格和表单基础
百合不是茶
html表格表单meta锚点
第一次用html来写东西,感觉压力山大,每次看见别人发的都是比较牛逼的 再看看自己什么都还不会,
html是一种标记语言,其实很简单都是固定的格式
_----------------------------------------表格和表单
表格是html的重要组成部分,表格用在body里面的
主要用法如下;
<table>
&
- ibatis如何传入完整的sql语句
bijian1013
javasqlibatis
ibatis如何传入完整的sql语句?进一步说,String str ="select * from test_table",我想把str传入ibatis中执行,是传递整条sql语句。
解决办法:
<
- 精通Oracle10编程SQL(14)开发动态SQL
bijian1013
oracle数据库plsql
/*
*开发动态SQL
*/
--使用EXECUTE IMMEDIATE处理DDL操作
CREATE OR REPLACE PROCEDURE drop_table(table_name varchar2)
is
sql_statement varchar2(100);
begin
sql_statement:='DROP TABLE '||table_name;
- 【Linux命令】Linux工作中常用命令
bit1129
linux命令
不断的总结工作中常用的Linux命令
1.查看端口被哪个进程占用
通过这个命令可以得到占用8085端口的进程号,然后通过ps -ef|grep 进程号得到进程的详细信息
netstat -anp | grep 8085
察看进程ID对应的进程占用的端口号
netstat -anp | grep 进程ID
&
- 优秀网站和文档收集
白糖_
网站
集成 Flex, Spring, Hibernate 构建应用程序
性能测试工具-JMeter
Hmtl5-IOCN网站
Oracle精简版教程网站
鸟哥的linux私房菜
Jetty中文文档
50个jquery必备代码片段
swfobject.js检测flash版本号工具
- angular.extend
boyitech
AngularJSangular.extendAngularJS API
angular.extend 复制src对象中的属性去dst对象中. 支持多个src对象. 如果你不想改变一个对象,你可以把dst设为空对象{}: var object = angular.extend({}, object1, object2). 注意: angular.extend不支持递归复制. 使用方法: angular.extend(dst, src); 参数:
- java-谷歌面试题-设计方便提取中数的数据结构
bylijinnan
java
网上找了一下这道题的解答,但都是提供思路,没有提供具体实现。其中使用大小堆这个思路看似简单,但实现起来要考虑很多。
以下分别用排序数组和大小堆来实现。
使用大小堆:
import java.util.Arrays;
public class MedianInHeap {
/**
* 题目:设计方便提取中数的数据结构
* 设计一个数据结构,其中包含两个函数,1.插
- ajaxFileUpload 针对 ie jquery 1.7+不能使用问题修复版本
Chen.H
ajaxFileUploadie6ie7ie8ie9
jQuery.extend({
handleError: function( s, xhr, status, e ) {
// If a local callback was specified, fire it
if ( s.error ) {
s.error.call( s.context || s, xhr, status, e );
}
- [机器人制造原则]机器人的电池和存储器必须可以替换
comsci
制造
机器人的身体随时随地可能被外来力量所破坏,但是如果机器人的存储器和电池可以更换,那么这个机器人的思维和记忆力就可以保存下来,即使身体受到伤害,在把存储器取下来安装到一个新的身体上之后,原有的性格和能力都可以继续维持.....
另外,如果一
- Oracle Multitable INSERT 的用法
daizj
oracle
转载Oracle笔记-Multitable INSERT 的用法
http://blog.chinaunix.net/uid-8504518-id-3310531.html
一、Insert基础用法
语法:
Insert Into 表名 (字段1,字段2,字段3...)
Values (值1,
- 专访黑客历史学家George Dyson
datamachine
on
20世纪最具威力的两项发明——核弹和计算机出自同一时代、同一群年青人。可是,与大名鼎鼎的曼哈顿计划(第二次世界大战中美国原子弹研究计划)相 比,计算机的起源显得默默无闻。出身计算机世家的历史学家George Dyson在其新书《图灵大教堂》(Turing’s Cathedral)中讲述了阿兰·图灵、约翰·冯·诺依曼等一帮子天才小子创造计算机及预见计算机未来
- 小学6年级英语单词背诵第一课
dcj3sjt126com
englishword
always 总是
rice 水稻,米饭
before 在...之前
live 生活,居住
usual 通常的
early 早的
begin 开始
month 月份
year 年
last 最后的
east 东方的
high 高的
far 远的
window 窗户
world 世界
than 比...更
- 在线IT教育和在线IT高端教育
dcj3sjt126com
教育
codecademy
http://www.codecademy.com codeschool
https://www.codeschool.com teamtreehouse
http://teamtreehouse.com lynda
http://www.lynda.com/ Coursera
https://www.coursera.
- Struts2 xml校验框架所定义的校验文件
蕃薯耀
Struts2 xml校验Struts2 xml校验框架Struts2校验
>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>
蕃薯耀 2015年7月11日 15:54:59 星期六
http://fa
- mac下安装rar和unrar命令
hanqunfeng
mac
1.下载:http://www.rarlab.com/download.htm 选择
RAR 5.21 for Mac OS X 2.解压下载后的文件 tar -zxvf rarosx-5.2.1.tar 3.cd rar sudo install -c -o $USER unrar /bin #输入当前用户登录密码 sudo install -c -o $USER rar
- 三种将list转换为map的方法
jackyrong
list
在本文中,介绍三种将list转换为map的方法:
1) 传统方法
假设有某个类如下
class Movie {
private Integer rank;
private String description;
public Movie(Integer rank, String des
- 年轻程序员需要学习的5大经验
lampcy
工作PHP程序员
在过去的7年半时间里,我带过的软件实习生超过一打,也看到过数以百计的学生和毕业生的档案。我发现很多事情他们都需要学习。或许你会说,我说的不就是某种特定的技术、算法、数学,或者其他特定形式的知识吗?没错,这的确是需要学习的,但却并不是最重要的事情。他们需要学习的最重要的东西是“自我规范”。这些规范就是:尽可能地写出最简洁的代码;如果代码后期会因为改动而变得凌乱不堪就得重构;尽量删除没用的代码,并添加
- 评“女孩遭野蛮引产致终身不育 60万赔偿款1分未得”医腐深入骨髓
nannan408
先来看南方网的一则报道:
再正常不过的结婚、生子,对于29岁的郑畅来说,却是一个永远也无法实现的梦想。从2010年到2015年,从24岁到29岁,一张张新旧不一的诊断书记录了她病情的同时,也清晰地记下了她人生的悲哀。
粗暴手术让人发寒
2010年7月,在酒店做服务员的郑畅发现自己怀孕了,可男朋友却联系不上。在没有和家人商量的情况下,她决定堕胎。
12月5日,
- 使用jQuery为input输入框绑定回车键事件 VS 为a标签绑定click事件
Everyday都不同
jspinput回车键绑定clickenter
假设如题所示的事件为同一个,必须先把该js函数抽离出来,该函数定义了监听的处理:
function search() {
//监听函数略......
}
为input框绑定回车事件,当用户在文本框中输入搜索关键字时,按回车键,即可触发search():
//回车绑定
$(".search").keydown(fun
- EXT学习记录
tntxia
ext
1. 准备
(1) 官网:http://www.sencha.com/
里面有源代码和API文档下载。
EXT的域名已经从www.extjs.com改成了www.sencha.com ,但extjs这个域名会自动转到sencha上。
(2)帮助文档:
想要查看EXT的官方文档的话,可以去这里h
- mybatis3的mapper文件报Referenced file contains errors
xingguangsixian
mybatis
最近使用mybatis.3.1.0时无意中碰到一个问题:
The errors below were detected when validating the file "mybatis-3-mapper.dtd" via the file "account-mapper.xml". In most cases these errors can be d