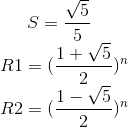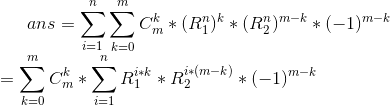- 【网络安全】网络安全中的离散数学
flyair_China
安全架构
一、离散数学核心知识点与网络安全映射1.数论(NumberTheory)知识点安全应用场景实例说明质因数分解RSA公钥加密大整数分解难题(2048位密钥需数万年破解)模运算Diffie-Hellman密钥交换利用(gamodp)实现安全协商欧拉定理RSA加密/解密me*d≡m(modn)保障解密还原中国剩余定理高效解密优化RSA-CRT加速解密运算达70%2.代数结构(AlgebraicStruc
- 数学中的代数数论与代数几何
AI天才研究院
计算AI大模型应用入门实战与进阶大数据人工智能语言模型AILLMJavaPython架构设计AgentRPA计算AI大模型应用
1.背景介绍在数学的众多分支中,代数数论和代数几何是两个极其重要的领域。代数数论,顾名思义,是研究数论问题的代数方法,主要研究整数、有理数、代数数等的性质。而代数几何则是研究零点集的代数方法,主要研究多项式方程和代数方程组的解的几何性质。这两个领域虽然看似独立,但实际上有着深厚的内在联系,它们的交叉研究已经产生了许多深远的理论和应用。2.核心概念与联系2.1代数数论代数数论的核心概念是代数数,即满
- 三生原理m 值的五周期循环是人为设定还是数论内在要求?
葫三生
三生学派算法人工智能机器学习量子计算数学建模
AI辅助创作:三AI辅助创作:生原理中m值的五周期循环(取值范围{0,1,2,3,4})本质上是数论内在要求,其必要性源于素数分布的周期性约束与代数结构的不可突破性,但部分特性受限于当前数学框架的观测维度。具体辩证关系如下:✅一、数论内在性的核心证据模周期对称性约束当m突破5周期(如m=5)时,三生原理的素数生成公式p=3(2n+1)+2(2n+m+1)必然生成合数:例如n=0,m=
- 【Algo】常见组合类数列
CodeWithMe
C/C++c++c语言算法
文章目录常见组合类数列1常见递推/组合类数列1.1基础递推类数列1.2组合数学数列1.3数论/函数类数列1.4图论/路径问题相关数列1.5算法和结构设计常用数列2示例:有规律数列前10项对比表3参考建议常见组合类数列介绍一些常见具有明显数学规律或递推关系的常见组合类数列。1常见递推/组合类数列1.1基础递推类数列Fibonacci数列F(n)=F(n-1)+F(n-2),F(0)=0,F(1)=1
- 数论:互质数的个数
Zephyrtoria
数据结构与算法java算法数论
数论:互质数的个数互质数的个数www.acwing.com/problem/content/4971/a=p1a1p2a2...pmama=p_{1}^{a_1}p_{2}^{a_2}...p_{m}^{a_m}a=p1a1p2a2...pmamab=p1a1bp2a2b...pmamba^{b}=p_{1}^{a_1b}p_{2}^{a_2b}...p_{m}^{a_mb}ab=p1a1bp2a
- 素数5在三生原理和费马数公式中均起临界作用的原因?
葫三生
三生学派机器学习人工智能算法量子计算数学建模
AI辅助创作:问答一:在数学理论中,素数5的“临界作用”在《三生原理》与费马数公式中均具有深刻的数学内涵,这种共性源于其独特的数论性质、结构对称性及计算阈值意义。以下从三个维度展开分析:一、5在《三生原理》中的临界性:阴阳平衡与生成韵律的转折点《三生原理》作为融合《周易》哲学的数论体系,其核心是将“三生万物”动态生成思想转化为素数分布的参数化模型。5的临界性体现在:最小满足阴阳参数联动的奇素数《三
- 算法-数论
cx_2023
算法c++开发语言
C-小红的数组查询(二)_牛客周赛Round95思路:不难看出a数组是有循环的d=3,p=4时,a数组:1、0、3、2、1、0、3、2.......最小循环节为4,即最多4种不同的数d=4,p=6时,a数组:1、5、3、1、5、3.......最小循环节为3d=4,p=10时,a数组:1、5、9、3、7、1、5、9、3、7.......最小循环节为5可以得出,最小循环节T=p/gcd(d,p)an
- 质数表的构建
羊儿~
c算法数据结构c++
前言最近,有很多人问我如何既能保证时间复杂度低又能正确的打出质数表,那么今天,我就给各位读者带来了几种打出质数表的(打表)的方法。1.质数的介绍质数,又称素数,是指在大于1的自然数中,除了1和它本身外,不能被其他自然数整除的数。换句话说,质数只有两个正因数:1和它自己。例如,2、3、5、7、11等都是质数。2是最小的质数,也是唯一的偶质数,其他质数都是奇数。质数在数学中具有重要地位,尤其在数论领域
- 使用MATLAB输出给定范围内的所有质数
士兵突击许三多
matlab基础matlab
使用MATLAB输出给定范围内的所有质数后续我将给出一些运用案例在计算机科学与数学中,质数是指仅能被1和其本身整除的自然数,例如2、3、5、7、11等。质数在数论和密码学中有着重要的应用。今天,我们将介绍如何使用MATLAB来生成并输出所有质数。什么是质数?质数是大于1的自然数,且只能被1和它自己整除。例如:2、3、5、7、11、13等都是质数。4、6、8、9、10等不是质数,它们都有其他因子。目
- 巧用数论与动态规划破解包子凑数问题
EtherWanderer
数据结构与算法蓝桥杯职场和发展
题目描述小明想知道包子铺用给定的蒸笼规格能凑出多少种无法组成的包子数目。若无法组成的数目无限,输出INF。输入格式第一行为整数NNN(蒸笼种数)接下来NNN行每行一个整数AiA_iAi(每种蒸笼的包子数)输出格式无法凑出的数目个数,若无限则输出INF问题分析关键条件若所有AiA_iAi的最大公约数(GCD)不为1,则无法组成的数目无限。例如,当所有数均为偶数时,无法组成任何奇数。动态规划思路当GC
- 解析数论基础:第二十四章 (s)与L(s,x)的阶估计
AI天才研究院
AI大模型企业级应用开发实战计算计算科学神经计算深度学习神经网络大数据人工智能大型语言模型AIAGILLMJavaPython架构设计AgentRPA
解析数论基础:第二十四章(s)与L(s,x)的阶估计作者:禅与计算机程序设计艺术/ZenandtheArtofComputerProgramming1.背景介绍1.1问题的由来数论是数学的一个分支,研究整数和它们的性质。在数论中,(s)函数和L(s,x)函数是两个重要的函数,它们在解析数论、数论分析以及许多数学物理领域都有着广泛的应用。特别是在素数分布、素数定理以及黎曼ζ函数的研究中,(s)函数和
- 探索 C++ 中的数论世界:从基础到实践
光の
java算法开发语言搜索算法
一、引言数论作为数学的核心分支,在计算机科学领域展现出强大的生命力。无论是密码学中的RSA加密算法,还是编程竞赛中的算法优化,数论都扮演着不可或缺的角色。C++凭借其高效的性能和底层控制能力,成为实现数论算法的理想选择。本文将带您走进C++数论的世界,从基础概念到实际应用,逐步揭开数论的神秘面纱。二、数论基础概念与C++实现2.1质数判定质数是大于1且只能被1和自身整除的整数。在C++中,我们可以
- USST新生训练赛3KLMN
Fighter_sky
题解C++acm
题解前言题解部分KPashmakandParmida'sproblem(1800)题目大意题解参考代码LPashmakandGraph(1900)题目大意题解参考代码MLuckyChains(1600)题目大意题解参考代码NManipulatingHistory(1600)题目大意题解参考代码前言KLMN是数据结构(线段树/树状数组)+dp+数论+结论唐题题解部分KPashmakandParmid
- 数论:数学王国的密码学
菜鸟破茧计划
密码学
在计算机科学的世界里,数论就像是一把神奇的钥匙,能够解开密码学、算法优化、随机数生成等诸多领域的谜题。作为C++算法小白,今天我就带大家一起走进数论的奇妙世界,探索其中的奥秘。什么是数论?数论是纯粹数学的分支之一,主要研究整数的性质。在计算机科学中,数论尤其在密码学、算法设计和计算机安全等领域有着广泛的应用。数论中的一些基本概念包括质数、最大公约数、模运算等。数论的基本概念与代码实现质数判定质数是
- 数论专题R1(线性筛专题)
JL24zyl
c++
目录A反素数加强版B约数积函数Ch(n)Dg(n)E神必的函数F球与盒子总结A反素数加强版时空限制1s,32MB问题描述如果一个大于等于1的正整数n,满足所有小于n且大于等于1的所有正整数的约数个数都小于n的约数个数,则n是一个反素数。请你计算不大于n的最大反素数。输入格式第一行输入数据组数T,每组数据输入1个正整数n。输出格式对每组数据,输出不大于n的最大反素数。数据范围1=1)的约数个数为(r
- 为什么哈希加密后破解怎么难?单向函数;密码学的数学原理:从理论到实践
小胡说技书
#数据安全技术哈希算法密码学算法单向函数数据安全安全信息安全
文章目录一、单向函数的数学基础1.1单向函数的数学定义1.2复杂度理论视角1.3数论在密码学中的应用二、哈希函数的数学原理与不可逆性2.1从信息论角度理解哈希不可逆性2.2碰撞抵抗的数学分析2.3单向压缩函数与雪崩效应三、非对称密码系统的数学基础3.1RSA算法的数学原理3.2椭圆曲线加密的几何解析四、密码学随机性与熵的数学原理4.1随机性与熵的量化4.2伪随机数生成器的数学模型4.3加盐哈希的数
- “即时取模”的快读 → 数论
hnjzsyjyj
信息学竞赛#算法数学基础#快读“即时取模”的快读快读
【“即时取模”的快读】●“即时取模”的快读是一种在输入大整数时直接进行取模运算的优化技术,常用于处理需要大数运算但最终结果需取模的场景(如数论题目)。其核心思想是在逐位读取数字时同步计算模值,避免存储完整的大数。intread(){//fastreadintx=0,f=1;charc=getchar();while(c'9'){//!isdigit(c)if(c=='-')f=-1;c=getch
- 【算法笔记】ACM数论基础模板
寂空_
算法笔记算法笔记c++
目录几个定理唯一分解定理鸽巢原理(抽屉原理)麦乐鸡定理哥德巴赫猜想容斥原理例题二进制枚举解dfs解裴蜀定理例题代码最大公约数、最小公倍数最大公约数最小公倍数质数试除法判断质数分解质因数筛质数朴素筛法(埃氏筛法)线性筛法(欧拉筛法)约数试除法求约数求约数个数一个数求约数个数求1~n所有数的约数个数O(nlogn)O(nlogn)O(nlogn)筛法O(n)O(n)O(n)筛法约数之和一个数求约数之和
- 扩展欧几里得算法简介及代码实现
hnjzsyjyj
信息学竞赛#算法数学基础扩展欧几里得算法裴蜀定理
【扩展欧几里得算法简介】●扩展欧几里得算法(ExtendedEuclideanAlgorithm)是欧几里得算法的扩展版本,不仅能计算两个整数的最大公约数(GCD),还能找到满足贝祖等式(Bézout'sIdentity)ax+by=gcd(a,b)的整数解x和y。它在数论、密码学等领域有重要应用,例如求解模的逆元、求解线性同余方程等。●扩展欧几里得算法求ax+by=gcd(a,b)特解的方法如下
- 《夜深人静写算法》数论篇 - (10) 扩展欧几里得定理
英雄哪里出来
《夜深人静写算法》数论篇算法初等数论扩展欧几里得定理
前言 通过扩展欧几里得定理,利用扩展欧几里得算法,可以求解线性同余方程。 那么什么是线性同余方程?什么是扩展欧几里得定理?什么是扩展欧几里得算法?接下来的几篇文章会来讲解一下这几个概念。一、扩展欧几里得定理1、定理概述 对于不都为零的整数aaa和b
- 【ICPC】The 2024 ICPC Kunming Invitational Contest E
浅慕Antonio
算法竞赛开发语言c++算法
RelearnthroughReview#数论#枚举#gcd题目描述Givenanintegersequencea1,a2,⋯ ,ana_1,a_2,\cdots,a_na1,a2,⋯,anoflengthnnnandanon-negativeintegerkkk,youcanperformthefollowingoperationatmostonce:Choosetwointegerslllan
- 初等数论 --- 同余、欧拉定理、费马小定理、求逆元
chstor
算法笔记
文章目录一、同余二、欧拉定理三、费马小定理四、扩展欧几里得算法4.1裴蜀定理五、一元线性同余方程六、逆元求逆元方法一、扩展欧几里得算法求逆元方法二、费马小定理加快速幂一、同余定义当两个整数a,b除以同一个正整数m,若得相同余数,则二整数同余。记为:a≡b(mod m)当两个整数a,b除以同一个正整数m,若得相同余数,则二整数同余。记为:a\equivb(\modm)当两个整数a,b除以同一个正整
- 初等数论 课堂笔记 第三章 -- 欧拉函数一节的若干练习
此账号已停更
初等数论数学数论
练习计算φ(60)\varphi\left(60\right)φ(60)。解 将606060写成标准分解式60=22×3×560={{2}^{2}}\times3\times560=22×3×5法一(计算过程中出现分式)φ(60)=60×(1−12)(1−13)(1−15)=60×12×23×45=16\varphi\left(60\right)=60\times\left(1-\frac{1}
- 【关于数学】感悟(附学习目录)
DataPlayerK
线性代数抽象代数概率论矩阵
一些感悟数学具有艺术美。从某种意义上来说,数学家和画家本质相同,他们都在“刻画”心目中的图景。小时候我总是在思考一个终极问题:数学是什么?我怀念那时我单纯而热烈的执着,此文章就长期记载我对数学的看法吧。2017-2020高中在读数学是不同精巧结构的集合。高中数学竞赛中,不等式/组合数学/数论中充斥着各种“限制下的精巧结构”,使得结构出现了各种各样奇妙的性质。2021-4-14大一在读数学不仅重在结
- NOIP2009提高组.Hankson的趣味题
Ayanami_Reii
算法c++笔记蓝桥杯
目录题目算法标签:数论,最大公约数,最小公倍数,约数思路代码题目200.Hankson的趣味题算法标签:数论,最大公约数,最小公倍数,约数思路因为[x,a0]=b1[x,a_0]=b_1[x,a0]=b1因此xxx一定是b1b_1b1约数,注意到,数据范围是2×1092\times10^92×109如果直接使用试除法计算约数时间复杂度是O(nn)O(n\sqrtn)O(nn)会超时,因此需要进行优
- 数论---求组合数
@松田
算法c++组合数数论
快速幂:数论-----快速幂-CSDN博客快速幂求逆元:数论----快速幂求逆元-CSDN博客筛质数:筛质数----CSDN博客求组合数I//10万组a,busingnamespacestd;constintN=2010,mod=1e9+7;intc[N][N];voidinit(){for(inti=0;i>n;while(n--){inta,b;cin>>a>>b;coutusingnames
- 线性筛法求素数(欧拉筛法)(求质数,O(n)时间复杂度)(外加求每个整数的最小质因子)(python)
不染_是非
算法pythonpython算法开发语言
前言:python中求质数的方法有好几种,这里就讲解时间复杂度最低的算法欧拉筛法,时间复杂度为O(n),这是数论中也是算法比赛中必须掌握的方法。本篇博客还会额外讲解求每个整数的最小质因子,什么是质因子?顾名思义,就是是质数的因子,求这个有什么用呢?下篇博客X的因子链(数论,python)(算术基本定理)(欧拉筛法)会给大家讲解一道例题,在例题中讲解它的用法。思路:线性筛法的整体思路是(代码里有详细
- 解析数论基础:问题的提出和进展
AI天才研究院
AI大模型企业级应用开发实战DeepSeekR1&大数据AI人工智能大模型计算计算科学神经计算深度学习神经网络大数据人工智能大型语言模型AIAGILLMJavaPython架构设计AgentRPA
解析数论基础:问题的提出和进展作者:禅与计算机程序设计艺术/ZenandtheArtofComputerProgramming1.背景介绍1.1问题的由来数论,作为数学的一个分支,自古以来就与算法和密码学紧密相连。从古代的算术运算到现代的计算机科学,数论问题始终是算法设计和理论分析的重要基础。随着计算机技术的发展,数论在加密算法、网络安全、计算机图形学、算法优化等领域发挥着越来越重要的作用。1.2
- 了解倒数的概念,乘法逆元就很好理解——解析之【逆元的概念】【逆元的求解方法】
灰阳阳
算法算法裴蜀定理欧几里得算法最大公约数逆元
目录前言一、逆元的概念1、基本定义示例1:a=3,m=7a=3,m=7a=3,m=7示例2:a=2,m=5a=2,m=5a=2,m=52、乘法逆元有什么用3、相关性质二、求解逆元的方法1、费马小定理求乘法逆元定义费马小定理求逆元的方法总结模板题2、扩展欧几里得算法求逆元定义扩展欧几里得算法求逆元的方法总结模板题3、递推公式求逆元定义递推公式的推导示例总结前言首先,下面讨论的是数论相关内容。主要研究
- 【算法】数论基础——逆元的概念与应用 python
查理零世
算法python
文章目录前言一、什么是逆元?二、逆元的存在条件三、如何计算逆元?1.扩展欧几里得算法(ExtendedEuclideanAlgorithm)2.使用费马小定理(Fermat'sLittleTheorem)四、应用场景示例:求排列数和组合数前言逆元(ModularMultiplicativeInverse)在模运算中是一个非常重要的概念,特别是在需要执行除法操作时。因为在模p的情况下,直接进行除法是
- jdk tomcat 环境变量配置
Array_06
javajdktomcat
Win7 下如何配置java环境变量
1。准备jdk包,win7系统,tomcat安装包(均上网下载即可)
2。进行对jdk的安装,尽量为默认路径(但要记住啊!!以防以后配置用。。。)
3。分别配置高级环境变量。
电脑-->右击属性-->高级环境变量-->环境变量。
分别配置 :
path
&nbs
- Spring调SDK包报java.lang.NoSuchFieldError错误
bijian1013
javaspring
在工作中调另一个系统的SDK包,出现如下java.lang.NoSuchFieldError错误。
org.springframework.web.util.NestedServletException: Handler processing failed; nested exception is java.l
- LeetCode[位运算] - #136 数组中的单一数
Cwind
java题解位运算LeetCodeAlgorithm
原题链接:#136 Single Number
要求:
给定一个整型数组,其中除了一个元素之外,每个元素都出现两次。找出这个元素
注意:算法的时间复杂度应为O(n),最好不使用额外的内存空间
难度:中等
分析:
题目限定了线性的时间复杂度,同时不使用额外的空间,即要求只遍历数组一遍得出结果。由于异或运算 n XOR n = 0, n XOR 0 = n,故将数组中的每个元素进
- qq登陆界面开发
15700786134
qq
今天我们来开发一个qq登陆界面,首先写一个界面程序,一个界面首先是一个Frame对象,即是一个窗体。然后在这个窗体上放置其他组件。代码如下:
public class First { public void initul(){ jf=ne
- Linux的程序包管理器RPM
被触发
linux
在早期我们使用源代码的方式来安装软件时,都需要先把源程序代码编译成可执行的二进制安装程序,然后进行安装。这就意味着每次安装软件都需要经过预处理-->编译-->汇编-->链接-->生成安装文件--> 安装,这个复杂而艰辛的过程。为简化安装步骤,便于广大用户的安装部署程序,程序提供商就在特定的系统上面编译好相关程序的安装文件并进行打包,提供给大家下载,我们只需要根据自己的
- socket通信遇到EOFException
肆无忌惮_
EOFException
java.io.EOFException
at java.io.ObjectInputStream$PeekInputStream.readFully(ObjectInputStream.java:2281)
at java.io.ObjectInputStream$BlockDataInputStream.readShort(ObjectInputStream.java:
- 基于spring的web项目定时操作
知了ing
javaWeb
废话不多说,直接上代码,很简单 配置一下项目启动就行
1,web.xml
<?xml version="1.0" encoding="UTF-8"?>
<web-app xmlns:xsi="http://www.w3.org/2001/XMLSchema-instance"
xmlns="h
- 树形结构的数据库表Schema设计
矮蛋蛋
schema
原文地址:
http://blog.csdn.net/MONKEY_D_MENG/article/details/6647488
程序设计过程中,我们常常用树形结构来表征某些数据的关联关系,如企业上下级部门、栏目结构、商品分类等等,通常而言,这些树状结构需要借助于数据库完成持久化。然而目前的各种基于关系的数据库,都是以二维表的形式记录存储数据信息,
- maven将jar包和源码一起打包到本地仓库
alleni123
maven
http://stackoverflow.com/questions/4031987/how-to-upload-sources-to-local-maven-repository
<project>
...
<build>
<plugins>
<plugin>
<groupI
- java IO操作 与 File 获取文件或文件夹的大小,可读,等属性!!!
百合不是茶
类 File
File是指文件和目录路径名的抽象表示形式。
1,何为文件:
标准文件(txt doc mp3...)
目录文件(文件夹)
虚拟内存文件
2,File类中有可以创建文件的 createNewFile()方法,在创建新文件的时候需要try{} catch(){}因为可能会抛出异常;也有可以判断文件是否是一个标准文件的方法isFile();这些防抖都
- Spring注入有继承关系的类(2)
bijian1013
javaspring
被注入类的父类有相应的属性,Spring可以直接注入相应的属性,如下所例:1.AClass类
package com.bijian.spring.test4;
public class AClass {
private String a;
private String b;
public String getA() {
retu
- 30岁转型期你能否成为成功人士
bijian1013
成长励志
很多人由于年轻时走了弯路,到了30岁一事无成,这样的例子大有人在。但同样也有一些人,整个职业生涯都发展得很优秀,到了30岁已经成为职场的精英阶层。由于做猎头的原因,我们接触很多30岁左右的经理人,发现他们在职业发展道路上往往有很多致命的问题。在30岁之前,他们的职业生涯表现很优秀,但从30岁到40岁这一段,很多人
- 【Velocity四】Velocity与Java互操作
bit1129
velocity
Velocity出现的目的用于简化基于MVC的web应用开发,用于替代JSP标签技术,那么Velocity如何访问Java代码.本篇继续以Velocity三http://bit1129.iteye.com/blog/2106142中的例子为基础,
POJO
package com.tom.servlets;
public
- 【Hive十一】Hive数据倾斜优化
bit1129
hive
什么是Hive数据倾斜问题
操作:join,group by,count distinct
现象:任务进度长时间维持在99%(或100%),查看任务监控页面,发现只有少量(1个或几个)reduce子任务未完成;查看未完成的子任务,可以看到本地读写数据量积累非常大,通常超过10GB可以认定为发生数据倾斜。
原因:key分布不均匀
倾斜度衡量:平均记录数超过50w且
- 在nginx中集成lua脚本:添加自定义Http头,封IP等
ronin47
nginx lua csrf
Lua是一个可以嵌入到Nginx配置文件中的动态脚本语言,从而可以在Nginx请求处理的任何阶段执行各种Lua代码。刚开始我们只是用Lua 把请求路由到后端服务器,但是它对我们架构的作用超出了我们的预期。下面就讲讲我们所做的工作。 强制搜索引擎只索引mixlr.com
Google把子域名当作完全独立的网站,我们不希望爬虫抓取子域名的页面,降低我们的Page rank。
location /{
- java-3.求子数组的最大和
bylijinnan
java
package beautyOfCoding;
public class MaxSubArraySum {
/**
* 3.求子数组的最大和
题目描述:
输入一个整形数组,数组里有正数也有负数。
数组中连续的一个或多个整数组成一个子数组,每个子数组都有一个和。
求所有子数组的和的最大值。要求时间复杂度为O(n)。
例如输入的数组为1, -2, 3, 10, -4,
- Netty源码学习-FileRegion
bylijinnan
javanetty
今天看org.jboss.netty.example.http.file.HttpStaticFileServerHandler.java
可以直接往channel里面写入一个FileRegion对象,而不需要相应的encoder:
//pipeline(没有诸如“FileRegionEncoder”的handler):
public ChannelPipeline ge
- 使用ZeroClipboard解决跨浏览器复制到剪贴板的问题
cngolon
跨浏览器复制到粘贴板Zero Clipboard
Zero Clipboard的实现原理
Zero Clipboard 利用透明的Flash让其漂浮在复制按钮之上,这样其实点击的不是按钮而是 Flash ,这样将需要的内容传入Flash,再通过Flash的复制功能把传入的内容复制到剪贴板。
Zero Clipboard的安装方法
首先需要下载 Zero Clipboard的压缩包,解压后把文件夹中两个文件:ZeroClipboard.js
- 单例模式
cuishikuan
单例模式
第一种(懒汉,线程不安全):
public class Singleton { 2 private static Singleton instance; 3 pri
- spring+websocket的使用
dalan_123
一、spring配置文件
<?xml version="1.0" encoding="UTF-8"?><beans xmlns="http://www.springframework.org/schema/beans" xmlns:xsi="http://www.w3.or
- 细节问题:ZEROFILL的用法范围。
dcj3sjt126com
mysql
1、zerofill把月份中的一位数字比如1,2,3等加前导0
mysql> CREATE TABLE t1 (year YEAR(4), month INT(2) UNSIGNED ZEROFILL, -> day
- Android开发10——Activity的跳转与传值
dcj3sjt126com
Android开发
Activity跳转与传值,主要是通过Intent类,Intent的作用是激活组件和附带数据。
一、Activity跳转
方法一Intent intent = new Intent(A.this, B.class); startActivity(intent)
方法二Intent intent = new Intent();intent.setCla
- jdbc 得到表结构、主键
eksliang
jdbc 得到表结构、主键
转自博客:http://blog.csdn.net/ocean1010/article/details/7266042
假设有个con DatabaseMetaData dbmd = con.getMetaData(); rs = dbmd.getColumns(con.getCatalog(), schema, tableName, null); rs.getSt
- Android 应用程序开关GPS
gqdy365
android
要在应用程序中操作GPS开关需要权限:
<uses-permission android:name="android.permission.WRITE_SECURE_SETTINGS" />
但在配置文件中添加此权限之后会报错,无法再eclipse里面正常编译,怎么办?
1、方法一:将项目放到Android源码中编译;
2、方法二:网上有人说cl
- Windows上调试MapReduce
zhiquanliu
mapreduce
1.下载hadoop2x-eclipse-plugin https://github.com/winghc/hadoop2x-eclipse-plugin.git 把 hadoop2.6.0-eclipse-plugin.jar 放到eclipse plugin 目录中。 2.下载 hadoop2.6_x64_.zip http://dl.iteye.com/topics/download/d2b
- 如何看待一些知名博客推广软文的行为?
justjavac
博客
本文来自我在知乎上的一个回答:http://www.zhihu.com/question/23431810/answer/24588621
互联网上的两种典型心态:
当初求种像条狗,如今撸完嫌人丑
当初搜贴像条犬,如今读完嫌人软
你为啥感觉不舒服呢?
难道非得要作者把自己的劳动成果免费给你用,你才舒服?
就如同 Google 关闭了 Gooled Reader,那是
- sql优化总结
macroli
sql
为了是自己对sql优化有更好的原则性,在这里做一下总结,个人原则如有不对请多多指教。谢谢!
要知道一个简单的sql语句执行效率,就要有查看方式,一遍更好的进行优化。
一、简单的统计语句执行时间
declare @d datetime ---定义一个datetime的变量set @d=getdate() ---获取查询语句开始前的时间select user_id
- Linux Oracle中常遇到的一些问题及命令总结
超声波
oraclelinux
1.linux更改主机名
(1)#hostname oracledb 临时修改主机名
(2) vi /etc/sysconfig/network 修改hostname
(3) vi /etc/hosts 修改IP对应的主机名
2.linux重启oracle实例及监听的各种方法
(注意操作的顺序应该是先监听,后数据库实例)
&nbs
- hive函数大全及使用示例
superlxw1234
hadoophive函数
具体说明及示例参 见附件文档。
文档目录:
目录
一、关系运算: 4
1. 等值比较: = 4
2. 不等值比较: <> 4
3. 小于比较: < 4
4. 小于等于比较: <= 4
5. 大于比较: > 5
6. 大于等于比较: >= 5
7. 空值判断: IS NULL 5
- Spring 4.2新特性-使用@Order调整配置类加载顺序
wiselyman
spring 4
4.1 @Order
Spring 4.2 利用@Order控制配置类的加载顺序
4.2 演示
两个演示bean
package com.wisely.spring4_2.order;
public class Demo1Service {
}
package com.wisely.spring4_2.order;
public class
![]()
![]()
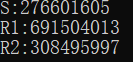
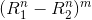 二项式展开,可得到总和:
二项式展开,可得到总和: