ZOJ 1007 Numerical Summation of a Series

Produce a table of the values of the series

Equation 1

for the 2001 values of x, x= 0.000, 0.001, 0.002, ..., 2.000. All entries of the table must have an absolute error less than 0.5e-12 (12 digits of precision). This problem is based on a problem from Hamming (1962), when mainframes were very slow by today's microcomputer standards.

Input

This problem has no input.

Output

The output is to be formatted as two columns with the values of x and y(x) printed as in the C printf or the Pascal writeln.

printf("%5.3f %16.12f\n", x, psix )		writeln(x:5:3, psix:16:12)

As an example, here are 4 acceptable lines out of 2001.

0.000   1.644934066848
...
0.500   1.227411277760
...
1.000   1.000000000000
...
2.000   0.750000000000

The values of x should start at 0.000 and increase by 0.001 until the line with x=2.000 is output.

Hint

The problem with summing the sequence in equation 1 is that too many terms may be required to complete the summation in the given time. Additionally, if enough terms were to be summed, roundoff would render any typical double precision computation useless for the desired precision.

To improve the convergence of the summation process note that

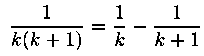
Equation 2

which implies y(1)=1.0. One can then produce a series for y(x) - y(1) which converges faster than the original series. This series not only converges much faster, it also reduces roundoff loss.

This process of finding a faster converging series may be repeated to produce sequences which converge more and more rapidly than the previous ones.

The following inequality is helpful in determining how may items are required in summing the series above.

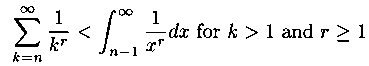
其实是一道数学题，给了你方法让你思考如何去求近似值。 求f(x)的近似值，先用f(x)-f(1)把每一项都写出来，提出分母中的1/k,剩下的合成一个分式(x-1)/(k+1)(k+x) 再利用第二个提示当k趋于无穷时近似值可以用积分来求。

#include <stdio.h>

int main(){
	double x = 0.000 , sum, k;
	for (int i = 1; i <= 2001;i++)
	{
		sum = 0.000;
		for (k = 1; k < 10000; k++) sum += 1.0 / (k * (k + 1) * (k + x));
		sum = (1.0 - x)*(sum + 1.0 / (20000 * 10000)) + 1.0;
		printf("%5.3f %16.12f\n", x, sum);
		x += 0.001;
	} 
	return 0;
}