第一章动态规划（十）

区间DP

例题：1068.环形石子合并

类比 282.合并石子

import java.util.Scanner;

public class Main {
	static int INF = Integer.MAX_VALUE >> 1;
	static int N = 410;//开两倍
    static int n;
    static int[] s = new int[N];
    static int[] w = new int[N];
    static int[][] f = new int[N][N];
    static int[][] g = new int[N][N];
    
    public static void main(String[] args) {
        Scanner sc = new Scanner(System.in);
        n = sc.nextInt();
        for (int i = 1; i <= n; i++) {
			w[i] = sc.nextInt();
			w[i + n] = w[i];
		}
        sc.close();
        //前缀和
        for (int i = 1; i <= n * 2; i++) {
			s[i] = s[i - 1] + w[i];
		}
        //初始化
        for (int i = 0;i < f.length;i++) {
			for (int j = 0; j < f[i].length; j++) {
				f[i][j] = INF;
			}
		}
        for (int i = 0;i < g.length;i++) {
			for (int j = 0; j < g[i].length; j++) {
				g[i][j] = -INF;
			}
		}
        for (int len = 1; len <= n; len++) {
			for (int l = 1; l + len - 1 <= n * 2; l++) {
				int r = l + len - 1;
				if (len == 1) {
					f[l][r] = 0;
					g[l][r] = 0;
				}else {
					for (int k = l; k < r; k++) {
						f[l][r] = Math.min(f[l][r], f[l][k] + f[k + 1][r] + s[r] - s[l - 1]);
						g[l][r] = Math.max(g[l][r], g[l][k] + g[k + 1][r] + s[r] - s[l - 1]);
					}
				}
			}
		}
        int minV = INF;
        int maxV = -INF;
        for (int i = 1; i <= n; i++) {
			minV = Math.min(minV, f[i][i + n - 1]);
			maxV = Math.max(maxV, g[i][i + n - 1]);
		}
        System.out.println(minV);
        System.out.println(maxV);
    }
}

例题：320. 能量项链

import java.util.Scanner;

public class Main {
    static int N = 210;
    static int n;
    static int[] w = new int[N];
    static int[] s = new int[N];
    static int[][] f = new int[N][N];
    static int INF = 0x3f3f3f3f;
    
    public static void main(String[] args)  {
        Scanner sc = new Scanner(System.in);
        n = sc.nextInt();
        for (int i = 1;i <= n;i++) {
            int x = sc.nextInt();
            w[i] = x;
            w[i + n] = x;   
        }
        sc.close();
        
        for (int len = 3;len <= n + 1;len ++) {
            for (int L = 1;L + len - 1 <= n * 2;L ++) {
                int R = L + len - 1;
                f[L][R] = -INF;
                for (int k = L + 1;k < R;k ++) {
                    f[L][R] = Math.max(f[L][R], f[L][k] + f[k][R] + w[L] * w[k] * w[R]); 
                }
            }
        }
        int res = 0;
        for (int i = 1;i <= n;i++) {
            res = Math.max(res, f[i][i + n]);
        }
        System.out.println(res);
    }
}

例题：凸多边形的划分
原题链接
【题目描述】
给定一个具有 N个顶点的凸多边形，将顶点从 1 至 N 标号，每个顶点的权值都是一个正整数。将这个凸多边形划分成 N−2个互不相交的三角形，试求这些三角形顶点的权值乘积和至少为多少。
【输入】
输入第一行为顶点数 N
第二行依次为顶点 1至顶点 N的权值。
【输出】
输出仅一行，为这些三角形顶点的权值乘积和的最小值。
【输入样例】
5
121 122 123 245 231
【输出样例】
12214884
【提示】
数据范围与提示：
对于 100% 的数据，有 N≤50，每个点权值小于 10⁹ 。
————————————————————————————————————————————————————
需要高精度

代码有错

import java.util.Arrays;
import java.util.Scanner;

public class Main {
    static int N = 55;
    static int M = 35;
    static int INF = (int) 1e9;
    static int n;
    static int[] w = new int[N];
    static long[][][] f = new long[N][N][M];
    static long[] c = new long[M];
    
    public static void main(String[] args)  {
        Scanner sc = new Scanner(System.in);
        n = sc.nextInt();
        for (int i = 1; i <= n; i++) {
			w[i] = sc.nextInt();
		}
        sc.close();
        
        long[] tmp = new long[M];
        for (int len = 3; len <= n; len++) {
			for (int l = 1; l + len - 1 <= n; l++) {
				int r = l + len - 1;
				f[l][r][M - 1] = 1;
				for (int k = l + 1; k < r; k++) {
					Arrays.fill(tmp, 0);
					tmp[0] = w[l];
					mul(tmp, w[k]);
					mul(tmp, w[r]);
					add(tmp, f[l][k]);
					add(tmp, f[k][r]);
					if (cmp(f[l][r], tmp) > 0) System.arraycopy(f[l][r], 0, tmp, 0, tmp.length);
				}
			}
		}
        print(f[1][n]);
    }
    
    private static void add(long[] a, long[] b) {
    	Arrays.fill(c, 0);
    	for (int i = 0, t = 0; i < M; i++) {
			t += a[i] + b[i];
			c[i] = t % 10;
			t /= 10;
		}
    	System.arraycopy(a, 0, c, 0, c.length);
    }
    
    private static void mul(long[] a, long b) {
    	Arrays.fill(c, 0);
    	long t = 0;
    	for (int i = 0; i < M; i++) {
			t += a[i] * b;
			c[i] = t % 10;
			t /= 10;
		}
    	System.arraycopy(a, 0, c, 0, c.length);
    }
    
    private static int cmp(long[] a, long[] b) {
    	for (int i = M - 1; i >= 0; i--) {
			if (a[i] > b[i]) {
				return 1;
			}else if (a[i] < b[i]){
				return -1;
			}
		}
    	return 0;
    }
    
    private static void print(long[] a) {
    	int k = M - 1;
    	while(k > 0 && a[k] == 0) k--;
    	while(k >= 0) System.out.print(a[k--]);
    	System.out.println();
    }
}

例题：479. 加分二叉树

import java.util.Scanner;

public class Main {
    static int N = 50;
    static int n;
    static int[] w = new int[N];
    static int[][] f = new int[N][N];
    static int[][] root = new int[N][N];
    
    public static void main(String[] args)  {
        Scanner sc = new Scanner(System.in);
        n = sc.nextInt();
        for (int i = 1; i <= n; i++) {
			w[i] = sc.nextInt();
		}
        sc.close();
        
        for (int len = 1; len <= n; len ++ ) {
            for (int l = 1; l + len - 1 <= n; l ++ ) {
                int r = l + len - 1;
                for (int k = l; k <= r; k ++ ) {
                    int left = k == l ? 1 : f[l][k - 1];
                    int right = k == r ? 1 : f[k + 1][r];
                    int score = left * right + w[k];
                    if (l == r) score = w[k];
                    if (f[l][r] < score) {
                        f[l][r] = score;
                        root[l][r] = k;
                    }
                }
            }
		}
        System.out.printf("%d\n", f[1][n]);
        dfs(1, n);
        System.out.println();
    }
    
    private static void dfs(int l, int r) {
        if (l > r) return;
        int k = root[l][r];
        System.out.printf("%d ", k);
        dfs(l, k - 1);
        dfs(k + 1, r);
    }
}

例题：321. 棋盘分割

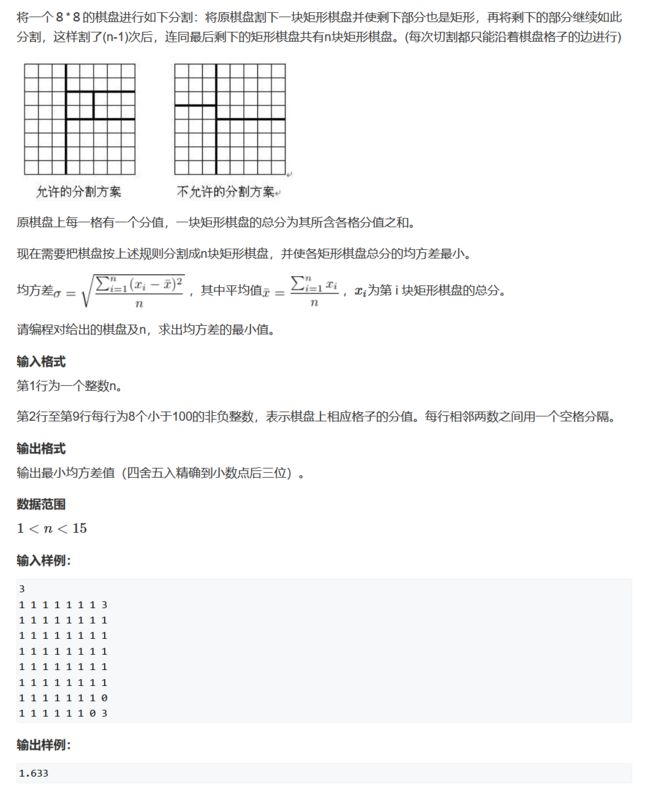
f[x1][y1][x2][y2][k] : 将矩阵(x1,y1)->(x2,y2)分割成k个矩阵的最小方差
记忆化搜索：

import java.util.*;

class Main{
	static int n, m = 8;
	static double X, INF = 1e9;
	static int[][] s;
	static double[][][][][] f;

	public static void main(String[] args){
		Scanner sc = new Scanner(System.in);
		n = sc.nextInt();
		s = new int[m+1][m+1];
		for(int i = 1; i <= m; i++){
			for(int j = 1; j <= m; j++){
				s[i][j] = sc.nextInt();
				s[i][j] += s[i - 1][j] + s[i][j - 1] - s[i - 1][j - 1];
			}
		}
		sc.close();
		
		X = (double) s[m][m] / n;
		f = new double[m + 1][m + 1][m + 1][m + 1][n + 1];
		for(int i1 = 0; i1 <= m; i1++){
			for(int j1 = 0; j1 <= m; j1++){
				for(int i2 = 0; i2 <= m; i2++){
					for(int j2 = 0; j2 <= m; j2++){
						for(int k = 0; k <= n; k++){
							f[i1][j1][i2][j2][k] = -INF;
						}
					}
				}
			}
		}
		System.out.println(String.format("%.3f", Math.sqrt(dp(1, 1, m, m, n))));
	}
	private static double dp(int x1, int y1, int x2, int y2, int k){
		double t = f[x1][y1][x2][y2][k];
		if(t >= 0) return t;
		if(k == 1){
			t = get(x1, y1, x2, y2);
			f[x1][y1][x2][y2][k] = t;
			return t;
		}
		t = INF;
		for(int i = x1; i < x2; i++){
			t = Math.min(t, dp(x1, y1, i, y2, k - 1) + get(i+1, y1, x2, y2));
			t = Math.min(t, dp(i+1, y1, x2, y2, k-1) + get(x1, y1, i, y2));
		}
		for(int j = y1; j < y2; j++){
			t = Math.min(t, dp(x1, y1, x2, j, k-1) + get(x1, j+1, x2, y2));
			t = Math.min(t, dp(x1, j+1, x2, y2, k-1) + get(x1, y1, x2, j));
		}
		f[x1][y1][x2][y2][k] = t;
		return t;
	}
	private static double get(int x1, int y1, int x2, int y2){
		double sum = s[x2][y2] - s[x1 - 1][y2] - s[x2][y1 - 1] + s[x1 - 1][y1 - 1] - X;
		return (double) (sum * sum) / n;
	}
}