- 备战蓝桥杯---数学之矩阵快速幂基础
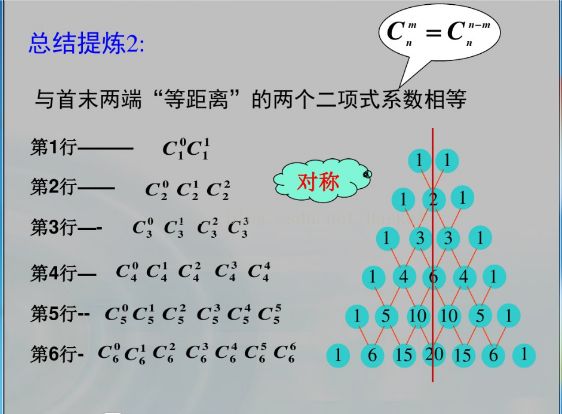
CoCoa-Ck
蓝桥杯矩阵算法c++
我们先不妨看一道题:看见n的数据范围就知道直接按以前的递归写肯定狗带,那我们有什么其他的方法吗?下面是分析:我们就拿斐波那契数列试试手吧:下面是AC代码,可以当作模板记:#includeusingnamespacestd;#defineintlonglongintm,n,mod=1e9+7;structnode{intm[100][100];}ans,res;nodemul(nodea,nodeb
- LeetCode:70.爬楼梯
nainaire
大一写的LeetCode题leetcode算法c语言数据结构
前言:好家伙,一直以为动态规划是啥高大上的,解释那么多,在我看来不过是找规律罢了,写那么多"专业术语"咋看咋像糊弄人的(手动扶额)另外,通项公式虽然抽象还能接受,但是矩阵快速幂是什么鬼?70.爬楼梯-力扣(LeetCode)目录题目:思路,分析:代码+注释:每日表情包:题目:思路,分析:一眼斐波那契数列,但有时间限制,搞不了递归,那就搞循环,(从前往后的加,不搞递归的大量且重复的计算)官方题解叫这
- 【小赛1】蓝桥杯双周赛第5场(小白)思路回顾
清风莫追
愚公搬算法蓝桥杯职场和发展python算法
我的成绩:小白(5/6)完稿时间:2024-2-13比赛地址:https://www.lanqiao.cn/oj-contest/newbie-5/相关资料:1、出题人题解:“蓝桥杯双周赛·第5次强者挑战赛/小白入门赛”出题人题解-知乎(zhihu.com)2、矩阵快速幂:算法学习笔记(4):快速幂-知乎(zhihu.com)讲得挺好的,从快速幂到矩阵快速幂,以及在求解递推式中的应用。3、矩阵乘法
- 【数论】矩阵快速幂
Texcavator
数论矩阵算法数据结构
参考:P3193[HNOI2008]GT考试题解放个板子structMartix{inta[30][30];//在这里修改矩阵的大小Martix(){memset(a,0,sizeof(a));}Martixoperator*(constMartix&B)const//乘法运算符重载{Martixres;for(inti=0;i>=1;a=a*a;}returnans;}
- 2024.2.7-8 寒假训练记录(21)
Texcavator
2024寒假训练记录算法
文章目录洛谷P3193[HNOI2008]GT考试ATCabc339ESmoothSubsequenceATCabc339FProductEquality洛谷P3193[HNOI2008]GT考试题目链接KMP+dp+矩阵快速幂还没有理解得很清楚,主要是对KMP理解还不够深刻#includeusingnamespacestd;#defineintlonglongusingi64=longlong;
- LC1220线性代数YYDS:多种解法:「状态机DP:一维 OR 二维」&「矩阵快速幂」
Chthollists
前言大家好,我是新人博主:「个人主页」主要分享程序员生活、编程技术、以及每日的LeetCode刷题记录,欢迎大家关注我,一起学习交流,谢谢!正在坚持每日更新LeetCode每日一题,发布的题解有些会参考其他大佬的思路(参考资料的链接会放在最下面),欢迎大家关注我~~~同时也在进行其他专项类型题目的刷题与题解活动,相关资料也会同步到「GitHub」上面~今天是坚持写题解的21天(haha,从21年圣
- 用动态规划、矩阵快速幂求解斐波那契数列
北辰2023
数据结构与算法设计动态规划矩阵算法
斐波那契数列(Fibonaccisequence),又称黄金分割数列,因数学家莱昂纳多·斐波那契(LeonardoFibonacci)以兔子繁殖为例子而引入,故又称“兔子数列”,其数值为:1、1、2、3、5、8、13、21、34……在数学上,这一数列以如下递推的方法定义:F(0)=1,F(1)=1,F(n)=F(n-1)+F(n-2)(n≥2,n∈N*)。本文将介绍Fibonacci数列的动态规划
- 矩阵快速幂 笔记
Daniel_1011
矩阵笔记算法
矩阵的运算矩阵(Matrix)是一个按照长方阵列排列的集合加法矩阵的加法必须保证都是同型矩阵即加减运算行列数都必须一样矩阵的加法运算满足结合律和交换律:A+B=B+AA+B=B+AA+B=B+AA+(B+C)=(A+B)+CA+(B+C)=(A+B)+CA+(B+C)=(A+B)+C减法同理数乘把矩阵A的行和列互相交换所产生的矩阵称为A的转置矩阵即所有元素都乘一遍一个数矩阵的加减法和矩阵的数乘合称
- 算法-递归迭代-青蛙跳台阶-阶乘-裴波那契数列-汉诺塔问题-全排列-
LXMXHJ
算法思路算法
文章目录==迭代和递归==递归案例:不死神兔案例:递归求阶乘案例:遍历目录递归与迭代区别递归、迭代与普通循环的区别==案例1阶乘==递归迭代==案例1-1青蛙跳台阶问题==分析递归迭代动态规划==案例2裴波那契数列==无技巧递归会超时递推实现动态规划递归实现动态规划矩阵快速幂打表==汉诺塔问题==递归(结束n==1)递归(结束n==0)==全排列==迭代回溯(不使用标记数组)回溯(使用标记数组)迭
- 蓝桥杯2015年第六届真题-垒骰子
不牌不改
#【蓝桥杯提高】线性代数概率论几何学算法
题目题目链接题解动态规划或矩阵快速幂。动态规划这个方法只能得到78%的分数,无法AC,但确实比较好想。笼统地说一下状态定义和转移方程。dp[i][j]表示从下向上数第i个骰子的上面点数为j的情况下,靠下的i个骰子摆放的全部方案数。(这个定义不准确,后面会说)那么转移方程可以比较容易地写出来了,第i个骰子上面点数为1,对应地其下面点数为4,因此第i个骰子上面点数为1的方案数(即dp[i][1])为第
- 垒骰子---蓝桥杯---矩阵快速幂---C++
Ashen_ffm
C++蓝桥杯矩阵快速幂垒骰子蓝桥杯矩阵快速幂C++快速幂
题目描述:赌圣atm晚年迷恋上了垒骰子,就是把骰子一个垒在另一个上边,不能歪歪扭扭,要垒成方柱体。经过长期观察,atm发现了稳定骰子的奥秘:有些数字的面贴着会互相排斥!我们先来规范一下骰子:1的对面是4,2的对面是5,3的对面是6。假设有m组互斥现象,每组中的那两个数字的面紧贴在一起,骰子就不能稳定的垒起来。atm想计算一下有多少种不同的可能的垒骰子方式。两种垒骰子方式相同,当且仅当这两种方式中对
- 蓝桥杯--2015第六届C/C++B组省赛
小胡同的诗
DPDFS数论LanQiaoOJ蓝桥杯历届省赛题目
相比较14年的难度下降,不过搜索以及DP的题目更多,多了一个树形DP(待补),DP+矩阵快速幂(待补)奖券数目有些人很迷信数字,比如带“4”的数字,认为和“死”谐音,就觉得不吉利。虽然这些说法纯属无稽之谈,但有时还要迎合大众的需求。某抽奖活动的奖券号码是5位数(10000-99999),要求其中不要出现带“4”的号码,主办单位请你计算一下,如果任何两张奖券不重号,最多可发出奖券多少张。请提交该数字
- 蓝桥杯 垒骰子(递归和矩阵快速幂两种算法)
!JianYun!
递归动态规划矩阵与快速幂蓝桥杯算法矩阵
题目:题目描述赌圣atm晚年迷恋上了垒骰子,就是把骰子一个垒在另一个上边,不能歪歪扭扭,要垒成方柱体。经过长期观察,atm发现了稳定骰子的奥秘:有些数字的面贴着会互相排斥!我们先来规范一下骰子:1的对面是4,2的对面是5,3的对面是6。假设有m组互斥现象,每组中的那两个数字的面紧贴在一起,骰子就不能稳定的垒起来。atm想计算一下有多少种不同的可能的垒骰子方式。两种垒骰子方式相同,当且仅当这两种方式
- 矩阵问题入门(矩阵乘法and矩阵快速幂)acm寒假集训日记22/1/15
Joanh_Lan
ACM大一上寒假集训日记矩阵线性代数acm竞赛蓝桥杯c++
今天凌晨3点才睡,没想到通过看小说抑制玩游戏,反而看小说的时间更长。u1s1:那小说太刺激了,晚上看很有感觉,风吹草动我就会猛地看过去(类似茄子说柜子动了,哈哈),真TM(语气词)练胆量!!!..QvQ..接下来就是正题了!矩阵乘法说真的,一开始没有接触过这东西的我是懵逼的!矩阵乘法的条件:只有两个矩阵类:A(x*y)andB(y*z)才可以矩阵相乘,用人话来说:第一个矩阵的列(大小)等于第二个矩
- 【牛客】几何糕手、国际裁判带师、数位dp?、灵异背包、矩阵快速幂签到、第一次放学
想要AC的sjh
练习题(记录做题想法)矩阵算法c++牛客
文章目录《几何糕手》题目描述思路代码《国际裁判带师》题目描述思路代码《数位dp?》题目描述思路代码《灵异背包》题目描述思路代码《矩阵快速幂签到》题目描述思路代码《第一次放学》题目描述思路代码《几何糕手》题目链接题目描述“芝士肾么?”地上有一根木桩,在木桩上栓有一根长度为a的木绳,木绳的末端还栓有一根长度为b的木棍,现在小沙想要知道,木棍可能扫过的位置在地面上的投影面积有多大。输入描述第一行输入两个
- 【动态规划】【记忆化搜索】【C++算法】664. 奇怪的打印机
闻缺陷则喜何志丹
#算法题算法动态规划c++leetcode记忆化搜索打印机
作者推荐【矩阵快速幂】封装类及测试用例及样例本文涉及知识点动态规划记忆化搜索字符串LeetCode:664奇怪的打印机有台奇怪的打印机有以下两个特殊要求:打印机每次只能打印由同一个字符组成的序列。每次可以在从起始到结束的任意位置打印新字符,并且会覆盖掉原来已有的字符。给你一个字符串s,你的任务是计算这个打印机打印它需要的最少打印次数。示例1:输入:s=“aaabbb”输出:2解释:首先打印“aaa
- 【动态规划】【C++算法】639 解码方法 II
闻缺陷则喜何志丹
#算法题算法动态规划c++leetcode编码解码通配符
作者推荐【矩阵快速幂】封装类及测试用例及样例涉及知识点动态规划字符串滚动向量LeetCode639.解码方法II一条包含字母A-Z的消息通过以下的方式进行了编码:‘A’->“1”‘B’->“2”…‘Z’->“26”要解码一条已编码的消息,所有的数字都必须分组,然后按原来的编码方案反向映射回字母(可能存在多种方式)。例如,“11106”可以映射为:“AAJF”对应分组(11106)“KJF”对应分组
- 【数位dp】【C++算法】600. 不含连续1的非负整数
闻缺陷则喜何志丹
#算法题c++算法动态规划leetcode数位dp连续1数学
作者推荐【矩阵快速幂】封装类及测试用例及样例涉及知识点数位dpLeetCode600.不含连续1的非负整数给定一个正整数n,请你统计在[0,n]范围的非负整数中,有多少个整数的二进制表示中不存在连续的1。示例1:输入:n=5输出:5解释:下面列出范围在[0,5]的非负整数与其对应的二进制表示:0:01:12:103:114:1005:101其中,只有整数3违反规则(有两个连续的1),其他5个满足规
- 【动态规划】【滑动窗口】【C++算法】 629K 个逆序对数组
闻缺陷则喜何志丹
#算法题算法动态规划c++数学数论滑动窗口数对
作者推荐【矩阵快速幂】封装类及测试用例及样例本文涉及知识点动态规划C++算法:滑动窗口总结LeetCode629:K个逆序对数组逆序对的定义如下:对于数组nums的第i个和第j个元素,如果满足0nums[j],则其为一个逆序对;否则不是。给你两个整数n和k,找出所有包含从1到n的数字,且恰好拥有k个逆序对的不同的数组的个数。由于答案可能很大,只需要返回对109+7取余的结果。示例1:输入:n=3,
- 【KMP】【二分查找】【C++算法】100207. 找出数组中的美丽下标 II
闻缺陷则喜何志丹
c++算法leetcodeKMP字符串二分查找美丽下标
作者推荐【矩阵快速幂】封装类及测试用例及样例本文涉及的基础知识点二分查找算法合集LeetCode100207.找出数组中的美丽下标II给你一个下标从0开始的字符串s、字符串a、字符串b和一个整数k。如果下标i满足以下条件,则认为它是一个美丽下标:0m_vSameLen;//m_vSame[i]记录s[i...]和t[0...]最长公共前缀,增加可调试性protected:voidCalLen(co
- 【矩阵快速幂】封装类及测试用例及样例
闻缺陷则喜何志丹
#算法基础数据结构与算法矩阵线性代数c++动态规划算法矩阵乘法
作者推荐视频算法专题通俗的说,就是矩阵的乘方。封装类核心代码classCMat{public://矩阵乘法staticvector>multiply(constvector>&a,constvector>&b){constintr=a.size(),c=b.front().size(),iK=a.front().size();assert(iK==b.size());vector>ret(r,ve
- 【动态规划】【矩阵快速幂】【滚动向量】C++算法552. 学生出勤记录 II
闻缺陷则喜何志丹
#算法题数据结构与算法算法动态规划矩阵c++leetcode滚动向量出勤
作者推荐【动态规划】458:可怜的小猪本题其它解法【矩阵快速幂】封装类及测试用例及样例预计2024年1月15(周一7:00)发布涉及知识点动态规划矩阵快速幂滚动向量LeetCode552.学生出勤记录II可以用字符串表示一个学生的出勤记录,其中的每个字符用来标记当天的出勤情况(缺勤、迟到、到场)。记录中只含下面三种字符:‘A’:Absent,缺勤‘L’:Late,迟到‘P’:Present,到场如
- POJ 3233 Matrix Power Series (矩阵快速幂+二分)
_TCgogogo_
数论二分/三分/两点法POJ矩阵快速幂二分
MatrixPowerSeriesTimeLimit:3000MSMemoryLimit:131072KTotalSubmissions:16403Accepted:6980DescriptionGivenan×nmatrixAandapositiveintegerk,findthesumS=A+A2+A3+…+Ak.InputTheinputcontainsexactlyonetestcase.
- Java程序员面试需要注意啥?面试常见手撕模板题以及笔试模板总结
Java_苏先生
一.目录排序二分二叉树非递归遍历01背包最长递增子序列最长公共子序列最长公共子串大数加法大数乘法大数阶乘全排列子集N皇后并查集树状数组线段树字典树单调栈单调队列KMPManacher算法拓扑排序最小生成树最短路欧拉回路GCD和LCM素数筛法唯一分解定理乘法快速幂矩阵快速幂二.面试常见手撕模板题以及笔试模板总结0.Java快速输入先给一个干货,可能有些题用Java会超时(很少),下面是Petr刷题时
- c++矩阵——超实用的数据结构
yzc_qiuse
c++c++矩阵数据结构
文章目录C++中的矩阵介绍什么是矩阵?C++中的矩阵表示矩阵的运算矩阵加法矩阵减法矩阵乘法矩阵转置这里是一道模板题:矩阵快速幂总结结语C++中的矩阵介绍什么是矩阵?矩阵是一个二维的数学结构,由行和列组成。在C++中,我们可以使用数组或者向量来表示矩阵。矩阵广泛应用于线性代数、图像处理、机器学习等领域。C++中的矩阵表示在C++中,我们可以使用数组来表示矩阵。例如,一个3×33×33×3的矩阵可以表
- 矩阵快速幂&斐波那契数列
yy代码
矩阵算法线性代数
矩阵快速幂&斐波那契数列矩阵快速幂:快速地求出斐波那契数列中的每一项可以快速地求出斐波那契数列的前n项的和首先我们来看如何快速地求出斐波那契数列的第n项1.快速求斐波那契数列的某一项设Fn=[fn,fn+1]F_n=[f_n,f_{n+1}]Fn=[fn,fn+1],构造这一个行向量,那么对于此,我们思考FnF_nFn乘一个什么样的矩阵可以得到Fn+1F_{n+1}Fn+1显然:可以乘一个这样子的
- hdu1005 矩阵快速幂
没天赋的学琴
题目NumberSequenceProblemDescriptionAnumbersequenceisdefinedasfollows:f(1)=1,f(2)=1,f(n)=(A*f(n-1)+B*f(n-2))mod7.GivenA,B,andn,youaretocalculatethevalueoff(n).InputTheinputconsistsofmultipletestcases.Ea
- 数论专题(寒假Day 5)
叔丁基锂_
Day5数论一些定义和性质,只有种取值数论函数:定义域为正整数,陪域为复数的函数。我们主要研究定义域为正整数,值域为整数的函数。积性函数:满足若a,b互质,则的数论函数称为积性函数。完全积性函数:满足的数论函数称为完全积性函数狄利克雷卷积:对于数论函数,定义其狄利克雷卷积两个积性函数的狄利克雷卷积仍为积性函数一些常见的积性函数单位函数常函数幂函数欧拉函数代表[1,x]中与x互质的个数=莫比乌斯函数
- 矩阵快速幂及应用实战[C/C++]
EQUINOX1
矩阵c语言c++数据结构算法线性代数
矩阵快速幂矩阵快速幂可以用来优化递推问题,如状态机DP,需要一丢丢线性代数里面矩阵的概念,只需要知道简单的矩阵乘法,结合我们普通的二分快速幂就能很快的掌握矩阵快速幂。问题引入三步问题。有个小孩正在上楼梯,楼梯有n阶台阶,小孩一次可以上1阶、2阶或3阶。实现一种方法,计算小孩有多少种上楼梯的方式。结果可能很大,你需要对结果模1000000007。对于这种递推入门题目,相信只要对于编程有着一定了解的人
- 机试练习Day6-有深度的题目--真题
一只天蝎
编程语言---C语言c++数据结构算法
目录矩阵快速幂快速幂算法代码部分复数的集合优先队列运算符重载结构体构造函数代码部分矩阵快速幂快速幂算法这个道理和转二进制很像:例如:现在要求3的9次方,最关键的是如何表示9,我们可以选择让3乘9次,也就是3*3*3*3*3*3*3*3*3;但是如果是10000000000次方就会让效率很低,现在可以考虑用二进制表示9,也就是1001,刚开始K=9是奇数,那么转成二进制后,最后一位一定是1,只要在大
- 用MiddleGenIDE工具生成hibernate的POJO(根据数据表生成POJO类)
AdyZhang
POJOeclipseHibernateMiddleGenIDE
推荐:MiddlegenIDE插件, 是一个Eclipse 插件. 用它可以直接连接到数据库, 根据表按照一定的HIBERNATE规则作出BEAN和对应的XML ,用完后你可以手动删除它加载的JAR包和XML文件! 今天开始试着使用
- .9.png
Cb123456
android
“点九”是andriod平台的应用软件开发里的一种特殊的图片形式,文件扩展名为:.9.png
智能手机中有自动横屏的功能,同一幅界面会在随着手机(或平板电脑)中的方向传感器的参数不同而改变显示的方向,在界面改变方向后,界面上的图形会因为长宽的变化而产生拉伸,造成图形的失真变形。
我们都知道android平台有多种不同的分辨率,很多控件的切图文件在被放大拉伸后,边
- 算法的效率
天子之骄
算法效率复杂度最坏情况运行时间大O阶平均情况运行时间
算法的效率
效率是速度和空间消耗的度量。集中考虑程序的速度,也称运行时间或执行时间,用复杂度的阶(O)这一标准来衡量。空间的消耗或需求也可以用大O表示,而且它总是小于或等于时间需求。
以下是我的学习笔记:
1.求值与霍纳法则,即为秦九韶公式。
2.测定运行时间的最可靠方法是计数对运行时间有贡献的基本操作的执行次数。运行时间与这个计数成正比。
- java数据结构
何必如此
java数据结构
Java 数据结构
Java工具包提供了强大的数据结构。在Java中的数据结构主要包括以下几种接口和类:
枚举(Enumeration)
位集合(BitSet)
向量(Vector)
栈(Stack)
字典(Dictionary)
哈希表(Hashtable)
属性(Properties)
以上这些类是传统遗留的,在Java2中引入了一种新的框架-集合框架(Collect
- MybatisHelloWorld
3213213333332132
//测试入口TestMyBatis
package com.base.helloworld.test;
import java.io.IOException;
import org.apache.ibatis.io.Resources;
import org.apache.ibatis.session.SqlSession;
import org.apache.ibat
- Java|urlrewrite|URL重写|多个参数
7454103
javaxmlWeb工作
个人工作经验! 如有不当之处,敬请指点
1.0 web -info 目录下建立 urlrewrite.xml 文件 类似如下:
<?xml version="1.0" encoding="UTF-8" ?>
<!DOCTYPE u
- 达梦数据库+ibatis
darkranger
sqlmysqlibatisSQL Server
--插入数据方面
如果您需要数据库自增...
那么在插入的时候不需要指定自增列.
如果想自己指定ID列的值, 那么要设置
set identity_insert 数据库名.模式名.表名;
----然后插入数据;
example:
create table zhabei.test(
id bigint identity(1,1) primary key,
nam
- XML 解析 四种方式
aijuans
android
XML现在已经成为一种通用的数据交换格式,平台的无关性使得很多场合都需要用到XML。本文将详细介绍用Java解析XML的四种方法。
XML现在已经成为一种通用的数据交换格式,它的平台无关性,语言无关性,系统无关性,给数据集成与交互带来了极大的方便。对于XML本身的语法知识与技术细节,需要阅读相关的技术文献,这里面包括的内容有DOM(Document Object
- spring中配置文件占位符的使用
avords
1.类
<?xml version="1.0" encoding="UTF-8"?><!DOCTYPE beans PUBLIC "-//SPRING//DTD BEAN//EN" "http://www.springframework.o
- 前端工程化-公共模块的依赖和常用的工作流
bee1314
webpack
题记: 一个人的项目,还有工程化的问题嘛? 我们在推进模块化和组件化的过程中,肯定会不断的沉淀出我们项目的模块和组件。对于这些沉淀出的模块和组件怎么管理?另外怎么依赖也是个问题? 你真的想这样嘛? var BreadCrumb = require(‘../../../../uikit/breadcrumb’); //真心ugly。
- 上司说「看你每天准时下班就知道你工作量不饱和」,该如何回应?
bijian1013
项目管理沟通IT职业规划
问题:上司说「看你每天准时下班就知道你工作量不饱和」,如何回应
正常下班时间6点,只要是6点半前下班的,上司都认为没有加班。
Eno-Bea回答,注重感受,不一定是别人的
虽然我不知道你具体从事什么工作与职业,但是我大概猜测,你是从事一项不太容易出现阶段性成果的工作
- TortoiseSVN,过滤文件
征客丶
SVN
环境:
TortoiseSVN 1.8
配置:
在文件夹空白处右键
选择 TortoiseSVN -> Settings
在 Global ignote pattern 中添加要过滤的文件:
多类型用英文空格分开
*name : 过滤所有名称为 name 的文件或文件夹
*.name : 过滤所有后缀为 name 的文件或文件夹
--------
- 【Flume二】HDFS sink细说
bit1129
Flume
1. Flume配置
a1.sources=r1
a1.channels=c1
a1.sinks=k1
###Flume负责启动44444端口
a1.sources.r1.type=avro
a1.sources.r1.bind=0.0.0.0
a1.sources.r1.port=44444
a1.sources.r1.chan
- The Eight Myths of Erlang Performance
bookjovi
erlang
erlang有一篇guide很有意思: http://www.erlang.org/doc/efficiency_guide
里面有个The Eight Myths of Erlang Performance: http://www.erlang.org/doc/efficiency_guide/myths.html
Myth: Funs are sl
- java多线程网络传输文件(非同步)-2008-08-17
ljy325
java多线程socket
利用 Socket 套接字进行面向连接通信的编程。客户端读取本地文件并发送;服务器接收文件并保存到本地文件系统中。
使用说明:请将TransferClient, TransferServer, TempFile三个类编译,他们的类包是FileServer.
客户端:
修改TransferClient: serPort, serIP, filePath, blockNum,的值来符合您机器的系
- 读《研磨设计模式》-代码笔记-模板方法模式
bylijinnan
java设计模式
声明: 本文只为方便我个人查阅和理解,详细的分析以及源代码请移步 原作者的博客http://chjavach.iteye.com/
import java.sql.Connection;
import java.sql.DriverManager;
import java.sql.PreparedStatement;
import java.sql.ResultSet;
- 配置心得
chenyu19891124
配置
时间就这样不知不觉的走过了一个春夏秋冬,转眼间来公司已经一年了,感觉时间过的很快,时间老人总是这样不停走,从来没停歇过。
作为一名新手的配置管理员,刚开始真的是对配置管理是一点不懂,就只听说咱们公司配置主要是负责升级,而具体该怎么做却一点都不了解。经过老员工的一点点讲解,慢慢的对配置有了初步了解,对自己所在的岗位也慢慢的了解。
做了一年的配置管理给自总结下:
1.改变
从一个以前对配置毫无
- 对“带条件选择的并行汇聚路由问题”的再思考
comsci
算法工作软件测试嵌入式领域模型
2008年上半年,我在设计并开发基于”JWFD流程系统“的商业化改进型引擎的时候,由于采用了新的嵌入式公式模块而导致出现“带条件选择的并行汇聚路由问题”(请参考2009-02-27博文),当时对这个问题的解决办法是采用基于拓扑结构的处理思想,对汇聚点的实际前驱分支节点通过算法预测出来,然后进行处理,简单的说就是找到造成这个汇聚模型的分支起点,对这个起始分支节点实际走的路径数进行计算,然后把这个实际
- Oracle 10g 的clusterware 32位 下载地址
daizj
oracle
Oracle 10g 的clusterware 32位 下载地址
http://pan.baidu.com/share/link?shareid=531580&uk=421021908
http://pan.baidu.com/share/link?shareid=137223&uk=321552738
http://pan.baidu.com/share/l
- 非常好的介绍:Linux定时执行工具cron
dongwei_6688
linux
Linux经过十多年的发展,很多用户都很了解Linux了,这里介绍一下Linux下cron的理解,和大家讨论讨论。cron是一个Linux 定时执行工具,可以在无需人工干预的情况下运行作业,本文档不讲cron实现原理,主要讲一下Linux定时执行工具cron的具体使用及简单介绍。
新增调度任务推荐使用crontab -e命令添加自定义的任务(编辑的是/var/spool/cron下对应用户的cr
- Yii assets目录生成及修改
dcj3sjt126com
yii
assets的作用是方便模块化,插件化的,一般来说出于安全原因不允许通过url访问protected下面的文件,但是我们又希望将module单独出来,所以需要使用发布,即将一个目录下的文件复制一份到assets下面方便通过url访问。
assets设置对应的方法位置 \framework\web\CAssetManager.php
assets配置方法 在m
- mac工作软件推荐
dcj3sjt126com
mac
mac上的Terminal + bash + screen组合现在已经非常好用了,但是还是经不起iterm+zsh+tmux的冲击。在同事的强烈推荐下,趁着升级mac系统的机会,顺便也切换到iterm+zsh+tmux的环境下了。
我为什么要要iterm2
切换过来也是脑袋一热的冲动,我也调查过一些资料,看了下iterm的一些优点:
* 兼容性好,远程服务器 vi 什么的低版本能很好兼
- Memcached(三)、封装Memcached和Ehcache
frank1234
memcachedehcachespring ioc
本文对Ehcache和Memcached进行了简单的封装,这样对于客户端程序无需了解ehcache和memcached的差异,仅需要配置缓存的Provider类就可以在二者之间进行切换,Provider实现类通过Spring IoC注入。
cache.xml
<?xml version="1.0" encoding="UTF-8"?>
- Remove Duplicates from Sorted List II
hcx2013
remove
Given a sorted linked list, delete all nodes that have duplicate numbers, leaving only distinct numbers from the original list.
For example,Given 1->2->3->3->4->4->5,
- Spring4新特性——注解、脚本、任务、MVC等其他特性改进
jinnianshilongnian
spring4
Spring4新特性——泛型限定式依赖注入
Spring4新特性——核心容器的其他改进
Spring4新特性——Web开发的增强
Spring4新特性——集成Bean Validation 1.1(JSR-349)到SpringMVC
Spring4新特性——Groovy Bean定义DSL
Spring4新特性——更好的Java泛型操作API
Spring4新
- MySQL安装文档
liyong0802
mysql
工作中用到的MySQL可能安装在两种操作系统中,即Windows系统和Linux系统。以Linux系统中情况居多。
安装在Windows系统时与其它Windows应用程序相同按照安装向导一直下一步就即,这里就不具体介绍,本文档只介绍Linux系统下MySQL的安装步骤。
Linux系统下安装MySQL分为三种:RPM包安装、二进制包安装和源码包安装。二
- 使用VS2010构建HotSpot工程
p2p2500
HotSpotOpenJDKVS2010
1. 下载OpenJDK7的源码:
http://download.java.net/openjdk/jdk7
http://download.java.net/openjdk/
2. 环境配置
▶
- Oracle实用功能之分组后列合并
seandeng888
oracle分组实用功能合并
1 实例解析
由于业务需求需要对表中的数据进行分组后进行合并的处理,鉴于Oracle10g没有现成的函数实现该功能,且该功能如若用JAVA代码实现会比较复杂,因此,特将SQL语言的实现方式分享出来,希望对大家有所帮助。如下:
表test 数据如下:
ID,SUBJECTCODE,DIMCODE,VALUE
1&nbs
- Java定时任务注解方式实现
tuoni
javaspringjvmxmljni
Spring 注解的定时任务,有如下两种方式:
第一种:
<?xml version="1.0" encoding="UTF-8"?>
<beans xmlns="http://www.springframework.org/schema/beans"
xmlns:xsi="http
- 11大Java开源中文分词器的使用方法和分词效果对比
yangshangchuan
word分词器ansj分词器Stanford分词器FudanNLP分词器HanLP分词器
本文的目标有两个:
1、学会使用11大Java开源中文分词器
2、对比分析11大Java开源中文分词器的分词效果
本文给出了11大Java开源中文分词的使用方法以及分词结果对比代码,至于效果哪个好,那要用的人结合自己的应用场景自己来判断。
11大Java开源中文分词器,不同的分词器有不同的用法,定义的接口也不一样,我们先定义一个统一的接口:
/**
* 获取文本的所有分词结果, 对比

 . Now, you need to write a program to calculate the number of the N-th cow in order to check if John’s cows can make it right.
. Now, you need to write a program to calculate the number of the N-th cow in order to check if John’s cows can make it right.

 as described above.
as described above.